Global dynamics of a Nonlocal Periodic Reaction–Diffusion Model of Chikungunya Disease

Abstract

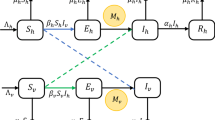
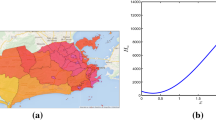
This paper is devoted to the study of a nonlocal reaction–diffusion model of Chikungunya disease with periodic time delays. We establish two threshold type results on the global dynamics for the growth of mosquitoes and the disease transmission, respectively. Further, we obtain the global attractivity of a positive steady state for a simplified nonlocal and time-delayed system with constant coefficients. We also conduct numerical simulations for the Chikungunya transmission in Ceará, Brazil to investigate the effects of spatial heterogeneity on the disease transmission.

Appendix

Proof of Theorem 4.10

From the analysis on the ultimate boundedness for system (2.4), we see that the set

$$\begin{aligned} \begin{aligned} H&: = \left\{ \phi \in U:\phi _1 (\theta _1,x) \leqslant \frac{{\Lambda _h }}{{\mu _h }},\phi _2 (\theta _2,x) \leqslant \frac{{\Lambda _h }}{{\mu _h }},\right. \\&\quad \phi _3 (0,x) \leqslant K_d,\phi _4 (\theta _3,x) \leqslant \frac{{k_d K_d }}{{\eta _w + \mu _E }}, \\&\quad \phi _5 (\theta _2,x) \leqslant \frac{{e^{ - \mu _L \tau _w } \eta _w \left( {\frac{{k_d K_d }}{{\eta _w + \mu _E }}} \right) }}{{\mu _m }},\phi _6 (\theta _1,x) \leqslant \frac{{e^{ - \mu _L \tau _w } \eta _w \left( {\frac{{k_d K_d }}{{\eta _w + \mu _E }}} \right) }}{{\mu _m }}, \\&\quad \left. \forall \theta _1 \in [ - \tau _h,0],\forall \theta _2 \in [ - \tau _m,0],\forall \theta _3 \in [ - \tau _w,0],x \in {{\bar{\Omega }}} \right\} \\ \end{aligned} \end{aligned}$$

is positively invariant for the solution semiflow Q(t) of system (4.8), and every forward orbit of system (4.8) from U enters H eventually. Therefore, it suffices to study the dynamics of system (4.8) on H. Note that cases (i) and (ii) are the straightforward consequences of Theorem 4.8 (i) and (ii) with $\rho = 0$. It remains to prove (iii). When ${\mathscr {R}}_m >1$ and ${\mathscr {R}}_0>1$, it follows from Theorem 4.8 (iii) that system (4.8) is uniformly persistent, that is, there exists $\xi > 0$ such that for any $\phi = \left( {\phi _1,\phi _2,\phi _3,\phi _4,\phi _5,\phi _6 } \right) \in H$ with $\phi _2(0,\cdot )\not \equiv 0$ or $\phi _6(0,\cdot )\not \equiv 0$, the solution $u=(t,x,\phi )$ satisfies

$$\begin{aligned} \mathop {\lim \inf }\limits _{t \rightarrow \infty } \mathop {\min }\limits _{x \in {{\overline{\Omega }}} } u_i (t,x,\phi ) \geqslant \xi ,(1 \leqslant i \leqslant 6). \end{aligned}$$

(6.1)

Let $H_0: = \left\{ {\phi \in H:\phi _i (0,x) > 0,\forall x \in {{\bar{\Omega }}},i = 1,2,3,4,5,6} \right\} \!.$ Next, we show that the system (4.8) is globally attractive by the method of Lyapunov functionals. Set $f(u) = u - 1 - \ln u,u \in (0,\infty )$. Clearly, $f(u) \geqslant 0$ for all $u \in (0,\infty )$ and $\min _{0< u < + \infty } f(u) = f(1) = 0$. Define a continuous functional $V:H_0 \rightarrow {\mathbb {R}}$:

$$\begin{aligned} V(\phi ) = \int \limits _\Omega {\left[ {V_1 (x,\phi ) + V_2 (x,\phi )} \right] } dx, \end{aligned}$$

where

$$\begin{aligned} \begin{aligned} V_1&= \frac{1}{{\beta _h I_{m*} }}f\left( {\frac{{\phi _1 (0,x)}}{{S_{h*} }}} \right) + \frac{{I_{h*} }}{{e^{ - \mu _h \tau _h } \beta _h I_{m*} S_{h*} }}\left( {f\left( {\frac{{\phi _2 (0,x)}}{{I_{h*} }}} \right) } \right) \\&\quad + \int \limits _{ - \tau _h }^0 {\int \limits _\Omega {\Gamma \left( {D( - s),x,y} \right) f\left( {\frac{{\phi _6 (s,y)\phi _1 (s,y)}}{{I_{m*} S_{h*} }}} \right) dyds} }, \end{aligned} \end{aligned}$$

and

$$\begin{aligned} \begin{aligned} V_2&= \frac{1}{{k_d }}f\left( {\frac{{\phi _3 (0,x)}}{{E_{d*} }}} \right) + \frac{{E_{w*} }}{{k_d E_{d*} }}f\left( {\frac{{\phi _4 (0,x)}}{{E_{w*} }}} \right) + \frac{1}{{\eta _w }}f\left( {\frac{{\phi _4 (0,x)}}{{E_{w*} }}} \right) \\&\quad + \frac{{S_{m*} }}{{e^{ - \mu _L \tau _w } \eta _w E_{w*} }}f\left( {\frac{{\phi _5 (0,x)}}{{S_{m*} }}} \right) + \int \limits _{ - \tau _w }^0 {\int \limits _\Omega {\Gamma \left( {D( - s),x,y} \right) f\left( {\frac{{\phi _4 (s,y)}}{{E_{w*} }}} \right) dyds} } \\&\quad + \frac{1}{{\beta _m I_{h*} }}f\left( {\frac{{\phi _5 (0,x)}}{{S_{m*} }}} \right) + \frac{{I_{m*} }}{{e^{ - \mu _m \tau _m } \beta _m I_{h*} S_{m*} }}f\left( {\frac{{\phi _6 (0,x)}}{{I_{m*} }}} \right) \\&\quad + \int \limits _{ - \tau _m }^0 {\int \limits _\Omega {\Gamma \left( {D( - s),x,y} \right) f\left( {\frac{{\phi _2 (s,y)\phi _4 (s,y)}}{{I_{h*} S_{m*} }}} \right) dyds} }. \end{aligned} \end{aligned}$$

Next we fix $\phi = \left( {\phi _1,\phi _2,\phi _3,\phi _4,\phi _5,\phi _6 } \right) \in H$ with $\phi _2(0,\cdot )\not \equiv 0$ or $\phi _6(0,\cdot )\not \equiv 0$. From (6.1), without loss of generality, we can assume that $u_t (\phi ) \in H_0,\forall t \geqslant 0$. Let $\omega (\phi )$ be the omega limit set for the semiflow Q(t). Then $\omega (\phi ) \subset H_0$. Now we calculate the time derivative of $V(u_t(\phi ))$ along the solution of system (4.8). It then follows that

$$\begin{aligned} \begin{aligned} \frac{{\partial V_1 }}{{\partial t}}&= \frac{1}{{\beta _h I_{m*} }}\left( {1 - \frac{{S_{h*} }}{{S_h }}} \right) \frac{1}{{S_{h*} }}\frac{{\partial S_h }}{{\partial t}} + \frac{{I_{h*} }}{{e^{ - \mu _h \tau _h } \beta _h I_{m*} S_{h*} }}\left( {1 - \frac{{I_{h*} }}{{I_h }}} \right) \frac{1}{{I_{h*} }}\frac{{\partial I_h }}{{\partial t}} \\&\quad + \left[ {f\left( {\frac{{I_m (t,x)S_h (t,x)}}{{I_{m*} S_{h*} }}} \right) - \int \limits _\Omega {\Gamma \left( {D\tau _h,x,y} \right) f\left( {\frac{{I_m (t - \tau _h,y)S_h (t - \tau _h,y)}}{{I_{m*} S_{h*} }}} \right) dy} } \right] \\&= - \frac{{\mu _h \left( {S_h - S_{h*} } \right) ^2 }}{{\beta _h I_{m*} S_{h*} S_h }} + \frac{1}{{I_{m*} S_{h*} }}\left( {1 - \frac{{S_{h*} }}{{S_h }}} \right) \left( {I_{m*} S_{h*} - I_m S_h } \right) \\&\quad + \frac{1}{{\beta _h I_{m*} }}\left( {1 - \frac{{S_{h*} }}{{S_h }}} \right) \frac{1}{{S_{h*} }}D_h \Delta S_h + \frac{{I_{h*} }}{{e^{ - \mu _h \tau _h } \beta _h I_{m*} S_{h*} }}\left( {1 - \frac{{I_{h*} }}{{I_h }}} \right) \frac{1}{{I_{h*} }}D_h \Delta I_h \\&\quad + \left( {\int \limits _\Omega {\Gamma \left( {D\tau _h,x,y} \right) \frac{{I_m (t - \tau _h,y)S_h (t - \tau _h,y)}}{{I_{m*} S_{h*} }}dy} - \frac{{I_h }}{{I_{h*} }}} \right) \\&\quad \left( {1 - \frac{{I_{h*} }}{{I_h }}} \right) + \frac{{S_h I_m }}{{S_{h*} I_{m*} }} - \ln \frac{{S_h I_m }}{{S_{h*} I_{m*} }} \\&\quad - \int \limits _\Omega {\Gamma \left( {D\tau _h,x,y} \right) \left( {\frac{{I_m (t - \tau _h,y)S_h (t - \tau _h,y)}}{{I_{m*} S_{h*} }} - \ln \frac{{I_m (t - \tau _h,y)S_h (t - \tau _h,y)}}{{I_{m*} S_{h*} }}} \right) dy} \\&= - \frac{{\mu _h \left( {S_h - S_{h*} } \right) ^2 }}{{\beta _h I_{m*} S_{h*} S_h }} + \frac{1}{{\beta _h I_{m*} }}\left( {1 - \frac{{S_{h*} }}{{S_h }}} \right) \frac{1}{{S_{h*} }}D_h \Delta S_h\\&\quad + \frac{{I_{h*} }}{{e^{ - \mu _h \tau _h } \beta _h I_{m*} S_{h*} }}\left( {1 - \frac{{I_{h*} }}{{I_h }}} \right) \frac{1}{{I_{h*} }}D_h \Delta I_h \\&\quad - f\left( {\frac{{S_{h*} }}{{S_h }}} \right) + \frac{{I_m }}{{I_{m*} }} - \frac{{I_h }}{{I_{h*} }} - \ln \frac{{I_{h*} }}{{I_h}} \\&\quad - \ln \frac{{I_m }}{{I_{m*} }} - \int \limits _\Omega {\Gamma \left( {D\tau _h,x,y} \right) f\left( {\frac{{I_m (t - \tau _h,y)S_h (t - \tau _h,y)I_{h*} }}{{I_{m*} S_{h*} I_h }}} \right) dy}, \end{aligned} \end{aligned}$$

and

$$\begin{aligned} \frac{{\partial V_2 }}{{\partial t}}= & {} \frac{1}{{k_d }}\left( {1 - \frac{{E_{d*} }}{{E_d }}} \right) \frac{1}{{E_{d*} }}\frac{{\partial E_d }}{{\partial t}} + \frac{{E_{w*} }}{{k_d E_{d*} }}\left( {1 - \frac{{E_{w*} }}{{E_w }}} \right) \frac{1}{{E_{w*} }}\frac{{\partial E_w }}{{\partial t}} \\{} & {} + \frac{1}{{\eta _w }}\left( {1 - \frac{{E_{w*} }}{{E_w }}} \right) \frac{1}{{E_{w*} }}\frac{{\partial E_w }}{{\partial t}} + \frac{{S_{m*} }}{{e^{ - \mu _L \tau _w } \eta _w E_{w*} }}\left( {1 - \frac{{S_{m*} }}{{S_m }}} \right) \frac{1}{{S_{m*} }}\frac{{\partial S_m }}{{\partial t}} \\{} & {} + \left[ {f\left( {\frac{{E_w (t,x)}}{{E_{w*} }}} \right) - \int \limits _\Omega {\Gamma \left( {D\tau _w,x,y} \right) f\left( {\frac{{E_w (t - \tau _w,y)}}{{E_{w*} }}} \right) dy} } \right] \\{} & {} + \frac{1}{{\beta _m I_{h*} }}\left( {1 - \frac{{S_{m*} }}{{S_m }}} \right) \frac{1}{{S_{m*} }}\frac{{\partial S_m }}{{\partial t}} + \frac{{I_{m*} }}{{e^{ - \mu _m \tau _m } \beta _m I_{h*} S_{m*} }}\left( {1 - \frac{{I_{m*} }}{{I_m }}} \right) \frac{1}{{I_{m*} }}\frac{{\partial I_m }}{{\partial t}} \\{} & {} + \left[ {f\left( {\frac{{I_h (t,x)S_m (t,x)}}{{I_{h*} S_{m*} }}} \right) } \right. \left. - \int \limits _\Omega {\Gamma \left( {D\tau _m,x,y} \right) f\left( {\frac{{I_h (t - \tau _m,y)S_m (t - \tau _m,y)}}{{I_{h*} S_{m*} }}} \right) dy} \right] \\= & {} - \frac{{\mu _E \left( {E_d - E_{d*} } \right) ^2 }}{{k_d E_{d*} E_d }} + \frac{1}{{E_{d*} }}\left( {1 - \frac{{E_{d*} }}{{E_d }}} \right) \left( {E_{d*} - E_d } \right) \\{} & {} + \frac{1}{{E_{d*} }}\left( {1 - \frac{{E_{w*} }}{{E_w }}} \right) \left( {E_d - \frac{{E_{d*}}}{{E_{w*} }}E_w } \right) \\{} & {} - \frac{{\left( {\eta _w + \mu _E } \right) \left( {E_w - E_{w*} } \right) ^2 }}{{\eta _w E_{w*} E_w }} + \frac{1}{{E_{w*} S_{m*} }}\left( {1 - \frac{{S_{m*} }}{{S_m }}} \right) \left( {E_{w*} S_{m*} - E_w S_m } \right) \\{} & {} + \frac{{S_{m*} }}{{e^{ - \mu _L \tau _w } \eta _w E_{w*} }}\left( {1 - \frac{{S_{m*} }}{{S_m }}} \right) \frac{1}{{S_{m*} }}D_m \Delta S_m + \frac{1}{{\beta _m I_{h*} }}\left( {1 - \frac{{S_{m*} }}{{S_m }}} \right) \frac{1}{{S_{m*} }}D_m \Delta S_m \\{} & {} + \frac{{I_{m*} }}{{e^{ - \mu _m \tau _m } \beta _m I_{h*} S_{m*} }}\left( {1 - \frac{{I_{m*} }}{{I_m }}} \right) \frac{1}{{I_{m*} }}D_m \Delta I_m \\{} & {} + \left( {\int \limits _\Omega {\Gamma \left( {D\tau _w,x,y} \right) \frac{{E_w (t - \tau _w,y)}}{{E_{w*} }}dy} - \frac{{S_m }}{{S_{m*} }}} \right) \left( {1 - \frac{{S_{m*} }}{{S_m }}} \right) \\{} & {} + \frac{{E_w }}{{E_{w*} }} - \ln \frac{{E_w }}{{E_{w*} }} - \int \limits _\Omega {\Gamma \left( {D\tau _w,x,y} \right) \left( {\frac{{E_w (t - \tau _w,y)}}{{E_{w*} }} - \ln \frac{{E_w (t - \tau _w,y)}}{{E_{w*} }}} \right) dy} \\{} & {} - \frac{{\mu _m \left( {S_m - S_{m*} } \right) ^2 }}{{\beta _m I_{h*} S_{m*} S_m }} + \frac{1}{{I_{h*} S_{m*} }}\left( {1 - \frac{{S_{m*} }}{{S_m }}} \right) \left( {I_{h*} S_{m*} - I_h S_m } \right) \\{} & {} + \left( {\int \limits _\Omega {\Gamma \left( {D\tau _m,x,y} \right) \frac{{I_h (t - \tau _m,y)S_m (t - \tau _m,y)}}{{I_{h*} S_{m*} }}dy} - \frac{{I_m }}{{I_{m*} }}} \right) \\{} & {} \left( {1 - \frac{{I_{m*} }}{{I_m }}} \right) + \frac{{I_h S_m }}{{I_{h*} S_{m*} }} - \ln \frac{{I_h S_m }}{{I_{h*} S_{m*} }} \\{} & {} - \int \limits _\Omega {\Gamma \left( {D\tau _m,x,y} \right) \left( {\frac{{I_h (t - \tau _m,y)S_m (t - \tau _m,y)}}{{I_{h*} S_{m*} }}} \right. } \left. { - \ln \frac{{I_h (t - \tau _m,y)S_m (t - \tau _m,y)}}{{I_{h*} S_{m*} }}} \right) dy \\= & {} - \frac{{\mu _E \left( {E_d - E_{d*} } \right) ^2 }}{{k_d E_{d*} E_d }} + 5 - \frac{{E_{d*} }}{{E_d }} - \frac{{E_w }}{{E_{w*} }} - \frac{{E_{w*} E_d }}{{E_w E_{d*} }} - \frac{{\left( {\eta _w + \mu _E } \right) \left( {E_w - E_{w*} } \right) ^2 }}{{\eta _w E_{w*} E_w }} \\{} & {} + \frac{1}{{e^{ - \mu _L \tau _w } \eta _w E_{w*} }}\left( {1 - \frac{{S_{m*} }}{{S_m }}} \right) D_m \Delta S_m + \frac{1}{{\beta _m I_{h*} S_{m*} }}\left( {1 - \frac{{S_{m*} }}{{S_m }}} \right) D_m \Delta S_m \\{} & {} + \frac{1}{{e^{ - \mu _m \tau _m } \beta _m I_{h*} S_{m*} }}\left( {1 - \frac{{I_{m*} }}{{I_m }}} \right) D_m \Delta I_m - \frac{{S_{m*} }}{{S_m }} + \frac{{E_w }}{{E_{w*} }} - \frac{{S_m }}{{S_{m*} }} - \ln \frac{{E_w }}{{E_{w*} }} - \ln \frac{{S_{m*}}}{{S_m }} \\{} & {} - \int \limits _\Omega {\Gamma \left( {D\tau _w,x,y} \right) f\left( {\frac{{E_w (t - \tau _w,y)S_{m*} }}{{E_{w*} S_m }}} \right) dy} \\{} & {} - \frac{{\mu _m \left( {S_m - S_{m*} } \right) ^2 }}{{\beta _m (A_{h*} + I_{h*} )S_{m*} S_m }} - \frac{{S_{m*} }}{{S_m }} + \frac{{I_h }}{{I_{h*} }} - \frac{{I_m }}{{I_{m*} }} - \ln \frac{{I_h S_m }}{{I_{h*} S_{m*} }} - \ln \frac{{I_{m*} }}{{I_m }} \\{} & {} - \int \limits _\Omega {\Gamma \left( {D\tau _m,x,y} \right) f\left( {\frac{{I_h (t - \tau _m,y)S_m (t - \tau _m,y)I_{m*} }}{{I_{h*} S_{m*} I_m }}} \right) } dy. \end{aligned}$$

Clearly, $\ln v \leqslant \frac{v}{u} + \ln u - 1,\forall u,v > 0$, and hence, $2 - u - \frac{v}{u} + \ln v \leqslant 0$ and $2 - u - \frac{v}{u} + \ln v = 0$ if and only if $u=v=1$. In particular, $2 - u - \frac{1}{u} \leqslant 0$ and $1 - v + \ln v \leqslant 0$. Note that $\int _\Omega {\Delta u} dx = 0$ and $\int _\Omega {\frac{{\Delta u}}{u}} dx = \int _\Omega {\frac{{\left\| {\nabla u} \right\| ^2 }}{{u^2 }}} dx$. Thus, we have

$$\begin{aligned} \frac{{dV\left( {u_t (\phi )} \right) }}{{dt}}= & {} - \int \limits _\Omega {\frac{{\mu _h \left( {S_h - S_{h*} } \right) ^2 }}{{\beta _h I_{m*} S_{h*} S_h }}dx} - \int \limits _\Omega {\frac{{\mu _E \left( {E_d - E_{d*} } \right) ^2 }}{{k_d E_{d*} E_d }}dx} \nonumber \\{} & {} - \int \limits _\Omega {\frac{{\left( {\eta _w + \mu _E } \right) \left( {E_w - E_{w*} } \right) ^2 }}{{\eta _w E_{w*} E_w }}dx} \nonumber \\{} & {} - \int \limits _\Omega {\frac{{\mu _m \left( {S_m - S_{m*} } \right) ^2 }}{{\beta _m (A_{h*} + I_{h*} )S_{m*} S_m }}dx} - \frac{{D_h }}{{\beta _h I_{m*} }}\int \limits _\Omega {\frac{{\left\| {\nabla S_h (t,x)} \right\| ^2 }}{{S_h^2 (t,x)}}dx} \nonumber \\{} & {} - \frac{{I_{h*} D_h }}{{e^{ - \mu _h \tau _h } \beta _h I_{m*} S_{h*} }}\int \limits _\Omega {\frac{{\left\| {\nabla I_h (t,x)} \right\| ^2 }}{{I_h^2 (t,x)}}dx} \nonumber \\{} & {} - \frac{{S_{m*} D_m }}{{e^{ - \mu _L \tau _w } \eta _w E_{w*} }}\int \limits _\Omega {\frac{{\left\| {\nabla S_m (t,x)} \right\| ^2 }}{{S_m^2 (t,x)}}dx} - \frac{{S_{m*} D_m }}{{\beta _m I_{h*} S_{m*} }}\int \limits _\Omega {\frac{{\left\| {\nabla S_m (t,x)} \right\| ^2 }}{{S_m^2 (t,x)}}dx} \nonumber \\{} & {} - \frac{{I_{m*} D_m }}{{e^{ - \mu _m \tau _m } \beta _m I_{h*} S_{m*} }}\int \limits _\Omega {\frac{{\left\| {\nabla I_m (t,x)} \right\| ^2 }}{{I_m^2 (t,x)}}dx} \nonumber \\{} & {} - \int \limits _\Omega {\int \limits _\Omega {\Gamma \left( {D\tau _h,x,y} \right) f\left( {\frac{{I_m (t - \tau _h,y)S_h (t - \tau _h,y)I_{h*} }}{{I_{m*} S_{h*} I_h }}} \right) dy} dx} \nonumber \\{} & {} - \int \limits _\Omega {\int \limits _\Omega {\Gamma \left( {D\tau _w,x,y} \right) f\left( {\frac{{E_w (t - \tau _w,y)S_{m*} }}{{E_{w*} S_m }}} \right) dy} dx} \nonumber \\{} & {} - \int \limits _\Omega {\int \limits _\Omega {\Gamma \left( {D\tau _m,x,y} \right) f\left( {\frac{{I_h (t - \tau _m,y)S_m (t - \tau _m,y)I_{m*} }}{{I_{h*} S_{m*} I_m }}} \right) } dydx} \nonumber \\{} & {} - \int \limits _\Omega {f\left( {\frac{{S_{h*} }}{{S_h }}} \right) dx} + \int \limits _\Omega {\left( {2 - \frac{{E_{d*} }}{{E_d }} - \frac{{E_{w*} E_d }}{{E_w E_{d*} }} - \ln \frac{{E_w }}{{E_{w*} }}} \right) dx} \nonumber \\{} & {} + \int \limits _\Omega {\left( {2 - \frac{{S_{m*} }}{{S_m }} - \frac{{S_m }}{{S_{m*} }}} \right) dx} \nonumber \\{} & {} - \int \limits _\Omega {\frac{{S_{m*} }}{{S_m }}dx} + \int \limits _\Omega {\left( {1 - \frac{{I_{m*} }}{{I_m }} - \ln \frac{{I_m }}{{I_{m*} }}} \right) dx} \nonumber \\\leqslant & {} - \int \limits _\Omega {\frac{{\mu _h \left( {S_h - S_{h*} } \right) ^2 }}{{\beta _h I_{m*} S_{h*} S_h }}dx} - \int \limits _\Omega {\frac{{\mu _E \left( {E_d - E_{d*} } \right) ^2 }}{{k_d E_{d*} E_d }}dx} \nonumber \\{} & {} - \int \limits _\Omega {\frac{{\left( {\eta _w + \mu _E } \right) \left( {E_w - E_{w*} } \right) ^2 }}{{\eta _w E_{w*} E_w }}dx} \nonumber \\{} & {} - \int \limits _\Omega {\frac{{\mu _m \left( {S_m - S_{m*} } \right) ^2 }}{{\beta _m (A_{h*} + I_{h*} )S_{m*} S_m }}dx} - \int \limits _\Omega \int \limits _\Omega \Gamma \left( {D\tau _h,x,y} \right) f\nonumber \\{} & {} \left( {\frac{{I_m (t - \tau _h,y)S_h (t - \tau _h,y)I_{h*} }}{{I_{m*} S_{h*} I_h }}} \right) dy dx \nonumber \\{} & {} - \int \limits _\Omega \int \limits _\Omega \Gamma \left( {D\tau _w,x,y} \right) f \left( {\frac{{E_w (t - \tau _w,y)S_{m*} }}{{E_{w*} S_m }}} \right) dy dx \nonumber \\{} & {} - \int \limits _\Omega {\int \limits _\Omega {\Gamma \left( {D\tau _m,x,y} \right) f\left( {\frac{{I_h (t - \tau _m,y)S_m (t - \tau _m,y)I_{m*} }}{{I_{h*} S_{m*} I_m }}} \right) } dydx} \nonumber \\{} & {} - \int \limits _\Omega {f\left( {\frac{{S_{h*} }}{{S_h }}} \right) dx} + \int \limits _\Omega {\left( {2 - \frac{{E_{d*} }}{{E_d }} - \frac{{E_{w*} E_d }}{{E_w E_{d*} }} - \ln \frac{{E_w }}{{E_{w*} }}} \right) dx} \nonumber \\{} & {} + \int \limits _\Omega {\left( {2 - \frac{{S_{m*} }}{{S_m }} - \frac{{S_m }}{{S_{m*} }}} \right) dx} \nonumber \\{} & {} - \int \limits _\Omega {\frac{{S_{m*} }}{{S_m }}dx} + \int \limits _\Omega {\left( {1 - \frac{{I_{m*} }}{{I_m }} - \ln \frac{{I_m }}{{I_{m*} }}} \right) dx} \nonumber \\&: =&U_\phi (t). \end{aligned}$$

(6.2)

Note that ${V\left( {u_t (\phi )} \right) }$ is nonincreasing and bounded below on $[0,\infty )$, so there is a real number $L \geqslant 0$ such that $\lim _{t \rightarrow \infty } V\left( {u_t (\phi )} \right) = L$. For any $\psi \in \omega (\phi )$, there is a sequence $t_n \rightarrow \infty$ such that $\lim _{n \rightarrow \infty } u_{t_n } (\phi ) = \psi$ in $H_0$. This shows that $V(\psi ) = L,\forall \psi \in \omega (\phi )$. Since $u_t (\psi ) \in \omega (\phi )$, it follows that $V(u_t (\psi )) = L,\forall t \geqslant 0$, and hence, $\frac{{dV(u_t (\psi ))}}{{dt}} = 0$. Replacing $\phi$ in (6.2) with $\psi$, we have $0 = \frac{{dV(u_t (\psi ))}}{{dt}} \leqslant U_\psi (t) \leqslant 0$. This implies that $U_\psi (t) = 0,\forall t \geqslant 0$. Combining with system (4.8), we obtain $u_t (\psi ) = u^*,\forall t \geqslant \max \left\{ {\tau _h,\tau _m,\tau _w } \right\}$. Since $\psi \in \omega (\phi )$ is arbitrary, there also holds $u_t (\omega (\phi )) = u^*,\forall t \geqslant \max \left\{ {\tau _h,\tau _m,\tau _w } \right\}$. In view of the invariance of omega limit sets, it is easy to see that $\omega (\phi ) = u_\tau (\omega (\phi )) = u^*,\tau = \max \left\{ {\tau _h,\tau _m,\tau _w } \right\}$, which implies that $\lim _{t \rightarrow \infty } u_t (\phi ) = u^*$. $\square$