Grain size dependence of strength of nanocrystalline materials as exemplified by copper: an elastic-viscoplastic modelling approach

Abstract

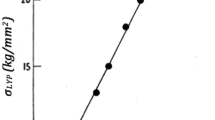
The purpose of this work is to model the mechanical behavior of nanocrystalline materials. Based on previous rigid viscoplastic models proposed by Kim et al. (Acta Mater, 48: 493, 2000) and Kim and Estrin (Acta Mater, 53: 765, 2005), the nanocrystalline material is described as a two phase composite material. Using the Taylor–Lin homogenisation scheme in order to account for elasticity, the yield stress of nanocrystalline materials can be evaluated. The transition from a Hall–Petch relation to an inverse Hall–Petch relation is defined and is related to a change in plastic deformation mode in the crystallite phase from a dislocation glide driven mechanism to a diffusion-controlled process.

Appendices

Appendix A: Relation between the transition grain size d _tr and loading rate D _o

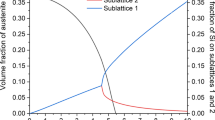
The transition from the Hall–Petch to an inverse Hall–Petch behavior with a decrease in the grain size is due to a change in the plastic deformation mechanism in the crystallite phase, see Figs. 8 and 9. For coarse grained materials, dislocation glide is predominant, while for fine grained ones, diffusion-controlled Coble creep is the main deformation mechanism. Based on Figs. 8 and 9, one can postulate that the transition occurs when the two modes of deformation contribute almost equally to the total viscoplastic strain rate of the crystallite. Thus the transition occurs when the following condition is fulfilled:

$$ d_{\rm GI}^{\rm disl-eq}=d_{\rm GI}^{\rm Co-eq} $$

(20)

From relation (18), the dislocation strain rate ${d_{\rm GI}^{\rm disl-eq}}$ can be linked to the Coble creep rate ${d_{\rm GI}^{\rm Co-eq}}$. Then using relation (20), the equivalent total plastic strain rate ${d_{\rm GI}^{\rm vp-eq}}$ , as defined by Eq. 7 is expressed (neglecting the Nabarro-Herring creep contribution) as a function of the Coble creep strain rate ${d_{\rm GI}^{\rm Co-eq}}$ :

$$ d_{\rm GI}^{\rm vp-eq} = 2d_{*}\left(\frac{k T d_{\rm tr}^3}{14 \pi \Omega_b D_{\rm bd}^{\rm sd} w \sigma_{\rm o}}d_{\rm GI}^{\rm Co-eq} \right)^m \left( \frac{\rho}{\rho_{\rm o}}\right)^{-\frac{m}{2}} $$

(21)

Here, d _tr is the transition grain size for which relation (20) is satisfied. Due to elasticity, the total plastic strain rate in the crystallite is lower than the total macroscopic strain rate D _o. A scalar β is introduced so that:

$$ d_{\rm GI}^{\rm vp-eq}= \beta D_{\rm o}$$

(22)

The last term to be evaluated is ρ/ρ_o. Considering that the dominant term for small strain in Eq. 7 is related to the constant C and that C is large for a nanocrystalline material, the dislocation density is approximately proportional to the inverse of the grain size d _tr:

$$ \frac{\rho}{\rho_{\rm o}} = \frac{K}{d_{\rm tr}} $$

(23)

Therefore, combining Eqs 22 and 23, one obtains the following relation between D _o and d _tr:

$$ \beta \frac{D_{\rm o}}{2}=d^* \left (\frac{k T d_{\rm tr}^3 }{28 \pi \Omega_b D_{\rm bd}^{\rm sd}w \sigma_{\rm o}}\beta D_{\rm o}\right)^m \left ( \frac{K}{d_{\rm tr}}\right)^{-\frac{m}{2}} $$

(24)

Since m is much larger than unity, this equation leads to:

$$(\beta D_{\rm o})^2 = \left (\frac{28 \pi \Omega_b D_{\rm bd}^{\rm sd} w \sigma_{\rm o}}{k T d_{\rm tr}^3}\right)^2 \frac{K}{d_{\rm tr}} $$

(25)

Finally, assuming that β and K will not vary strongly with loading conditions, one obtains:

$$ D_{\rm o}^2 d_{\rm tr}^7 = \hbox{const} $$

(26)

Appendix B: Relationship between equivalent stresses

During uniaxial tensile loading, the material is subjected to the following macroscopic stress:

$$ \underline{\Sigma}= \left\lbrack \begin{array}{rrr} \Sigma_{11} & 0 & 0 \\ 0 & 0 & 0\\ 0 & 0 & 0 \end{array} \right\rbrack $$

(27)

In the present work, the material is assumed isotropic. Since the loading is axisymetric and due to the Taylor–Lin assumption for which the local strain rate in each phase is equal to the macroscopic strain rate, the stress state in the grain interior can be written as:

$$ \begin{aligned} \underline{\sigma}_{\rm GI}= \left\lbrack \begin{array}{rrr} \sigma_{11}^{\rm GI} & 0 & 0 \\ 0 & \sigma_{22}^{\rm GI} & 0\\ 0 & 0 & \sigma_{22}^{\rm GI} \end{array} \right\rbrack \end{aligned} $$

(28)

The stress state in the grain boundary is given by Eq. 28 replacing the superscript GI by GB. With volume averaging, the macroscopic stress ${ \underline{\Sigma}}$ is linked to stresses ${\underline{\sigma}_{\rm GI}}$ and ${\underline{\sigma}_{\rm GB}}$ in the two phases by:

$$ \underline{\Sigma} = f \underline{\sigma}_{\rm GI} + (1-f) \underline{\sigma}_{\rm GB} $$

(29)

Here, f represents the volume fraction of the grain interior. By combination of Eqs. 28 and 29, one obtains:

$$ \Sigma_{11} = f \sigma_{11}^{\rm GI}+(1-f) \sigma_{11}^{\rm GB},\quad f \sigma_{22}^{\rm GI}+(1-f)\sigma_{22}^{\rm GB}= 0 $$

(30)

The grain interior and grain boundary phases are not subjected to uniaxial tensile loading. Nevertheless, the volume average of the microscopic stresses restitutes a stress tensor of uniaxial tension.

The deviatoric Cauchy stress tensor in the grain interior ${\underline{s}_{\rm GI}}$ , obtained from relation (28) is given by:

$$ \underline{s}_{\rm GI}= \left\lbrack \begin{array}{rrr} \frac{2}{3} \sigma^{\rm eq}_{\rm GI} & 0 & 0 \\ 0 &- \frac{1}{3} \sigma^{\rm eq}_{\rm GI} & 0\\ 0 & 0 & - \frac{1}{3} \sigma^{\rm eq}_{\rm GI} \end{array} \right\rbrack $$

(31)

with ${\sigma^{\rm eq}_{\rm GI} = \sigma_{11}^{\rm GI} - \sigma_{22}^{\rm GI}}$ being the equivalent stress in the grain interior phase. The same expression is valid for ${\underline{s}_{\rm GB}}$ with replacing GI by GB in expression (31). Using Eq. 14, the macroscopic deviatoric Cauchy stress components are:

$$ S_{11}= \frac{2}{3} (f \sigma^{\rm eq}_{\rm GI} + (1-f) \sigma^{\rm eq}_{\rm GB}) \quad S_{22}=-\frac{S_{11}}{2} \quad S_{33}= -\frac{S_{11}}{2} $$

(32)

The equivalent macroscopic stress Σ^eq has the form:

$$ \Sigma^{\rm eq} = f \sigma^{\rm eq}_{\rm GI} + (1-f) \sigma^{\rm eq}_{\rm GB} $$

(33)