A Morphoelastic Shell Model of the Eye

Abstract

The eye grows during childhood to position the retina at the correct distance behind the lens to enable focused vision, a process called emmetropization. Animal studies have demonstrated that this growth process is dependent upon visual stimuli, but dependent on genetic and environmental factors that affect the likelihood of developing myopia. The coupling between optical signal, growth, remodeling, and elastic response in the eye is particularly challenging to understand. To analyse this coupling, we develop a minimal morphoelastic model of an eye growing under intraocular pressure in response to visual stimuli. Distinct to existing three-dimensional finite-element models of the eye, we treat the sclera as a thin axisymmetric hyperelastic shell which undergoes local growth in response to external stimulus. This simplified analytic morphoelastic model provides a tractable framework in which we can evaluate various emmetropization hypotheses and understand different types of growth feedback. As an example, we demonstrate that local growth laws are sufficient to tune the global size and shape of the eye for focused vision across a wide range of parameter values.

Appendices

Appendix A: Shell Stresses

We briefly discuss the standard rationale for the functional form of the stresses specified by Eqns. (17a) and (17b). In a fully three-dimensional elastic body, the Cauchy stress, $\mathbf{\sigma }$, is

$$ \mathbf{\sigma } = -p\mathbf{I} + 2\mathbf{F} \frac{\partial W}{\partial \mathbf{C}}\mathbf{F}^{T}\,, $$

(27)

where $\mathbf{F}$ is the deformation gradient, $\mathbf{C}=\mathbf{F}^{T}\mathbf{F}$ is the right Cauchy-Green tensor, $p$ is the hydrostatic contribution to the stress associated with enforcing incompressibility, and $W$ is the strain-energy function. For a detailed account, we direct the interested reader to, for example, the work of [61]. If we suppose that $W=W(I_{1},I_{4},I_{6})$ where

$$\begin{aligned} I_{1} &= \mathit{tr}\mathbf{C}\,, \end{aligned}$$

(28a)

$$\begin{aligned} I_{4} &= \mathbf{a}^{T}\mathbf{C}\mathbf{a}\,, \end{aligned}$$

(28b)

$$\begin{aligned} I_{6} &= \mathbf{b}^{T}\mathbf{C}\mathbf{b}\,, \end{aligned}$$

(28c)

so that $I_{4}$ and $I_{6}$ represent the stretch of fibres that lie in the directions $\mathbf{a}$ and $\mathbf{b}$, then

$$ \mathbf{\sigma } = -p\mathbf{I} + 2\mathbf{F}\left ( \frac{\partial W}{\partial I_{1}}\mathbf{I} + \frac{\partial W}{\partial I_{4}}\mathbf{a}\otimes \mathbf{a} + \frac{\partial W}{\partial I_{6}}\mathbf{b}\otimes \mathbf{b} \right )\mathbf{F}^{T}\,. $$

(29)

Furthermore, if we select the basis used for our scleral model so that $\mathbf{F}$ is diagonal with entries $\alpha _{s},\alpha _{\phi },\alpha _{n}$, define the fibre directions as in Eqns. (12a), (12b) and (13), so that $I_{4}=I_{6}$, and finally require $W(I_{1},I_{4},I_{6})=W(I_{1},I_{6},I_{4})$, then the off-diagonal terms in $\mathbf{a}\otimes \mathbf{a}$ and $\mathbf{b}\otimes \mathbf{b}$ cancel. Thus, the only non-zero components in Eq. (29) are

$$\begin{aligned} \sigma _{ss} &= -p + 2\alpha _{s}^{2} \frac{\partial W}{\partial I_{1}} + 4\alpha _{s}^{2}\sin ^{2}\psi \frac{\partial W}{\partial I_{4}}\,, \end{aligned}$$

(30a)

$$\begin{aligned} \sigma _{\phi \phi } &= -p + 2\alpha _{\phi }^{2} \frac{\partial W}{\partial I_{1}} + 4\alpha _{\phi }^{2}\cos ^{2} \psi \frac{\partial W}{\partial I_{4}}\,, \end{aligned}$$

(30b)

$$\begin{aligned} \sigma _{nn} &= -p + 2\alpha _{n}^{2} \frac{\partial W}{\partial I_{1}}\,. \end{aligned}$$

(30c)

The shell’s thin geometry can be exploited as discussed in the context of membranes in [68]. We apply the key results in our shell model by working with resultant stresses of the form

$$\begin{aligned} t_{s}&=\alpha _{n}\zeta \sigma _{ss}\,, \end{aligned}$$

(31a)

$$\begin{aligned} t_{\phi }&=\alpha _{n}\zeta \sigma _{\phi \phi } \end{aligned}$$

(31b)

and setting $\sigma _{nn}=0$, often termed the ‘membrane assumption’. This is akin to noting that the curved shell is so thin that load across the surface due to the intraocular pressure is supported by in-shell tension, as opposed to stress across the shell thickness. The membrane assumption enables the elimination of the hydrostatic pressure, $p$, and our incompressibility assumption, $\alpha _{n} = 1/\alpha _{s}\alpha _{\phi }$, gives principal in-shell stress resultants of the form Eq. (14a), (14b).

Appendix B: Intrinsic Growth Capacity

In Eq. (19), we posed a phenomenological functional form for the intrinsic growth capacity $g_{c}$, which we restate here as

$$ g_{c}(\Sigma ) = \frac{\eta _{0}}{2}\left (1 + \tanh \left ( \frac{\Gamma -\Sigma }{\delta }\right ) \right )\,. $$

(32)

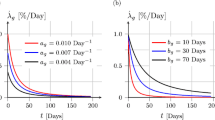
Via the parameters $\Gamma$ and $\delta$, this functional form allows for significant variation in the intrinsic growth capacity. This is illustrated in Fig. 8, from which increasing $\Gamma$ can be seen to extend the region of high growth capacity, whilst $\delta$ governs the sharpness of the interface between regions of high and low growth capacity.

Fig. 8

The intrinsic growth capacity, $g_{c}(\Sigma )$, and its dependence on its parameters $\Gamma$ and $\delta$. Increasing $\Gamma$ can be seen to extend the region of high growth capacity away from the posterior sclera, whilst increasing $\delta$ serves to smooth out the interface between high and low growth regions. Here, we have sampled $\Gamma \in \{4,8,12\}$ and $\delta \in \{2,6\}$, each with units of millimetres as in Fig. 6. The parameter $\eta _{0}$ determines the maximum growth capacity

Appendix C: Optical Calculations

The optical components of the anterior eye, the cornea, anterior chamber, lens and vitreous chamber, focus the light wavefronts that are incident on the eye on a fictitious curved surface near the retina, which we term the best-focus surface. The position and shape of this surface are dependent on the wavelength of the incident light due to chromatic aberrations in the light focusing components. Modeling the geometrical and optical properties of the anterior eye as in [69], a raytracing algorithm was employed in order to compute the individual surfaces of best focus for red and blue incident light wavefronts, exemplified in Fig. 9. Dense arrays of parallel coherent rays were traced through the anterior optics, with the phase of the wavefront emerging on the posterior surface of the lens fitted to Zernike polynomial functions. These are propagated via a Kirchhoff integral and the Strehl ratio is computed on various test surfaces perpendicular to the central ray. The surface corresponding to the maximum Strehl ratio represents the best-focus surface, which is constructed for incident angles between 0 and 40 degrees, appealing to assumed axisymmetry. This approach may be readily extended to include the effects of additional or non-uniform lenses, enabling the modeling of corrective lenses and their effects on ocular development, for example.

Fig. 9

Computing the best-focus surfaces. A dense array of parallel, coherent rays (thin lines) is traced through the anterior optics (cornea, pupil, lens – medium lines) and the phase of the wavefront emerging through the posterior surface of the lens is fitted to Zernike polynomial functions. Bottom – Left: Example of a lens-emerging waveform. A Kirchhoff integral is applied to further propagate the wavefront and compute its modulation transfer function (MTF) on small surfaces perpendicular to the central ray. Bottom – Right: Example MTFs along probed surfaces. Surface C maximizes the Strehl ratio and hence corresponds to the best-focus surface for this wavelength. Computing the best-focus distance for a range of incidence angles (0-40 degrees), assuming axial symmetry, we reconstruct the best-focus surfaces for two wavelengths: 400 nm (blue) and 600 nm (red)

Appendix D: Initial and Boundary Conditions

When considering a non-uniform scleral thickness, following [11] we prescribe

$$ H(\Sigma ) = \left \{ \textstyle\begin{array}{l@{\quad }r} 0.65-0.2\tanh \left (\frac{\Sigma -0.167\pi R_{0}}{1.5}\right )\,, & x \in [0,0.5\pi R_{0}]\,, \\ 0.475+0.025\tanh (\Sigma -0.7\pi R_{0})\,, & x\in (0.5\pi R_{0},L]\,, \end{array}\displaystyle \right . $$

(33)

as shown in Fig. 10 alongside the initial fibre orientation, prescribed as

$$ \frac{\psi ({\Sigma })}{\pi } = 0.25 + \left \{ \textstyle\begin{array}{l@{\quad }r} 0\,, & x\in [0,0.025\pi R_{0}]\,, \\ 0.06\cos \left (\frac{\Sigma -0.025\pi R_{0}}{0.1R_{0}} \right )- 0.06 \,, & x\in (0.025\pi R_{0},0.125\pi R_{0}]\,, \\ 0.15 - 0.17\cos \left (\frac{\Sigma -0.125\pi R_{0}}{0.375R_{0}} \right )\,, & x\in (0.125\pi R_{0},0.5\pi R_{0}]\,, \\ 0.22\cos \left (\frac{ \pi (\Sigma -0.5\pi R_{0})}{L - 0.5\pi R_{0}} \right )\,, & x\in (0.5\pi R_{0},L]\,, \end{array}\displaystyle \right . $$

(34)

following the observations of [16, 51].

Fig. 10

Reference non-uniform scleral thickness and fibre orientation, shown in (a) and (b), respectively, from the works of [11] and [16]

At the anterior point of the sclera, we match the scleral displacement to the inflation of a thin, spherically symmetric, non-growing shell of uniform thickness with no fibres, minimally modeling the cornea. Firstly, for this simple shell, we see that $\alpha _{s}^{c}=\alpha _{\phi }^{c}$, so for notational convenience we denote the stretch simply by $\alpha$, where the superscript on all other variables denotes that we are considering the cornea. Since the corneal reference configuration is spherical, we have

$$ R^{c} = R_{0}^{c}\sin \left (\frac{\Sigma ^{c}}{R_{0}^{c}} \right ), $$

(35)

for $\Sigma ^{c}\in [0,\pi R_{0}^{c}]$, where $R_{0}^{c}$ is the radius of the sphere. The position of a point on the inflated sphere is thus given by

$$\begin{aligned} r^{c}&=\alpha R_{0}^{c}\sin \left (\frac{\Sigma ^{c}}{R_{0}^{c}} \right )\,, \end{aligned}$$

(36a)

$$\begin{aligned} z^{c}&=\alpha R_{0}^{c}\cos \left (\frac{\Sigma ^{c}}{R_{0}^{c}} \right )+B\,, \end{aligned}$$

(36b)

where $B$ is a constant of integration. The constraint of spherical symmetry ensures there is no normal shear force, $Q^{c}=0$, so that the shell deforms as a membrane. The solution to this problem is presented in [70], where it is shown that

$$ \Delta P=\frac{4C^{c}H^{c}}{\alpha R_{0}^{c}}\left (1- \frac{1}{\alpha ^{6}}\right ), $$

(37)

where $C^{c}$ is the neo-Hookean constant, $H^{c}$ is the undeformed thickness and $R_{0}^{c}$ is the undeformed radius of the shell. We take $C^{c}$, $H^{c}$ and $R_{0}^{c}$ to have values based on the mechanics of the cornea and we calculate $\alpha$ for the required pressure difference numerically, restricting $\alpha \in (1,7^{(1/6)})$ due to the non-injective relation between $\alpha$ and $\Delta P$. In particular, the upper limit here is the $\alpha$ value corresponding to the maximum of $1/\alpha -1/\alpha ^{7}$ for $\alpha >1$, it placing a bound on the maximum pressure difference that we can consider, though we don’t vary the intraocular pressure in this work. Finally, evaluating the shape of the cornea at the point of attachment to the sclera, we find the boundary conditions for the scleral shell to be

$$\begin{aligned} r^{\star }&=\alpha R_{0}^{c}\sin \left (\frac{L}{R_{0}^{c}}\right ), \end{aligned}$$

(38a)

$$\begin{aligned} \theta ^{\star }&=\frac{L}{R_{0}^{c}}, \end{aligned}$$

(38b)

$$\begin{aligned} z^{\star }&=\alpha R_{0}^{c}\left (1+\cos \left (\frac{L}{R_{0}^{c}} \right )\right ). \end{aligned}$$

(38c)

Appendix E: Implementation

The governing equations presented in Sect. 2.6 have a singularity when $r=0$, so we solve the system numerically on the truncated domain $\sigma \in [\sigma (\varepsilon ,t),\sigma (L,t)]$ for $0<\varepsilon \ll 1$. By expanding the variables $r$, $\theta$, $\alpha _{s}$, $\kappa _{s}$ and $Q$ around $\sigma =0$ and evaluating at $\sigma =\sigma (\varepsilon ,t)$, following [45], then substituting the expansions into (21a), (21c), (21d), (21e) and Eq. (22) subject to Eq. (24), it becomes clear that the singularity is removable for compatible initial fibre directions. Indeed, isotropy is required as $\sigma \rightarrow 0$ because any preferred direction is undefined at the pole, and if we do not require $\psi \rightarrow \pi /4$ as $\sigma \rightarrow 0$ then there is a singularity in the stress as $\sigma \rightarrow 0$ in the fibre-reinforced shells. Intuitively, this is due to the preferred fibre orientation needing to change direction increasingly quickly as we approach the pole. In order to circumvent this in all the fibre-reinforced simulations in this work, we ensure that $\psi \rightarrow \pi /4$ as $\sigma \rightarrow 0$, subject to which the stress is finite and the boundary conditions on $r$ and $\theta$ can be replaced by the notationally cumbersome

$$\begin{aligned} r(\varepsilon )&= \sigma (\varepsilon )\alpha _{s}(\sigma ( \varepsilon )), \end{aligned}$$

(39a)

$$\begin{aligned} \theta (\varepsilon )&= \sigma (\varepsilon )\alpha _{s}(\sigma ( \varepsilon ))\kappa _{s}(\sigma (\varepsilon ))\,, \end{aligned}$$

(39b)

where we have suppressed the $t$-dependence of all quantities here for brevity. Now considering $Q$, we further manipulate Eq. (21a), (21b), (21c) (21d), (21e) and (21f) to admit the first integral

$$ Q\cos \theta + rt_{s}\sin \theta - \frac{r^{2}\Delta P}{2} = A, $$

(40)

where $A$ is a constant. Since we require solutions that pass through $r=0$ with $\theta =\pi /2$, we find $A=0$. Thus, evaluating Eq. (40) at $\sigma =\sigma (\varepsilon ,t)$ provides the analogous truncated boundary condition for $Q$. Note that whilst it is possible to use Eq. (40) to eliminate $Q$ from Eqns. (21a), (21c) to (21e) and (22), preliminary numerical simulations suggest that it is easier to solve the five ordinary differential equations than the reduced system. Hence, we retain $Q$ in the governing equations and use Eq. (40) as a check on the numerical solutions.

We utilise MATLAB’s inbuilt adaptive boundary problem solver bvp4c to solve equations Eqns. (21a), (21c) to (21e) and (22) subject to the boundary conditions truncated boundary conditions. The initial conditions are provided on a regular grid for $\Sigma \in [0,L]$ and growth is approximated with an explicit Euler scheme for Eqns. (9) and (10). For each simulation, we ensure that the solution has converged with respect to our choices of grid size, timestep, truncation point and error tolerances in the solver. For example, the simulations in Fig. 3 were rerun on a refined spatial grid, with a smaller timestep, with a lower error tolerance in the bvp4c solver, and with a reduced truncation value $\varepsilon$. The largest relative errors in the variables $\kappa _{s}$, $\alpha _{s}$, $r$ and $\theta$ at $t=1$ in this refined simulation are $1.7\times 10^{-3}$, $2.1\times 10^{-5}$, $9.4\times 10^{-4}$ and $7.4\times 10^{-4}$ respectively, well below practical tolerance. Typical parameter values for the simulations in this work are given in Table 1. Typical simulation runtime on modest hardware (Intel^® Core™ i7-6920HQ CPU) is, without significant optimisation of the implementation, approximately two minutes.