Utilization of the Theory of Small on Large Deformation for Studying Mechanosensitive Cellular Behaviors

Abstract

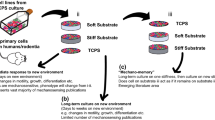
Recent studies suggest that cells routinely probe their mechanical environments and that this mechanosensitive behavior regulates some of their cellular activities. The finite elasticity theory of small-on-large deformation (SoL) has been shown to be effective in interpreting the mechanosensitive behavior of cells on a substrate that has been subjected to a prior large static stretch before the culturing of the cells. Small on large deformation is the superposition of a small deformation onto a prior large deformation that serves as the new reference configuration. This article aims to refine SoL theory to develop a theoretical framework for improved physical interpretation of mechanosensing. Given the initial large deformation in SoL, the stress generated by the small deformation is linearized, and the linearized elasticity tensor is taken to be a significant factor facilitating prediction of cellular behavior. The pre-stretch is shown to produce direction-based, effective elastic moduli for cellular mechanosensing. The utility of this SoL theory is illustrated by culturing of two different cell types grown on uniaxially pre-stretched surfaces that induce changes to the cell orientation and behavior.

Appendix: Symmetry Groups of a Pre-stressed Elastic Body for Uniaxial and Biaxial Stretches

This appendix illustrates the material symmetry groups for pre-stretches of uniaxial and biaxial deformation. Wineman et al. [48] showed that, based on the application of Noll’s theory, the new material symmetry group includes non-orthogonal transformations, which are unimodular, i.e., $\operatorname{det} {\mathbf{Q}} = \pm 1$. Here, $\mathbf{Q}$ is a member in the symmetry group. For a positive orthogonal group, we use the notation $\mathbf{R}_{ \mathbf{n}}^{\theta }$ for the right-handed rotation through the angle $\theta$, $0\le \theta <2\pi$, about an axis in the direction of the unit vector $\mathbf{n}$ (Sect. 33 of [45] by Truesdell and Noll).

From (6) and (7), the total Cauchy stress $\mathbf{T}$ on the current configuration $\kappa (\mathcal{B})$ is

$$ {\mathbf{T}}= \frac{1}{J^{\ast }J^{o}}{\mathbf{F}}^{\ast }{ \mathbf{F}} ^{o}{\mathbf{S}} {\mathbf{F}}^{oT}{ \mathbf{F}}^{\ast T}. $$

(53)

In the stress-free reference configuration $\kappa _{R}(\beta )$, the body is assumed to be isotropic and a general form of the constitutive relation for an isotropic material (whose symmetry group is $\mathcal{Q}=\{ \mathbf{R}_{\mathbf{i}}^{\theta _{1}}, \mathbf{R}_{ \mathbf{j}}^{\theta _{2}}, \mathbf{R}_{\mathbf{k}}^{\theta _{3}}\}$ for $0\le \theta _{1}, \theta _{2},\theta _{3}<2\pi$ and reflective transformation groups, where $\mathbf{i}$, $\mathbf{j}$, and $\mathbf{k}$ are orthonormal vectors) is [20]

$$ {\mathbf{S}}=\alpha _{1} {\mathbf{C}}^{-1}+\alpha _{2}{\mathbf{I}}+ \alpha _{3}{\mathbf{C}}, $$

(54)

where $\mathbf{C}=\mathbf{F}^{T}{\mathbf{F}}$ is the right Cauchy-Green tensor, and

$$ \alpha _{1}=2I_{3}\frac{\partial W}{\partial I_{3}}, \qquad \alpha _{2}=2 \biggl(\frac{ \partial W}{\partial I_{1}}+I_{1} \frac{\partial W}{\partial I_{2}} \biggr), \qquad \alpha _{3}=-2\frac{\partial W}{\partial I_{2}}. $$

(55)

Here $I_{1}$, $I_{2}$, and $I_{3}$ are the three principal invariants of $\mathbf{C}$, respectively.

Let the new symmetry group in the pre-stressed configuration $\kappa _{o}(\beta )$ be $\mathcal{Q}^{\ast }$. For any $\mathbf{Q}^{*} \in \mathcal{Q}^{\ast }$ operating on the pre-stressed configuration, the updated deformation gradient tensor from the reference configuration $\kappa _{R}(\beta )$ to the current configuration $\kappa (\beta )$ is thus

$$ {\mathbf{F}}'=\mathbf{F}^{\ast }{ \mathbf{Q}}^{\ast }{\mathbf{F}}^{o}. $$

(56)

By virtue of (6) and (56), the updated Cauchy stress tensor is

$$ {\mathbf{T}}'={J'}^{-1}{ \mathbf{F}}'{\mathbf{S}}'{\mathbf{F}'}^{T}= \frac{1}{J ^{\ast }J^{o}J_{Q}}{\mathbf{F}}^{\ast }{\mathbf{Q}}^{\ast }{ \mathbf{F}} ^{o}{\mathbf{S}}'{\mathbf{F}}^{oT}{ \mathbf{Q}}^{\ast T}{\mathbf{F}} ^{\ast T}, $$

(57)

where $J'=\text{det}\mathbf{F}'$, $J_{Q}=\operatorname{det} \mathbf{Q}^{*}=1$, and $\mathbf{S}'$ is the updated second Piola-Kirchhoff stress tensor. Under operation of the symmetry group, the Cauchy stress tensor is unchanged, and thus

$$ {\mathbf{T}}'=\mathbf{T}. $$

(58)

From (53) and (57), Eq. (58) gives

$$ {\mathbf{S}}'=J_{Q}{\mathbf{F}}^{o-1}{ \mathbf{Q}}^{\ast -1}{\mathbf{F}} ^{o}{\mathbf{S}} { \mathbf{F}}^{oT}{\mathbf{Q}}^{\ast -T}{\mathbf{F}} ^{o-T}. $$

(59)

By the representation form of the Piola-Kirchhoff stress tensor, similar to (54), one has

$$ {\mathbf{S}}'=\alpha _{1}^{\prime } { \mathbf{C}}^{\prime \,-1}+\alpha _{2}^{\prime }{\mathbf{I}}+ \alpha _{3}^{\prime }{\mathbf{C}}' , $$

(60)

where

$$ \alpha _{1}'=2I_{3}' \frac{\partial W}{\partial I_{3}'}, \qquad \alpha _{2}'=2 \biggl( \frac{\partial W}{\partial I_{1}'}+I_{1}'\frac{\partial W}{ \partial I_{2}'} \biggr), \qquad \alpha _{3}'=-2\frac{\partial W}{\partial I _{2}'}, $$

(61)

and

$$\begin{aligned} I_{1}^{\prime } =& \operatorname{tr} \bigl( \mathbf{Q}^{\ast }{\mathbf{B}}^{o}{\mathbf{Q}} ^{\ast T}{ \mathbf{C}}^{\ast } \bigr), \end{aligned}$$

(62)

$$\begin{aligned} I_{2}^{\prime } =& \frac{1}{2} \bigl( \bigl(I_{1}^{\prime } \bigr)^{2}-\operatorname{tr} \bigl( \mathbf{Q} ^{\ast }{\mathbf{B}}^{o}{\mathbf{Q}}^{\ast T}{ \mathbf{C}}^{\ast } {\mathbf{Q}}^{\ast }{\mathbf{B}}^{o}{ \mathbf{Q}}^{\ast T}{\mathbf{C}} ^{\ast } \bigr) \bigr), \end{aligned}$$

(63)

$$\begin{aligned} I_{3}^{\prime } =& \operatorname{det} \bigl( \mathbf{F}^{oT}{\mathbf{Q}}^{\ast T}{\mathbf{C}} ^{\ast }{ \mathbf{Q}}^{\ast }{\mathbf{F}}^{o} \bigr)=I_{3}, \end{aligned}$$

(64)

$$\begin{aligned} \mathbf{C}' =&\mathbf{F}^{oT}{\mathbf{Q^{*}}}^{T}{ \mathbf{C}}^{\ast } {\mathbf{Q}}^{\ast }{\mathbf{F}}^{o}, \end{aligned}$$

(65)

$$\begin{aligned} \mathbf{C}^{\prime \,-1} =& \mathbf{F}^{o-1}{\mathbf{Q}}^{\ast -1}{ \mathbf{C}} ^{\ast -1}{\mathbf{Q}}^{\ast -T}{\mathbf{F}}^{o-T}. \end{aligned}$$

(66)

Here $\mathbf{B}^{o}=\mathbf{F}^{o}{\mathbf{F}}^{oT}$. Then (60) becomes

$$ {\mathbf{S}}'=\alpha _{1}^{\prime }{ \mathbf{F}}^{o-1}{\mathbf{Q}}^{\ast -1} {\mathbf{C}}^{\ast -1}{ \mathbf{Q}}^{\ast -T}{\mathbf{F}}^{o-T}+ \alpha _{2}^{\prime }{ \mathbf{I}}+ \alpha _{3}^{\prime }{ \mathbf{F}}^{oT}{ \mathbf{Q}}^{ \ast T}{\mathbf{C}}^{\ast }{ \mathbf{Q}}^{\ast }{ \mathbf{F}}^{o}. $$

(67)

In order to satisfy the symmetry condition, the symmetry group should satisfy (59), and this condition can be written as

$$\begin{aligned} {\mathbf{S}}' =& J_{Q} \bigl(\alpha _{1}{ \mathbf{F}}^{o-1}{\mathbf{Q}} ^{\ast -1}{\mathbf{C}}^{\ast -1}{ \mathbf{Q}}^{\ast -T}{\mathbf{F}} ^{o-T}+\alpha _{2}{ \mathbf{F}}^{o-1}{\mathbf{Q}}^{\ast -1}{\mathbf{F}} ^{o}{ \mathbf{F}}^{oT}{\mathbf{Q}}^{\ast -T}{\mathbf{F}}^{o-T} \\ &{}+\alpha _{3} {\mathbf{F}}^{o-1}{\mathbf{Q}}^{\ast -1}{ \mathbf{F}} ^{o}{\mathbf{F}}^{oT}{\mathbf{C}}^{\ast }{ \mathbf{F}}^{o}{\mathbf{F}} ^{oT}{\mathbf{Q}}^{\ast -T}{ \mathbf{F}}^{o-T} \bigr). \end{aligned}$$

(68)

Substituting (67) into (68) results in

$$\begin{aligned} &\mathbf{F}^{o-1}{\mathbf{Q}}^{\ast -1} \bigl\{ \bigl(\alpha _{1}^{\prime }- {\alpha _{1}} {J_{Q}} \bigr)\mathbf{C}^{\ast -1}+ \alpha _{2}^{\prime }{ \mathbf{Q}} ^{\ast }{\mathbf{B}}^{o}{\mathbf{Q}}^{\ast T}-{ \alpha _{2}} {J_{Q}} {\mathbf{B}}^{o} \\ &\quad {}+ \alpha _{3}^{\prime }{\mathbf{Q}}^{\ast }{ \mathbf{B}}^{o}{\mathbf{Q}} ^{\ast T}{\mathbf{C}}^{\ast }{ \mathbf{Q}}^{\ast }{\mathbf{B}}^{o} {\mathbf{Q}}^{\ast T} -{ \alpha _{3}} {J_{Q}} {\mathbf{B}}^{o}{ \mathbf{C}} ^{\ast }{\mathbf{B}}^{o} \bigr\} {\mathbf{Q}}^{\ast -T}{ \mathbf{F}}^{o-T}= \mathbf{0}. \end{aligned}$$

(69)

Theorem 1

For an arbitrary$\mathbf{C}^{\ast }$, (69) is true if

$$ {\mathbf{B}}^{o}=\mathbf{Q}^{\ast }{ \mathbf{B}}^{o}{\mathbf{Q}}^{ \ast T}. $$

(70)

Proof

Under (70), Eq. (69) gives

$$\begin{aligned} &{\mathbf{F}}^{o-1}{\mathbf{Q}}^{\ast -1} \bigl\{ \bigl(\alpha _{1}^{\prime }-{\alpha _{1}} {J_{Q}} \bigr)\mathbf{C}^{\ast -1}+ \bigl(\alpha _{2}^{\prime }-{\alpha _{2}} {J_{Q}} \bigr) \mathbf{B}^{o} \\ &\quad {}+ \bigl(\alpha _{3}^{\prime }-{\alpha _{3}} {J_{Q}} \bigr)\mathbf{B}^{o}{\mathbf{C}}^{ \ast }{ \mathbf{B}}^{o} \bigr\} {\mathbf{Q}}^{\ast -T}{ \mathbf{F}}^{o-T}=0. \end{aligned}$$

(71)

We show (71) is true.

Under (70) and (62), one has $I_{1}^{\prime } = \operatorname{tr} (\mathbf{Q}^{\ast }{\mathbf{B}}^{o}{\mathbf{Q}}^{\ast T} {\mathbf{C}}^{\ast })=\text{tr}(\mathbf{B}^{o}{\mathbf{C}}^{\ast })=I _{1}$, and $\operatorname{tr} (\mathbf{C'}^{2})=\operatorname{tr}(\mathbf{B}^{o}{\mathbf{C}} ^{*} {\mathbf{B}}^{o}{\mathbf{C}}^{*})$. It can be shown that $\mathbf{C}^{2}=\mathbf{F}^{oT}{\mathbf{C}}^{*} {\mathbf{B}}^{o}{\mathbf{C}} ^{*}{\mathbf{F}}^{o}$. Thus $\operatorname{tr} (\mathbf{C'}^{2})=\operatorname{tr} ( \mathbf{C}^{2})$. Under the formulation of $I_{2}'$ given in (63), it therefore shows $I_{2}'=I_{2}$.

Since $I_{1}'=I_{1}$, $I_{2}'=I_{2}$, and $I_{3}'=I_{3}$ (given in (64)), it is obvious that $\alpha _{1}'=\alpha _{1}$, $\alpha _{2}'=\alpha _{2}$ and $\alpha _{3}'=\alpha _{3}$ under $J_{Q}=1$ according to (55) and (61). And then (71) holds. □

In summary, if a material symmetry transformation with $\operatorname{det} {\mathbf{Q}}^{\ast }=1$ in a symmetry group $\mathbf{Q}^{\ast }\in \mathcal{Q}^{\ast }$ satisfies the condition $\mathbf{B}^{o}= \mathbf{Q}^{\ast }{\mathbf{B}}^{o}{\mathbf{Q}}^{\ast T}$, then the body, which was isotropic with respect to the reference configuration, has a new symmetry group $\mathcal{Q}^{\ast }$ with respect to the pre-stressed configuration. Following Noll’s theorem [32], the new symmetry group has the relation $\mathcal{Q}^{\ast }\subseteq \widehat{\mathcal{Q}}$, where

$$ \widehat{\mathcal{Q}}=\mathbf{F}^{o}\mathcal{Q} {\mathbf{F}}^{o-1}. $$

(72)

The following two examples illustrate this result.

Example 1

Let a pre-stressed configuration be the image of a mapping of a uniaxial stretch (or equi-biaxial stretch) from the reference configuration, which is isotropic and stress-free, as

$$ {\mathbf{F}}^{o}=\beta _{1} {\mathbf{n}}_{1} \otimes {\mathbf{n}}_{1} + \beta _{2} {\mathbf{I}}, $$

(73)

where $\mathbf{n}_{1}$ is the unit vector in the stretched direction, and $\beta _{1}$ and $\beta _{2}$ are two stretch constants. Using (72), the transformations in the group $\widehat{\mathcal{Q}}$ become

$$\begin{aligned} {\mathbf{{\widehat{Q}}}}_{1} =& \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} 1 & 0 & 0 \\ 0 & \cos \theta & \sin \theta \\ 0 & -\sin \theta & \cos \theta \end{array}\displaystyle \right ] , \end{aligned}$$

(74)

$$\begin{aligned} \mathbf{{\widehat{Q}}}_{2} =& \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} \cos \theta & \frac{\beta _{1}+\beta _{2}}{\beta _{2}}\sin \theta & 0 \\ -\frac{\beta _{2}}{\beta _{1}+\beta _{2}}\sin \theta & \cos \theta & 0 \\ 0 & 0 & 1 \end{array}\displaystyle \right ] , \end{aligned}$$

(75)

$$\begin{aligned} \mathbf{{\widehat{Q}}}_{3} =& \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} \cos \theta & 0 & -\frac{\beta _{1}+\beta _{2}}{\beta _{2}}\sin \theta \\ 0 & 1 & 0 \\ \frac{\beta _{2}}{\beta _{1}+\beta _{2}}\sin \theta & 0 & \cos \theta \end{array}\displaystyle \right ] , \end{aligned}$$

(76)

where the basis of the tensors is $\mathbf{n}_{i}\otimes {\mathbf{n}} _{j}$. The reflective material symmetry of the planes are

$$ {\mathbf{{\widehat{Q}}}}_{4} = \left [ \textstyle\begin{array}{c@{\quad }c@{\quad }c} -1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & 1 \end{array}\displaystyle \right ] , \qquad \mathbf{{\widehat{Q}}}_{5} = \left [ \textstyle\begin{array}{c@{\quad}c@{\quad }c} 1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & -1 \end{array}\displaystyle \right ] , $$

(77)

where those tensors are evaluated in the reference system. Thus, the fundamental elements in the symmetry group $\mathcal{Q}^{\ast }$ are $\mathbf{{\widehat{Q}}}_{1}$, $\mathbf{{\widehat{Q}}}_{2}$, $\mathbf{{\widehat{Q}}}_{3}$, $\mathbf{{\widehat{Q}}}_{4}$ and $\mathbf{{\widehat{Q}}}_{5}$ for uniaxial stretch.

Example 2

Let a pre-stressed configuration be the image of a mapping of a biaxial stretch from the reference configuration, which is isotropic and stress-free, as

$$ {\mathbf{F}}^{o}=\beta _{1} {\mathbf{n}}_{1} \otimes {\mathbf{n}}_{1} + \beta _{2} {\mathbf{n}}_{2} \otimes {\mathbf{n}}_{2}+ \beta _{3} {\mathbf{n}} _{3}\otimes {\mathbf{n}}_{3}. $$

(78)

Thus using (72), the transformations in the group $\widehat{\mathcal{Q}}$ are

$$\begin{aligned} {\widehat{\mathbf{Q}}}_{1} =& \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} 1 & 0 & 0 \\ 0 & \cos \theta &\frac{\beta _{2}}{\beta _{3}}\sin \theta \\ 0 & -\frac{\beta _{3}}{\beta _{2}}\sin \theta & \cos \theta \end{array}\displaystyle \right ] , \end{aligned}$$

(79)

$$\begin{aligned} {\widehat{\mathbf{Q}}}_{2} =& \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} \cos \theta & \frac{\beta _{1}}{\beta _{2}}\sin \theta & 0 \\ -\frac{\beta _{2}}{\beta _{1}}\sin \theta & \cos \theta & 0 \\ 0 & 0 & 1 \end{array}\displaystyle \right ] , \end{aligned}$$

(80)

$$\begin{aligned} {\widehat{\mathbf{Q}}}_{3} =& \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} \cos \theta & 0 & \frac{\beta _{1}}{\beta _{3}}\sin \theta \\ 0 & 1 & 0 \\ -\frac{\beta _{3}}{\beta _{1}}\sin \theta & 0 & \cos \theta \end{array}\displaystyle \right ] , \end{aligned}$$

(81)

$$\begin{aligned} {\widehat{\mathbf{Q}}}_{4} =& \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} -1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & 1 \end{array}\displaystyle \right ] , \qquad {\widehat{\mathbf{Q}}}_{5}= \left [ \textstyle\begin{array}{c@{\quad}c@{\quad}c} 1 & 0 & 0 \\ 0 & 1 & 0 \\ 0 & 0 & -1 \end{array}\displaystyle \right ] , \end{aligned}$$

(82)

where the basis of the tensors is $\mathbf{n}_{i}\otimes {\mathbf{n}} _{j}$. By adding the reflective symmetry group, the fundamental elements in the symmetry group $\mathcal{Q}^{\ast }$ are $\mathbf{{\widehat{Q}}}_{1}$, $\mathbf{{\widehat{Q}}}_{2}$, $\mathbf{{\widehat{Q}}}_{3}$, $\mathbf{{\widehat{Q}}}_{4}$ and $\mathbf{{\widehat{Q}}}_{5}$ for biaxial stretch.