1 Introduction

Mathematical reasoning (MR) involves developing mathematical claims by processes related to searching for similarities and differences between mathematical objects, like comparing, classifying, and conjecturing, and processes related to validating, like justifying and proving (Jeannotte & Kieran, 2017; G. J. Stylianides, 2008). Several countries, such as the USA and Norway, emphasize embedding MR in teaching across all topics and grades (National Council of Teachers of Mathematics, 2000; Ministry of Education and Research, 2019). However, despite being integral to the discipline of mathematics, it is unclear how MR can be integrated into school mathematics (Ball & Bass, 2003; Chazan & Lueke, 2010). To promote MR, studies emphasize problem-solving tasks and tasks requiring conjectures and justifications (Bieda, 2010; Herbert & Williams, 2023; Ponte et al., 2023). However, Gardiner (2004) suggests that mathematical claims are developed in all school mathematics, not just in particular tasks. He emphasizes that deductive reasoning is essential even in work on calculation strategies because all mathematics teaching shapes students’ understanding of the nature of mathematics. We argue, therefore, that there is a need to investigate opportunities for MR in school mathematics, entailing studying diverse teaching situations in mathematics classrooms, such as introducing new mathematical objects or procedures and working on different types of tasks. A framework broadly identifying MR opportunities in classroom data is crucial to reveal when and how MR opportunities arise, how they are utilized, and how MR can be strengthened.

Previous research has introduced various frameworks addressing (opportunities for) MR. In their textbook analysis, Davis et al. (2014) built on G. J. Stylianides' (2009) work and identified tasks and expositions (narrative parts of the textbook) with the potential for investigating patterns, conjecturing, or argumentation. These were further subjected to a deeper content analysis. Davis et al. (2014) used one or more sentences in expositions and separate questions in tasks as their unit of analysis. Similar approaches were employed in other frameworks for textbook analysis (such as Otten et al., 2014; Thompson et al., 2012; see also Weingarden et al., 2022). In the case of adapting such frameworks from textbook/task analysis to classroom data, it is challenging to determine the unit of analysis as the data is rather different.

Other studies explored MR in classroom interactions, often focusing on specific MR processes. For example, Bieda (2010) investigated work on proving tasks, identifying events where students justified conjectures, categorizing justifications by mathematical validity, and analyzing teacher moves. This approach, also seen in studies by Ellis et al. (2019), Herbert and Williams (2023), Nordin and Boistrup (2018), and Reuter (2023), requires identifying data excerpts involving particular MR processes and analyzing related aspects. Adapting their frameworks to the broader context of MR in school mathematics may pose challenges in determining the unit of analysis. However, we assume it is possible by identifying excerpts in classroom transcripts involving any MR processes.

The aforementioned studies share the approach of identifying MR processes (required in tasks or played out in interactions). Hence, given a task or discussion about some calculations involving no MR processes, these approaches would not capture it as an opportunity for MR, even though it can be such according to Gardiner (2004). MR is fundamentally about deriving claims (Jeannotte & Kieran, 2017), and we propose that a framework highlighting claims rather than MR processes would give broader insight into MR opportunities. Starting by identifying a mathematical claim that can be developed by MR processes, one can analyze how it was developed in the data, even though it was not by MR. The framework would capture such situations as opportunities for MR, providing room for discussion and analysis. Sometimes, promoting MR in a given situation does not make sense, while, in other cases, it can enhance learning. Beyond offering a comprehensive perspective on opportunities for MR, initiating the analysis by identifying MR-relevant claims could also provide a suitable unit of analysis for classroom data.

After identifying MR-relevant claims, the framework must encompass the processes involved in their development within the classroom. As pointed out above, we suggest that these processes can be MR processes and other types of processes. In their framework for task analysis, Weingarden and Buchbinder (2023) introduce “school-based processes” (e.g., solving equations, drawing graphs, and calculating) as a counterpoint to MR processes. However, they acknowledge that “solving any mathematical task can (and should) involve reasoning” (p. 5), blurring the distinction between school-based processes and MR processes in teaching situations. Also, Jeannotte and Kieran’s (2017) theoretical distinctions, e.g., between comparing and classifying, may not be crucial in analyzing MR opportunities in classrooms. To effectively identify how and where MR can be strengthened, we propose the need for an alternative distinction between processes involved in the development of mathematical claims. This distinction should emphasize the nature of the different processes. Moreover, to enhance applicability, this distinction should be derived not only theoretically but also inductively through classroom data analysis. This paper's primary contribution lies in the introduction of such a framework, and our research question is:

How can opportunities for mathematical reasoning in school mathematics be investigated, taking MR-relevant claims and their development as the unit of analysis?

To address the research question, we started by developing the framework theoretically (Section 2), drawing on previous studies and utilizing commognition (Sfard, 2008) as our theoretical lens. Commognition has shown merit in prior studies analyzing MR (e.g., Shinno & Fujita, 2021; Valenta & Enge, 2022; Weingarden & Buchbinder, 2023). Specifically, Sfard (2008) emphasizes constructing and substantiating narratives as central aspects of mathematics (p. 225). We find these three notions valuable for investigating MR: MR-relevant claims constitute a particular kind of narrative, and their development in the classroom can be seen as construction and substantiation. The framework was then refined by analyzing data from Norwegian primary school classrooms. The study employs data for a dual purpose: refining the framework (Sections 3.2 and 3.3) and illustrating how the framework can be used to analyze classroom data and what the analysis can reveal (Sections 3.3 and 4). In Section 5, we discuss the study’s theoretical and methodological contribution along with the practical value of the framework — both for teachers and researchers.

2 Theoretical framework

This section describes the critical constructs of commognition (Sfard, 2008), the definition of MR given by Jeannotte and Kieran (2017), and other theoretical notions used to develop the framework.

2.1 A commognitive perspective on mathematics and mathematical reasoning

Sfard (2008) argues that mathematics is a particular discourse: a form of communication within a community. This discourse employs specific words, visual mediators, routines, and narratives. Words like “sum” and visual mediators like “ + ” are used to identify the objects of communication. Routines, like calculations or deductive reasoning, are regular actions within this discourse. Narratives are spoken or written utterances describing objects or relations between objects or activities with or by objects, such as definitions and theorems. They are subjected to endorsement or rejection by being labelled as true or false (Sfard, 2008, p. 300).

Jeannotte and Kieran (2017) define MR processes, through a commognitive frame, as “processes that derive narratives about objects or relations by exploring the relations between objects” (p. 9). They distinguish between processes related to searching for similarities and differences, validating, and exemplifying. Searching for similarities and differences involves generalizing, classifying, identifying patterns, comparing, and conjecturing, all inferring narratives. Conjecturing infers narratives with the epistemic value of “likely,” creating a need for validation. For example, identifying a pattern and conjecturing may lead to the claim that “a number must end with 5 or 0 to be divisible by 5,” which requires validation. The validating MR processes are justification, proving, and formal proving, aiming to change a conjecture's epistemic value (e.g., from likely to true). Justification allows for modifying the epistemic value, but it can be from “likely to more likely” and does not need to be mathematically valid. For instance, checking several examples that a claim holds would make it more likely (but not certain) that it is true. However, proof must be mathematically valid, and the epistemic value must be modified from likely to true (Jeannotte & Kieran, 2017).

Sfard (2008) describes constructing, substantiating, and recalling narratives as core routines of mathematical discourse (p. 225). Constructing is a process resulting in new endorsable narratives and substantiation as a process that helps participants decide whether to endorse previously constructed narratives. MR processes related to the search for similarities and differences infer narratives and are thus a form of construction. Similarly, MR processes related to validation are substantiation processes. Besides MR processes, there can be other forms of construction and substantiation, as we elaborate in Sections 2.3 and 2.4.

2.2 Mathematical narratives that are subject to MR processes

Sfard (2007) distinguishes between object-level narratives, which are stories about objects, such as “1/2 is equivalent to 2/4”, and meta-level mathematical narratives, which are stories about the discourse itself, including “how mathematics is done” (Sfard, 2007, p. 572), such as “In mathematics, we always want to know why a pattern arises.” Jeannotte and Kieran (2017) highlight that MR involves deriving narratives about objects or relations (p. 9), meaning they are object-level narratives. However, while both definitions and theorems are examples of object-level narratives, their nature is somewhat different, so we need to specify object-level narratives further to identify opportunities for MR.

Hewitt (1999, 2001a, b) classifies mathematics into arbitrary, agreed upon within the community, and necessary, which can be derived. Examples of arbitrary mathematics include names, definitions, and conventions, while necessary mathematics involves procedures, results of calculations, and theorems. Based on Hewitt, we distinguish between arbitrary object-level narratives (AOLs) and necessary object-level narratives (NOL). AOLs are substantiated by reasonableness (e.g., Kontorovich & Zazkis, 2017), aiming to ease communication or as products of historical development. In contrast, NOLs can be substantiated by proving, following deductive chains of object-level claims. Therefore, NOLs, along with their construction and substantiation, are relevant for investigating opportunities for MR. Thus, the first step of our framework is to identify NOLs that are constructed and/or substantiated in the data, differentiating them from AOLs and meta-level narratives. The unit of analysis is thus an NOL and its construction and substantiation.

A. J. Stylianides and Ball (2008) emphasize that, in primary school, the complexity of developing and validating a conjecture depends on the number of cases involved—single, multiple but finite, or infinite. If a conjecture involves an infinite number of cases, it can be challenging for students to find an appropriate representation and a way to validate it. As the number of cases in a conjecture plays a significant role in the involved MR processes, we follow A. J. Stylianides and Ball's (2008) categorization of conjectures and differentiate between three types of NOLs:

  • single case (e.g., “101 is a prime number”),

  • multiple but finitely many cases (those that can be proved by systematic enumeration of all cases, e.g., “11 is not possible to write as a sum of two prime numbers”), and

  • infinitely many cases/general NOLs (e.g., “square numbers have always an odd number of factors”).

Approaches to substantiation of NOLs concerning different numbers of cases are discussed further in Section 2.4.

2.3 Construction of NOLs

Narratives can be constructed by MR processes related to the search for similarities and differences (Jeannotte & Kieran, 2017). For example, by investigating multiples of five, one can infer the narrative “The ten first multiples of five end with five or zero” through classification or pattern identification. While the distinction between various MR processes might not be crucial for classroom analysis, it is essential to note that MR processes involve exploring relations between objects.

Alternatively, narratives can be constructed by calculating or solving equations (Sfard, 2008). For instance, following an equation-solving procedure may lead to the narrative “Five is the only solution of the given equation.” Procedures in mathematics are based on properties of and relations between objects, and properties and relations can be discussed more or less while performing procedures. However, the distinction between discussing mathematical objects and detailing actions is crucial in mathematical discourseFootnote 1 (Sfard, 2016a, 2016b), and recognizing this distinction is central to analyzing MR, given that MR processes focus on properties and relations (Jeannotte & Kieran, 2017). Therefore, considering the construction of NOLs, we distinguish between construction based on procedures and construction based on properties and relations.

2.4 Substantiation of NOLs

No additional substantiation is needed when a new NOL is constructed through deductive reasoning or by correctly applying an endorsed procedure (Sfard, 2008, p. 230, 232). For example, suppose the definition of prime numbers is used to infer the narrative “There are more prime numbers between 1 and 50 than between 51 and 100” or that the narrative “12 × 9 = 108” is constructed by correctly applying a known procedure for multiplication. Then, endorsement follows from construction, and substantiation thus happens simultaneously. However, a separate substantiation is required when constructing a narrative by conjecturing or when the NOL is constructed using an unfamiliar procedure. For example, if “12 × 9 = 108” is proposed in grade 2, where the procedure is unlikely known, a separate substantiation is needed. Therefore, we claim that some narratives are substantiated implicitly through their construction, while others are substantiated explicitly by a separate validation.

As for construction, we differentiate between substantiation using procedures and substantiation using properties and relations, as informed by Sfard (2016a, 2016b). Also, Nachlieli and Tabach (2019) point out that substantiation can happen through detailing either the steps of a procedure or the underlying reasoning (see also Drageset's (2021) distinction between explaining reasons and explaining actions). For instance, to validate the narrative “1.5 plus 2.3 equals 3.8,” an argument using procedures might be “First, I took five and three to get eight, then I took one and two and got three; so, I got 3.8.” Conversely, an argument for the same narrative using properties and relations could be “The sum of five tenths and three tenths is eight tenths, and the sum of one and two is three. Hence, the sum is three and eight tenths, thus, 3.8.” While these arguments may appear similar, the first is about actions while the second is about mathematical objects. Again, this distinction is crucial since MR processes are about mathematical objects rather than describing actions.

As previously discussed, Jeannotte and Kieran (2017) identify justifying, proving, and formal proving as MR processes related to validation, with the distinction that justification need not be mathematically valid, while proving must be. A. J. Stylianides and Ball (2008) stress that the validity of argumentation modes depends on the number of cases involved—single, multiple but finite, or infinite. Reid (2002) distinguishes arguments for single-case claims based on the number of steps and premises involved, labelling one-step reasoning from one premise as a specialization. “Prime numbers have only two factors, five has only two factors, thus, five is a prime number.” is an example of specialization. For claims involving finitely many cases, showing a systematic approach to finding all cases is considered valid (A. J. Stylianides & Ball, 2008). For general claims, learners often use empirical arguments by checking examples, and such arguments are not proof (Sowder & Harel, 1998). However, examples can be used generically to uncover the underlying properties and/or relations causing the conjecture to hold, which is considered valid proof in school (e.g., Rø & Arnesen, 2020; Rowland, 1998).

2.5 A theoretically derived framework for NOLs, their construction and substantiation

Following the synthesis above, we summarize categories of NOLs, their construction and substantiation in Table 1. The first step in applying the framework is identifying NOLs constructed and/or substantiated in the data, differentiating them from AOLs and meta-level narratives. NOLs can take either of three forms based on the number of cases. Construction can occur by using procedures or properties and relations. Substantiation can be implicitly embedded in construction or explicit. As for construction, explicit substantiation can occur using procedures, or properties and relations. The latter relates to proving and can be valid or non-valid.

Table 1 Theoretically derived framework for NOLs, their construction and substantiation
Full size table

3 Refining the theoretical framework using empirical data

We seek a detailed understanding of a specific phenomenon (e.g., Cohen et al., 2011, pp. 219–223): the phenomena of teaching and learning MR and opportunities for such in school mathematics. The framework in Table 1 is refined using data collected from classrooms chosen by a generic purposive sampling (Bryman, 2016, pp. 412–413). We chose primary schoolFootnote 2 classrooms to refine the framework (Section 3.2) and demonstrate its applicability (Section 4), acknowledging that data from later grades could have shed light on other aspects of MR. Below, we elaborate on the participants and data collection and analysis processes.

3.1 Data and participants

This study is situated within a larger project to promote MR in primary education (ProPrimEd), wherein instructional materials were developed and tested through an intervention-based study. The data collection reported here was conducted in preparation for the intervention, investigating teachers' current instructional practices and opportunities for MR in their classrooms. Data was collected in five classrooms (grades 4–7) in two Norwegian primary schools, of which four are used in this study.Footnote 3 The local government suggested that the schools and the principals encouraged the teachers to participate, to which they agreed. The four male participants had four to 17 years of experience as mathematics teachers and reported having no specific competence or experience regarding MR. Hence, they represent teachers who might benefit from the instructional materials developed through ProPrimEd. Informed consent was collected from the teachers and the students’ guardians.

Two researchers observed two weeks of mathematics teaching in each classroom, totaling 18 lessons lasting 40 to 90 min. For all lessons, whole-class discussions and group work were video-recorded, and the teacher carried a sound recorder. The data material is verbatim transcripts of whole-class discussions and teachers’ verbal exchanges with students during group work. We only analyzed interactions in which the teacher participated, as we considered the teacher to set the tone for the classroom discourse.

Table 2 gives an overview of the four teachers’ (pseudonyms) lessons. The purpose of the table is to offer insight into the data material used to refine the framework. We use the term exercise tasks for tasks where it appears clear how the students should proceed; otherwise, we use problem-solving tasks.

Table 2 Overview of the four teachers, lessons, and the mathematics content
Full size table

3.2 Analytical approach

The analysis was carried out through two stages based on the framework in Table 1. Stage 1, referring to the first column of Table 1, entailed separating the NOLs from other narratives (i.e., AOLs, meta-level narratives, non-mathematical narratives) by locating sequences in the classroom dialogue that revolved around new NOLs. Based on our interpretation, we put the narratives into words, as in this example from Einar’s classroom, where the students worked with prime numbers:

figure a

We only considered new narratives constructed or substantiated in the classroom (e.g., from task solutions) and disregarded known narratives recalled during task solutions. In the above excerpt, we identified two such NOLs, as shown in Table 3 (the NOL “2 times 2 equals 4” is not included in Table 3, as it is considered recalled). After identifying and phrasing the NOLs appearing in the classroom dialogues, we distinguished between NOLs concerning a single case, multiple cases, and infinitely many cases.

Table 3 NOLs identified in the excerpt above
Full size table

In Stage 2, the utterances concerning each NOL’s construction and substantiation were coded. Thus, this stage adhered to the second and third columns of Table 1 and was undertaken by identifying whether the construction or substantiation was done by referring to a procedure or properties and relations. The coding was initially deductive, following the categories in Table 1. Further, the coding scheme was expanded by open coding. The analysis thus followed an abductive approach (Kennedy & Thornberg, 2018), as the theoretical framework was complemented by categories found by inductive analysis in cases where the framework fell short of capturing the nuances of the data material. For example, there were NOLs suggested by either the teacher or the students for which there were no observable construction processes. Some NOLs were presented in the classroom as a rule or fact. Also, NOLs were constructed by a mixture of using procedures and properties and relations. Further, some NOLs were substantiated by being unchallenged in the classroom (where students were free to disagree), by a short confirmation or through a mixture of procedures and properties. Table 4 provides an overview of the codes for construction and substantiation of NOLs identified in the data, with examples.

Table 4 Codes of instruction and substantiation of NOLs identified in the data
Full size table

3.3 Refined framework for NOLs, their construction and substantiation

The theoretical framework was refined following the abovementioned process, as shown in Table 5. Codes resulting from the inductive analysis are italicized.

Table 5 Refined framework for NOLs, their construction, and substantiation
Full size table

Table 6 shows an excerpt of the analysis of lesson 2 in Einar’s class. In addition to coding the processes of construction and substantiation, we noted whether the substantiation processes were valid or not, as well as the argument type. The substantiation identified in Einar’s lesson on prime numbers [28] was interpreted as a generic example since Einar used the number 4 generically. Below, we provide more in-depth examples of analysis.

Table 6 Example of analysis of construction and substantiation of NOLs
Full size table

4 Illustrative episodes

To illustrate the use of the framework and the phenomena it can reveal, we proceeded to identify different teaching situations in the eighteen lessons. Following a thematic analysis approach (Braun and Clarke, 2006), we identified four categories of teaching situations: introducing new objects, introducing new strategies or procedures, working on exercise tasks, and working on problem-solving tasks. Examples of in-depth analysis of opportunities for MR from each category are provided below.

4.1 Introducing new objects

Introducing new mathematical objects, definitions, and representations involves arbitrary object-level narratives (AOLs). As discussed before, substantiation of such narratives is not related to MR. However, the analysis revealed that MR processes can appear when new definitions are introduced.

Lesson 1 from Einar’s classroom illustrates the MR processes that occur while defining prime numbers. Moreover, the episode shows how the discussion can develop as the definition is repeatedly used. Einar approached the definition of prime numbers by letting the students search for similarities and differences related to the notion of factors, as factors were known to the students. In the following excerpt, the class discussed factors of ten:

figure b

After checking factors in several numbers and writing the results on the blackboard, the discussion continued:

figure c

Einar then introduced prime numbers as those with only two factors that can be written as a product in just one way. Moreover, the use of the definition was practised, as in the following excerpt:

figure d

While introducing the definition of prime numbers, several NOLs were constructed and substantiated using properties and relations. For example, in [69–71], factors of 10 were discussed, and the narrative “1, 10, 2, and 5 are all the factors of 10” was substantiated by systematically listing all possible products, and in [101] students were asked to look for patterns. After the presentation of the definition, new NOLs were again constructed and substantiated using properties and relations by classifying numbers as prime or not. These NOLs are of the same form and about single cases (“x is a prime/not prime”). During [152–154] and [169–173], the substantiation was explicit and deductive, as the class referred to the definition (denoted as a specialization by Reid (2002)). However, later in the dialogue [249–250], substantiation was less emphasized: the NOLs were now substantiated by no protests or short confirmations. When the same substantiation process has been undertaken several times, it can be reasonable not to repeat it during a classroom dialogue.

4.2 Introducing new strategies or procedures

Strategies and procedures are general NOLs, and their construction and substantiation can be undertaken by MR. One episode where a new procedure was introduced occurred in Arne’s classroom, lesson 3. The general NOL discussed was: “The sum of two decimal numbers is a decimal number where each digit is given as the sum of digits on corresponding positions, and values for ten and more are exchanged to a higher position.” In the episode, the construction processes appeared as a mixture of procedures and properties, and the substantiation was implicit by construction. The discussion started with an example:

figure e

In [36–56], the single case NOL “1.22 + 0.8 equals 2.02” was constructed using a mixture of procedures and properties. The procedures emerged in Arne’s and the students’ talk about actions, such as “we add eight tenths” [36], “you get” [40], and “we transfer” [43]. However, they also talked about properties of the positioning system, such as in [44], where Arne uttered that there is no room for more than nine tenths in the given position. The substantiation was implicit (and thus of the same type as construction – by a mixture of procedures and properties) as there was no additional discussion on the validity of the narrative “1.22 + 0.8 equals 2.02” after reaching the sum.

In [57], the general NOL, which we formulate as “The sum of two decimal numbers is a decimal number where each digit is given as the sum of digits on corresponding positions, and values for ten and more are exchanged to a higher position,” was constructed by generalizing from the example “1.22 + 0.8 equals 2.02” (construction using properties and relations). The substantiation was implicit by construction, and the example was used generically in the substantiation process.

4.3 Work on exercise tasks

In our data, all exercise tasks were about constructing or substantiating single-case NOLs. Following our theoretical account, single-case NOLs can be constructed and substantiated using properties and relations, thus offering opportunities for MR. Sometimes, the exercise tasks were about properties of numbers or operations (e.g., involving processes of classifications). Other times, they involved practicing procedures.

Examples of tasks on properties were checking if a number is a prime number (Einar’s classroom), trying to find out “which number is on my mind” (Arne’s classroom), and relating multiplication to different representations (Hans’ classroom). The following episode is from Hans’ classroom and a whole-class discussion about a picture of three tables with six people seated around each. The student Nelly suggested that the situation could refer to the calculation six times three.

figure f

The construction of the narrative “six times three fits the situation in the picture” was coded as unclear since the origin of Nelly’s suggestion was unknown. The discussion above concerned substantiation and used properties and relations, explicitly using the definition of multiplication previously endorsed by the class.Footnote 4 Hence, the substantiation can be considered a specialization: applying a definition to the given case.

Exercise tasks about practicing procedures were treated in various ways in the data – sometimes emphasizing procedures, sometimes properties and relations. In the following, Hans helped a student calculate seven times six, emphasizing properties and relations in multiplication:

figure g

In contrast, the following episode from Arne’s lesson 4 (grade 6) emphasizes procedures: the student describes actions of taking away and having something left, and there is no further discussion.

figure h

4.4 Work on problem-solving tasks

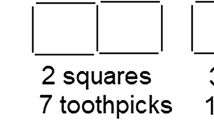
We define problem-solving tasks as those involving several steps, where at least some appear challenging or new to the students. In our data, all problem-solving tasks were about constructing or substantiating NOLsFootnote 5 (single, multiple case, or general). We provide two episodes from work on multiple case NOLs; first, from Einar’s classroom, working on the task presented in Fig. 1.

Fig. 1
figure 1

A problem-solving task from Einar’s classroom generating a multiple case NOL

Full size image

The following excerpt comes from the final discussion in a student group:

figure i

The solution of the task, “Kine has to buy 16 large and nine small buttons”, was coded as multiple case NOL since it can be proved by systematically comparing all possible combinations to the given premises (total cost maximum NOK 100, and as many large buttons as possible). Earlier, Aksel compared different combinations. Hence, the construction was coded as a mixture of procedures (calculating) and properties (comparing to other solutions and premises). The excerpt above demonstrates substantiation; it is explicit and valid by systematically listing all solutions.

Another episode involving work on multiple case NOLs appeared in Per’s grade 7 classroom. The task is shown in Fig. 2.

Fig. 2
figure 2

A problem-solving task from Per’s classroom, generating a multiple case NOL

Full size image

The following episode took place after group work:

figure j

This task is about constructing and substantiating two multiple case NOLs of the form “This position of parenthesis gives largest/least result,” which systematic listing can prove. The construction of both NOLs was unclear. One emerged from students’ work, the teacher suggested the other, and we do not know which processes were involved in either. Unlike in the previous episode from Einar’s classroom, the substantiation was coded as no protests, as no one claimed to have found a position of parenthesis giving the biggest/least possible answer, and no one disputed that the suggested NOLs were true.

5 Discussion

In the introduction, we hypothesized that using MR-relevant claims and their development as the unit of analysis holds merit for investigating opportunities for MR in school mathematics. In addition to developing the framework, classroom data was utilized above to illustrate how the framework can be used in analysis and what the analysis of four typical teaching situations can reveal. Here, we will discuss the theoretical and methodological contribution of our work and the practical value of the framework.

5.1 Theoretical and methodological contribution

We argue that the proposed framework for investigating opportunities for MR in school mathematics contributes theoretically and methodologically in three ways.

Firstly, identifying NOLs and their construction and substantiation captures opportunities for MR that the frameworks utilized in previous studies would omit, thus applying to a greater range of teaching situations. For example, the episode where students work with the task about 7 s and parentheses (Section 4.4) would not be captured by searching for MR processes only. Also, opportunities for MR in exercise tasks (4.3) would not be captured if one analyzed just particular types of tasks.

Secondly, the framework proposes a new categorization of processes of construction and substantiation. Weingarden and Buchbinder (2023) suggested distinguishing between school-based and MR processes. However, MR can be involved while performing “school-based” processes, as seen in work on exercise tasks (Section 4.3). Our distinction between referring to procedures and referring to properties and relations resembles that of Weingarden and Buchbinder (2023). Still, our framework emphasizes the main difference between MR and other possible processes, drawing on the commognitive definition of MR (Jeannotte & Kieran, 2017) and the commognitive framing more generally (Nachlieli & Tabach, 2019; Sfard, 2016a, 2016b). Moreover, our analysis shows that substantiation can happen as a mixture of referring to procedures and properties and relations. In this way, we offer supporting examples of the phenomenon Christiansen et al. (2023) propose as hybrids between ritual and explorative routines.

Thirdly, our analysis shows that taking NOLs as the unit of analysis holds merit as an analytic approach. We suggest that such a “narrative approach” can also be used to investigate other aspects of mathematics teaching. For example, identifying meta-level narratives and investigating their construction and substantiation can provide insight into processes of establishing new meta-rules in classrooms.

5.2 Practical contribution

This study was initiated by the need for a framework to capture opportunities for MR in school mathematics. We propose that the framework and its application offer insights that can benefit practitioners (teachers, pre-service teachers) and researchers. As specified in Section 3.1, we have analyzed classroom interactions in which the teacher participated, thus illustrating how teachers may create opportunities for MR. Yet, the framework and the related analytical approach do not distinguish between the actors involved, meaning that the framework applies to both teacher-student and student–student interactions.

The framework can make (pre-service) teachers conscious of the difference between arbitrary and necessary mathematics (Hewitt, 1999) and that MR is possible in all situations where some necessary claim about mathematical objects (NOL) is developed. The framework also provides an “easy way” to distinguish between MR processes, which are about properties of and relations between objects, and other kinds of processes, such as detailing the steps of a procedure in a task solution. Hence, the framework can give a better understanding of what MR is about and what it can entail in practice.

Analyzing classroom data using the framework can provide insights into opportunities for and occurrences of MR in school mathematics and foster discussions on how to enhance it in different teaching situations. While problem-solving tasks inherently offer MR opportunities, these opportunities can also be missed, as shown in the second episode in 4.4. On the other hand, exercise tasks can give opportunities for MR when properties, relations, and questions like “Why is this true?” are emphasized, as highlighted in Section 4.3. Although reasoning in exercise tasks may be simple and repetitive, it can facilitate a habit of justifying claims, which is essential in mathematics (Gardiner, 2004; Sfard, 2008). However, there can be many repetitions, and even though MR is possible in some situations, it does not always make sense, as discussed in the episode on prime numbers in Section 4.1. Furthermore, although definitions and conventions are arbitrary and not something to prove, the episode in Section 4.1 illustrates that MR can be fostered before and after introducing definitions. Still, mathematical procedures are necessary mathematical knowledge (Hewitt, 1999) and can be deduced, so, unsurprisingly, MR can be promoted while introducing procedures. Even though MR revolves around properties and relations while procedures focus on actions, the episode in Section 4.2 shows that it is possible to promote properties and relations when introducing and practicing procedures.

In addition to its application in working with (pre-service) teachers, the framework can be valuable for research. It can be used to characterize and compare different classrooms, revealing opportunities for MR and their utilization, and to identify opportunities for MR that are taken or missed. Different phenomena can be revealed, such as one we observed in our data: that students in primary schools have minimal experience with reasoning on general mathematical claims. In the 18 lessons analyzed, only one task in grade 7 involved validating general NOLs. Proving general claims is considered challenging (G. J. Stylianides et al., 2017), and students’ limited experience with general claims can be a part of the explanation. Additionally, the framework can be used to characterize and compare work on different mathematical topics, investigating whether and how the mathematical content influences opportunities for MR. For instance, the distinction between using procedures and using properties can point to why promoting MR in work on procedures can be challenging, a phenomenon discussed by Chazan and Lueke (2010): MR is about properties and relations between objects while introducing procedures naturally entails discussing doings. Furthermore, the framework can identify areas to strengthen MR in school mathematics and compare changes before and after interventions. This last objective was our primary motivation for developing the framework, and we aim to demonstrate its efficacy in future studies.

While the framework's applicability is discussed above, there is potential for further development. For example, the framework stems from analyzing teaching practices in four Norwegian primary school classrooms. Exploring different contexts may reveal additional framework categories. Furthermore, including meta-level learning about MR, as emphasized by Weingarden and Buchbinder (2023), could introduce a new dimension to the analysis. Hence, the proposed analytic framework is an initial step toward more insight into opportunities for MR in school mathematics.