Abstract
The European Union (EU) Green Deal and its Farm to Fork Strategy are intended to promote sustainable food systems to achieve EU climate-neutrality by 2050. The Farm to Fork action plan also foresees the introduction of a harmonized mandatory front-of-pack nutrition labelling scheme in 2023. The EU countries have yet to reach agreement on the nutrition labelling scheme, which will also have environmental impacts. This article raises the question of whether at the European level, countries should seek agreements on both climate mitigation and nutrition policies (full agreement as in the case of the Green Deal) or should negotiate separate climate and nutrition policy agreements (as for the nutritional labelling). To address this question, this paper develops a game-theoretic model with multiple countries where each country implements a climate policy and a nutrition policy. We compare the consequences in terms of total emissions, the level of the nutrition policy and the welfare under different institutional arrangements of a non-cooperative equilibrium, full agreement, and three alternative agreements. Our results show in particular that full agreement always leads to the lowest total emissions at the expense of the level of nutrition policy in some cases. In an extension of our analysis, we show that agreements that include cooperation over nutrition policies do not necessarily imply formation of a larger coalition of signatory countries, even if a nutrition policy has positive or negative impacts on emissions.
Similar content being viewed by others
Notes
This strategy foresees implementation of several instruments: harmonized mandatory front-of-pack nutrition labelling, a sustainable labelling framework which covers the nutritional, climate, environmental and social aspects of food products, and tax incentives such as differentiated VAT rates to support cultivation of organic fruit and vegetables for instance (European Commission 2020).
Food production and consumption are responsible for \(60\%\) of terrestrial biodiversity loss and \(70\%\) of freshwater use (UNSCN 2017).
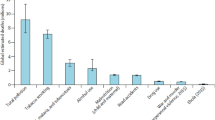
The rise in NCDs has been driven primarily by 4 major risk factors: tobacco use, physical inactivity, harmful use of alcohol, and unhealthy diets.
In 2014, NCDs represented the major share of the disease burden in Europe and were responsible for 86% of all deaths (European Commission 2014).
The growing burden of NCDs represents a major challenge for health systems: \(70\%-80\%\) of EU health care budgets are spent on NCDs (European Commission 2014).
The Nutri-Score scheme implemented in France in 2017 and later in several other European countries is based on a quantitative algorithm which ranks products on a qualitative scale from A being the healthiest to E being the unhealthiest.
Canada and New Zealand have similar healthy school-food standards (Nathan et al. (2016)).
In Vieux et al. (2012), reduced meat consumption supplemented isocalorically by fruit and vegetables induces an increase in GHG emissions, since some fruits and vegetables generate higher GHG emissions per calorie than dairy and non-ruminant meats. In Irz et al. (2019), the recommendation of a \(5\%\) decrease in the consumption of animal products raises GHG emissions in France (but not Denmark or Finland) by \(0.9\%\) due to the higher carbon content of the substitute products.
The DALY index measures the disease burden and is commonly used to analyse cost-effectiveness (Sassi 2006).
See Appendix A for the second-order conditions.
See Appendix A for the second-order conditions.
See Appendix A for the second-order conditions.
Parameters \(a_{1}\) and \(b_{1}\) move from 10 to 30 by 10; \(a_{2}\) moves from 1 to 2 by 1; \(b_{2}\) moves from 5 to 10 by 1; c moves from 1 to 5 by 1; d moves from 0.01 to 0.09 by \(0.01;\alpha\) moves from \(-5\) to 5 by 1; and n is equal to 10.
Parameters \(a_{1}\) and \(b_{1}\) move from 10 to 30 by 10; \(a_{2}\) moves from 1 to 2 by 1; \(b_{2}\) moves from 5 to 10 by 1; c moves from 1 to 5 by 1; d moves from 0.01 to 0.09 by \(0.01;\alpha\) moves from \(-5\) to 5 by 1; \(\gamma\) moves from 0.1 to 0.5 by 0.1; and n is equal to 10.
References
Aleksandrowicz L, Green R, Joy EJ, Smith P, Haines A (2016) The impacts of dietary change on greenhouse gas emissions, land use, water use, and health: a systematic review. PloS one 11(11):e0165797
Barrett S (1994) Self-enforcing international environmental agreements. Oxford economic papers, pp 878-894
Barrett S (2003) Environment and statecraft: the strategy of environmental treaty-making. Oxford
Bayramoglu B, Finus M, Jacques JF (2018) Climate agreements in a mitigation-adaptation game. J Publ Econ 165:101–113
Berger Richardson S, Lambek N (2018) Federalism and fragmentation: addressing the possibilities of a food policy for Canada. Canad Food Stud
Borrero M, Rubio SJ (2022) An adaptation-mitigation game: does adaptation promote participation in international environmental agreements? Int Environ Agreem Polit Law Econ Forthcom 22(3):439–479
Breton M, Sbragia L (2017) Adaptation to climate change: commitment and timing issues. Environ Resourc Econ 68(4):975–995
Carraro C, Siniscalco D (1993) Strategies for the international protection of the environment. J Publ Econ 52(3):309–328
Cornes R, Sandler T (1984) Easy riders, joint production, and public goods. Econ J 94(375):580–598
Cornes R, Sandler T (1994) The comparative static properties of the impure public good model. J Publ Econ 54(3):403–421
d’Aspremont C, Jacquemin A, Gabszewicz JJ, Weymark JA (1983) On the stability of collusive price leadership. Canad J Econ, pp 17–25
Ebert U, Welsch H (2012) Adaptation and mitigation in global pollution problems: economic impacts of productivity, sensitivity, and adaptive capacity. Environ Resourc Econ 52(1):49–64
Etilé F (2019) The economics of diet and obesity: public policy. In: Oxford Research Encyclopedia of Economics and Finance
European Commission (2014) The 2014 EU summit on chronic diseases. Brussels, 3 and 4 April 2014, Conference Conclusions. http://ec.europa.eu/health/major_chronic_diseases/docs/ev_20140403_mi_en.pdf (accessed 20 December 2018)
European Commission (2020) A farm to fork strategy for a fair, healthy and environmentally-friendly food system. In: Communication from the commission to the European parliament, the council, the European economic and social committee, and the committee of the regions. COM(2020) 381 final. https://ec.europa.eu/info/sites/info/files/communication-annex-farm-fork-green-deal_en.pdf (2020)
Finus M (2008) Game theoretic research on the design of international environmental agreements: insights, critical remarks, and future challenges. Int Rev Environ Resourc Econ 2(1):29–67
Finus M, Rübbelke DT (2013) Public good provision and ancillary benefits: the case of climate agreements. Environ Resourc Econ 56(2):211–226
Gerber PJ, Steinfeld H, Henderson B, Mottet A, Opio C, Dijkman J, Falcucci A, Tempio G (2013) Tackling climate change through livestock – a global assessment of emissions and mitigation opportunities. Food Agric Organ United Nations (FAO), Rome
Golombek R, Hoel M (2011) International cooperation on climate-friendly technologies. Environ Resourc Econ 49(4):473–490
Irz X, Jensen JD, Leroy P, Réquillart V, Soler LG (2019) Promoting climate-friendly diets: what should we tell consumers in Denmark, Finland and France? Environ Sci Polic 99:169–177
Kotchen MJ (2005) Impure public goods and the comparative statics of environmentally friendly consumption. J Environ Econ Manag 49(2):281–300
Kotchen MJ (2006) Green markets and private provision of public goods. J Polit Econ 114(4):816–834
Masoudi N, Zaccour G (2017) Adapting to climate change: is cooperation good for the environment? Econ Lett 153:1–5
Masoudi N, Zaccour G (2018) Adaptation and international environmental agreements. Environ Resourc Econ 71(1):1–21
Melaku YA, Renzaho A, Gill TK, Taylor AW, DalGrande E, deCourten B, Baye E, Gonzalez-Chica D, Hyppönen E, Shill Z, Riley M, Adams R (2018) Burden and trend of diet-related non-communicable diseases in Australia and comparison with 34 OECD countries, 1990–2015: findings from the global burden of disease study 2015. Eur J Nutr 58(3):1299–1313
Nathan N, Yoong SL, Sutherland R, Reilly K, Delaney T, Janssen L, Wolfenden L (2016) Effectiveness of a multicomponent intervention to enhance implementation of a healthy canteen policy in Australian primary schools: a randomised controlled trial. Int J Behav Nutr Phys Act 13(1):1–9
Poinsot R, Vieux F, Maillot M, Darmon N (2022) Number of meal components, nutritional guidelines, vegetarian meals, avoiding ruminant meat: what is the best trade-off for improving school meal sustainability? Eur J Nutr 61(6):3003–3018
Rubio SJ (2017) Sharing R &D investments in breakthrough technologies to control climate change. Oxford Econ Pap 69(2):496–521
Sassi F (2006) Calculating QALYs, comparing QALY and DALY calculations. Health Polic Plan 21(5):402–408
Springmann M, Godfray HCJ, Rayner M, Scarborough P (2016) Analysis and valuation of the health and climate change cobenefits of dietary change. Proc Natl Acad Sci 113(15):4146–4151
UNSCN (2017) Sustainable diets for healthy people and a healthy planet. United nations system standing committee on nutrition discussion paper. Rome
Vieux F, Darmon N, Touazi D, Soler LG (2012) Greenhouse gas emissions of self-selected individual diets in France: changing the diet structure or consuming less? Ecol Econ 75:91–101
Zehaie F (2009) The timing and strategic role of self-protection. Environ Resourc Econ 44(3):337
Funding
Funding was supported by Agence Nationale de la Recherche (DIET+, Grant No: ANR-17-CE21-0003), Agence Nationale de la Recherche (CLAND, Grant No: ANR-16-CONV-0003). Agence Nationale de la Recherche is not accountable for the content of this research.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
The authors are grateful for comments and suggestions from the co-editor and the three reviewers, which have helped to improve the paper considerably. We would like to thank Eugenie Dugoua, Marion Leroutier, and Julie Lochard for detailed comments and suggestions, and the participants of the economics seminar at PSAE (Paris, June 2020) and at CESAER (Dijon, October 2020), the FAERE Conference (Grenoble, September 2020), and the EAERE Conference (Manchester, June-July 2019) for useful comments.
Appendices
Appendices
A Second-Order Conditions
In Nash equilibrium, the Hessian matrix of the second derivative of the payoff function \(H^{N}\) is given by:
The first determinant of \(H^{N}\), \(D_{1}=B_{ee}-D_{EE}\), is negative by assumption a), and the second \(D_{2}=Det(H^{N})=B_{ee}\left( \gamma A_{ff}-C_{ff}-\alpha ^{2}D_{EE}\right) -D_{EE}(\gamma A_{ff}-C_{ff})\) is positive by assumptions a) and b). Thus, \(H^{N}\) is defined as positive, and \(U_{i}\) is strictly concave. Then, there is a unique solution to the optimization program (2), \((e^{N},f^{N})\) defined by equations 5 and 6.
At the full agreement, the Hessian matrix of the second derivatives of the welfare function, \(H^{C}\), is a symmetric matrix of size 2n with \(\frac{ \partial ^{2}W}{\partial e_{i}^{2}}=B_{ee}-nD_{EE}\), \(\frac{\partial ^{2}W}{ \partial e_{i}\partial e_{j}}=-nD_{EE}\), \(\frac{\partial ^{2}W}{\partial e_{i}\partial f_{j}}=n\alpha D_{EE}\), \(\frac{\partial ^{2}W}{\partial f_{i}^{2}}=\gamma A_{ff}-C_{ff}-n\alpha ^{2}D_{EE}\), and \(\frac{\partial ^{2}W}{\partial f_{i}\partial f_{j}}=-n\alpha ^{2}D_{EE}\quad \forall i,\,j.\) Assumptions a) and b), ensure that all the eigenvalues of the matrix \(H^{C}\) are negative; therefore, the welfare function is quasi-concave. As a result, there is a unique solution to the optimization program (23 ), \((e^{C},f^{C})\) defined by equations 24 and 25.
At the climate policy agreement, the Hessian matrix of the second derivatives of the welfare function is given by \(H^{P}\), a symmetric matrix of size n with \(\frac{\partial ^{2}W}{\partial e_{i}^{2}}=B_{ee}-nD_{EE}\), \(\frac{\partial ^{2}W}{\partial e_{i}\partial e_{j}}=-nD_{EE}\quad \forall i,\,j.\) Assumptions a) and b) ensure that all the eigenvalues of the matrix \(H^{P}\) are negative; therefore, the welfare function is quasi-concave. As a result, there is a unique solution to the optimization program (13), \((e^{P},f^{P})\) defined by equations 16 and 17.
B Proof of Proposition 1
Here, we investigate the reaction functions in Nash equilibrium.
The total differential of Equation (3) is:
The total differential of Equation (4) is:
Equations (B1) and (B2) can be written in matrix form:
-
(i).
Equation (B4) leads to \(\frac{de_{i}}{dET_{-i}}=\frac{(\gamma A_{ff}-C_{ff})D_{EE}}{Det(H^{N})}<0\), since \(A_{ff}<0\), \(D_{EE}>0\), and \(Det(H^{N})>0\).
-
(ii).
Equation (B4) leads to \(\frac{df_{i}}{dET_{-i}}=\frac{\alpha B_{ee}D_{EE}}{Det(H^{N})}\), with the sign depending on that of \(\alpha\), since \(B_{ee}<0\), \(D_{EE}>0\), and \(Det(H^{N})>0\).
-
(iii).
The first-order conditions reduce to \(\gamma A_{f}(f_{i})-C_{f}(f_{i})=\alpha B_{e}(e_{i})\). The total differential of this equation leads to \(\frac{de_{i}}{df_{i}}=\frac{\gamma A_{ff}-C_{ff}}{ \alpha B_{ee}}\) \(\Leftrightarrow sgn(\frac{de_{i}}{df_{i}})=sgn(\alpha )\) since \(A_{ff}<0\), \(C_{ff}>0\), and \(B_{ee}<0\).
C Proof of Proposition 2
Here, we investigate the reaction functions at the climate agreement solution. The total differential of Equation (14) is:
The total differential of Equation (15) is:
Equations (C1) and (C2) can be written in matrix form:
-
(i).
Equation (C4) leads to \(\frac{de_{i}}{dET_{-i}}=\frac{n(\gamma A_{ff}-C_{ff})D_{EE}}{Det(H^{P})}<0\), since \(A_{ff}<0\), \(C_{ff}>0\), \(D_{EE}>0\), and \(Det(H^{P})>0\).
-
(ii).
Equation (C4) leads to \(\frac{df_{i}}{dET_{-i}}=\frac{\alpha B_{ee}D_{EE}}{Det(H^{P})}\), with the sign depending on that of \(\alpha\), since \(B_{ee}<0\), \(D_{EE}>0\), and \(Det(H^{P})>0\).
-
(iii).
The first-order conditions reduce to \(n(\gamma A_{f}(f_{i})-C_{f}(f_{i}))=\alpha B_{e}(e_{i})\). The total differential of this equation leads to \(\frac{de_{i}}{df_{i}}=\frac{n(\gamma A_{ff}-C_{ff})}{ \alpha B_{ee}}\) \(\Leftrightarrow sgn(\frac{de_{i}}{df_{i}})=sgn(\alpha )\) since \(A_{ff}<0\), \(C_{ff}>0\), and \(B_{ee}<0\).
D Table of Results
Equilibria | With emissions from nutrition policy |
|---|---|
No agreement | \(B_{e}(e^{N})=D_{E}\left( n(e^{N}+\alpha f^{N})\right)\) |
\(\gamma A_{f}(f^{N})-C_{f}(f^{N})=\alpha D_{E}\left( n(e^{N}+\alpha f^{N})\right)\) | |
Agreement on climate policy | \(B_{e}(e^{P})=nD_{E}\left( n(e^{P}+\alpha f^{P})\right)\) |
\(\gamma A_{f}(f^{P})-C_{f}(f^{P})=\alpha D_{E}\left( n(e^{P}+\alpha f^{P})\right)\) | |
Full agreement | \(B_{e}(e^{C})=nD_{E}(n(e^{C}+\alpha f^{C}))\) |
\(\gamma A_{f}(f^{C})-C_{f}(f^{C})=\alpha nD_{E}(n(e^{C}+\alpha f^{C}))\) | |
Agreement on nutrition policy | \(B_{e}(e^{V})=D_{E}\left( n(e^{V}+\alpha f^{V})\right)\) |
\(\gamma A_{f}(f^{V})-C_{f}(f^{V})=\alpha nD_{E}\left( n(e^{V}+\alpha f^{V})\right)\) | |
Separate agreements | \(B_{e}(e^{S})=nD_{E}(n(e^{S}+\alpha f^{S}))\) |
\(\gamma A_{f}(f^{S})-C_{f}(f^{C})=0\) |
E Proof of Proposition 3
1.1 E.1 Part 1 of the Proof
We will use the method of proof by contradiction to compare the levels of the variables between the full agreement and the non-cooperative solution.
-
Suppose that \(\alpha >0\) and \(e^{N}\le e^{C}\); this implies that
-
Suppose that \(\alpha <0\) and \(e^{N}\le e^{C}\); this implies that
We will now use the method of proof by contradiction to compare the levels of the variables between the full agreement and the climate policy agreement.
-
Suppose that \(\alpha >0\) and \(e^{P}\ge e^{C}\); this implies that
-
Suppose that \(\alpha <0\) and \(e^{P}\ge e^{C}\); this implies that
We will now use the method of proof by contradiction to compare the levels of the variables between the climate policy agreement and the non-cooperative solution.
-
Suppose that \(\alpha >0\) and \(e^{N}\le e^{P}\); this implies that
-
Suppose that \(\alpha <0\) and \(e^{N}\le e^{P}\); this implies that
1.2 E.2 Part 2 of the Proof
1.2.1 E.2.1 For f
From first-order conditions on f ((6), (17), (25) and (11)) and
-
if \(\alpha >0\), we obtain that \(\gamma A_{f}(f^{k})-C_{f}(f^{k})>\gamma A_{f}(f^{S})-C_{f}(f^{S})=0\), for \(k=N,\,P,\,C.\)
Since \(G_ {ff}(f)<0\), \(f^k<f^S\) and \(0<\alpha f^k<\alpha f^S\), with \(k=N,\,P,\,C.\)
-
if \(\alpha <0\), we obtain that \(\gamma A_{f}(f^{k})-C_{f}(f^{k})< \gamma A_{f}(f^{S})-C_{f}(f^{S})=0\), for \(k=N,\,P,\,C.\)
Since \(G_ {ff}(f)<0\), \(f^k>f^S\) and \(\alpha f^k<\alpha f^S<0\), with \(k=N,\,P,\,C.\)
We obtain that
1.2.2 E.2.2 For e
We will use the method of proof by contradiction to compare the levels of the variables between different institutional arrangements.
Suppose that \(e^{k}\le e^{S}\) for \(k=P,\,C\); this implies that
Since \(e^{N}>e^{P}\) and \(e^{C}>e^{P}\), we obtain \(e^{S}<e^{P}<e^{C}<e^{N},\, \forall \alpha\).
1.2.3 E.2.3 For ET
From the ranking of individual emissions \(e^{S}<e^{P}<e^{C}\), we obtain:
which imply \(ET^{S}>\) \(ET^{P}\) and \(ET^{S}>ET^{C}\), respectively.
We will use the method of proof by contradiction to compare \(ET^{N}\) and \(ET^{S}\).
When \(\alpha <0\), suppose that \(ET^{N}\le ET^{S}\). This implies that \(\alpha f^{N}\ge \alpha f^{S}\) since \(e^{S}<e^{N}\). This in turn implies that \(f^{N}\le f^{S}\), which is not true (see part (2) of the proposition). Thus, we have \(ET^{N}>ET^{S}\).
When \(\alpha >0\), suppose that \(ET^{N}\le ET^{S}\). This implies that \(\alpha f^{N}\ge \alpha f^{S}\) since \(e^{S}<e^{N}\). This in turn implies that \(f^{N}\ge f^{S}\), which is not true (see part (2) of the proposition). Thus, we have \(ET^{N}>ET^{S}\).
1.3 E.3 Part 3 of the Proof
Let \(G(f)=\gamma A(f)-C(f)\).
In the case of the agreement on nutrition policy, the first-order conditions are given by:
Recall that the first-order conditions for the Nash equilibrium are:
The first-order conditions for the full agreement are:
1.3.1 E.3.1 For e
We will use the method of proof by contradiction to compare the levels of the variables between different institutional arrangements.
Suppose that \(e^{V}\le e^{N}\); this implies that
With the previous results, we obtain that
1.3.2 E.3.2 For f
Since \(e^{N}>e^{C}\), we have \(e^{V}>e^{C}.\)
-
if \(\alpha >0\), then \(f^{V}<f^{C}\). On the contrary, suppose that \(f^{V}>f^{C}\). Since \(G_{ff}(f)<0\), this implies that \(G_{f}(f^{V})<G_{f}(f^{C})\), and \(D_{E}(n(e^{V}+\alpha f^{V}))<D_{E}(n(e^{C}+\alpha f^{C}))\) from Eq. (E2) and (17). Thus, we have \(e^{V}+\alpha f^{V}<e^{C}+\alpha f^{C}\), which is a contradiction with \(e^{V}>e^{C}\) and \(f^{V}>f^{C}\). Thus, we have \(f^{V}<f^{C}\).
-
if \(\alpha <0\), then \(f^{V}>f^{C}\). On the contrary, suppose that \(f^{V}<f^{C}\). Since \(G_{ff}(f)<0\), this implies that \(G_{f}(f^{V})>G_{f}(f^{C})\), and \(D_{E}(n(e^{V}+\alpha f^{V}))<D_{E}(n(e^{C}+\alpha f^{C}))\) from Eq. (E2) and (17) because \(\alpha <0\). Thus, we have \(e^{V}+\alpha f^{V}<e^{C}+\alpha f^{C}\), which is a contradiction with \(e^{V}>e^{C}\)and \(f^{V}<f^{N}\). Thus, we have \(f^{V}>f^{C}\).
With the previous results, we obtain that
1.3.3 E.3.3 For ET
Equation (E1) and (5) imply \(D_{E}(n(e^{V}+\alpha f^{V}))<D_{E}(n(e^{N}+\alpha f^{N}))\). Thus, we have \(ET^{V}<ET^{N}\) \(\forall \alpha\).
We now compare \(ET^{V}\) and \(ET^{C}\).
From the ranking of nutrition policy \(f^{V}<f^{C}\) when \(\alpha >0\), we obtain:
This is also confirmed in the case of \(\alpha <0\). From the ranking of nutrition policy \(f^{V}>f^{C}\), when \(\alpha <0\), we obtain:
Thus, we have \(ET^{V}>ET^{C}\) \(\forall \alpha\).
The comparison of \(ET^{V}\) with \(ET^{P}\) and \(ET^{S}\) is not possible in the case of general functional forms.
F Quadratic Model
The quadratic functional forms should respect the model assumptions:
-
\(\left| \alpha f_{i}\right| <e_{i}.\) When \(\alpha >0\), this condition is equal to \(\left( e_{i}-\alpha f_{i}\right) >0\). When \(\alpha <0\), this condition is equal to \(\left( e_{i}+\alpha f_{i}\right) >0\).
-
\(D_{E}=d(ET)>0\) and \(D_{EE}=d>0\).
-
\(A_{f}=a_{1}-a_{2}f_{i}>0\) and \(A_{ff}=-a_{2}<0\).
-
\(C_{f}=cf_{i}>0\) and \(C_{ff}=c>0\).
-
\(B_{e}=b_{1}-b_{2}e_{i}>0\) and \(B_{ee}=-b_{2}<0\).
1.1 F.1 Nash Equilibrium
The reactions functions are given by:
The solution is given by:
The total payoff function then is given by:
1.1.1 F.1.1 Proof of Proposition 4 for the Nash Equilibrium
Here, we investigate how parameter \(\gamma\) affects the variables in Nash equilibrium, defined by the Eq. F1.
The effect of parameter \(\gamma\) on the level of nutrition policies is given by:
The numerator is positive thanks to the condition \(A_{f}=a_{1}-a_{2}f^{N}>0\), which is given by \(\left[ nd(\alpha ^{2}a_{1}b_{2}+\alpha a_{2}b_{1}+a_{1}c)+a_{1}b_{2}c\right] >0.\)
The effect of parameter \(\gamma\) on \(e^{N}\) depends on \(A_{f}=a_{1}-a_{2}f^{N}>0\) and on the sign of parameter \(\alpha\):
Similarly, the effect of parameter \(\gamma\) on the total level of emissions depends on \(A_{f}=a_{1}-a_{2}f^{N}>0\) and on the sign of parameter \(\alpha\):
1.2 F.2 Full Agreement
The solution is given by:
The total payoff function then is given by:
1.2.1 F.2.1 Proof of Proposition 4 for the Full Agreement
Here, we investigate how parameter \(\gamma\) affects the variables at the full agreement, defined by the equations F2.
The effect of parameter \(\gamma\) on the level of nutrition policies is given by:
The numerator is positive thanks to the condition \(A_{f}=a_{1}-a_{2}f^{C}>0\), which is given by \(\left[ n^{2}d(\alpha ^{2}a_{1}b_{2}+\alpha a_{2}b_{1}+a_{1}c)+a_{1}b_{2}c\right] >0.\)
The effect of parameter \(\gamma\) on \(e^{C}\) depends on \(A_{f}=a_{1}-a_{2}f^{C}>0\) and on the sign of parameter \(\alpha\):
Similarly, the effect of parameter \(\gamma\) on the total level of emissions depends on \(A_{f}=a_{1}-a_{2}f^{C}>0\) and on the sign of parameter \(\alpha\):
1.3 F.3 Agreement on climate policy
The solution is given by:
The total payoff function then is given by:
1.3.1 F.3.1 Proof of Proposition 4 for the Climate Policy Agreement
Here, we investigate how parameter \(\gamma\) affects the variables at the climate agreement, defined by the equations F3.
The effect of parameter \(\gamma\) on the level of nutrition policies is given by:
The numerator is positive thanks to the condition \(A_{f}=a_{1}-a_{2}f^{P}>0\), which is given by \(\left[ nd(\alpha ^{2}a_{1}b_{2}+\alpha a_{2}b_{1}+na_{1}c)+a_{1}b_{2}c\right] >0.\)
The effect of parameter \(\gamma\) on \(e^{P}\) depends on \(A_{f}=a_{1}-a_{2}f^{P}>0\) and on the sign of parameter \(\alpha\):
Similarly, the effect of parameter \(\gamma\) on the total level of emissions depends on \(A_{f}=a_{1}-a_{2}f^{P}>0\) and on the sign of parameter \(\alpha\):
1.4 F.4 Agreement on Nutrition Policy
The solution is given by:
The total payoff function then is given by:
1.4.1 F.4.1 Proof of Proposition 4 for the Agreement on Nutrition Policy
Here, we investigate how parameter \(\gamma\) affects the variables at the agreement on nutrition policy, defined by the equations F4.
The effect of parameter \(\gamma\) on the level of nutrition policies is given by:
The numerator is positive thanks to the condition \(A_{f}=a_{1}-a_{2}f^{V}>0\), which is given by \([a_{1}b_{2}c+nd(ca_{1}+\alpha n(\alpha a_{1}b_{2}+a_{2}b_{1})]>0.\)
The effect of parameter \(\gamma\) on \(e^{V}\) depends on \(A_{f}=a_{1}-a_{2}f^{P}>0\) and on the sign of parameter \(\alpha\):
Similarly, the effect of parameter \(\gamma\) on the total level of emissions depends on \(A_{f}=a_{1}-a_{2}f^{V}>0\) and on the sign of parameter \(\alpha\):
1.5 F.5 Separate Agreements
The solution is given by:
The total payoff function then is given by:
1.5.1 F.5.1 Proof of Proposition 4 for Separate Agreements
Here, we investigate how parameter \(\gamma\) affects the variables at the separate agreements equilibrium, defined by the equations F5.
The effect of parameter \(\gamma\) on the level of nutrition policies is given by:
The effect of parameter \(\gamma\) on \(e^{S}\) depends on the sign of parameter \(\alpha\):
Similarly, the effect of parameter \(\gamma\) on the total level of emissions depends on the sign of parameter \(\alpha\):
G Coalition Stability
1.1 G.1 Quadratic Case
Here, we consider the quadratic model exposed in section 5.1.
The timing of the coalition formation game is as follows:
\(\mathbf {Stage\ 1}\)
All countries choose simultaneously whether to join coalition \(P\subseteq N\) or to remain a singleton player. Countries \(i\in P\) are called signatories, and countries \(j\notin P\) are called non-signatories.
\(\mathbf {Stage\ 2}\)
All non-signatories \(j\notin P\;\)choose their economic strategies (levels of climate and nutrition policies) to maximize their individual payoffs, and all signatories \(i\in P\) do so to maximize the aggregate payoff to all coalition members. The choices of all the players are simultaneous.
Stage 1 is the cartel formation game, which originates from the industrial organization literature (d’Aspremont et al. 1983) and has been widely applied to study international environmental agreements (e.g., Barrett 1994; Carraro and Siniscalco 1993). This is an open-membership, single-coalition game because by assumption, i) membership in coalition P is open to all players, and ii) players can choose only between joining coalition P or remaining a singleton. Stage 1 requires the internal and externality stability conditions to be satisfied. Internal stability means that each player i that announces its intention to join coalition \(P^{*}\) will have no incentive to (unilaterally) change its strategy by leaving coalition \(P^{*}\). External stability means that each player j that announces its intention to remain a singleton (not to join coalition \(P^{*}\)) will have no incentive to (unilaterally) change its strategy and join coalition \(P^{*}\), given the equilibrium announcements of all the other players. As expected, the internal stability condition is more difficult to satisfy than the external stability condition.
The internal and external stability functions, which must be positive, can be written as follows:
Stage 2 follows the standard assumption in the literature on coalition formation: the coalition acts as a unique player, internalizing the externality among its members, whereas non-signatories act selfishly, maximizing their own payoff. We also follow the standard assumption of this literature and assume that signatories and non-signatories choose their economic strategies simultaneously. The two-stage coalition formation game is solved by backward induction.
1.1.1 G.1.1 Full Agreement
For a coalition of size p, the levels of nutrition and climate policy are, respectively (ns denotes a non-signatory and s denotes a signatory):
\(ET=\frac{\tfrac{n\gamma \alpha a_{1}}{\gamma a_{2}+c}+\tfrac{nb_{1}}{b_{2}} }{1+(n-p+p^{2})(\tfrac{\alpha ^{2}d}{\gamma a_{2}+c}+\tfrac{d}{b_{2}})};\,\,f^{s}=\frac{\gamma a_{1}-p\alpha dET}{\gamma a_{2}+c}\), \(f^{ns}=\frac{ \gamma a_{1}-\alpha dET}{\gamma a_{2}+c}\), \(e^{s}=\frac{b_{1}-dpET}{b_{2}}\) and \(e^{ns}=\frac{b_{1}-dET}{b_{2}}\).
We search for the size of the coalition for which the internal and external stability conditions are satisfied. We do not write the expressions for the internal and external stability conditions here because they are cumbersome. They are available from the authors upon request. In the quadratic model considered here, the stable size of the coalition cannot be derived analytically, in contrast to the linear-quadratic case (see below). We thus determine it numerically by using our initial parameter set described in section 5.1. We consider both cases for parameter \(\gamma\): its value is lower than 1 and larger than 1. We find that the stable size of the coalition is equal to 2, regardless of the sign of parameter \(\alpha\), that is whether the nutrition policy decreases or increases the GHG emissions generated by a country.
1.1.2 G.1.2 Agreement on Nutrition Policy
The countries do not cooperate on emissions \(e_{i}.\) Because we assume identical countries, individual emission levels are identical \(e_{i}=e^{V}.\)
The nutrition policy f is decided either cooperatively by the members, denoted by s as signatories, of the coalition of size p, or individually by the non-members of the coalition denoted by ns as non-signatories.
For a coalition of size p, the levels of nutrition policy and emissions are, respectively: \(ET=\frac{\tfrac{n\gamma \alpha a_{1}}{\gamma a_{2}+c}+ \tfrac{ nb_{1}}{b_{2}}}{1+(n-p+p^{2})(\tfrac{\alpha ^{2}d}{\gamma a_{2}+c})+ \tfrac{nd }{b_{2}}}\), \(f^{s}=\frac{\gamma a_{1}-p\alpha dET}{\gamma a_{2}+c}\), \(f^{ns}=\frac{\gamma a_{1}-\alpha dET}{\gamma a_{2}+c}\), \(e^{s}=e^{ns}= \frac{ b_{1}-dET}{b_{2}}\).
We search for the size of the coalition for which the internal and external stability conditions are satisfied. As for the full agreement, we do not write the expressions for the internal and external stability conditions here because they are cumbersome. They are available from the authors upon request.
In the quadratic model, the stable size of the coalition cannot be derived analytically, in contrast to the linear-quadratic case (see below). We thus determine it numerically by using our initial parameter set described in section 5.1. We consider both cases for parameter \(\gamma\): its value is lower than 1 and larger than 1. For this parameter set and when \(\alpha <0\), there is no stable coalition. Keeping the other parameter constellations unchanged, we now modify the value of parameter \(a_{2}:\) \(a_{2}\) now moves from 0.1 to 0.2 by 0.1, instead of from 1 to 2 by 1. In this case, we obtain the same result as for the full agreement. The stable size of the coalition is equal to 2, regardless of the sign of parameter \(\alpha\), that is whether the nutrition policy decreases or increases the GHG emissions generated by a country.
1.2 G.2 Linear-Quadratic Case
We consider the following payoff function:
with \(a_{1,}c,b_{1},b_{2},d,\) and \(\gamma\) positive constants. We note that the function \(G(f_{i})\) is at the maximum for \(f_{i}=\frac{\gamma a_{1}}{c}\), and the function \(B(e_{i})\) is at the maximum for \(e_{i}=\frac{b_{1}}{b_{2} }\).
1.2.1 G.2.1 Full Agreement
For a coalition of size p, the levels of nutrition and climate policy are, respectively (ns denotes a non-signatory and s denotes a signatory): \(f^{s}=\frac{\gamma a_{i}-p\alpha d}{c}\), \(f^{ns}=\frac{\gamma a_{i}-\alpha d }{c}\), \(e^{s}=\frac{b_{1}-dp}{b_{2}}\) and \(e^{ns}=\frac{b_{1}-d}{b_{2}}\). Thus, we note that regardless of the sign of \(\alpha\), the larger the coalition size is, the smaller the G and B functions.
This is an established finding for the B function in the literature on international environmental agreements but is a more novel finding for the G function. If \(\alpha >0\), the negotiated nutrition policy decreases with p because of its negative impact on the environment; if \(\alpha <0\), the nutrition policy increases with p because of its favourable impact on the environment, but it becomes increasingly costly as the coalition size increases, with the result that G decreases.
The stability function, which must be positive to respect internal stability, can be written as follows:
As is usual, the term in the second line related to climate policy is decreasing in p due to free-riding incentives (positive externality effect). The term in the third line \(2dp\frac{2\alpha ^{2}}{c}\) comes from consideration of the negative externality of GHG emissions on payoffs. It is positive and increasing in p, as the negative externality is internalized by more countries.
What are more interesting and new are the terms in the first line associated with the nutrition policy (the terms related to \(\alpha\)).
The decrease in the G and B functions as p increases means that the terms in \(\alpha\) in the first and second lines, \(-\gamma a_{1}\frac{\alpha d(p-1)}{c}+\frac{\alpha d(p-1)}{2}\left( \frac{2\gamma a_{1}-(p+1)\alpha d}{c }\right)\) are negative. For the nutrition policy (thus the terms in \(\alpha\)), we need to know whether the term \(2d(p-1)\frac{2\alpha ^{2}}{c}\) is larger (in absolute value) than the expression \(-\gamma a_{1}\frac{\alpha d(p-1)}{c}+\frac{\alpha d(p-1)}{2}\left( \frac{2\gamma a_{1}-(p+1)\alpha d}{c }\right)\). We note that the first term grows linearly with p but the second term decreases quadratically with p. Thus, the difference in the terms in \(\alpha\) is negative, and so the nutrition policy reduces the stability function. In other words, the nutrition policy makes formation of a large coalition more difficult.
The three terms ultimately simplify to the following expression for the stability function:
The stability function is strictly positive for \(p=2\) and null for \(p=1\) and \(p=3\). For \(p\ge 3\), external stability (\(S(p+1)<0)\) is respected. The stable size of the coalition is thus equal to 3.
1.2.2 G.2.2 Agreement on Nutrition Policy
The countries do not cooperate on emissions \(e_{i}.\) Because we assume identical countries, individual emission levels are identical \(e_{i}=e^{V}.\) The nutrition policy f is decided either cooperatively by the members, denoted by s as signatories, of the coalition of size p, or individually by the non-members of the coalition denoted by ns as non-signatories.
The first-order conditions for the agreement on nutrition policy with a coalition of size p are then:
For a coalition of size p, the levels of nutrition policy and emissions are, respectively: \(f^{s}=\frac{\gamma a_{i}-p\alpha d}{c}\), \(f^{ns}=\frac{ \gamma a_{i}-\alpha d}{c}\), \(e^{s}=\frac{b_{1}-dp}{b_{2}}\) and \(e^{ns}=\frac{ b_{1}-d}{b_{2}}\).
We can then express the stability function, which must be positive to respect the internal stability condition:
The stability function is strictly positive for \(p=2\) and null for \(p=1\) and \(p=3\). For \(p\ge 3\), external stability (\(S(p+1)<0)\) is respected. The stable size of the coalition is thus equal to 3.
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Bayramoglu, B., Jacques, JF. & Poret, S. Nutrition and Climate Policies in the European Union: Friends or Enemies?. Environ Resource Econ 86, 807–849 (2023). https://doi.org/10.1007/s10640-023-00815-0
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10640-023-00815-0