Abstract
The linear dynamic viscoelastic properties and non-linear transient rheology of sodium carboxymethylcellulose solutions (Na-CMC) in propylene glycol/water mixtures were investigated. Measurements were carried out for the solutions of Na-CMC with three different degrees of substitution (DS), namely 0.62, 0.79, 1.04, and the similar average molecular weight (Mw ≈ 250,000 g/mol). The strong synergism between the molecules of Na-CMC with DS of 0.62 and 0.79, and propylene glycol has been observed. The occurrence of the overshoot shear stress and the low loss tangent values indicate the physical cross-linking of the polymer chains. The increase of propylene glycol concentration over 80 wt% and sodium carboxymethylcellulose (DS = 0.7) over 1.6 wt% leads to the formation of a physical cross-link network. The absence of overshoot shear stress and terminal behaviour in SAOS flow of the Na-CMC1.04 solutions in the PG/water mixture shows that no intermolecular cross-linking of polymer chains occurred in them.
Similar content being viewed by others
Avoid common mistakes on your manuscript.
Introduction
The sodium salt of carboxymethylcellulose (Na-CMC) is a water-soluble derivative of cellulose which has found applications in a number of industrial sectors including food, paints, pharmaceuticals and cosmetics (Li et al. 2009; Kono et al. 2016). It serves as a viscosity modifier, thickener, emulsion stabilizer, and water-retention agent (Li et al. 2009). Na-CMC is also used in the production of hydrogels with biomedical applications (drug delivery, tissue engineering) (Ali et al. 2016).
In the majority of cases, Na-CMC-based hydrogels are obtained through chemical cross-linking as a result of reaction with bifunctional cross-linking agents (for example: epichlorohydrin (Chang et al. 2010), diepoxy (Rodriguez et al. 2003; Kono et al. 2013), dicarboxylic acid (Gorgieva and Kokol 2011; Akar et al. 2012), aldehydes (Patenaude and Hoare 2012) and polyethylene glycol (Kono 2014). Also, the synthesis of multi-component hydrogels based on carboxymethyl cellulose have been reported (Maswal et al. 2015).
So far, there have been relatively few reports on the physical cross-linking gel of Na-CMC. Gels of this type can be obtained by dissolving Na-CMC in 0.1 M of HCl (Gulrez et al. 2011) or adding poly(vinyl alcohol) to an aqueous solution of Na-CMC (Congming and Yongkang 2008). Yang and Zhu (2007) have observed that the viscosity of Na-CMC aqueous solutions rises rapidly after the addition of glycerin. In all enumerated cases, gel formation or an increase in the viscosity of a Na-CMC solution is associated with physical cross-linking through hydrogen bonds. Sharma et al. (2010), and Chatterjee and Das (2013), have presented results of measurements of intrinsic viscosity in ethylene glycol/water and methanol/water mixtures. The studies investigated the conformation of Na-CMC macromolecules in diluted solutions.
The rheological properties of Na-CMC aqueous solutions are relatively well known. Their viscosity depends not only on the average molecular weight and the distribution of molecular weights, but also on the degrees of substitution (DS) at the 2-, 3-, and 6-positions along the cellulose chains of CH2COONa groups, and on the total DS (Kono et al. 2016). Studies conducted by Kulicke et al. (1996) show that the maximum zero shear viscosity of Na-CMC aqueous solutions occurs at DS ≈ 0.9. Changes in the viscosity of Na-CMC aqueous solutions accompanying an increase in DS are most likely related to the presence of inter- and intrachain hydrogen bonds (Kulicke et al. 1999). The interchain hydrogen bonds which have the greatest impact on the viscosity of the solution form primarily between O6H6 groups and CH2COONa groups.
In this paper, both the linear and nonlinear transient rheology of Na-CMC solutions in propylene glycol/water mixtures were studied. The main purpose of the study was to determine the effect of the degree of substitution on the rheological properties of Na-CMC/PG/water mixtures. Furthermore, rheological measurements were performed for solutions with varying concentrations of the polymer and propylene glycol. Na-CMC solutions with a low content of propylene glycol (ca. 20 wt% of PG) have found application in the production of hydrogel polymer wound dressings. The study below presents results of measurements obtained for solutions containing between 60 and 95 wt% of PG. Systems of this type, similarly to solutions of other polysaccharides in PG/water and glycerol/water mixtures, may be potentially employed as drug delivery systems because of their high viscosity (Lin et al. 1993; Moser et al. 2001).
Experimental
Materials
Three sodium carboxymethylcellulose with different degrees of substitution (DS = 0.7, 0.9 and 1.2—based on manufacturer’s data) and with a similar average molecular weight (Mw = 250,000 g/mol—based on manufacturer’s data) were obtained from Sigma–Aldrich. Propylene glycol (PG) used in the study was supplied by Donauchem with a purity of 99.94 wt% (PG for pharmaceutical use).
The DS values specified by the manufacturer were verified using a standard method (ASTM 1961; Toğrul and Arslan 2003). A 0.5 g portion of Na-CMC was shaken with 20 ml of a HNO3–methanol mixture (10.8 ml of 65% HNO3 was made to 100 ml with methanol) and left aside for 3 h, after which surplus acid was washed with 70% methanol. Next, a 0.2 g portion of Na-CMC, previously dried at 55 °C for 1 h, was dissolved in 20 ml of distilled water and 3 ml of 1 M NaOH. A detailed description of the method can be found, for example, in a study by Toğrul and Arslan (2003). The DS values thus determined were lower than those specified by the manufacturer and amounted to 0.62, 0.79 and 1.04. For the distinction of polymers with different degrees of substitution the following notations are used Na-CMC0.62 for DS = 0.62; Na-CMC0.79 for DS = 0.79 and Na-CMC1.04 for DS = 1.04.
Size-exclusion chromatography
The molar mass distributions of Na-CMC samples were characterized by size-exclusion chromatography (SEC) with triple detection on the chromatograph composed of Knauer K-501 HPLC pump with LDC RI detector and Viscotek T60A dual detector (right angle laser light scattering at λ = 670 nm (RALLS) and differential viscometer). Three TSK-GEL columns: G5000 PWXL + 3000 PWXL + 2500 PWXL (7.8 mm × 300 mm; Tosoh Corporation, Tokyo, Japan) were used in a series. The analysis was performed at 26 °C with the mobile phase 0.1% NaN3 at a flow rate of 0.1 ml/min. The system was calibrated by poly(ethylene oxide)s standards. The number average molecular weight (Mn) and weight average molecular weight (Mw) were calculated by means of OmniSEC software (Viscotek) (Lapienis et al. 2015).
Raman spectroscopy
Raman spectrometry was conducted (DXR Raman Microscope, Thermo Fischer Scientific, USA) with a laser wavelength of 622 nm. A 1 cm3 portion of the Na-CMC solution was placed in a quartz cuvette and subjected to Raman spectroscopy measurements. Each spectrum was recorded 5 times during the collection time of 30 s. The aperture was 25 µm slit with 4.2 cm−1 resolution. The laser power was 5 mW with a spot size of 0.1 µm. The sample was investigated in ten different locations.
Preparation of solutions
Depending on the concentration (Na-CMC and propylene glycol) preparation of solutions were done in two ways. All the solutions with concentrations below 1.6 wt% of Na-CMC and 80 wt% of PG were prepared at room temperature. The required amount of Na-CMC was added gradually to a beaker with exactly measured amount of distilled water. The solutions were at the same time mixed with use of a magnetic stirrer for the next 24 h to ensure complete hydration of Na-CMC. Then the propylene glycol was gradually added and thus obtained mixture was stirred for 24 h.
The solutions with the Na-CMC concentration of above 1.6 wt% and the PG concentration of above 70 wt% obtained through the above procedure were heterogeneous, which is why they were prepared according to the method detailed below. As the initial step, solutions containing lower Na-CMC and PG concentrations were prepared according to the first procedure. As the next step, the obtained Na-CMC/PG/water mixture was heated to the temperature of 40 °C and the excess of water was slowly evaporated. During evaporation, the sample was constantly stirred and weighed in order to achieve specific Na-CMC and PG concentrations. A higher temperature was not desirable in view of the risk of sample degradation. The time needed to evaporate the water depends on the concentrations of Na-CMC and PG (from 8 to 14 h). It was possible to prepare Na-CMC/PG/water solutions according to the procedure described above due to low PG vapour pressure. Also, the aqueous solution of PG was concentrated from 70 to 80 wt%. The viscosity of the concentrated solution was 0.8% lower than the viscosity of the solution of the same concentration obtained by mixing the ingredients.
Preliminary rheological measurements showed the Na-CMC/PG/water solutions to be time-dependent fluids. During the preparation phase the solutions were stirred mechanically, so it was necessary to determine the time after which their microstructure was restored. To this end, a strain sweep experiment was conducted at intervals of approximately 24 h. Between successive measurements the samples were stored at 4 °C. It was demonstrated that repeatable results for the Na-CMC0.62/PG/water solutions (PG concentration ≥ 80%) were obtained after about 9 days. Further measurements revealed the samples with a high PG content to be stable even after 30 days. On that basis, the period between sample preparation and measurements was set at 14 days. Studies on aqueous solutions were carried out 48 h after the preparation.
Rheological measurements
All rheological experiments were performed in a stress and strain controlled rheometer (Anton-Paar Physica MCR 501) at 20 °C. Small amplitude oscillatory shear measurements were performed using 60 mm diameter parallel plates (gap 1 mm). The sample introduced into the measuring system was maintained at rest for 4 min in order to eliminate residual stress histories. The linear viscoelastic range of each solution was identified by oscillatory strain sweep test which was conducted by increasing the strain amplitude γ 0 from 0.01 to 1000% at a constant frequency of 1 Hz. The range of linear viscoelasticity for all the solutions studied ended at the strain amplitude value γ0 ≥6%. The frequency sweep experiments were conducted at γ0 = 2%. The parameters obtained from the dynamic test data were: storage modulus G′ and loss modulus G″. All rheological tests were performed at a stabilized temperature of 20 ± 0.1 °C. In addition, all the samples before measurements were stabilized in water bath for 2 h at 20 °C.
For the transient rheological measurements, cone-and-plate geometry (diameter—59.974 mm, angle—2.014°, truncation—253 μm) was used. The growth in transient shear stress was measured as a function of time. The sample loading procedures were essentially the same as those used in the SAOS tests described above. The measurements were performed at four different shear rates (0.01, 0.1, 1 and 10 s−1).
Results and discussion
Raman spectroscopy
Figure 1 shows the Raman spectra for the Na-CMC0.62; Na-CMC0.79 and Na-CMC1.04 solutions in the PG/water mixture. The fingerprint spectra for sodium carboxymethylcellulose confirmed the molecular structure of the compound skeleton. Several bands recorded for the solutions with different DS reflected minor changes in the region assumed to be responsible for hydrogen bonding (2600–3500 cm−1) or—more specifically—in the OH stretching region. The intensity of the spectra recorded for different solutions depends on the Na-CMC DS level; it was then assumed that hydrogen associates had been formed between OH groups coming from PG and OH groups along the cellulose chain. Furthermore, the increase in intensity at 3270 and 3390 cm−1 suggests that there are several types of hydrogen bonding; 3270 cm−1 possibly reflects the intrachain hydrogen bonds O2–H2…O6, and 3390 cm−1 confirms the bonding between the cellulose chain OH group from PG.
A quantitative analysis of these spectra is impossible since the precise amount of PG contributing to the hydrogen association is impossible to determine. On the other hand, it seems that the presence of PG strongly influences the Raman response and also depends on the substitution degree. To some extent, it might suggest that the specific bonds and their excitation energies strongly correlate with the polymer structure and accessibility of the chain to the OH groups from glycol. Finally, the solutions prepared with glycol demonstrated increased intensities in the OH stretching region, with a decrease on the DS level.
Dynamic rheological properties
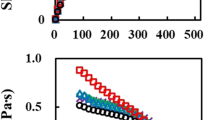
The linear viscoelasticity measured using small amplitude oscillatory shear (SAOS) is presented in Fig. 2a for sodium carboxymethylcellulose aqueous solutions with varying degrees of substitution. The Na-CMC0.79 and Na-CMC1.04 solutions satisfy the relation G″ ∝ ω, and the modulus G′ is proportional to ω1.13 and ω1.57, whereby G″ >G′. A different nature of the relations G′ = f(ω) and G″ = f(ω) is noted for the Na-CMC0.62 aqueous solution. The loss modulus are also greater than the storage modulus, however the scaling exponents are similar in value, amounting to 0.97 and 0.91 for G′ and G″, respectively.
Changes in the mechanical spectrum noted for the sodium carboxymethylcellulose aqueous solutions with varying degrees of substitution may be associated with the polydispersity of the polymer. However, measurements of molecular mass distributions performed by size-exclusion chromatography show that the polydispersity indexes (PDI = Mw/Mn) are similar in value for sodium carboxymethylcellulose used in the studies (for DS 0.62; 0.79 and 1.04, they are equal to 2.09 (Mw = 264,400 g/mol); 1.95 (Mw = 242,200 g/mol) and 1.83 (Mw = 262,400 g/mol), respectively). This shows that differences in the scaling exponents for the Na-CMC solutions with varying DS will be related to interactions between the polymer macromolecules (Lewis et al. 2014; Shabbir et al. 2015).
Xiquan et al. (1990) demonstrated in X-ray diffraction experiments that in the sodium carboxymethylcellulose aqueous solution for DS ≤0.82 the derivative crystalline regions are present, i.e. the polymer chains are aggregated by hydrogen bonds. The occurrence of aggregates in aqueous solutions of Na-CMC with low degree of substitution was also reported by Kulicke et al. (1999) (although the authors do not mention about the crystalline regions). As a consequence, the similar scaling exponents for Na-CMC0.62 may result from the presence of aggregates. Figure 3 shows a representation of hydrogen bonds found in an aqueous solution of sodium carboxymethylcellulose (DS = 0.7), as proposed by Li et al. (2009). It follows from it that the interchain hydrogen bonds formed mainly between O6H6 and COONa. As DS grows, there is a decrease in the number of O6H6 groups and an increase in the number of COONa groups, the presence of which may lead to the destruction of interchain hydrogen bonds and the disappearance of aggregates. Consequently, at high DS values, sodium carboxymethylcellulose is molecularly dispersed in water (Lopez et al. 2015), and the scaling exponent assumes values that are similar to those characteristic for liquid-like terminal behaviour (G′ ∝ ω 2; G″ ∝ ω). A deviation of the scaling exponent from the value of 2 for Na-CMC1.04 may be a result of polymer polydispersity.
The scheme of hydrogen bonds in sodium carboxymethylcellulose aqueous solutions proposed by Li et al. (2009)
Figure 2b shows mechanical spectra obtained for the sodium carboxymethylcellulose solutions dissolved in a PG/water mixture with varying degrees of substitution (with the concentration of PG amounting to 80 wt%). At low frequencies, the Na-CMC1.04 solution exhibits typical terminal behaviour for both G′ (∝ ω 2) and G′′ (∝ ω). At the highest achievable angular frequencies, the values of both modulus are similar, and their slope is approximately 0.5. Such a shape of the mechanical spectrum is characteristic for unentangled polymer solutions (Lu et al. 2015). A difference is noted in the relation between G′ and G″ as a function of angular frequency in the sodium carboxymethylcellulose solutions with DS values equal to 0.62 and 0.79. In this case, the slope of the modulus G′ rises along with an increase in angular frequency from 0.19 to 0.45 for Na-CMC0.62, and from 0.23 to 0.54 for Na-CMC0.79. The slope of the modulus G″ is roughly constant from the value ω = 0.019 rad/s, and equals 0.46 for Na-CMC0.62 and 0.51 for Na-CMC0.79. In addition, both in Na-CMC0.62 and Na-CMC0.79, the values of the modulus G′ are greater than those of the modulus G″ over the entire range of changes in angular frequency. The change in the slope of the modulus G′ noted for the Na-CMC0.62 and Na-CMC0.79 solutions may point to the fact that the measurements were performed in the angular frequency range in which there is a passage from the plateau zone to the transition zone. Differences in the shape of the mechanical spectra obtained for the solutions containing Na-CMC1.04 and Na-CMC0.62 and Na-CMC0.79 show that the lowering of the degree of substitution leads to the cross-linking of the polyion molecules. Figure 2b additionally shows photographs of the sodium carboxymethylcellulose solution samples. The flow of the fluid in a slanted test tube is clearly visible only in the Na-CMC1.04 solution. In the two remaining cases, no change in the location of the fluid in the test tube is visible even after 60 s (i.e. the time point when the photograph was taken).
Yang and Zhu (2007) have observed a synergistic effect manifested as a considerable increase in the viscosity of the solution or the formation of gel in Na-CMC/glycerine/water and Na-CMC/Al(OH)3/water systems. The effect is attributed to the ability of OH groups to cross-link Na-CMC polyions in the solution through a hydrogen bond. Probably a similar mechanism of cross-linking occurs in the Na-CMC0.62/PG/water and Na-CMC0.79/PG/water solutions. The Raman spectroscopy results show that the number of hydrogen bonds between OH groups of propylene glycol and Na-CMC macromolecules, increases with a decrease of the DS level. Since PG contains two OH groups, the physical cross-linking of the chain in the solution can occur, resulting in an increase in the value of the modulus G′ and G″ and, following the formation of a cross-link network, in the formation of a weak physical gel. At the same time, the results of the SAOS experiments indicate that a weak physical gel was formed exclusively in the Na-CMC0.62 and Na-CMC0.79 solutions, whereas the Na-CMC1.04 solution has the properties of a viscoelastic fluid. Furthermore, the values of the modulus G′ for Na-CMC with DS = 0.62 are greater than for DS = 0.79. This signifies that the number of interchain cross-links of the Na-CMC macromolecules increases along with a decrease in the polyion degree of substitution.
As previously mentioned, in aqueous solutions of Na-CMC1.04 a large number of COONa groups prevents the interchain hydrogen bond formation, which is evidenced by the lack of aggregates (Na-CMC1.04 is molecularly dispersed in water) (Lopez et al. 2015). The absence of cross-linking of Na-CMC1.04 macromolecules in the aqueous solutions is most likely an effect of their solvation (Xiquan et al. 1990). Probably, also in the PG/water mixture the similar effect might occur. However, it needs to be noted that rheological studies alone are not sufficient to fully elucidate the microstructure of the fluid, and the described causes of changes in the rheological properties of Na-CMC/PG/water mixtures accompanying an increase in DS need to be verified by other measurement methods.
A parameter which characterizes the viscoelastic behaviour of fluid is loss tangent (tan δ = G″/G′). Generally, tan δ < 1 indicates a dominance of elastic fluid properties, and tan δ > 1 shows a dominance of viscous fluid characteristics. The effect of Na-CMC0.62 and PG concentrations on changes in tan δ as a function of angular frequency is shown in Fig. 4.
For solutions with the Na-CMC0.62 concentration of 1.6 wt% and the PG concentration of 70 wt%, the values determined for tan δ are >1, which shows a fluid-like behaviour of the sample (Fig. 4b). An increase in the PG concentration to 80 wt% causes a rapid drop in the loss tangent value. Changes are also noted in the qualitative characteristics of the relation tan δ = f(ω). In solutions where the PG concentration is ≥80 wt%, the loss tangent values increase in line with rising frequencies from a value within the range from 0.11 to 0.17 at 0.0056 rad/s to a value ranging between 0.76 and 1.0 at 50 rad/s.
Rapid changes in the loss tangent values are also observed at the increasing concentration of Na-CMC0.62 and steady concentration of PG (80 wt%) (Fig. 4a). A rise in the concentration of Na-CMC0.62 from 1.2 to 1.4 wt% results in a considerable decrease in tan δ, however its values are still greater than 1 at ω >3.4 rad/s. A further increase in the polymer concentration induces primarily a reduction in the loss tangent value at higher angular frequencies (ω > 4 rad/s). Notably, for the highest concentrations (≥1.8 wt%) its values are similar over the entire angular frequency range. The finding shows that the fluid microstructure was fully formed (Winter et al. 1988). At the same time, the relatively high values of the loss tangent (>0.1) and the fact that they increase in line with increasing angular frequencies show that the samples are not true gels but rather weak biopolymer gels (Mandala et al. 2004; Razmkhah et al. 2017).
The photographs in Fig. 4a, b demonstrate that an increase in the concentration of PG from 80 to 85 wt%, and a rise in the concentration of Na-CMC0.62 from 1.6 to 1.8 wt%, trigger a rapid change in the rheological properties of the solutions. A clear flow is observed in the samples with the PG concentration of 70 wt% and the Na-CMC0.62 concentration of 1.6 wt%. A sample of the solution with the PG and Na-CMC0.62 concentrations of 80 and 1.8 wt%, respectively, still retains its shape after 60 s.
Transient rheology
Figure 5 presents responses of the transient shear stress as a function of strain (\(\gamma = \dot{\gamma } \cdot t\)), for the initial start-up experiments after different rest times for the Na-CMC0.62 (2.0 wt%) solutions in PG/water mixtures containing 80 wt% of PG. A fresh sample (i.e. a sample resting for 14 days prior to the commencement of the test) can be observed to reach the maximum shear stress (overshoot stress, τmax) before it achieves the equilibrium value. The overshoot stress was noted in a large number of systems, for example entangled polymer melts and solutions (Ravindranath and Wang 2008; Hernadez et al. 2013; Lu et al. 2014), nanocrystalline cellulose suspensions, hydrogels (Yin et al. 2008; Mahi and Rodrigue 2012; Bagheriasl et al. 2016; Chen et al. 2017), organoclay nanocomposites (Letwimolnun et al. 2007; Chen et al. 2017). The initial monotonic increase in the shear stresses is proof for the occurrence of elastic deformation of polymer chains (Ravindranath and Wang 2008; Wang and Wang 2009). In the wake of structural changes at the stress overshoot the shear stress begins to decrease, which means a transformation from the initial elastic deformation to the final plastic deformation, i.e. flow (Wang and Wang 2009). In the Na-CMC0.62 solutions, the formed network is probably a result of hydrogen bonding between OH groups of propylene glycol and the polymer chains. It follows that the observed overshoot shear stress should be associated with the breakdown of the network following the breaking of hydrogen bonds.
Figure 5 also shows the results of start-up experiments obtained at various time intervals after pre-shearing at \(\dot{\gamma } = 100\,{\text{s}}^{ - 1}\) for 30 s. The stress overshoot, which is not visible for short rest times (1 and 60 s), increases regularly with rest times ranging from 60 to 960 s. After 960 s the value of \(\tau_{\hbox{max} }^{ + }\) and the equilibrium value of shear stress are approximately a half lower than those recorded for the fresh sample. The finding indicates the process of very slow reconstruction of the fluid microstructure. The measurement results discussed below relate to fresh samples.
Figure 6 shows the transient stress responses to the start-up of the shear flow for the sodium carboxymethylcellulose (2.2 wt%) solutions with varying DS in PG/water mixtures containing 90 and 95 wt% of GP. The overshoot shear stress occurs in the Na-CMC0.62 and Na-CMC0.79 solutions at the PG concentration of 95 wt%. In the Na-CMC1.04 solution, the shear stress is not observed to change in the function of time. A comparison of the nature of the relation τ+ = f(γ) for the sodium carboxymethylcellulose solutions with DS equal to 0.62 and 0.79 also shows that in the polymer solution with a lower degree of substitution the maximum stress occurs at a lower strain value (γmax). An increase in τmax together with a reduction in γmax along with decreasing DS points to the formation of a greater number of cross-links between sodium carboxymethylcellulose chains. This decreases the entanglement length, i.e. the average distance along the sodium carboxymethylcellulose chain between two junction points in the polymer network. A reduced entanglement length most probably results in the breaking of cross-links at lower strain values. At the same time, the absence of overshoot shear stress in the Na-CMC1.04 solutions provides additional evidence suggesting that it was not affected by the intermolecular cross-linking of polymer chains.
Figure 7a shows results obtained in the measurements of transient shear stress for four shear rates (0.01; 0.1; 1 and 10 s−1) for solutions at the Na-CMC0.62 and PG concentrations of 2.0 and 80 wt%, respectively. The maximum transient shear stress is recorded roughly at the same strain value γmax (3.3 ± 0.3), which means that the time of the stress maximum is \(t_{peak} \propto \dot{\gamma }^{ - 1}\). The strain scaling of the stress is characteristic for textured liquid crystalline polymers and non-Brownian suspensions and other a highly elastic fluid (Solomon et al. 2001; Chatterjee and Krishnamoorti 2008; Kagarise et al. 2010; Ji-Seok et al. 2015). Figure 7b additionally presents images obtained using a polarized light microscope (fluid at rest) and a glass parallel plates system placed between crossed polarizers (fluid at rest and at the shear rate of 0.1 s−1). The images did not show the presence of a liquid crystalline-like structure in any of the cases.
Transient shear stress versus strain at various shear rates (a) and crossed polarizers pictures (b) (1—cross-polarization microscope; 2—parallel plates, \(\dot{\gamma } = 0\,{\text{s}}^{ - 1}\); 3—parallel plates, \(\dot{\gamma } = 0.1\,{\text{s}}^{ - 1}\)) for Na-CMC/PG/water mixture (2.0 wt% of Na-CMC0.62 and 80 wt% of PG)
Figures 8 and 9 show the relation between transient shear stress and strain for solutions with varying Na-CMC0.62 and PG concentrations. The overshoot shear stress occurs at the polymer concentration of 1.8 wt% (GP concentration: 80 wt%) and the PG concentration equal to 85 wt% (Na-CMC0.62 concentration: 1.6 wt%). The concentration values given above are consistent with the concentrations at which weak physical gel formation was identified based on the SAOS measurements (Fig. 4). At lower polymer and PG concentrations the value of transient shear stress initially also rises, however then stabilizes at a steady level. The initial increase in τ+ suggests the presence of elastic deformation in the samples, which most likely arises from a partially formed fluid microstructure. However, there was no formation of a network which—when broken—would result in the maximum value of transient shear stress.
Data shown in Fig. 9b reveal, at the same time, that increasing the concentration of PG from 85 to 90 wt% causes a reduction in the strain value γmax, at which the shear stress overshoot from 3.5 ± 0.1 to 3.0 ± 0.1 occurs, suggesting the formation of a greater number of junctions. Increasing the concentration of PG to 95 wt% triggers an increase in transient shear stress, whereas γmax continues to have the same value as in the solution with the concentration of 90 wt% (γmax = 3.0 ± 0.1). The results are consistent with the SAOS measurements (Fig. 4b) which also demonstrate that a physical cross-link network is fully formed at the PG concentration of 90 wt%. The observed transient shear stress increase accompanying the PG concentration change from 90 to 95 wt% can be accounted for by an increase in the viscosity of the PG/water mixture.
Conclusion
The study presents results obtained in measurements of small amplitude oscillatory shear flow and transient shear flow performed for sodium carboxymethylcellulose with varying degrees of substitution in a PG/water mixture. Strong synergy was found between the molecules of sodium carboxymethylcellulose with DS equal to 0.62 and 0.79, and propylene glycol. The occurrence of overshoot shear stress, coupled with low loss tangent values, point to the physical cross-linking of the chain in the solution. A hypothetical mechanism was proposed, under which the cross-linking of the Na-CMC0.62/PG/water and Na-CMC0.79/PG/water solutions resulted from the formation of hydrogen bonds between the OH groups of propylene glycol and sodium carboxymethylcellulose macromolecules. An increase in the concentrations of PG and sodium carboxymethylcellulose, coupled with a decrease in DS, lead to the emergence of a greater number of junction, which ultimately results in the formation of a physical cross-link network. The absence of overshoot shear stress and terminal behaviour in SAOS flow of the Na-CMC1.04 solutions in the PG/water mixture shows that no intermolecular cross-linking of polymer chains occurred in them. Although a more in-depth analysis is necessary, a correlation seems to exist between a decrease in the number of O6H6 groups in a sodium carboxymethylcellulose molecules accompanying an increase in DS and a reduction in cross-linking density.
References
Akar E, Antinişk A, Seki Y (2012) Preparation of pH-and ionic-strength responsive biodegradable fumaric acid crosslinked carboxymethylcellulose. Carbohydr Polym 90:1634–1641. doi:10.1016/j.carbpol.2012.07.043
Ali A, Maryam DS, Alireza A, Ghanbar E (2016) Preparation and characterization of sodium carboxymethyl cellulose/silk fibroin/graphene oxide nanocomposite films. Polym Test 52:218–224. doi:10.1016/j.polymertesting.2016.03.020
ASTM (1961) Tentative methods of testing sodium carboxymethyl cellulose. ASTM: D1439-61T, pp 1164–1173
Bagheriasl D, Carreau PJ, Riedl B, Dubois C, Hamad WY (2016) Shear rheology of polylactide (PLA)–cellulose nanocrystal (CNC) nanocomposites. Cellulose 23:1885–1897. doi:10.1007/s10570-016-0914-1
Chang C, Duan B, Cai J, Zhang L (2010) Superabsorbent hydrogels based on cellulose for smart swelling and control able delivery. Eur Polym J 46:92–100. doi:10.1016/j.carbpol.2011.10.017
Chatterjee A, Das B (2013) Radii of gyration of sodium carboxymethylcellulose in aqueous and mixed solvent media from viscosity measurement. Carbohydr Polym 98:1297–1303. doi:10.1016/j.carbpol.2013.08.019
Chatterjee T, Krishnamoorti R (2008) Steady shear response of carbon nanotube networks dispersed in poly(ethylene oxide). Macromolecules 41:5333–5338. doi:10.1021/ma800640w
Chen Y, Xu CH, Huang J, Wu D, Lv Q (2017) Rheological properties of nanocrystalline cellulose suspensions. Carbohydr Polym 157:303–310. doi:10.1016/j.carbpol.2016.10.002
Congming X, Yongkang G (2008) Preparation and properties of physically crosslinked sodium carboxymethylcellulose/poly(vinyl alcohol) complex hydrogels. J Appl Polym Sci 107:1568–1572. doi:10.1002/app.27203
Gorgieva S, Kokol V (2011) Synthesis and application of new temperature-responsive hydrogels based on carboxymethyl and hydroxyethyl cellulose derivatives for the functional finishing of cotton knitwear. Carbohydr Polym 85:664–673. doi:10.1016/j.carbpol.2011.03.037
Gulrez SK, Phillips GO, Al-Assaf S (2011) Hydrogels: methods of preparation, characterisation and applications. In: Carpi A (ed) Progress in molecular and environmental bioengineering—from analysis and modeling to technology applications, InTech. doi: 10.5772/24553. http://www.intechopen.com/books/progress-in-molecular-and-environmental-bioengineering-from-analysis-and-modeling-to-technology-applications/hydrogels-methods-of-preparation—characterisation-and-applications. Accessed 14 Dec 2016
Hernadez AR, Detcheverry FA, Peters BL, Chappa VC, Schweizer KS, Muller M, Pablo JJ (2013) Dynamical simulations of coarse grain polymeric systems: rouse and entangled dynamics. Macromolecules 46:6287–6299. doi:10.1021/ma400526v
Ji-Seok L, Yong-Seok K, Ki-Won S (2015) Transient rheological behavior of natural polysaccharide xanthan gum solutions in start-up shear flow fields: an experimental study using a strain-controlled rheometer. Korea-Aust Rheol J 27(3):227–239. doi:10.1007/s13367-015-0023-y
Kagarise C, Xua J, Wang Y, Mahboob M, Koelling KW, Bechtel SE (2010) Transient shear rheology of carbon nanofiber/polystyrene melt composites. J Non-Newton Fluid Mech 165:98–109. doi:10.1016/j.jnnfm.2009.10.005
Kono H (2014) Characterization and properties of carboxymethyl cellulose hydrogels crosslinked by polyethylene glycol. Carbohydr Polym 106:84–93. doi:10.1016/j.carbpol.2014.02.020
Kono H, Onishi K, Nakamura T (2013) Characterization and bisphenol A adsorption capacity of β-cyclodextrin–carboxymethylcellulose-based hydro-gels. Carbohydr Polym 98:784–792. doi:10.1016/j.carbpol.2013.06.065
Kono H, Oshima K, Hashimoto H, Shimizu Y, Tajima K (2016) NMR characterization of sodium carboxymethyl cellulose: substituent distribution and mole fraction of monomers in the polymer chains. Carbohydr Polym 146:1–9. doi:10.1016/j.carbpol.2016.03.021
Kulicke WM, Kull AH, Kull W, Thielking H (1996) Characterization of aqueous carboxymethylcellulose solutions in terms of their molecular structure and its influence on rheological behavior. Polymer 37:2723–2731. doi:10.1016/0032-3861(96)87634-8
Kulicke WM, Reinhardt U, Fuller GG, Arendt O (1999) Characterization of the flow properties of sodium carboxymethylcellulose via mechanical and optical techniques. Rheol Acta 38:26–33. doi:10.1007/s003970050153
Lapienis G, Szymanski R, Penczek S (2015) Star polymers formed by MPEG reaction with diepoxides. The course of reaction. Polymer 72:142–153. doi:10.1016/j.polymer.2015.07.004
Letwimolnun W, Vergnes B, Ausias G, Carreau PJ (2007) Stress overshoots of organoclay nanocomposites in transient shear flow. J Non-Newton Fluid Mech 141:167–179. doi:10.1016/j.jnnfm.2006.11.003
Lewis CL, Stewart K, Anthamatten M (2014) The influence of hydrogen bonding side-groups on viscoelastic behavior of linear and network polymers. Macromolecules 47:729–740. doi:10.1021/ma402368s
Li W, Sun B, Wu P (2009) Study on hydrogen bond of carboxymethylcellulose sodium film with two-dimensional correlation infrared spectroscopy. Carbohydr Polym 78:454–461. doi:10.1016/j.carbpol.2009.05.002
Lin SY, Amidon GL, Weiner ND, Goldberg AH (1993) Viscoelasticity of cellulose polymers and mucociliary transport on frog palates. Int J Pharm 95:57–65. doi:10.1016/0378-5173(93)90390-2
Lopez CG, Rogers SE, Colby RH, Graham P, Cabral JT (2015) Structure of sodium carboxymethyl cellulose aqueous solutions: a SANS and rheology study. J Polym Sci B Polym Phys 53:492–501. doi:10.1002/polb.23657
Lu Y, An L, Wang SQ, Wang ZG (2014) Origin of stress overshoot during startup shear of entangled polymer melts. ACS Macro Lett 3:569–573. doi:10.1021/mz500260h
Lu F, Wang L, Zhang C, Cheng B, Liu R, Huang Y (2015) Influence of temperature on the solution rheology of cellulose in 1-ethyl-3-methylimidazolium chloride/dimethyl sulfoxide. Cellulose 22:3077–3087. doi:10.1007/s10570-015-0740-x
Mahi H, Rodrigue D (2012) Linear and non-linear viscoelastic properties of ethylene vinyl acetate/nano-crystalline cellulose composites. Rheol Acta 51:127–142. doi:10.1007/s00397-011-0603-9
Mandala I, Savvas T, Kostaropoulos A (2004) Xanthan and locust bean gum influence on the rheology and structure of a white model-sauce. J Food Eng 64:335–342. doi:10.1016/j.jfoodeng.2003.10.018
Maswal M, Chat OA, Dar AA (2015) Rheological characterization of multi-component hydrogel based on carboxymethyl cellulose: insight into its encapsulation capacity and release kinetics towards ibuprofen. Colloid Polym Sci 293:1723–1735. doi:10.1007/s00396-015-3545-4
Moser K, Kriwet K, Kalia YN, Guy RH (2001) Stabilization of supersaturated solutions of a lipophilic drug for dermal delivery. Int J Pharm 224:169–176. doi:10.1016/S0378-5173(01)00762-1
Patenaude M, Hoare T (2012) Injectable, mixed natural synthetic polymer hydrogels with modular properties. Biomacromolecules 13:369–378. doi:10.1021/bm2013982
Ravindranath S, Wang SQ (2008) Universal scaling characteristics of stress overshoot in startup shear of entangled polymer solutions. J Rheol 52:681–695. doi:10.1122/1.2899147
Razmkhah S, Razavi SMA, Mohammadifar MA (2017) Dilute solution, flow behavior, thixotropy and viscoelastic characterization of cress seed (Lepidium sativum) gum fractions. Food Hydrocoll 63:404–413. doi:10.1016/j.foodhyd.2016.09.030
Rodriguez R, Alvarez-Lorenzo C, Concheiro A (2003) Cationic cellulose hydrogels: kinetics of the cross-linking process and characterization as pH-/ion- sensitive drug delivery systems. J Control Release 86:253–265. doi:10.1016/S0168-3659(02)00410-8
Shabbir A, Goldansaz H, Hassager O, Ruymbeke E, Alvarez NJ (2015) Effect of hydrogen bonding on linear and nonlinear rheology of entangled polymer melts. Macromolecules 48:5988–5996. doi:10.1021/acs.macromol.5b00757
Sharma R, Das B, Nandi P, Das C (2010) Viscosity of sodium carboxymethylcellulose in ethylene glycol–water mixed solvent media: separation of the influences of polyion conformation and electrostatic interactions on the reduced viscosity. J Polym Sci Part B Polym Phys 48:1196–1202. doi:10.1002/polb.22009
Solomon MJ, Almusallam AS, Seefeldt KF, Somwangthanaroj A, Varadan P (2001) Rheology of polypropylene/clay hybrid materials. Macromolecules 34:1864–1872. doi:10.1021/ma001122e
Toğrul H, Arslan N (2003) Production of carboxymethyl cellulose from sugar beet pulp cellulose and rheological behaviour of carboxymethyl cellulose. Carbohydr Polym 54:73–82. doi:10.1016/S0144-8617(03)00147-4
Wang Y, Wang SQ (2009) Exploring stress overshoot phenomenon upon startup deformation of entangled linear polymeric liquids. J Rheol 53(6):1389–1401. doi:10.1122/1.3208063
Winter HH, Morganelli P, Chambon F (1988) Stoichiometry effects on rheology of model polyurethanes at the gel point. Macromolecules 21:532–535. doi:10.1021/ma00180a048
Xiquan L, Tingzhu QU, Shaoqu QI (1990) Kinetics of the carboxymethylation of cellulose in the isopropyl alcohol system. Acta Polym 41:220–222. doi:10.1002/actp.1990.010410406
Yang XH, Zhu WL (2007) Viscosity properties of sodium carboxymethylcellulose solutions. Cellulose 14:409–417. doi:10.1007/s10570-007-9137-9
Yin Y, Ji X, Dong H, Ying Y, Zheng H (2008) Study of the swelling dynamics with overshooting effect of hydrogels based on sodium alginate-g-acrylic acid. Carbohydr Polym 71:682–689. doi:10.1016/j.carbpol.2007.07.012
Acknowledgments
The financial support of Ministry of Science and High Education (Poland) is gratefully acknowledged (Grant 03/32/DSPB/0702).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
Open Access This article is distributed under the terms of the Creative Commons Attribution 4.0 International License (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted use, distribution, and reproduction in any medium, provided you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made.
About this article
Cite this article
Komorowska, P., Różańska, S. & Różański, J. Effect of the degree of substitution on the rheology of sodium carboxymethylcellulose solutions in propylene glycol/water mixtures. Cellulose 24, 4151–4162 (2017). https://doi.org/10.1007/s10570-017-1444-1
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10570-017-1444-1