Homoclinic dynamics in a restricted four-body problem: transverse connections for the saddle-focus equilibrium solution set

Abstract

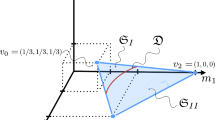
We describe a method for computing an atlas for the stable or unstable manifold attached to an equilibrium point and implement the method for the saddle-focus libration points of the planar equilateral restricted four-body problem. We employ the method at the maximally symmetric case of equal masses, where we compute atlases for both the stable and unstable manifolds. The resulting atlases are comprised of thousands of individual chart maps, with each chart represented by a two-variable Taylor polynomial. Post-processing the atlas data yields approximate intersections of the invariant manifolds, which we refine via a shooting method for an appropriate two-point boundary value problem. Finally, we apply numerical continuation to some of the BVP problems. This breaks the symmetries and leads to connecting orbits for some nonequal values of the primary masses.

Appendices

Rotational symmetry for the equal mass case

Let $m_1 = m_2 = m_3 = 1/3$ and $\theta = \frac{2 \pi }{3}$. Define the linear map, $\varphi : \mathbb {R}^4 \rightarrow \mathbb {R}^4$, by

$$\begin{aligned} \varphi (x,\dot{x},y,\dot{y}) = \left( \begin{array}{cccc} \cos (\theta ) &{}\quad 0 &{}\quad - \sin (\theta ) &{}\quad 0 \\ 0 &{}\quad \cos (\theta ) &{}\quad 0 &{}\quad - \sin (\theta ) \\ \sin (\theta ) &{}\quad 0 &{}\quad \cos (\theta ) &{}\quad 0 \\ 0 &{}\quad \sin (\theta ) &{}\quad 0 &{}\quad \cos (\theta ) \\ \end{array} \right) \left( \begin{array}{c} x \\ \dot{x} \\ y \\ \dot{y} \end{array} \right) =(\varphi _1, \varphi _2, \varphi _3, \varphi _4)^\mathrm{T}. \end{aligned}$$

Note that $\varphi$ acts as a rotation by $\theta$ in the (x, y) and $(\dot{x},\dot{y})$ coordinate planes independently. Now, suppose that $\mathbf {x}: \mathbb {R}\rightarrow \mathbb {R}^4$ is a trajectory for f, then $\tilde{\mathbf {x}} = \varphi \circ \mathbf {x}$ is also a trajectory for f. Moreover, if $\mathbf {x} \subset W^{s,u}(\mathcal {L}_i)$ for $i \in \{0,4,5,6\}$, then $\tilde{\mathbf {x}} \subset W^{s,u}(L_{\sigma (i)})$, where $\sigma$ is the permutation given by $\sigma = (0)(4,5,6)$.

Proof

Let $\hat{x} = (x, \dot{x},y,\dot{y}) \in \mathbb {R}^4$ and suppose $\mathbf {x}$ is the trajectory through $\hat{x}$ satisfying $\mathbf {x}(0) = \hat{x}$. By definition, $\tilde{\mathbf {x}}(0) = \varphi (\mathbf {x}(0)) = \varphi (\hat{x})$, and we note that $\tilde{\mathbf {x}}$ will parameterize a trajectory for f if and only if $\tilde{\mathbf {x}}(t)$ is tangent to $f(\tilde{\mathbf {x}}(t))$ for all $t \in \mathbb {R}$. Thus, it clearly suffices to prove that $f \circ \varphi = \varphi \circ f$ holds for any $\hat{x}$ on $\mathbb {R}^4$.

With this in mind, define the planar rotation $\eta : \mathbb {R}^2 \rightarrow \mathbb {R}^2$ by

$$\begin{aligned} \eta (x,y) = \left( \begin{array}{cc} \cos (\theta ) &{}\quad - \sin (\theta ) \\ \sin (\theta ) &{}\quad \cos (\theta ) \\ \end{array} \right) \left( \begin{array}{c} x \\ y \end{array} \right) = \left( \begin{array}{c} \eta _1(x,y) \\ \eta _2(x,y) \end{array} \right) , \end{aligned}$$

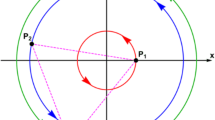
Recall that for the symmetric mass case, we have equal masses given by $m_1 = m_2 = m_3 = \frac{1}{3}$. Set $m = \frac{1}{3}$, then the primaries are located at $P_1,P_2,P_3$ given by

$$\begin{aligned} P_1 = \left( -\frac{\sqrt{3}}{3}, 0 \right) \quad P_2 = \left( \frac{\sqrt{3}}{6}, -\frac{1}{2} \right) \quad P_3 = \left( \frac{\sqrt{3}}{6}, \frac{1}{2} \right) \end{aligned}$$

and note that $\left| \left| P_1 \right| \right| = \left| \left| P_2 \right| \right| = \left| \left| P_3 \right| \right| = \frac{1}{\sqrt{3}}$. Moreover, $P_1,P_2,P_3$ are vertices of an equilateral triangle and a direct computation shows that $\eta$ acts as a cyclic permutation on the primary bodies in configuration space given by the cycle $\pi = (1,2,3)$. Recalling that $r_i(x,y) = \sqrt{(x-x_i)^2 + (y-y_i)^2} = \left| \left| (x,y) - P_i \right| \right|$, it follows from this symmetry that for $i \in \{1,2,3\}$ we have

$$\begin{aligned} r_i \circ \eta (x,y) = \left| \left| \eta (x,y) - P_i \right| \right| = \left| \left| (x,y) - P_{\pi ^{-1}(i)} \right| \right| = r_{\pi ^{-1}(i)}. \end{aligned}$$

(17)

Now, we recall that in the symmetric case, the CRFBP vector field is given by

$$\begin{aligned} f(x,\dot{x},y,\dot{y}) = \left( \begin{array}{c} \dot{x} \\ 2\dot{y} + x - \frac{1}{3}\sum _{i=1}^{3} \frac{x - x_i}{r_i} \\ \dot{y} \\ -2\dot{x} + y - \frac{1}{3}\sum _{i=1}^{3} \frac{y - y_i}{r_i} \end{array} \right) , \end{aligned}$$

which we write in scalar coordinates as $f = \left( f_1,f_2,f_3,f_4\right)$. Similarly, write $\varphi = \left( \varphi _1,\varphi _2,\varphi _3,\varphi _4 \right)$ and we note that $(\varphi _1(\hat{x}),\varphi _3(\hat{x})) = \eta (x,y)$. Now, we check that $f_i \circ \varphi = \varphi _i \circ f$ holds for each $i \in \{1,2,3,4\}$. For $i =1$, we have the direction computation

$$\begin{aligned} \varphi _1 \circ f \left( \hat{x}\right) = \dot{x} \cos (\theta ) - \dot{y} \sin (\theta ) = f_1 \circ \varphi \left( \hat{x}\right) . \end{aligned}$$

Now, for $i = 2$ we first compute each expression

$$\begin{aligned} \varphi _2 \circ f \left( \hat{x}\right)&= \left( 2 \dot{y} + x - \frac{1}{3}\sum _{i=1}^{3} \frac{x - x_i}{r_i(x,y)}\right) \cos (\theta ) - \left( -2\dot{x} + y - \frac{1}{3} \sum _{i=1}^{3} \frac{y - y_i}{r_i(x,y)}\right) \sin (\theta ) \\ f_2 \circ \varphi \left( \hat{x}\right)&= 2(\dot{x} \sin (\theta ) + \dot{y} \cos (\theta )) + x \cos (\theta ) - y \sin (\theta ) - \frac{1}{3} \sum _{i = 1}^{3} \frac{\eta _1(x,y) - x_i}{r_i \circ \eta (x,y)}. \end{aligned}$$

After canceling like terms in each expression, we are left to prove the following equality

$$\begin{aligned} \sum _{i = 1}^{3} \frac{\eta _1(x,y) - x_i}{r_i \circ \eta (x,y)} = \cos (\theta ) \sum _{i = 1}^{3} \frac{x - x_i}{r_i(x,y)} - \sin (\theta ) \sum _{i=1}^{3} \frac{y - y_i}{r_i(x,y)}. \end{aligned}$$

(18)

Applying the result from (17) to the left side, we have

$$\begin{aligned} \sum _{i = 1}^{3} \frac{\eta _1(x,y) - x_i}{r_{\pi ^{-1}(i)}} = \frac{\eta _1(x,y) - x_1}{r_3(x,y)} + \frac{\eta _1(x,y) - x_2}{r_1(x,y)} + \frac{\eta _1(x,y) - x_3}{r_2(x,y)}, \end{aligned}$$

so that for each $i \in \{1,2,3\}$, the numerator for $r_i$ is given by $\eta _1(x,y) - x_{\pi (i)}$. Now, we compute the numerators for $r_i(x,y)$ on the right-hand side as

$$\begin{aligned} \cos (\theta )(x-x_i) - \sin (\theta )(y-y_i) = \eta _1(x,y) - \eta _1(x_i,y_i) = \eta _1(x,y) - x_{\pi (i)}. \end{aligned}$$

We conclude that the numerators for each $r_i$ are equal, and therefore, the equality in (18) holds which proves that $\varphi _2 \circ f = f_2 \circ \varphi$. The proofs for the $i = 3,4$ cases are computationally similar to the corresponding proofs for $i = 1,2$ which concludes the proof that $f \circ \varphi = \varphi \circ f$, or equivalently, $\tilde{\mathbf {x}}$ is a trajectory for f.

To prove the second claim, fix $i \in \{0,4,5,6\}$ and suppose $\mathbf {x}(t) \rightarrow L_i$ as $t \rightarrow \infty$ implying that $\mathbf {x} \subset W^s(L_i)$. Let $\tilde{\mathbf {x}} = \varphi (\mathbf {x})$, and note that $L_i$ is an equilibrium solution for f implying that $\mathbf {x}_{2,4}(t) \rightarrow 0$. Noting that $\eta$ is a unitary operator, it follows that $\tilde{\mathbf {x}}_{2,4}(t) \rightarrow 0$ as well. Moreover, $\varphi$ is a dynamical conjugacy implying that in configuration space we have

$$\begin{aligned} \lim \limits _{t \rightarrow \infty } \tilde{\mathbf {x}}_{1,3}(t) = \lim \limits _{t \rightarrow \infty } \eta \left( x(t),y(t)\right) = \eta (L_i). \end{aligned}$$

Taken together it follows that $\eta (L_i)$ is again an equilibrium solution for f. Thus, $\eta$ acts as a permutation on equilibria. A direct computation shows that $\eta (L_i) = L_{\sigma (i)}$ where $\sigma$ is the permutation given by $\sigma = (0)(4,5,6)$. The preceding argument applies equally well to the unstable manifold of each equilibrium with $t \rightarrow -\infty$ which completes the proof of the second claim. $\square$

Power series manipulation, automatic differentiation, and the radial gradient

Our local invariant manifold computations are based on formal power series manipulations. The main technical challenge is to compute $f \circ P$ with P an arbitrary power series and f the vector field for the CRFBP. As usual in gravitational N body problems, the nonlinearity contains terms raised to the minus three halves power.

Consider two formal power series $P, Q :\mathbb {C}^2 \rightarrow \mathbb {C}$ given by

$$\begin{aligned} P(z_1, z_2) = \sum _{m=0}^\infty \sum _{n=0}^\infty a_{m,n} z_1^m z_2^n, \quad \quad \text{ and } \quad \quad Q(z_1, z_2) = \sum _{m=0}^\infty \sum _{n=0}^\infty b_{m,n} z_1^m z_2^n, \end{aligned}$$

where $a_{m,n}, b_{m,n} \in \mathbb {C}$ for all $(m,n) \in \mathbb {N}^2$. The collection of all formal power series forms a complex vector space, so that for any $\alpha , \beta \in \mathbb {C}$ we have that

$$\begin{aligned} (\alpha P+ \beta Q)(z_1, z_2) = \sum _{m=0}^\infty \sum _{n=0}^\infty \left( \alpha a_{m,n} + \beta b_{m,n}\right) z_1^m z_2^n. \end{aligned}$$

The collection becomes an algebra when endowed with the Cauchy product

$$\begin{aligned} (P \cdot Q)(z_1, z_2) = \sum _{m=0}^\infty \sum _{n=0}^\infty \left( \sum _{j=0}^m \sum _{k=0}^n a_{m-j,n-k} b_{j k} \right) \, z_1^m z_2^n. \end{aligned}$$

(19)

We evaluate elementary functions of formal power series using a technique called automatic differentiation by many authors. Suppose, for example, we are given a formal series

$$\begin{aligned} P(z_1, z_2) = \sum _{m=0}^\infty \sum _{n=0}^\infty p_{m,n} z_1^m z_2^n, \end{aligned}$$

with $p_{0,0} \ne 0$. We seek the formal series coefficients $q_{m,n}$ of the function

$$\begin{aligned} Q(z_1, z_2) = \sum _{m=0}^\infty \sum _{n=0}^\infty q_{m,n} z_1^m z_2^n = P(z_1, z_2)^{\alpha }, \quad \quad \quad \alpha \in \mathbb {R}. \end{aligned}$$

Our approach follows the discussion given by Haro et al. (2016). Consider the first-order partial differential operator

$$\begin{aligned} \nabla _{\mathrm{rad}} P(z_1, z_2) = \nabla P(z_1, z_2) \left( \begin{array}{c} z_1 \\ z_2 \end{array} \right) = z_1 \frac{\partial }{\partial z_1} P(z_1, z_2) + z_2 \frac{\partial }{\partial z_2} P(z_1, z_2), \end{aligned}$$

which is referred to as the radial gradient of P. Evaluating on the level of formal power series leads to

$$\begin{aligned} \nabla _{\mathrm{rad}} P(z_1, z_2) = \sum _{m=0}^\infty \sum _{n=0}^\infty (m+n) p_{m,n} z_1^m z_2^n. \end{aligned}$$

Observe that

$$\begin{aligned} \nabla _{\mathrm{rad}} Q(z_1, z_2)&= \nabla Q(z_1, z_2) \left( \begin{array}{c} z_1 \\ z_2 \end{array} \right) \\&= \nabla P(z_1, z_2)^\alpha \left( \begin{array}{c} z_1 \\ z_2 \end{array} \right) \\&= \alpha P(z_1, z_2)^{\alpha - 1} \nabla P(z_1, z_2) \left( \begin{array}{c} z_1 \\ z_2 \end{array} \right) . \end{aligned}$$

Multiplying both sides of the equation by P, we obtain

$$\begin{aligned} P(z_1, z_2) \nabla Q(z_1, z_2) \left( \begin{array}{c} z_1 \\ z_2 \end{array} \right) = \alpha Q(z_1, z_2) \nabla P(z_1, z_2) \left( \begin{array}{c} z_1 \\ z_2 \end{array} \right) . \end{aligned}$$

(20)

Here the fractional power is replaced by operations involving only differentiation and multiplication. This is the virtue of the radial gradient in automatic differentiation schemes. Plugging the power series expansions into Eq. (20) leads to

$$\begin{aligned}&\left( \sum _{m=0}^\infty \sum _{n=0}^\infty p_{m,n} z_1^m z_2^n \right) \left( \sum _{m=0}^\infty \sum _{n=0}^\infty (m+n) q_{m,n} z_1^m z_2^n \right) \\&\quad =\left( \sum _{m=0}^\infty \sum _{n=0}^\infty \alpha q_{m,n} z_1^m z_2^n \right) \left( \sum _{m=0}^\infty \sum _{n=0}^\infty (m+n) p_{m,n} z_1^m z_2^n \right) , \end{aligned}$$

and taking Cauchy products gives

$$\begin{aligned}&\sum _{m=0}^\infty \sum _{n=0}^\infty \sum _{j=0}^m \sum _{k=0}^n (j+k) p_{m-j, n-k} q_{j,k} z_1^m z_2^n\\&\quad = \sum _{m=0}^\infty \sum _{n=0}^\infty \sum _{j=0}^m \sum _{k=0}^n \alpha (j+k) q_{m-j, n-k} p_{j,k} z_1^m z_2^n. \end{aligned}$$

Match like powers to get

$$\begin{aligned} \sum _{j=0}^m \sum _{k=0}^n (j+k) p_{m-j, n-k} q_{j,k} = \sum _{j=0}^m \sum _{k=0}^n \alpha (j+k) q_{m-j, n-k} p_{j,k}, \end{aligned}$$

or

$$\begin{aligned}&(m+n) p_{0,0} q_{m,n} + \sum _{j=0}^m \sum _{k=0}^n \hat{\delta }_{j,k}^{m,n} (j+k) p_{m-j, n-k} q_{j,k}\\&\quad = \alpha (m+n) q_{0,0} p_{m,n} + \sum _{j=0}^m \sum _{k=0}^n \hat{\delta }_{j,k}^{m,n} \alpha (j+k) q_{m-j, n-k} p_{j,k}, \end{aligned}$$

for $m + n \ge 1$. Here

$$\begin{aligned} \hat{\delta }_{j,k}^{m,n} := {\left\{ \begin{array}{ll} 0 &{}\quad \text{ if } \,\,j = m \text{ and } k = n \\ 0 &{}\quad \text{ if } \,\,j = 0 \text{ and } k = 0 \\ 1 &{}\quad \text{ otherwise } \end{array}\right. }. \end{aligned}$$

The $\hat{\delta }$ appears to remind us that terms of order (m, n) are extracted from the sum. Isolating $q_{m,n}$ gives

$$\begin{aligned} q_{m,n} = \alpha p_{0,0}^{\alpha -1} p_{m,n} + \frac{1}{(m+n) p_{0,0}} \sum _{j=0}^m \sum _{k=0}^n \hat{\delta }_{j,k}^{m,n} (j+k) \left( \alpha q_{m-j, n-k} p_{j,k} - p_{m-j, n-k} q_{j,k} \right) , \end{aligned}$$

(21)

for $m + n \ge 1$. Note that $q_{0,0} = p_{0,0}^{\alpha } \ne 0$ by hypothesis, so that the coefficients $q_{m,n}$ are formally well defined to all orders. Using the recursion given in Eq. (21) we can compute the formal series coefficients for Q for the cost of a Cauchy product. This allows us to compute power series representations for the nonlinear terms in f(P) and Df(P) in the CRFBP. Another approach which converts the CRFB field to a higher-dimensional polynomial field in discussed in Kepley and Mireles James (2018).