Seismic risk assessment of residential buildings by the Heuristic vulnerability model: influence of fragility curve models and inventory scale

Abstract

Typological-observational methods still constitute one of the most commonly applied tools for evaluation of the seismic risk and vulnerability of the existing building stock. Their efficiency is mainly related to the effectiveness of the procedure for deriving fragility curves, and the reliability and completeness of the database that describes the building stock. This paper presents a comparison between the vulnerability and damage distribution assessment provided by fragility curves used in the Macroseismic and Heuristic methods, and a comparison of exposure evaluation methodologies according to two different approaches, namely a compartment- and a building-scale survey. An application to the case study of the residential building stock in the historic center of Alcamo, a town of 45,000 inhabitants in Western Sicily (Italy), shows the major reduction in fragility provided by recalibration of the masonry buildings’ ductility values that characterize the Heuristic method. Moreover, the efficiency of the compartment scale survey approach, based on the CARTIS typological-structural characterization method of ordinary buildings in urban areas, is underlined.

1 Introduction

Preservation of the historical-architectural heritage, aimed at preserving the functionality, shape and use of the buildings over time, is increasingly becoming an essential necessity for safeguarding the cultural identity of places. In this context, the numerous seismic events that occur in Italy, usually characterized by disastrous effects, underline the need to plan and implement interventions aimed at reducing the seismic vulnerability of the existing building stock (Vecchio et al. 2021; Ludovico et al. 2021). This need is particularly evident in historic centers, characterized mostly by the presence of masonry buildings, which are particularly vulnerable due to the lack of adequate connections between structural elements, able to guarantee a box behavior of the structure (Borri et al. 2020).

The planning of interventions on large portions of the territory, from the broader national scale to an increasingly smaller scale, such as the regional, territorial and municipal ones, up to that of the historic center, cannot disregard an assessment of the seismic vulnerability of the built environment (Tempesta 2018; Rosti et al. 2022; Basaglia et al. 2021) and requires availability of seismic risk maps (Zanini et al. 2019), which should be able to establish priorities and methods of intervention, on one side, and to constitute the basis for more general assessment of the increase in seismic resilience obtainable with the various prevention and reduction strategies for seismic risk, on the other side.

The continuous researches in these fields and the available multidisciplinary approaches nowadays provide numerous efficient and complex techniques, which should potentially provide reliable and robust results.

A thorough review of the methodologies available in the literature for seismic vulnerability assessment of RC and masonry structures can be found in Calvi et al. (2006), Rossetto et al. (2013), Rossetto et al. (2014), D'ayala et al. (2014), Maio and Tsionis (2015), Lagomarsino and Cattari (2013).

Methods for vulnerability assessments at the territorial scale can be divided into three main categories: empirical, mechanical/analytical, and hybrid methods (Calvi et al. 2006). Empirical methods are based on statistical analysis of the damage observed after a seismic event. These methods identify typological classes and vulnerability indices, based on several structural, geometrical and mechanical characteristics (Romano et al. 2017). A review of these methods can be found in Calvi et al. (2006), Dolce et al. (2021), Yepes et al. (2016).

Mechanical methods are based on a simplified representation of a building’s mechanical features (Romano et al. 2017). Correlations between the latter, expected damage, and seismic intensity can be evaluated through analytical or numerical methods. Both approaches evaluate the response of either archetypes of the structures that are representative of a building typology or class of building typologies, or a simplified structural scheme with geometrical and mechanical features randomly generated. Numerical approaches allow more reliable predictions of the seismic response of each structural typology to seismic events of predetermined intensity, but they need large computational efforts and, for this reason, are more suitable for analysis of a reduced number of buildings. On the other hand, the analytical approach has less accuracy in terms of seismic-response assessment. Indeed, this approach uses simplified models for the evaluation of the seismic response, with several advantages in terms of computational effort, but a loss in terms of accuracy of the analysis.

Mechanical methods for evaluating seismic vulnerability at a territorial scale adopt very simplified models of the built environment, which usually can represent only a few of the main features determining the seismic vulnerability of buildings (Donà et al. 2019; Lagomarsino and Giovinazzi 2006), such as the building height or number of floors, material mechanical characteristics of vertical structures, stiffness and strength of horizontals, and characteristics of the connections between vertical-resistant elements or between the latter and horizontals. Accordingly, this approach presents limitations related to the reduced capability of a prototype of representing a more complex building stock and the simplifications connected to the structural modelling (Masi et al. 2021).

Hybrid methods integrate the potential of empirical and mechanical approaches. These methods can use mechanical models to correlate typological characteristics and expected damage, recalibrating them based on damage data collected after major seismic events (Dolce et al. 2021; Donà et al. 2020).

Empirical methods, based on a typological classification of the building stock according to qualitative information, play a key role in vulnerability characterization at the territorial scale. These approaches prove to be especially effective for vulnerability statistical assessment at the territorial scale, aimed at allocation of reduced economic resources available for seismic risk mitigation, or for evaluation of damage scenarios for post-earthquake emergency management (Donà et al. 2019; Lagomarsino and Cattari 2013; Lagomarsino et al. 2021). Indeed, the Italian Civil Protection Department (ICPD) chose to conduct the latest National Seismic Risk Assessment (NSRA) using a multi-model methodology, where three of the six models were based on an empirical approach (Rosti et al. 2020a, b; Zuccaro et al. 2020), two adopted a mechanical approach to derive the fragility curves (Donà et al. 2020; Borzi et al. 2020b) and the last one, the Heuristic method used in the present paper, a hybrid approach (Lagomarsino et al. 2021). The Heuristic model (Lagomarsino et al. 2021) is obtained by recalibration, for masonry buildings only, of some parameters and damage distribution—based on the data provided by the post-earthquake damage database D.A.DO (Dolce et al. 2017, 2019)—of the Macroseismic method of Lagomarsino and Giovinazzi (2006). The latter is a well-known method and bases its origins on the information contained in the European Macroseismic scale EMS-98 (Grunthal 1998).

As for exposure, a European model has recently been proposed in Crowley et al. (2020). Currently, in Italy, NSRA (Italian Civil Protection Department 2018) is still evaluated based on the ISTAT (National Office of Statistics) database (ISTAT 2020), where the characteristics available for each building are only the construction material, floor number, and construction year. In order to complete and improve the ISTAT census database, many researchers are collecting data for large-scale assessments (e.g., Cacace et al. 2018; Zuccaro and Cacace 2015). The Italian Protection Department, with the support of ReLUIS ((Italian Network of Academic Laboratory of Earthquake and Structural Engineering), promotes use of the interview-based CARTIS (D’Amato et al. 2022) form for collecting information useful for seismic vulnerability assessment based on local structural characteristics. The CARTIS card contains a detailed description of the structural characteristics of residential building typologies, provided by interviews with technicians with experience in the structural construction field, who are based in the territory and have accurate knowledge of the construction techniques of the place. The numerical data (number of buildings, percentages of each typology) and the percentage of the detailed structural features of each typology are based on a reasonable estimate provided by the interviewed technician and the expert that fills out the form. To date, the CARTIS form has been employed to survey roughly 6% of all Italian municipalities. Some Italian researchers are involved in seismic vulnerability studies at the territorial scale based on information collected using the CARTIS form, which has been made available on an online platform (Polese et al. 2019, 2020; Brando et al. 2021).

This paper presents a comparison between the vulnerability and damage distribution assessment provided by the Macroseismic and Heuristic methods, through an application to the case study of the residential building stock in the historic center of Alcamo, a town of 45,000 inhabitants in Western Sicily (Italy). Moreover, with the aim of investigating the influence of the distribution of structural and typological characteristics provided by the CARTIS form methodology, an assessment of the seismic vulnerability of residential buildings is performed according to two different exposure survey scales: the interview-based CARTIS survey, which is characterized by a survey at the compartment scale, as is described in detail in the following sections, and a similar survey performed at the building scale, filling in a form containing the indications of the CARTIS form for each building.

2 Macroseismic and Heuristic methods: vulnerability index and fragility curves

In the following sections, the Macroseismic and Heuristic methods for evaluation of seismic vulnerability are presented and discussed. Moreover, the CARTIS form for assessment of exposure is presented with reference to the inventory scale, namely compartment- and building-scale surveys.

2.1 Macroseismic and Heuristic methods

2.1.1 Macroseismic method

Among the most well-known typological-observational methods in the literature, the Macroseismic method proposed in Lagomarsino and Giovinazzi (2006) plays a key role. The method, unlike most typological–observational methods, is derived from an analysis by probabilistic approaches and the fuzzy set theory of the correlations between typological characteristics and expected damage for seismic events of a given intensity, implicitly contained in the European Macroseismic scale EMS98 (Grunthal 1998). The scale identifies six vulnerability classes, from A to F, with decreasing vulnerability from class A to F, and the residential building stock is categorized through identification of 15 main structural types (see Table 1), whose seismic vulnerabilities are described by the most probable value of the typological vulnerability index V_I*. The value of the Macroseismic vulnerability index V_I for each building or sub-typology has to be evaluated by first assigning the building or sub-typology to one of the 15 structural types, and then changing the most probable value (white value) of the vulnerability index (V_I*) by assigning DV_I vulnerability/behavior modifiers defined according to detailed characteristics. Moreover, using the fuzzy set theory, from the EMS98 scale the authors obtained, for each typology, the range of V_I⁻  − V_I⁺ values within which the modified vulnerability index was likely to stay, and the range defined by the boundary values V_Imin − V_Imax beyond which the index value for an element of that typology could not be assigned.

Table 1 Structural types and characteristic values of the vulnerability index

The evaluation of building typology vulnerabilities is an essential premise for the probabilistic description of the expected damage as a function of the seismic event intensity. In the Macroseismic method (Lagomarsino and Giovinazzi 2006), the damage description is identified with the description reported in the EMS98 Macroseismic scale, which, in addition to the D0 level of no damage, includes the following 5 additional damage levels: D1 mild; D2 moderate; D3 severe, D4 very severe; D5 structural collapse.

Based on the V_I value, it is possible to assess the average damage µ_D for an earthquake of Macroseismic intensity I (vulnerability curve) by the relationship:

$$\mu_{D} = 2,5\left[ {1 + \tanh \left( {\frac{{I + 6,25V_{I} - 13,1}}{Q}} \right)} \right]$$

(1)

In the Macroseismic method the value Q = 2.3 is assumed for masonry and reinforced concrete building typologies. The distribution of the expected damage and its correlation with the Macroseismic intensity is traditionally described by the Damage Probability Matrices (DPM), which provide, for a fixed vulnerability class, the percentage of buildings suffering different levels of expected damage for each Macroseismic intensity I.

The damage distribution of DPMs is well approximated by the binomial function that provides the probability p_k to achieve the generic damage level Dk(k = 1,2,…5), as a function of the average damage value only (Lagomarsino and Giovinazzi 2006).

In (Bernardini et al. 2007) it is stressed that the use of the binomial function, besides providing a discrete damage distribution, is characterized by a fixed value of the standard deviation, which does not accurately approximate the relationship between mean damage and observed standard deviation for damage distributions derived from the implicit EMS-98 matrices. Therefore, the authors suggest describing the probabilistic distribution function of the damage derived from the discretization of the β function as follows:

$$p_{\beta } \left( x \right) = \frac{\Gamma \left( t \right)}{{\Gamma \left( r \right)\Gamma \left( {t - r} \right)}} \cdot \frac{{\left( {x - a} \right)^{r - 1} \left( {b - x} \right)^{t - r - 1} }}{{\left( {b - a} \right)^{r - 1} }}$$

(2)

with the Γ Gamma function and a ≤ x ≤ b, where it is possible to assume a = 0, b = 6, t = 8 and $r = t \cdot \left( {0,007\mu_{D}^{3} - 0,0525\mu_{D}^{2} + 0,2875\mu_{D} } \right)$.

Once the density probability function of the damage and its cumulative distribution $P_{\beta } \left( x \right) = \int_{0}^{x} {p_{\beta } (x)dx}$ for a building type are known, it is possible to derive numerical fragility curves in terms of intensity, expressing the probability of exceeding the damage level k for a given intensity of seismic action I.

Otherwise, it is possible to estimate the percentage of buildings that experience a predetermined level of damage (damage probability matrices) by using the relation allowing calculation of the probability associated with the damage level k.

In recent papers, fragility curves are also expressed as a function of the peak ground acceleration value PGA, by adopting one of the well-known correlation laws between Macroseismic intensity I and PGA values.

Based on the aforementioned relationships, it was possible, based on the seismic hazard estimated by the recent New Technical Standards for Construction (MIT 2018), to evaluate the expected Macroseismic intensity for prefixed return periods, thus enabling assessment of damage scenarios.

2.1.2 Fragility curves in the Heuristic method and the role of the dispersion model β

The Heuristic method recently proposed by Lagomarsino et al. (2021) starts form the Macroseismic method (Lagomarsino and Giovinazzi 2006) and has further developed in recent years for the study of the seismic vulnerability of existing unreinforced masonry buildings. The method, starting from the damage distribution derived by fuzzy theory interpretation of the expertise judgement that is implicit in the European Macroseismic Scale (EMS98), recalibrated the damage distribution on the observed damage in Italy, available in the database Da.D.O. (Dolce et al. 2017, 2019) developed by the Italian Department of Civil Protection (DPC), allowing for important improvements.

The Heuristic method introduces several innovations with respect to the original Macroseismic method, but still identifies the vulnerability of building structural types through the index V_I. However, as mentioned above, the ductility value suggested in the Macroseismic method is Q_MM = 2.3, for all residential buildings. By contrast, the Heuristic method recalibrates this value for masonry buildings, according to the data included in Da.D.O., which have been converted into damage degrees according to EMS98 (Grunthal 1998). The calibration takes into account the variation of the vulnerability index (V_I) with the age of construction. Using a numerical regression procedure, the following correlation was proposed between the ductility Q_HM and the vulnerability index: V_I:

$$Q_{HM} = 0.9 + 2.8V_{I} \ge 1.8$$

(3)

One innovation consists in calibration of the parameters that define the fragility curve represented, in accordance with the HAZUS model (HAZUS 1999), through cumulative Lognormal distribution, which already in previous works replaced representation of the fragility curves by means of the β (β function) distribution. Another innovation is the conversion of the measurement parameter of the seismic action intensity from Macroseismic intensity I to the peak value of the ground acceleration on rigid ground PGA, achieved by fitting the observed data. Using the equations available in the literature for construction of the fragility curves, it is possible to obtain an average PGA value for each damage level, through the corresponding average intensity of the fragility curves of the original model (Eq. 4). The equation proposed by the new model is as follows:

$$PGA_{Dk} \left( {V_{I} ,k} \right) = c_{1} c_{2}^{{\left[ {6,7 - 3,45V_{I} + \left( {0,9 + 2,8V} \right){\text{atanh}}\left( {0,36k - 1,08} \right)} \right]}}$$

(4)

where c₁ and c₂ are assumed equal to 0.05 and 1.66, respectively. These values are close to those proposed by Margottini et al. (Margottini et al. 1992). The fragility curves are described by the two-parameter cumulative lognormal distribution function, with PGA_Dk (V_I, k) given by Eq. (4) and by calibrating the dispersion value β_Dk. The dispersion value depends on the new calibration of the vulnerability curves, on the binomial distribution of the damage level and on the assumed I-PGA relationship, in particular through the parameter c₂. An equation was obtained from calibration of the model, which allows the dispersion to be correlated to parameters V and c2, or in a simplified form as follows:

$$\beta_{D} (V_{I} ) = 0.25 + 0.65V_{I}$$

(5)

Thus, the model makes it possible to derive the fragility curves of a set of buildings classified by its own taxonomy, through attribution of a vulnerability index.

With the aim of deriving the vulnerability curve of the entire masonry buildings heritage in a given area, the new Heuristic method proposes obtaining the vulnerability index V_I* as a weighted average, according to the percentage of the buildings belonging to each class and the vulnerability indices of the classes to which they belong, to be used in Eq. (4) for calculation of the average value of PGA. Then the model proposes a new relation (Eq. 6) for calculating the dispersion of whole masonry building family:

$$\beta * = \sqrt {\sum\nolimits_{i = A}^{F} {W_{i} \left[ {\beta_{D} (V_{i} )} \right]}^{2} + \beta_{1} + \beta_{2} }$$

(6)

where the last two contributions both depend on the damage level k, according to the equations reported in Lagomarsino et al. (2021).

2.2 Database for seismic exposure evaluation: the CARTIS form

The CARTIS form (Zuccaro 2004; Zuccaro et al. 2015) allows a detailed exposure survey of the common residential building types by identifying areas, called “compartments”, which are characterized by homogeneity of the building stock in terms of construction age, construction techniques, structural characteristics and distribution of typologies. The detailed description of building typologies allows exposure evaluation at the municipal scale based on an estimate of the total number of units and the percentage of each typology within each compartment. The CARTIS form has to be filled out based on one or more face-to-face interviews with one or more experts in seismic engineering and one or more local technicians who have deep knowledge of the history and evolution of the urbanization process, materials, and construction techniques. For more recent buildings, they need to have a knowledge of the criteria and rules of design and construction of the building typology.

General information about the CARTIS form is available in Polese et al. (2019); Polese et al. 2020; Brando et al. 2021), while a more detailed description can be found in Zuccaro et al. (2015), Zuccaro et al. (2023). One possible reason of uncertainty in vulnerability evaluation based on exposure surveys carried out with the CARTIS form may derive from the accuracy of what is declared by the interviewed technician and the expert that fills out the form, to predict the distribution of different typologies within the compartments, as reported in Colajanni et al. (2019).

For this reason, in this paper, the estimates obtained through surveys carried out at the compartment scale are compared with the exposure and vulnerability estimates obtained by a detailed survey campaign, performed by completing a survey form, similar to the one that defines the structural typologies in the CARTIS form, but for each building of the historic center. This second approach was conducted with some uncertainty connected to the hurried nature and limitations of the investigations, which could not take advantage of the inside survey of the typological-structural features of the buildings. However, it allowed a more reliable estimate of the number of buildings of each typology, and of the main characteristics determining seismic vulnerability, such as number of floors, type of wall texture, structural regularity in plan and elevation, stiffness and weight of floors and roofing, degree of connection between structural parts, and state of maintenance.

2.2.1 Behavior modifiers for historic centers

With the aim of defining the characteristic value of the vulnerability index of CARTIS typologies it is still necessary to use the DV_I vulnerability index modifiers (Lagomarsino and Giovinazzi 2006), also called behavior modifiers. These can be divided into two broad classes. The first class consists of modifiers of the regional vulnerability index DV_IR, adopted to take into account the different features of the building typologies in the region or geographical area where they are located. This modifier can be evaluated considering the characteristics of local construction techniques, taking advantage of the judgment of an expert. The second class includes the DVIM behavior modifiers, which take into account all those features of the building not strictly related to the types defined in Table 1. These features considerably affect the expected seismic behavior. The values of most of them have been coded, usually through statistical regression of the data on the variability of damage observed after real seismic events.

Different modifiers should be used in relation to the context to which they must be applied. Therefore, the modifiers will be presented in the following sections, detailing them for application to the historic centers of Sicily.

3 Seismic risk of the historic center of Alcamo (TP)

In the following sections, an assessment of the exposure, seismic vulnerability, and damage scenarios of the residential building stock in the historic center of Alcamo is performed. Exposure is evaluated according to the two different approaches mentioned above, namely, at the compartment and building scales, using the Heuristic method. Vulnerability and damage distribution assessments are performed according to the Macroseismic method and the Heuristic method, respectively, and comparisons are performed. Il should be noted that the entire municipality was surveyed through CARTIS procedure, even if this paper focuses only on the analysis of the historic centre.

3.1 Compartments C01 and C02: comparison of exposure

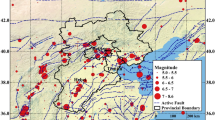
The historic center of Alcamo, a town in Sicily (Italy) (Fig. 1a), includes an area of about 509,000 m2 and coincides with areas A1 and A2 of the current town plan. In order to assess the seismic vulnerability, two compartments were identified in the area (Fig. 1b). Compartment C01, which originated in the fourteenth century, is named “Historic center: the walled city” and coincide with area A1 of the town plan. The compartment has an area of 109,280 m² and it includes about 640 buildings and a population of about 1300 residents. Compartment C02, which originated in the sixteenth century, is called “Historic center: the old quarters” and coincides with area A2 of the town plan. It has an extension of about 398,700 m² and contains about 2465 building units, with a population of about 5700 residents.

Fig. 1

Town of Alcamo (TP): a location of the town in Siclily and municipal boundaries; b historic centre of Alcamo divided into compartments

Following the classification criteria that characterize the preparation of the CARTIS sheet (Zuccaro 2004), five typologies were identified in Compartment C01: three typologies relate to masonry constructions and two others relate to reinforced concrete constructions.

The characteristics of the masonry types according to the CARTIS form classification criteria, presented in Fig. 2, and the acronyms that identify the types are discussed in the following sections.

Fig. 2

Typological classification of masonry according to the CARTIS form

The first typology, identified by the acronym MAS1, included units fabricated before the 1920s, characterized by irregular vertical masonry elements, rough stone with a haphazard texture, without recourse (A2.1 in Fig. 2), with lime mortar, predominantly 2 or 3 stories, average floor height of less than 3.50 m, average area between 60 and 110 m², mostly irregular configurations in plan, and average irregular configuration in elevation. The average wall thickness of the ground floor was about 50 cm and the average most frequent wall spacing was 3.0 m. The prevailing type of floor slab (90%) was represented by single plank wood, creating a deformable diaphragm, while the rest (10%), following restoration, was made of reinforced concrete with prefabricated joists and cast-in-place slab. The stairs, made of stone, were usually characterized by cantilevered steps or rarely by ramped vaulting. Most of the roofs (70%) were wooden, lightweight, with predominantly single pitch, while the remainder show sloping pitches. The building units showed a lack of adequate buttresses between the orthogonal walls and a presence of lintels with reduced flexural rigidity. Sometimes, there were localized reductions in the wall thickness due to the presence of flues, niches, or cavities. Many buildings of this typology present irregular number, sizing and position of the openings with respect to the outer wall box. The roof was usually not adequately connected to the walls. The foundations were superficial and continuous, made of rubble stone. Almost all of these constructions were in aggregate and a high number of buildings were connected, sharing with the adjacent units the end-bearing vertical structures. The vulnerability was frequently increased by location in aggregate, with the floors staggered with respect to the adjacent buildings. Few constructions of this type have been affected by local interventions, which, in any case, have only resulted in modest seismic improvement.

The second typology, identified as MAS2, was built between the early 1920s and 1960. The masonry of the vertical elements was made of pseudo-regular rough-hewn stone without recourses (B2.1). The typology included 2 or 3 floors. Numerous typological-structural features coincided with those of the previous type. There was a prevalent average spacing of the masonry walls that extended up to 4 m, a stronger connection between the orthogonal walls and a stronger connection of the roof to the external walls. The stairs were usually made of reinforced concrete ramped slab. The foundations were superficial and continuous, made of rubble stone or squared blocks.

Finally, the MAS3 typology was the most recent, built until the late 1970s. This typology was made of regular squared stone masonry, without recourses (C1.1). These constructions were characterized by a greater height, with the number of floors between 3 and 4. About a quarter of the buildings of this typology had cast-in-place reinforced concrete and brick floors that, representing a rigid diaphragm, distribute seismic actions on the vertical-resistant elements proportionally to their stiffness. Some of the units of this typology have the masonry walls surrounded with reinforced concrete beams and columns, thus constituting mixed confined masonry structures.

Among the reinforced concrete constructions that were built in the place of buildings that were demolished because of the widespread deterioration, or that saturated the few vacant spaces, the most common typology was RC1, including buildings built in the 1970s without earthquake-resistant design. These buildings had 3–4 stories, a regular configuration in plan and elevation, and bidirectional frames with infills of calcarenite masonry. They were characterized by ramped slab staircases and foundations made of a lattice of inverted beams. The constructions are usually built adjacent to pre-existing buildings, without adequate earthquake-resistant joints, and have first-floor pillar dimensions of no more than 45 cm and structural mesh sizes between 4 and 5 m. The percentage of longitudinal reinforcement of the columns was near to 0.6% and the transverse reinforcement generally was constituted by ϕ6 stirrups placed at 30 cm spacing. The state of preservation was in the normal range and a significant number of buildings underwent recent maintenance work.

A small percentage of buildings of the more recent RC3 typology was also available. This typology dates to a period more recent than 1996. The buildings of this type were characterized by 3–4 stories and they were made with bidirectional frames with calcarenite infills, like RC1, but with adequate earthquake-resistant design, including capacity design approach and seismic joints between buildings. The percentages of longitudinal reinforcement in the columns were close to 2% and the transverse reinforcement consisted of ϕ8 stirrups with spacing near the nodes of around 15/20 cm.

According to the CARTIS sheet (Zuccaro 2004), six typologies were identified in Compartment C02: three typologies relate to masonry constructions and three others relate to reinforced concrete constructions. The typologies characterizing Compartment C02 were similar to those in Compartment C01, with some differences related to the year of construction, number of floors, some construction details, and intended use, as a high number of buildings were partially destined to commercial activities. In addition to the abovementioned characteristics, the principal difference between the two compartments was the different distribution of typologies in the compartments. The MAS1 and MAS2 typologies in Compartment C02 were similar to their counterparts in Compartment C01. However, for both the types there was a higher number of constructions that were regular both in plan and elevation. Among the buildings in the MAS2 typology of Compartment C02, almost all of the staircases had ramped slabs, and there were far more buildings that were subjected to local strengthening. The MAS3 type of Compartment C02 differed from its counterpart in Compartment C01, mainly because of the prevalence of rigid decks made of cast-in-place reinforced concrete floors and the significant presence (40% of the typology, 11% of the total) of buildings with reinforced concrete piers and curbs inserted into the masonry, thus obtaining a mixed confined masonry structure.

Regarding reinforced concrete typologies, RC1 differed from its counterpart in Compartment C01 due to the presence of a large number of constructions dating from the 1960s, with frames arranged only along one of the two main directions of the building (about 4%). There were frequent ground-floor infills characterized by large openings and only a few buildings constructed with isolated plinth foundations. The RC2 type of Compartment C02 was also similar to the RC1 type of Compartment C01, differing in moderate earthquake-resistant design, and in some other aspects: the construction period (1980s); the presence (50% of cases) of earthquake-resistant joints between the constructions in accordance with the standards; a higher number of longitudinal column reinforcements (0.8–1%); stirrups of diameter of ϕ8, with spacing about 20 cm close to the nodes. Compartment C02 also included buildings belonging to the RC3 typology, which are more modern and taller, built from the late 1990s with 4 or 5 floors, with a resistant system RC classifiable as an infilled bidirectional frame with generally sturdy masonry, rarely light, and regular in plan and height dimensions.

Table 2 presents the distributions of the different typologies in the two compartments, obtained based on estimates of the interviewed technicians and comparison with ISTAT data from the 2001 and 2011 censuses. For both Compartments C01 and Compartment C02, two sub-types were generated from the MAS2 and MAS3 typologies in order to match the EMS98 building classification reported in Table 1: sub-type MAS2/3_RCF, including buildings with masonry walls and cast-in-place RC floors, and sub-typology MAS2/3_CM, collecting buildings with reinforced concrete floors and mixed confined masonry structure..

Table 2 Vulnerability indexes and percentages of wall types

The compartment scale survey (see Table 2) showed that in Compartment C01 there is a prevalence of masonry buildings with pseudo-regular rough-hewn stone MAS2 (26%) or squared MAS3 (22%), with a small presence of sub-types MAS2/3_RCF (5%) and MAS2/3_CM (3%). All of the abovementioned typologies were characterized by good quality masonry. However, the presence of irregular stone masonry irregularly textured MAS1 (18%), which is not negligible, should also be pointed out. There was also a considerable presence of reinforced concrete constructions, with the oldest in the RC1 typology (21% of the total) and the newest in RC3 (5% of the total). In Compartment C02, there was still a major presence of MAS2 masonry (20%). On the other hand, the overall predominant situation was the percentage of types characterized by reinforced concrete floors (17%) or confined masonry (11%), along with 7% of MAS3. Approximately a third of the buildings in the compartment were made of reinforced concrete (35%), with a prevalence of the most vulnerable RC1 buildings (20%), constructed without an adequate earthquake-resistant design.

As for the height, for masonry constructions in Compartment C01, 58% were 2- or 3-story (44% in C02), while only 15% (14%) were 4-story masonry, a characteristic that increases their seismic vulnerability. The reinforced concrete buildings in the two compartments were predominantly 3–4 stories, while only 2.5% in Compartment C02 had more than 4 stories. Even though the data on the construction period provided by the ISTAT census and by experts’ estimations presented a reduced reliability level, overall it can be assumed that, in the historic center, there is a considerable presence of buildings built after the 1970s, a period influenced by the memory of the Belice earthquake of 1968. This circumstance encouraged the use of earthquake-resistant features both in the consolidation works carried out on existing buildings (curbs and rigid slabs in RC), and in the construction of new buildings with confined masonry, allowing a reduction of their seismic vulnerability. It also favored construction of RC buildings with anti-seismic designs (frames in two orthogonal directions and lattice foundations with inverted beams).

3.2 The vulnerability of the buildings in the historic center of Alcamo

In order to assess the seismic vulnerability using the Macroseismic (Lagomarsino and Giovinazzi 2006) or Heuristic method (Lagomarsino et al. 2021) and for the exposure estimates through the CARTIS forms, the first operation to be done is the assignment to each of the CARTIS typologies of a corresponding typology derived from the EMS-98 Macroseismic scale (Grunthal 1998). It has to be stressed that the role of rigid reinforced concrete floors is interpreted in two different manners by the EMS98 type characterization and by the CARTIS form. More precisely, while in the EMS98 type characterization there is a type characterized by unreinforced masonry with R.C. floors (M7), in the CARTIS form the presence of R.C. floors is considered as an attribute of a given masonry type. Thus, a proper definition of the modifiers related to floor typology should be achieved in order to make the two different approaches consistent.

For types MAS1, MAS2 and MAS3 of both compartments it was simple to identify the corresponding types among those available in the Macroseismic scale EMS 98. In particular, these are types M1 (rough stone masonry), for MAS1, and M3 (squared stone masonry), for MAS2 and MAS3. However, as mentioned before, for typologies MAS2 and MAS3 it was necessary to derive the two sub-typologies MAS2/3_RCF and MAS2/3_CM, which respectively identify buildings with rigid reinforced concrete floors and buildings with confined masonry. The first of these two sub-typologies can be associated to the M6 typology of the EMS 98 classification, the second to the M7 typology.

The abovementioned correspondences and the corresponding most-probable values (white values) of the vulnerability index V_I* of the macro-types are reported in Table 2.

As mentioned in Sect. 2, in order to define the characteristic value of the vulnerability index of CARTIS sub-typologies, it was still necessary to introduce the vulnerability index modifiers DV_I (Lagomarsino and Giovinazzi 2006). Table 3 presents the values of the modifiers, differentiated for masonry and reinforced concrete structures, according to the level of seismic design. With reference to masonry structures, regarding the floor, assuming that masonry buildings belonging to class M1 and M3 usually have deformable wooden floors, the presence of a semi-rigid floor (double wooden floor or iron and hollow tiles) decreases the vulnerability (DV = -0.02); on the other hand, the presence of a reinforced concrete floor, due to the weight increase, increases the vulnerability of a building type with poor quality irregular masonry and lacking edge beams. Thus, a DV_Im =  + 0.06 for a brick-concrete floor on rounded stone irregular masonry, and a modifier equal to DV_Im =  + 0.03 in the case of rough stone irregular masonry were set.

Table 3 DVIM vulnerability index modifiers

According to the EMS98 type characterization, the presence of a rigid reinforced concrete floor produces a vulnerability variation for regular masonry M2 and M3, which leads to a shift from the classes belonging to type M6, characterized by V_I* (M6) = 0.616,

A regional modifier was used for typology M7, confined masonry, in order to account for the fact that confined masonry in Sicily is usually made with calcarenite blocks, instead of the commoner and more resistant solid bricks. This modifier had a value of DV_IR,M7 =  + 0.06.

For aggregate buildings, widespread in historic centers, a decisive role is played by the location within the aggregate (intercluded, corner or head) and by vertical interaction with adjacent buildings, with particular regard to the possible presence of buildings with staggered floors (Giovinazzi et al. 2004). According to Colajanni and Pennisi (2020) and Colajanni et al. (2023), two further modifiers are defined with reference to the presence of openings on a building facade and to the type of floor. In particular, with reference to the presence of openings, indicating with n the building floor number and with ρfi the opening percentage on floor i (1 ≤ i ≤ n), the modifier is defined as DV_m = max1 ≤ i ≤ n [(ρfi -0.4/(10 i)]. This modifier takes into account the different vulnerability induced by the openings in relation to the floor where they are located.

The choice of the value of the modifier taking into account the thickness of the walls and the distance between them is made according to the building type and number of floors, considering Table 4. These values were chosen based on the following considerations: the wall thickness, for a fixed masonry type, is generally higher for a greater number of floors, while it is a decreasing value if, for a fixed number of floors, the masonry mechanical characteristics are better, as happens in the transition from M1 to M7. A similar consideration can be made for the spacing between the walls, which can reach higher values in the presence of better-quality masonry, typical of more recent constructions. For attribution of the modifier value, the thickness of the masonry type is compared with the reference value reported in Table 4. If the two values present a difference of less than 20%, the modifier value is halved and assumed equal to ± 0.02, while the maximum modifier value is used for differences equal to or greater than 20%.

Table 4 Reference values of the ground floor walls thickness and the center distance of the walls, according to the masonry type and the number of floors

The DV_I values of the behavior modifiers were assigned to the different types by means of weights w_i that indicate the percentages of buildings of the types that present that particular feature. The sum value of the vulnerability index modifiers, Σ wi DV_IM, and final values V_I of the characteristic vulnerability index for the CARTIS sub-typologies thus obtained (VI = VI* + Σ wi DVI_M,i) are reported in the last two columns of Table 2. It can be noted that, for masonry typologies, the introduction of the behavior modifiers produced an increment in the vulnerability index. In particular, in Compartment C01, the increase was about 10% of the characteristic value of the EMS98 typology for MAS1 and MAS3 typologies, and 17% for the MAS2 typology, due to a higher percentage of roof spread and a smaller presence of local strengthening interventions. The variation of the vulnerability index of confined masonry was considerable (38%), due to the presence of the regional modifier DVI_R,M7. The increments in the vulnerability index in Compartment C02 were smaller, thanks to the reduced irregularities in the plan and elevation and the better connection of roofs to the masonry walls, especially for the MAS2 and MAS 3 types. The variations in the vulnerability index for reinforced concrete typologies, in both compartments, were very small and all negative, except for RC1 in Compartment C02, because of the absence of seismic joints responding to the standards and the presence of a large percentage of constructions that were irregular in plan and/or elevation or had an irregular infill distribution.

Aiming at summarizing the difference in seismic vulnerability between the two compartments, an average vulnerability index of the compartment was evaluated, through a weighted average, according to the percentages of the presence of the vulnerability indexes of each typology. Therefore, the values of VI = 0.766 for Compartment C01 and VI = 0.682 for Compartment C02 were obtained. The difference was mainly related to the higher percentage of reinforced concrete buildings available in Compartment C02 and their lower vulnerability.

3.3 Exposure and vulnerability by building-scale survey

The outcomes presented in the previous sections showed that the exposure estimates conducted with the described procedure were highly influenced by the estimated percentage of a given typology within the compartment. In addition, the variation in the vulnerability index due to detailing characteristics (influence of modifiers) was considerable for masonry typologies, while this variation was much lower for reinforced concrete structures.

The survey of individual structural units could apparently lead to a more accurate estimate of exposure and vulnerability. Actually, this approach guarantees a totally reliable estimate only if the survey is carried out with great thoroughness, through an inspection of each individual unit from the inside too, and an interview with the owner in order to acquire information about the construction that could not be obtained by a cursory survey. This procedure is so demanding in terms of time and cost that it is possible to adopt it only for small territories. More frequently and over larger areas, it is possible to conduct a cursory building-scale survey, limited to a photographic survey and an inspection from the outside of the construction. In this situation, the structural characteristics are usually not clear, and the survey and attribution of these characteristics is highly influenced by the surveyor’s knowledge and skill in accurately identifying elements representative of these characteristics.

In this context, the outcomes of an investigation performed by means of cursory surveys conducted out of the buildings are here presented. The analysis was carried out through a complete photographic survey of the building units, the support of images from the web and a following in-depth investigation by additional on-site surveys. The photos and results were transferred to a geo-referenced database, which was adopted as support for evaluation of the vulnerability index of the individual structural unit. These are the parameters of structural interest that were surveyed: number of floors, number of raised floors, regularities in plan and elevation, state of preservation, type of masonry or type of characteristic framed structure, material of the elevation, type of roofing, type of floors, presence of openings near the eaves, percentage of openings on the ground floor, position in aggregate and interaction with adjacent buildings, presence of earthquake-resistant devices, and presence of seismic strengthening or improvement interventions.

It has to be remarked that when performing a building scale survey, a large source of uncertainty is related to attribution of masonry typology to units with totally plastered façades. Indeed, the architectural features allowed one to identify, with a certain degree of reliability, among the plastered constructions, those that were reinforced concrete types, while identification of masonry types was not as simple, save in the presence of strongly characterizing typological indicators (Colajanni et al. 2019; Colajanni and Pennisi 2020). The high percentage of buildings with plastered façades (31.6%) influenced the accuracy of the cursory building-scale survey for the seismic vulnerability evaluation.

In order to overcome this problem, based on previous studies, it was evidenced that the age of construction and the number of floors of the building were two of the parameters most correlated with masonry typology. Thus, considering that in a fixed compartment the age of construction is supposed to be similar for most of the building units, it follows that only the number of floors could be attributed to individual building with a high level of reliability. Therefore, this characteristic was chosen to assign to plastered buildings a value of the most probable vulnerability index V_I* as a function of the number of floors. The value for each number of floors was obtained as a weighted average according to the percentage of buildings of different typologies and the corresponding most probable vulnerability indices EMS98 of the compartment.

Table 5a reports the percentages of different types of masonry buildings divided by floors and the value of the most probable vulnerability index attributed to plastered buildings. It can be noted that, as the number of floors increases, the percentage of buildings in the least vulnerable classes increases, and thus the “initial” value of the most probable vulnerability index decreases as the number of floors increases. This characteristic should not be confused with the influence of height on a building’s seismic vulnerability, which has an opposite correlation, but represents the correlation between the material of the vertical load-bearing elements and the number of floors.

Table 5 (a) Percentages of masonry building types divided by floors and most probable vulnerability index for plastered buildings; (b) estimated percentage presence types for the entire historic center

Table 5b compares the rates of each building type predicted by the building and compartment-scale (CARTIS) surveys. The outcomes point out the difficulty of the building-scale survey in identifying both buildings with confined masonry, which are largely underestimated, and RC buildings designed according to the most recent seismic code. In any case, the overall estimation rates for masonry buildings provided by the two survey approaches were similar, i.e., 0.73 and 0.67, even though the differences in the distribution across the three different masonry building types were quite significant (particularly for the MAS3 type). This circumstance affected the vulnerability estimations since these features significantly reduced the vulnerability of both masonry and reinforced concrete constructions.

For the whole historic centre, Table 6 presents the influence of the behavior modifiers on the evaluation of the vulnerability indexes for the different typologies estimated with the building-scale analysis (for non-plastered buildings).

Table 6 Vulnerability index values estimated by the building-scale survey

The table reports the statistical parameters (mean, standard deviation, coefficient of variation, lower and upper extremes and width of the variation range, percent change in the mean value, and percent of the width of the variation range respect to the mean value) of the vulnerability index for each of the typologies. The percentage values of the variation range, ranging from 35.8% for MAS2/3_RFC up to 60.2% for MAS1, underline that the influence of modifiers at the building-scale is very significant on the individual building. Nevertheless, by comparing the values of the most probable vulnerability index V_I with the average value for the typological class, it can be noted that it becomes modest on the average value of V_I. Excluding the influence of the regional modifier, the greatest variation in the average value is found for the MAS2/3_CM type and is equal to 5.1%, while it is only 0.4% for the MAS1 type. A comparison with the effect of modifiers estimated with compartment-scale survey, highlighted by the results presented in Table 2, points out that in this case the influence on the V_I value is significant, being equal to 22% for MAS2/3_CM (excluding the rate due to the regional modifier), standing at values close to 10% for most masonry typologies and with smaller variation for reinforced concrete typologies. These circumstances have significant repercussions on the expected mean damage (vulnerability curve) and damage distribution (fragility curve) estimation as the intensity of the seismic event changes for each building type, but the influence is greatly reduced when an overall assessment of the expected damage is performed.

4 Vulnerability assessment and damage scenarios by macroseismic vs Heuristic method

One of the goals of the present work is to compare the description of the vulnerability and the damage scenarios obtained with the Macroseismic and the Heuristic methods, previously described. The substantial difference between the two models lies in the different formulation of the average damage, due to a different evaluation of the structural ductility, and in the different functions used for the representation of the fragility curves. The series of comparisons that will be summarized in the following sections have the purpose of highlighting the main differences obtained by varying the average damage and using the same formulation for the fragility curves; and, vice versa, showing the changes obtained adopting a different mathematical representation of the fragility curves while keeping the starting data relating to the average damage unchanged.

It should be noted that the input data for both models are the same, with reference to the subdivision of the compartment into types and the attribution of vulnerability indices, as set out in Sect. 3.

4.1 Evaluation of average damage

First of all, Table 7 shows the variation of the ductility Q predicted by the two models, for the considered masonry typologies of Compartment C01.

Table 7 Comparison between the estimated ductility values for the typologies of the historic center of Alcamo according to the Macroseismic method Q_MM and the Heuristic method Q_HM

For the Macroseismic method, the ductility value Q_MM was assumed to be constant, while for the Heuristic method, Q_HM was expressed according to Eq. (3), as a function of the vulnerability index. It should be noted that the ductility values increase as the vulnerability value increases. A more careful analysis leads to the following consideration: higher vulnerability values are attributable to older structures having greater ductility, which can be interpreted as greater displacement capacity due to brick displacement on the mortar joints, or reduced degree of constraint in the masonry, accompanied by a simultaneous reduced value of displacement at the elastic limit, due to reduced resistance. The most modern structures show greater strength, but less ductility due to the use of hollow bricks, which exhibit fragile failure.

The first comparison concerns the mean damage curves, evaluated according to the formulations provided by the two models. Based on the data in Table 7, it is easy to predict that, for the same Macroseismic intensity, the average expected damage is lower if calculated with the Heuristic model.

The results are shown in Fig. 3. The graph shows the vulnerability curves obtained with the Macroseismic model in a continuous line, while the dashed line is used for the curves obtained with the Heuristic model; the same colour is used for similar construction typologies. A difference between the two series of curves can be clearly detected. Although the initial values, corresponding to a Macroseismic intensity equal to 5, are almost coincident, as the Macroseismic intensity I increases, there is a strong decrease in the average damage predicted with the Heuristic method. Moreover, for all the different typologies, a µ_d value predicted for the destructive earthquake of intensity I = 12 can be noted, even more marked than in the Macroseismic model.

Fig. 3

Comparison between the vulnerability curves provided by the Macroseismic model (MM) and the Heuristic model (HM)

4.2 Fragility curves

4.2.1 Heuristic model: influence of the mean damage variation on the fragility curves

The Heuristic model allows one to evaluate the fragility curves as a function of the peak ground acceleration PGA. Furthermore, besides the variation of the ductility factor Q, as already highlighted, the cumulative lognormal function is used instead of the function β for analytic representation of the fragility curves.

In this section it is shown how the variation of the mean damage predicted by the two models affects the fragility function, when this is provided by the lognormal cumulative probability function, as described in Sect. 2.

In this case, the difference in the calculation of the average damage is implicitly taken into account in the equation for evaluation of the average value of the PGA that characterizes the fragility curve. In the Heuristic model, the PGA is evaluated adopting Eq. (4), derived by using the average damage in Eq. (1), in which Q is calculated according to Eq. 3. On the other hand, using the expression of average damage provided by the Macroseismic model, namely Eq. (1) with Q = 2.3, the average PGA value is calculated using the following equation (Eq. 7):

$$PGA_{DK} \left( {V,k} \right) = c_{1} c_{2}^{{\left[ {8,1 - 6,25V + 2,3a\tanh \left( {0,36k - 1,08} \right)} \right]}}$$

(7)

In both cases, the dispersion β* was evaluated with Eq. (6).

Two graphs are shown below: the first shows the fragility curves for the MAS1 typology (Fig. 4a), while the second shows the curves for the MAS2/3_CM type (Fig. 4b). In both cases, the differences are negligible for damage level 1, and they grow, the greater the vulnerability of the typology, the more the damage level increases.

Fig. 4

Influence of the variation in the estimate of the ductility and mean damage (Macroseismic model—QMM vs Heuristic model—QHM) on the fragility curves represented by the cumulative lognormal distribution (Heuristic model) for a the MAS1 masonry typology and b the MAS2/3_CM masonry typology

4.2.2 Heuristic model: influence of the dispersion β

In order to investigate the influence of calculation of the β dispersion, the fragility curves evaluated by using the lognormal distribution, but calculating the dispersion in the different ways proposed by the Heuristic model, were compared with each other. For the sake of brevity, only the results related to the MAS1 typology are discussed in the following.

In particular, the dispersion was calculated using the following approaches:

- in the first case, β was calculated according to Eq. (5), considering the vulnerability index attributed to the typology, thus obtaining a single value for each typology, constant as the level of damage varies. The curves thus obtained are identified with the label Dk_ βD (V)_HM in the graphs below;

- in the second case, β was calculated by means of Eq. (6), assuming that each typology is represented by a weighed combination of two of the 6 vulnerability classes, from A to F, contained in EMS98 (), with vulnerability index values that define the narrowest range in which the vulnerability index of the structural type to be represented is included. The weight is defined in order to match the vulnerability index V_i value. The curves thus obtained are identified with the label Dk_ β *_HM in the graphs below:

- in the third case, β was evaluated as in the previous case (Eq. 6), but neglecting the contribution of β2, as was done in the numerical applications reported in Lagomarsino et al. (2021). The curves thus obtained are indicated as Dk_ β * (− β2) _HM in the graphs.

Table 8 shows the dispersion values obtained for the three cases considered, with reference to the MAS1 typology. It should be noted that the average value β_D (V) = 0.881, calculated as a function of the vulnerability index of the class, is smaller than the β* values calculated both when β₂ is considered and when β₂ is neglected, for damage levels up to D4. Moreover, the average value β_D (V) is close to the value that β* assumes for damage level 5.

Table 8 Calculation of dispersion for the MAS1 typology

For all the considered typologies, it was found that there are no significant variations in the fragility curves evaluated using the different models for calculating β.

4.2.3 Influence of the variation of the fragility curves model, using variable mean damage

In order to highlight the differences that the two methods involve in evaluation of the fragility curves, the curves obtained using the probability distribution β and the cumulative lognormal distribution were compared, using the variable mean damage proposed by the Heuristic method (Eq. 3 for masonry buildings, Q = 2.3 for RC frames).

Figure 5a and 9b show the fragility curves for MAS1 and RC1 types respectively. The curves obtained using the lognormal distribution are represented with a solid line, while those obtained using the cumulative distribution β are shown with a dashed line.

Fig. 5

Fragility curves represented by the cumulative lognormal distribution and function β for mean damage according to Heuristic model (HM) for a MAS1 and b RC1

In the curves referring to MAS1 (Fig. 5a), QHM = 3.6 is assumed. The results show that the expected damage distribution is not greatly influenced by updating the function used to represent the fragility curves, with some not negligible differences in the assessment of D5 distribution for high intensity seismic excitation. By contrast, when fragility curves referring to the RC1 type are considered (Fig. 5b), for which the value of the ductility was not updated by the Heuristic method, the use of lognormal distribution provides a noticeable reduction of the expected fragility.

4.2.4 Comparison between fragility curves predicted by the Macroseismic model and the Heuristic model

In the previous sections, it has been highlighted that, in addition to the variation between the average damage predicted by the two models, the different analytical expression adopted entails a reduction in dispersion in the new formulation. In particular, the dispersion shows major variation for the higher damage levels of the less vulnerable structures. In the following sections, the forecasts of the fragility curves provided by the two models are compared, thus highlighting the differences obtained by varying both the analytical formulation and the average damage calculation. The curves thus obtained, shown in continuous lines for the Heuristic model and in broken lines for the Macroseismic model, show that for highly vulnerable typologies, such as MAS1 (Fig. 6a), the reduction in fragility is already significant starting from damage level D2 and gradually amplifies, with a strong increase in the dispersion predicted by the most recent model.

Fig. 6

Fragility curves for predicted by the Macroseismic model (MM) and the Heuristic model (HM), for a MAS1 and b MAS2/3_CM

For less vulnerable typologies (MAS2/3_CM) (Fig. 6b), the differences between the predicted fragilities are significantly smaller and the increase in dispersion becomes larger for higher levels of damage.

4.3 Seismic damage scenarios for the historic center of Alcamo

The damage scenarios for the buildings in the historic center of Alcamo are here evaluated, with reference to the seismic hazard predicted by the current NTC2018. The analysis was performed with reference to the intensity expected for a return time of 475 years, corresponding to the verifications at Safeguard-Life Limit State SLV. It was assumed that the entire historic center is built on category B soil, which is the one most frequently found in the analyzed territory, with a stratigraphic amplification coefficient equal to 1,2. The value PGA = 0.144g was found. In this context, the forecasts provided by the two models, Macroseismic and Heuristic, will be compared.

The results obtained according to the Heuristic method with exposure survey conducted at the compartment scale are shown in Table 9, while Table 10 shows, for each typology, the differences in the number of buildings for which a predetermined level of damage is foreseen by the Heuristic and Macroseismic methods, i.e. the results of the Macroseismic method can be obtained by subtraction of Tables 11 and 12. Lastly, in Table 13 the results just given are reported in percentage form with respect to the number of buildings of each type.

Table 9 Damage scenarios: number of buildings by typology per damage level achieved

Table 10 Differences in the number of buildings that suffer a level of damage predicted by the two methods

Table 11 Differences in the percentage by type of buildings that suffered a damage level predicted by the two methods

Table12 Damage scenarios obtained by building scale survey (BBS): number of buildings by typology per damage level achieved

Table 13 Differences in the percentage on type of buildings that suffer a level of damage predicted by the two survey scales

The tables show numerically the trends highlighted by the fragility curves, with a strong reduction of the damage foreseen by the Heuristic method with respect to the Macroseismic method. Overall, there is a significant reduction in the percentage of buildings that suffer higher damage levels (D3, D4 and D5), and a correspondent large increment of the percentage of building that suffer no (D0) or low damage (D1). This trend is more evident for the most vulnerable typologies (MAS1 MAS2, and MAS3), which move into the field of low or intermediate damage (D1 and D2) for MAS1, and no or low damage (D0 and D1) for the MAS2 and MAS3 typologies. Also, the less vulnerable masonry typologies, MAS2/3_RCF and MAS2/3_CM, move towards low or no damage levels (D1 and D0). It should be noted that for the most vulnerable typologies this variation depends mainly on the variation of the ductility (and therefore of the average damage).

5 Vulnerability assessment and damage scenarios by compartment- vs building-scale survey

In this section the outcomes obtained by assessing the seismic vulnerability of the residential built stock of the historic center of Alcamo based on the building-scale exposure survey are presented and compared to the results obtained at compartment-scale. The analyses are performed by using the formulations provided by the Heuristic method, so that the only difference is represented by the exposure estimate scale.

Figure 7 presents the residential building units of the historic centre of Alcamo divided considering the number of elevations, along with the photo of two representative units. The total number of surveyed buildings can be detected in Table 11.

Fig. 7

Building units of the historic centre of Alcamo divided considering the number of elevations

5.1 Vulnerability and fragility curves

A first comparison is made in terms of mean damage (vulnerability) curves, evaluated according to the formulation provided by the Heuristic model. The results are presented in Fig. 8, which shows the vulnerability curves obtained by the building-scale exposure estimate in the continuous line, while the dashed line is used for the curves obtained by compartment-scale estimates; the same color is used for analogous building typologies.

Fig. 8

Comparison between the vulnerability curves provided by a compartment-scale survey and a building-scale survey (BBS)

Some differences between the two series of curves can be observed. The initial values, corresponding to the Macroseismic intensity of 5, are almost coincident for each construction typology, considering the two different analysis scales, apart from the most vulnerable masonry types, namely MAS1 and MAS2, for which the mean damage obtained from the building-scale analysis is slightly lower than that provided by the compartment scale survey. With the increase of the Macroseismic intensity, the curves related to the two series develop almost in parallel for each construction type, with a more marked scatter for the masonry types MAS1, MAS2 and MAS 2/3_CM, and for the reinforced concrete type RC1. The µd value predicted for the destructive earthquake of intensity I = 12 is almost the same for the two series, for each building typology. The curve of plastered building is similar to that of MAS2 for the building-scale survey (MAS2_BBS). Overall, the vulnerability reduction of buildings provided by the building-scale survey produces a decrease of the mean damage, which is more marked for low Macroseismic intensity.

Another comparison is made in terms of fragility curves, for the MAS1 and MAS 2/3_RCF types, for the sake of brevity. In Fig. 9, the curves related to building-scale survey are reported as continuous lines, while the curves representative of the compartment-scale survey are presented as dashed lines. In this case too, the same color is used for analogous building typologies. The results underline that for highly vulnerable typologies, such as MAS1 (Fig. 9a), the scatter between the two series is already evident starting from damage level D1, but there is no increase in dispersion. For less vulnerable typologies, the difference between the predicted fragilities is negligible for all damage levels (Fig. 9b).

Fig. 9

Fragility curves predicted by a compartment-scale survey and a building-scale survey (BBS), for a MAS1 and b MAS2/3_CM

5.2 Seismic damage scenarios

In order to highlight the effect of the two different exposure survey scales, Table 11 shows the outcomes obtained by adopting the Heuristic method with an exposure survey conducted at the building scale. It has to be remarked that a direct comparison of the number of buildings that suffered a given damage level, obtained by using the two survey scales, is hindered by the presence of the plastered façade building type in the BSS. These buildings belong to different masonry types, in unknown percentages. In Table 12, for each typology, the difference in the percentage of buildings for which a predetermined level of damage is obtained by adopting the compartment- and the building-scale survey is shown; the percentage is evaluated with respect to the number of buildings of each typology. The results show a reduction in the percentage of buildings that suffer no (DO) or low (D1) damage predicted by the BSS (negative values) with respect to the percentage that suffer medium damage (D2 and D3), while the percentage that suffer high damage (D4 and D5) remain almost constant. This trend is the numerical representation of the different fragility curves obtained by the two scale surveys.

It should be pointed out that the comparison does not include the plastered buildings, which are a “typology” that only exists in the BSS. Thus, in order to have a comparison of the overall damage distribution assessment, the total results shown in the last two rows of Tables 10 and 11 have to be compared. The results are shown as histograms in Fig. 10.

Fig. 10

Damage distributions of compartment survey scale (CSS) and building survey scale (BSS)

The outcomes stress that negligible differences of overall damage distribution are predicted by the two scale surveys, with an increment of 3.18% of the buildings that suffer no damage (D0) assessed by CSS, a reduction of low damaged (D1) building of 3.93%, and other variations that do not exceed 0.57%.

6 Conclusions

This paper presents a comparison between the vulnerability and damage assessment provided by the Macroseismic and Heuristic methods, and investigates the influence of exposure evaluation methodology in the seismic vulnerability assessment by using two different approaches, namely compartment- and building-scale survey. The analyses were performed with reference to the case study of the residential building stock in the historic center of Alcamo, a town in Western Sicily (Italy). Based on the outcomes presented in the previous sections, the following conclusions can be drawn:

• Regarding the comparison between Macroseismic and Heuristic method, the results showed a strong decrease in the average damage predicted with the Heuristic method.

It was shown that the mean damage variation in the cumulative lognormal function had a certain influence on the trend of the fragility curves: in particular, the differences were negligible for damage level D1, and with greater vulnerability of the typology and an increase in the damage level, the larger they grew.

Investigation of the influence of the β dispersion calculation in evaluation of the fragility curves through the Heuristic model highlighted that there were no substantial variations in the fragility curves.

The results of the vulnerability analyses performed adopting the probability distribution β and the cumulative lognormal distribution, using the variable damage proposed by the Heuristic method, pointed out that, for masonry type MAS1, the expected damage distribution is not greatly influenced by updating the function used to represent the fragility curves, with some not negligible difference in the assessment of D5 distribution for high intensity seismic excitation. On the other hand, when fragility curves referring to RC1 type are considered, for which the value of the ductility was not updated by the Heuristic method, the use of lognormal distribution provides a noticeable reduction of the expected fragility.

The comparison between the fragility curves predicted by the Macroseismic and the Heuristic methods (varying both the analytical formulation and the average damage calculation) pointed out that for highly vulnerable typologies, such as MAS1, the reduction in fragility is already significant starting from damage level D2 and gradually amplifies, with a strong increase in the dispersion predicted by the Heuristic model. For less vulnerable typologies, the differences between the predicted fragilities are significantly smaller and the increase in dispersion becomes relevant only for the higher levels of damage D4 and D5.

• The damage scenarios confirmed the trends evidenced by the fragility curves, with a strong reduction of the damage evaluated by the Heuristic method with respect to the Macroseismic method. In particular, a substantial reduction of the percentage of constructions that suffer higher damage levels was found, and a corresponding large increase of the percentage of constructions that suffer no or low damage. This trend was more evident for the most vulnerable types, for which this variation depended mainly on the variation of the ductility.

• As for the exposure evaluation methodology, the results showed that both the two procedures, namely compartment- and building-scale survey, present weaknesses. Indeed, the outcomes obtained by the compartment-scale survey are strongly influenced by the estimate of the percentage of distribution of each type within the compartments, and the descriptions of the type features that characterize the expected seismic response have major influences on the attributed vulnerability indexes. For the case study, the compartment-scale survey leads one to predict a considerable increase in vulnerability, especially for the oldest and most vulnerable masonry types. On the other hand, the vulnerability index variation produced by the behavior modifiers for building-scale exposure assessment campaigns, while significantly influencing the vulnerability of the single building, tended to compensate for all the constructions in the compartment, resulting in average vulnerability values close to those corresponding to the most probable value of the typology. In other words, modification factors have little influence. It should be noted that this conclusion is influenced by the values attributed to the behavior modifiers, which is an aspect that could have relevance on the results and requires further investigation.

• Comparisons in terms of vulnerability and fragility curves and in terms of damage scenarios were also made between the results obtained from the estimates at compartment and building-scale. The damage curves underlined reduced differences between the two series of outcomes, with only limited scatter for some building typologies (MAS1, MAS2, MAS 2/3_CM, RC1)

The results in term of fragility curves showed that for highly vulnerable types, such as MAS1, the scatter between the two series was already evident starting from the damage level D1, but there is no increase in the dispersion. For less vulnerable typologies, the difference between the predicted fragilities was negligible for all the damage levels.

• The overall comparison of the damage distribution obtained by the two surveys at different scales point out negligible differences, confirming the effectiveness of the CARTIS approach.

• The outcomes presented in the current paper represent the base for possible future developments. Due to the huge effort in terms of time and work, it would be very difficult carrying out a building-scale analysis for all the Italian municipalities. However, the building-scale analysis represents a useful tool in order to confirm the reliability of the compartment-scale survey. For this reason, it would be important to perform additional similar analyses for other municipalities.

The influence of different values of modifiers on the final value of vulnerability index is another aspect that need further investigations. The modifier related to the age of construction requires particular attention, since it plays a key role in the Heuristic method.

It would be useful and interesting extending the typological characterization to a vast area such as Sicily, through the identification of a certain number of building types that can be representative of the whole built stock of the region.