Direct Numerical Simulation of Turbulent Flow in a Circular Pipe Subjected to Radial System Rotation

Abstract

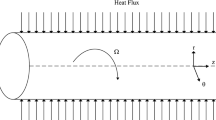
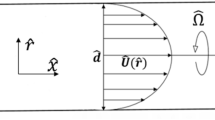
Direct numerical simulations have been performed with a high-order spectral element method computer code to investigate the Coriolis force effect on a fully-developed turbulent flow confined within a circular pipe subjected to radial system rotations. In order to study the radially rotating effects on the flow, a wide range of rotation numbers (Ro_τ) have been tested. In response to the system rotation imposed, large-scale secondary flows appear as streamwise counter-rotating vortices, which highly interact with the boundary layer and have a significant impact on the turbulent flow structures and dynamics. A quasi Taylor-Proudman region occurs at low rotation numbers, where the mean axial velocity is invariant along the rotating axis. As the rotation number increases, laminarization occurs near the bottom wall of the pipe, and the flow becomes fully laminarized when the rotation number approaches Ro_τ = 1.0. The characteristics of the flow field are investigated in both physical and spectral spaces, which include the analyses of the first- and second-order statistical moments, pre-multiplied spectra of velocity fluctuations, budget balance of the transport equation of Reynolds stresses, and coherent flow structures.

Appendix

In this appendix, the transport equations of Reynolds stresses ($\langle u^{\prime }_{z} u^{\prime }_{z}\rangle$, $\langle u^{\prime }_{r} u^{\prime }_{r}\rangle$, $\langle u^{\prime }_{z} u^{\prime }_{r}\rangle$ and $\langle u^{\prime }_{\theta } u^{\prime }_{\theta }\rangle$) in a cylindrical coordinate system are provided. Based on a literature study, it is concluded that there is a necessity to present these transport equations here, as the exact forms of these equations are not readily available in the literature.

The transport equation of $\langle u^{\prime }_{z} u^{\prime }_{z}\rangle$ reads

$$ \begin{array}{@{}rcl@{}} \begin{array}{lll} &\underbrace{\langle u_{r}\rangle\frac{\partial\langle u^{\prime}_{z} u^{\prime}_{z}\rangle}{\partial r} +\frac{\langle u_{\theta}\rangle}{r}\frac{\partial\langle u^{\prime}_{z} u^{\prime}_{z}\rangle}{\partial \theta} +\langle u_{z}\rangle\frac{\partial\langle u^{\prime}_{z} u^{\prime}_{z}\rangle}{\partial z}}_{H_{zz}} \\ &-\left( \underbrace{-2\langle u^{\prime}_{r} u^{\prime}_{z}\rangle\frac{\partial\langle u_{z}\rangle}{\partial r} - 2\frac{\langle u^{\prime}_{\theta} u^{\prime}_{z}\rangle}{r}\frac{\partial\langle u_{z}\rangle}{\partial \theta} - 2\langle u^{\prime}_{z} u^{\prime}_{z}\rangle\frac{\partial\langle u_{z}\rangle}{\partial z}}_{{P_{zz}}}\right) -\underbrace{\frac{2}{\rho}\langle p^{\prime}\frac{\partial u^{\prime}_{z}}{\partial z}\rangle}_{{\Pi}_{zz}} \\ &+\underbrace{2\nu\left[\left\langle\frac{\partial u^{\prime}_{z}}{\partial r}\frac{\partial u^{\prime}_{z}}{\partial r}\right\rangle +\frac{1}{r^{2}}\left\langle\frac{\partial u^{\prime}_{z}}{\partial \theta}\frac{\partial u^{\prime}_{z}}{\partial \theta}\right\rangle +\left\langle\frac{\partial u^{\prime}_{z}}{\partial z}\frac{\partial u^{\prime}_{z}}{\partial z}\right\rangle\right]}_{\varepsilon_{zz}} \\ &-\left\{\underbrace{\begin{array}{llll}&-\frac{1}{r}\frac{\partial r \langle u^{\prime}_{r} u^{\prime}_{z} u^{\prime}_{z}\rangle}{\partial r} - \frac{1}{r}\frac{\partial\langle u^{\prime}_{\theta} u^{\prime}_{z} u^{\prime}_{z}\rangle}{\partial \theta} - \frac{\partial\langle u^{\prime}_{z} u^{\prime}_{z} u^{\prime}_{z}\rangle}{\partial z} -\frac{2}{\rho}\frac{\partial\langle u^{\prime}_{z} p^{\prime}\rangle}{\partial z}\\ &+\nu\left[\frac{1}{r}\frac{\partial}{\partial r}\left( r \frac{\partial\langle u^{\prime}_{z} u^{\prime}_{z}\rangle}{\partial r}\right) +\frac{1}{r^{2}}\frac{\partial^{2}\langle u^{\prime}_{z} u^{\prime}_{z}\rangle}{\partial \theta^{2}} +\frac{\partial^{2}\langle u^{\prime}_{z} u^{\prime}_{z}\rangle}{\partial z^{2}}\right] \end{array}}_{D_{zz}}\right\}\\ &-\left[\underbrace{-4{\Omega}\left( \langle u^{\prime}_{r} u^{\prime}_{z}\rangle\sin\theta + \langle u^{\prime}_{\theta} u^{\prime}_{z}\rangle\cos\theta\right)}_{{C_{zz}}}\right] = 0 \quad. \end{array} \end{array} $$

(10)

The transport equation of $\langle u^{\prime }_{r} u^{\prime }_{r}\rangle$ is

$$ \begin{array}{@{}rcl@{}} \begin{array}{lll} &&{}\underbrace{\langle u_{r}\rangle\frac{\partial\langle u^{\prime}_{r} u^{\prime}_{r}\rangle}{\partial r} +\frac{\langle u_{\theta}\rangle}{r}\frac{\partial\langle u^{\prime}_{r} u^{\prime}_{r}\rangle}{\partial \theta} +\langle u_{z}\rangle\frac{\partial\langle u^{\prime}_{r} u^{\prime}_{r}\rangle}{\partial z} -2\langle u_{\theta}\rangle\frac{\langle u^{\prime}_{r} u^{\prime}_{\theta}\rangle}{r}}_{{H_{rr}}}\\ &&-\left( \underbrace{2\langle u^{\prime}_{r} u^{\prime}_{\theta}\rangle\frac{\langle u_{\theta}\rangle}{r}-2\langle u^{\prime}_{r} u^{\prime}_{r}\rangle\frac{\partial\langle u_{r}\rangle}{\partial r} - 2\frac{\langle u^{\prime}_{r} u^{\prime}_{\theta}\rangle}{r}\frac{\partial\langle u_{r}\rangle}{\partial \theta} - 2\langle u^{\prime}_{r} u^{\prime}_{z}\rangle\frac{\partial\langle u_{r}\rangle}{\partial z}}_{{P_{rr}}}\right) -\underbrace{\frac{2}{\rho}\langle p^{\prime}\frac{\partial u^{\prime}_{r}}{\partial r}\rangle}_{{{\Pi}_{rr}}}\\ &&+\underbrace{2\nu\left[\left\langle\frac{\partial u^{\prime}_{r}}{\partial r}\frac{\partial u^{\prime}_{r}}{\partial r}\right\rangle +\frac{1}{r^{2}}\left\langle\left( \frac{\partial u^{\prime}_{r}}{\partial\theta}- u^{\prime}_{\theta}\right)^{2}\right\rangle +\left\langle\frac{\partial u^{\prime}_{r}}{\partial z}\frac{\partial u^{\prime}_{r}}{\partial z}\right\rangle - \frac{2}{r^{2}}\frac{\partial \langle u^{\prime}_{r} u^{\prime}_{\theta}\rangle}{\partial\theta}\right]}_{{\varepsilon_{rr}}}\\ &&-\left\{ \underbrace{\begin{array}{llll} &2\frac{\langle u^{\prime}_{r} u^{\prime}_{\theta} u^{\prime}_{\theta}\rangle}{r}-\frac{1}{r}\frac{\partial r \langle u^{\prime}_{r} u^{\prime}_{r} u^{\prime}_{r}\rangle}{\partial r} - \frac{1}{r}\frac{\partial\langle u^{\prime}_{r} u^{\prime}_{r} u^{\prime}_{\theta}\rangle}{\partial \theta} - \frac{\partial\langle u^{\prime}_{r} u^{\prime}_{r} u^{\prime}_{z}\rangle}{\partial z} -\frac{2}{\rho}\frac{\partial\langle u^{\prime}_{r} p^{\prime}\rangle}{\partial r}\\ &+\nu\left[\frac{1}{r}\frac{\partial}{\partial r}\left( r \frac{\partial\langle u^{\prime}_{r} u^{\prime}_{r}\rangle}{\partial r}\right) +\frac{1}{r^{2}}\frac{\partial^{2}\langle u^{\prime}_{r} u^{\prime}_{r}\rangle}{\partial \theta^{2}} -\frac{2}{r^{2}}\left( \langle u^{\prime}_{r} u^{\prime}_{r}\rangle -\langle u^{\prime}_{\theta} u^{\prime}_{\theta}\rangle\right) +\frac{\partial^{2}\langle u^{\prime}_{r} u^{\prime}_{r}\rangle}{\partial z^{2}}\right] \end{array}}_{{D_{rr}}}\right\}\\ &&-\left( \underbrace{4{\Omega}\langle u^{\prime}_{r} u^{\prime}_{z}\rangle\sin\theta}_{{C_{rr}}}\right) = 0 \quad. \end{array} \end{array} $$

(11)

The transport equation of $\langle u^{\prime }_{z} u^{\prime }_{r}\rangle$ is

$$ \begin{array}{@{}rcl@{}} \begin{array}{lll} &\underbrace{\langle u_{r}\rangle\frac{\partial\langle u^{\prime}_{r} u^{\prime}_{z}\rangle}{\partial r} +\frac{\langle u_{\theta}\rangle}{r}\frac{\partial\langle u^{\prime}_{r} u^{\prime}_{z}\rangle}{\partial \theta} +\langle u_{z}\rangle\frac{\partial\langle u^{\prime}_{r} u^{\prime}_{z}\rangle}{\partial z} -\langle u_{\theta}\rangle\frac{\langle u^{\prime}_{\theta} u^{\prime}_{z}\rangle}{r}}_{{H_{zr}}} \\ &-\left( \underbrace{\begin{array}{llll}&\langle u^{\prime}_{\theta} u^{\prime}_{z}\rangle\frac{\langle u_{\theta}\rangle}{r} -\langle u^{\prime}_{r} u^{\prime}_{z}\rangle\frac{\partial\langle u_{r}\rangle}{\partial r}-\langle u^{\prime}_{r} u^{\prime}_{r}\rangle\frac{\partial\langle u_{z}\rangle}{\partial r} -\frac{\langle u^{\prime}_{\theta} u^{\prime}_{z}\rangle}{r}\frac{\partial\langle u_{r}\rangle}{\partial \theta}\\ &-\frac{\langle u^{\prime}_{r} u^{\prime}_{\theta}\rangle}{r}\frac{\partial\langle u_{z}\rangle}{\partial \theta} -\langle u^{\prime}_{z} u^{\prime}_{z}\rangle\frac{\partial\langle u_{r}\rangle}{\partial z} -\langle u^{\prime}_{r} u^{\prime}_{z}\rangle\frac{\partial\langle u_{z}\rangle}{\partial z} \end{array}}_{{P_{zr}}}\right) \\ &-\underbrace{\frac{1}{\rho}\left( \langle p^{\prime}\frac{\partial u^{\prime}_{r}}{\partial z}\rangle +\langle p^{\prime}\frac{\partial u^{\prime}_{z}}{\partial r}\rangle\right)}_{{{\Pi}_{zr}}} \\ &+\underbrace{2\nu\left[\left\langle\frac{\partial u^{\prime}_{r}}{\partial r}\frac{\partial u^{\prime}_{z}}{\partial r}\right\rangle +\frac{1}{r^{2}}\left\langle\left( \frac{\partial u^{\prime}_{r}}{\partial\theta}- u^{\prime}_{\theta}\right)\frac{\partial u^{\prime}_{z}}{\partial\theta}\right\rangle +\left\langle\frac{\partial u^{\prime}_{r}}{\partial z}\frac{\partial u^{\prime}_{z}}{\partial z}\right\rangle +\frac{1}{r^{2}}\frac{\partial \langle u^{\prime}_{\theta} u^{\prime}_{z}\rangle}{\partial\theta}\right]}_{{\varepsilon_{zr}}} \\ &-\left\{\underbrace{\begin{array}{llll}&\frac{\langle u^{\prime}_{\theta} u^{\prime}_{\theta} u^{\prime}_{z}\rangle}{r}-\frac{1}{r}\frac{\partial r \langle u^{\prime}_{r} u^{\prime}_{r} u^{\prime}_{z}\rangle}{\partial r} - \frac{1}{r}\frac{\partial\langle u^{\prime}_{r} u^{\prime}_{\theta} u^{\prime}_{z}\rangle}{\partial \theta} - \frac{\partial\langle u^{\prime}_{r} u^{\prime}_{z} u^{\prime}_{z}\rangle}{\partial z} \\ &-\frac{1}{\rho}\left( \frac{\partial\langle u^{\prime}_{z} p^{\prime}\rangle}{\partial r} +\frac{\partial\langle u^{\prime}_{r} p^{\prime}\rangle}{\partial z}\right) \\ &+\nu\left[\frac{1}{r}\frac{\partial}{\partial r}\left( r \frac{\partial\langle u^{\prime}_{r} u^{\prime}_{z}\rangle}{\partial r}\right) +\frac{1}{r^{2}}\frac{\partial^{2}\langle u^{\prime}_{r} u^{\prime}_{z}\rangle}{\partial \theta^{2}} -\frac{\langle u^{\prime}_{r} u^{\prime}_{z}\rangle}{r^{2}} +\frac{\partial^{2}\langle u^{\prime}_{r} u^{\prime}_{z}\rangle}{\partial z^{2}}\right] \end{array}}_{{D_{zr}}}\right\} \\ &-\left[\underbrace{2{\Omega}\left( \langle u^{\prime}_{z} u^{\prime}_{z}\rangle\sin\theta -\langle u^{\prime}_{r} u^{\prime}_{r}\rangle\sin\theta -\langle u^{\prime}_{r} u^{\prime}_{\theta}\rangle\cos\theta\right)}_{{C_{zr}}}\right] = 0 \quad. \end{array} \end{array} $$

(12)

The transport equation of $\langle u^{\prime }_{\theta } u^{\prime }_{\theta }\rangle$ is

$$ \begin{array}{@{}rcl@{}} \begin{array}{lll} &\underbrace{\langle u_{r}\rangle\frac{\partial\langle u^{\prime}_{\theta} u^{\prime}_{\theta}\rangle}{\partial r} + \frac{\langle u_{\theta}\rangle}{r}\frac{\partial\langle u^{\prime}_{\theta} u^{\prime}_{\theta}\rangle}{\partial \theta} + \langle u_{z}\rangle\frac{\partial\langle u^{\prime}_{\theta} u^{\prime}_{\theta}\rangle}{\partial z} + 2\langle u_{\theta}\rangle\frac{\langle u^{\prime}_{\theta} u^{\prime}_{\theta}\rangle}{r}}_{{H_{\theta\theta}}} \\ &-\left( \underbrace{-2\langle u^{\prime}_{r} u^{\prime}_{\theta}\rangle\frac{\langle u_{\theta}\rangle}{r}-2\langle u^{\prime}_{r} u^{\prime}_{\theta}\rangle\frac{\partial\langle u_{\theta}\rangle}{\partial r} - 2\frac{\langle u^{\prime}_{\theta} u^{\prime}_{\theta}\rangle}{r}\frac{\partial\langle u_{\theta}\rangle}{\partial \theta} - 2\langle u^{\prime}_{\theta} u^{\prime}_{z}\rangle\frac{\partial\langle u_{\theta}\rangle}{\partial z}}_{{P_{\theta\theta}}}\right) \\ &-\underbrace{\frac{2}{\rho r}\langle p^{\prime}\frac{\partial u^{\prime}_{\theta}}{\partial {\theta}}\rangle}_{{{\Pi}_{\theta\theta}}} \\ &+\underbrace{2\nu\left[\left\langle\frac{\partial u^{\prime}_{\theta}}{\partial r}\frac{\partial u^{\prime}_{\theta}}{\partial r}\right\rangle +\frac{1}{r^{2}}\left\langle\left( \frac{\partial u^{\prime}_{\theta}}{\partial\theta}+ u^{\prime}_{r}\right)^{2}\right\rangle +\left\langle\frac{\partial u^{\prime}_{\theta}}{\partial z}\frac{\partial u^{\prime}_{\theta}}{\partial z}\right\rangle - \frac{2}{r^{2}}\frac{\partial \langle u^{\prime}_{r} u^{\prime}_{\theta}\rangle}{\partial\theta}\right]}_{{\varepsilon_{\theta\theta}}} \\ &-\left\{\underbrace{\begin{array}{llll}&-2\frac{\langle u^{\prime}_{r} u^{\prime}_{\theta} u^{\prime}_{\theta}\rangle}{r}-\frac{1}{r}\frac{\partial r \langle u^{\prime}_{r} u^{\prime}_{\theta} u^{\prime}_{\theta}\rangle}{\partial r} - \frac{1}{r}\frac{\partial\langle u^{\prime}_{\theta} u^{\prime}_{\theta} u^{\prime}_{\theta}\rangle}{\partial \theta} - \frac{\partial\langle u^{\prime}_{\theta} u^{\prime}_{\theta} u^{\prime}_{z}\rangle}{\partial z} - \frac{2}{\rho r}\frac{\partial\langle u^{\prime}_{\theta} p^{\prime}\rangle}{\partial {\theta}} \\ &+\nu\left[\frac{1}{r}\frac{\partial}{\partial r}\left( r \frac{\partial\langle u^{\prime}_{\theta} u^{\prime}_{\theta}\rangle}{\partial r}\right) +\frac{1}{r^{2}}\frac{\partial^{2}\langle u^{\prime}_{\theta} u^{\prime}_{\theta}\rangle}{\partial \theta^{2}} +\frac{2}{r^{2}}\left( \langle u^{\prime}_{r} u^{\prime}_{r}\rangle -\langle u^{\prime}_{\theta} u^{\prime}_{\theta}\rangle\right) +\frac{\partial^{2}\langle u^{\prime}_{\theta} u^{\prime}_{\theta}\rangle}{\partial z^{2}}\right] \end{array}}_{{D_{\theta\theta}}}\right\} \\ &-\left( \underbrace{4{\Omega}\langle u^{\prime}_{\theta} u^{\prime}_{z}\rangle\cos\theta}_{{C_{\theta\theta}}}\right) = 0 \quad. \end{array} \end{array} $$

(13)