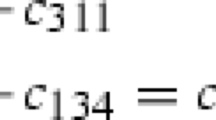A five-dimensional Riemannian manifold with an irreducible SO(3)-structure as a model of abstract statistical manifold

Abstract

In the present paper, we consider a five-dimensional Riemannian manifold with an irreducible SO(3)-structure as an example of an abstract statistical manifold. We prove that if a five-dimensional Riemannian manifold with an irreducible SO(3)-structure is a statistical manifold of constant curvature, then the metric of the Riemannian manifold is an Einstein metric. In addition, we show that a five-dimensional Euclidean sphere with an irreducible SO(3)-structure cannot be a conjugate symmetric statistical manifold. Finally, we show some results for a five-dimensional Riemannian manifold with a nearly integrable SO(3)-structure. For example, we prove that the structure tensor of a nearly integrable SO(3)-structure on a five-dimensional Riemannian manifold is a harmonic symmetric tensor and it defines the first integral of third order of the equations of geodesics. Moreover, we consider some topological properties of five-dimensional compact and conformally flat Riemannian manifolds with irreducible SO(3)-structure.

1 Introduction

In the present paper, we consider a five-dimensional Riemannian manifold with an irreducible SO(3)-structure as an example of an abstract statistical manifold used in information geometry. On one side, our paper shows new geometrical interpretation to the theory of abstract statistical manifolds, on the other side, we study new geometrical and topological properties of five-dimensional manifolds with SO(3)-structures.

This paper is a continuation of studies of abstract statistical manifolds, which the second author began in her papers [49, 50].

The paper is organized as follows. In Sect. 2, we give brief overview of the information geometry, the theories of abstract statistical manifolds and irreducible SO(3)-structures. In Sect. 3 of the paper we consider conjugate connections on statistical manifolds which are one of the fundamental concepts of information geometry. We also consider properties of a well-known conjugate symmetric statistical manifold and define a harmonic statistical manifold. In addition, we prove “vanishing theorems” about obstructions to the existence of compact conjugate symmetric statistical manifolds with the positive definite curvature operator and compact harmonic statistical manifolds with the negative definite curvature operator. For this, we use the Bochner technique that is one of the oldest and most important techniques in modern Riemannian geometry [41, pp. 187–234]. In Sect. 4, we study a five-dimensional Riemannian manifold with an irreducible SO(3)-structure as a model of an abstract statistical manifold. For example, we prove that if a five-dimensional Riemannian manifold with an irreducible SO(3)-structure is a statistical manifold of constant curvature, then the metric of this Riemannian manifold is an Einstein metric. In addition, we show that a five-dimensional Euclidean sphere with an irreducible SO(3)-structure cannot be a conjugate symmetric statistical manifold. Moreover, we consider some topological properties of such manifolds. Finally, we present some results for a five-dimensional Riemannian manifold with a nearly integrable SO(3)-structure. For example, we prove that the structure tensor of a nearly integrable SO(3)-structure on a five-dimensional Riemannian manifold is a harmonic symmetric tensor and it defines the first integral of third order of the equations of geodesics. Moreover, we show that an irreducible SO(3)-structure on a five-dimensional Riemannian manifold cannot be nearly integrable if the curvature operator of Riemannian manifold is negative definite.

2 Basis definitions and notations

2.1 Information geometry

In this section, we present brief historical overview of information geometry. We want to highlight how many researchers have contributed to the development of information geometry, its theory and applications.

Information geometry studies invariant properties of a family of probability distributions and can be applied to various problems in science. Statisticians use statistical models to derive inferences; they use families of probability distributions which form, in most cases, a finite-dimensional manifold which in information geometry is called a statistical manifold. Then one can ask: What are the intrinsic properties of such manifold and how the geometrical structure of such manifold is related to characteristics of statistical inference? Information geometry emerged from these questions [4].

Many authors contributed to the development of information geometry and geometrical theory of statistics. The results of their research are reflected in numerous papers and the following monographs ([1, 3, 7, 8, 12, 27, 33, 34, 53] and etc.). Moreover, a number of international workshops and symposiums on this subject were held: in UK (London, July 10–14, 2000), Italy (Pescara, Sept. 1–5, 2002), Denmark (Leipzig, August 27, 2003), Canada (Toronto, May 8–18, 2004), USA (Ann Arbor, July 29, 2004), Japan (Tokyo, December 12–16, 2005 and Nara, March 6–10, 2012), China (Chenghu, September 3, 2006) and other countries.

There are two main natural geometrical objects on any statistical model equipped with a differentiable structure. Namely, they are the Fisher information tensor and the Chentsov–Amari connection.

The information tensor was introduced by R.A. Fisher in 1925 as an information characterization of a statistical model [22]. In the 1945 paper [43], Rao pointed out that the Fisher information tensor determines a Riemannian metric (which is now called the information metric or Fisher metric) for the manifold obtained from the family of probability density functions.

Let us consider the recent concept of this metric in more details. Let $\Theta \subseteq \mathbf{R}^{n}$ be the parameter space of an $n$-dimensional smooth family defined on some fixed event space $\Omega$, i.e., $\{ {p_\theta | { \theta \in \Theta }}\}$ with $\int _\Omega {p_\theta } =1$ for all $\theta \in \Theta$. Under certain assumptions, the Fisher information matrix defines the unique Riemannian metric $g$ on $\Omega$ with the following components [3, p. 32], [7, p. 28]:

$$\begin{aligned} g_{ij} =E_\theta [ {\partial _i \text{ log }\,p_\theta \partial _i \text{ log }\,p_\theta }] =\int _\Omega {p_\theta \left( {\frac{\partial l}{\partial \theta ^{i}}\frac{\partial l}{\partial \theta ^{j}}} \right) } =-\int _\Omega {p_\theta \left( {\frac{\partial ^{2}l}{\partial \theta ^{i}\partial \theta ^{j}}} \right) }, \end{aligned}$$

where $E_\theta$ denotes the expectation with respect to $p_\theta$, i.e., $E_\theta [f]:=\int _\Omega {f(x)} p_\theta , l=\text{ log }\,p_\theta$ and $\theta ^{i}$ are coordinates of $\theta \in \Theta \subseteq \mathbf{R}^{n}$. Then the set $S=\{ {p_\theta | { \theta \in \Theta \subset \mathbf{R}^{n}}}\}$ with the Fisher metric $g$ can be considered as a Riemannian manifold ($S, g$) [3, p. 34], [7, p. 32].

Chentsov proved that the Fisher metric is a unique invariant metric [14]. Moreover, he defined in [14] a one-parameter group of invariant affine connections in the space of statistic distributions (which is now called the Chentsov–Amari connections), leaving Efron [19] to expose the relationship between statistical curvature and the characteristics of inference. S.-I. Amari and H. Nagaoka introduced a conjugate structure (or duality structures) in information geometry, a finding that has played a fundamental role in development of more applications of information geometry [7]. In particular, the notion of dually flat metrics was first introduced by Amari and Nagaoka [7] when they studied information geometry on Riemannian spaces. Later on, Shen extended the notion of locally dually flatness for Finsler metrics [47]. He identified and studied the dually flat Finsler metrics that are a special and valuable class of Finsler metrics in Finsler geometry, which play a very important role in studying flat Finsler information structures [16].

Next, we shall inform the reader about the basic facts of the geometry of Chentsov–Amari connections. Namely, consider for $\alpha \in \mathbf{R}$ functions ${\mathop {\Gamma }\limits ^{\alpha }}_{ij,k}$ which map each point $\theta \in \Theta$ to the following value [3, p. 34], [7, p. 32]:

$$\begin{aligned} {\mathop {\Gamma }\limits ^{\alpha }}_{ij,k}=E_\theta \left[ {\left( {\partial _i \partial _j l+\frac{1-\alpha }{2}\partial _i l\partial _j l} \right) \left( {\partial _k l} \right) } \right] =\int _\Omega {\left( {\partial _i \partial _j l+\frac{1-\alpha }{2}\partial _i l\partial _j l} \right) } \quad \partial _k l\;p_\theta . \end{aligned}$$

For every $\alpha \in \mathbf{R}$, the $n^{3}$ functions ${\mathop {\Gamma }\limits ^{\alpha }}_{ij,k}$ are the Christoffel symbols of an affine $\alpha$-connection ${\mathop {\nabla }\limits ^{\alpha }}$ on $S$. This connection is clearly a symmetric connection. Moreover, the relationship between the $\alpha$-connection ${\mathop {\nabla }\limits ^{\alpha }}$ and $\beta$-connection ${\mathop {\nabla }\limits ^{\beta }}$ is given by (see [7, p. 33])

$$\begin{aligned} {\mathop {\Gamma }\limits ^{\beta }}_{ij,k} - {\mathop {\Gamma }\limits ^{\alpha }}_{ij,k} =\frac{\alpha -\beta }{2}\,T_{ijk}, \end{aligned}$$

where $T_{ijk}$ are components of a covariant completely symmetric tensor field of order 3 which is defined by the equation $T_{ijk} =E_\theta [ {\partial _i l \partial _j l \partial _k l}]$. In addition, we also note that ${\mathop {\nabla }\limits ^{\alpha }} =\frac{1+\alpha }{2} \,{\mathop {\nabla }\limits ^{1}} +\frac{1-\alpha }{2}\,{\mathop {\nabla }\limits ^{-1}}$ and the 0-connection is the Levi-Civita connection with respect to the Fisher metric [7, p. 33]. Numerous articles develop further the theory of the Chentsov–Amari connection.

In addition, now we can see a new trend of the information geometry which is called Quantum information theory [12, 21, 23, 37, 42]. This theory is based on information geometry and quantum mechanics. For example, Nagaoka [37] and Petz [42] studied the information geometry of quantum probability. On the other hand, Gibilisco and Isola in [21] gave a mathematical foundation that extended information geometry to the function space. In particular, they showed that a family of inequalities, which relates to the uncertainty principle, has a geometric interpretation in terms of quantum Fisher information. At the same time, Cafaro [12] considered problems of classical and quantum chaos using the information geometry.

Further on the role of differential geometry and its applications in the probability theory and statistics, one can refer to [11] and to the special issue of AISM 59 : 1 (2007) with the title “Information Geometry and its Applications”. In particular, some authors [6, 51, 54] reported applications of information geometry to Bayesian statistics [32] and Bartlett correction [15]. In addition, we must say that information geometry is applied not just to statistics and information theory but also to combinatorics of neural networks and psychology [5], physics [20] and many other fields.

2.2 An abstract statistical manifold

Lauritzen [8, pp. 165–215] gave a modern differential geometric treatment of statistical problems by introducing the notion of an abstract statistical manifold. This generalization has been considered in many papers by other geometers ([6, 24, 29, 30, 49, 50] and etc.). In this section, we give an overview of the geometry of such manifold.

According to Lauritzen [8, p. 179], a statistical manifold is a triplet ($M, g, T$), where $M$ is a connected $C^{\infty }$-manifold, $g$ is a Riemannian metric with components $g_{ij}$ and $T$is a smooth covariant completely symmetric tensor field of order 3 with components $T_{ijk} =T( { \partial _i, \partial _j, \partial _k })$ on each chart $U({x^{1},\ldots ,x^{n}})\subset M$, i.e., $T \in C^{\infty }S^{3}M$. This tensor $T$ is called the skewness tensor [8, p. 179]. Further, Lauritzen defines a one-parameter group of affine torsion-free $\alpha$-connections ${\mathop {\nabla }\limits ^{\alpha }}$ whose Christoffel symbols ${{\mathop {\Gamma }\limits ^{\alpha }}}{_{ij}^{k}}$ on each chart $U( {x^{1},\ldots ,x^{n}})\subset M$are related to the Cristoffel symbols $\Gamma _{ij}^k$ of the Levi-Civita connection $\nabla$ by the following identities:

$$\begin{aligned} {\mathop {\Gamma }\limits ^{\alpha }}{_{ij}^{k}} =\Gamma _{ij}^k -\frac{\alpha }{2}T_{ij}^{k} \end{aligned}$$

(2.1)

for a real parameter $\alpha$ and

$$\begin{aligned} T_{ij}^{l}=g^{lk}T_{ijk}, \end{aligned}$$

(2.2)

where the inverse of the matrix $({g_{ij}})$ is denoted by $({g^{ij}})$. After that, Lauritzen proves the identity [8, p. 180]

$$\begin{aligned} {\mathop {\nabla }\limits ^{\alpha }}_{k}\,g_{ij} =\alpha \cdot T_{kij}, \end{aligned}$$

(2.3)

and hence ${\mathop {\nabla }\limits ^\alpha }g$ is a covariant completely symmetric tensor field of order 3. The identities (2.3) are called the Codazzi equations and $g$ is called a Codazzi tensor with respect to $\alpha$-connections ${\mathop {\nabla }\limits ^{\alpha }}$ [39, p. 21], [48, pp. 56, 68, 142].

It is well known from [17], [48, p. 53] that the affine torsion-free connections ${\mathop {\nabla }\limits ^{\alpha }}$ and ${\mathop {\nabla }\limits ^{\beta }}$ are called conjugate connections (or dual connections) relative to the non-degenerate bilinear form $g$ if their Christoffel symbols ${\mathop {\Gamma }\limits ^\alpha }{_{ik}^{l}}$ and ${\mathop {\Gamma }\limits ^\beta }{_{ik}^{l}}$ on each chart $U({x^{1},\ldots ,x^{n}})\subset M$ satisfy the following conditions:

$$\begin{aligned} \partial _k\,g_{ij}=g_{jl} {\mathop {\Gamma }\limits ^{\alpha }}{_{ik}^{l}} +g_{il} {\mathop {\Gamma }\limits ^{\beta }}{_{jk}^{l}}. \end{aligned}$$

(2.4)

In this case, we say that $\{ {\mathop {\nabla }\limits ^{\alpha }},g, {\mathop {\nabla }\limits ^{\beta }}\}$ are conjugates. In particular, from (2.4) we conclude that $\{{\mathop {\nabla } \limits ^\alpha }, g, {\mathop {\nabla }\limits ^{-\alpha }}\}$ are conjugate and the Levi-Civita connection $\nabla :={\mathop {\nabla }\limits ^0}$ is a self-conjugate connection [8, p. 181]. Conjugate connections have a simple interpretation [39, p. 21].

Next, Lauritzen [8, p. 185] introduces a tensor field $F\in C^{\infty }S^{3}M$ which plays an important role for the statistical manifold. It is defined as follows:

$$\begin{aligned} F_{ijkl} =\nabla _l T_{ijk}. \end{aligned}$$

(2.5)

If $F\in C^{\infty }S^{4}M$, i.e., $F$ is completely symmetric, then ($M, g, T$) is said to be a conjugate symmetric statistical manifold. For this manifold, a simple calculation shows that

$$\begin{aligned} 0={\mathop {\nabla }\limits ^{\alpha }}_{l} {\mathop {\nabla }\limits ^{\alpha }}_{k}\,g_{ij} -{\mathop {\nabla }\limits ^{\alpha }}_{l} {\mathop {\nabla }\limits ^{\alpha }}_{k}\,g_{ij} = - {\mathop {R}\limits ^{\alpha }}_{jilk} -{\mathop {R}\limits ^{\alpha }}_{ijlk}, \end{aligned}$$

where ${\mathop {R}\limits ^\alpha }_{jilk} =g_{jm} {\mathop R\limits ^\alpha }{_{ilk}^{m}}$ for the local components ${\mathop R\limits ^\alpha }{_{ilk}^{m}}$ of the $\alpha$-curvature tensor ${\mathop {R}\limits ^{\alpha }}$ of ${\mathop {\nabla }\limits ^{\alpha }}$. Therefore, a conjugate symmetric statistical manifold has the following curvature: properties [8, p. 185]

$$\begin{aligned} {\mathop {R}\limits ^{\alpha }} (X,Y,Z,V)=-{\mathop {R}\limits ^{\alpha }} (X,Y,V,Z);\quad {\mathop {R}\limits ^{\alpha }} (X,Y,Z,V)={\mathop {R}\limits ^{\alpha }}(Z,V,X,Y), \end{aligned}$$

(2.6)

where ${\mathop {R}\limits ^{\alpha }}(X,Y,Z,V)=g({\mathop {R} \limits ^{\alpha }}(X,Y)Z,V)$ for any vectors $X,Y,Z,V\in T_{x} M$ at each point $x\in M$.

On the other hand, Takeuchi and Amari showed in [52] that the sufficient condition for a statistical manifold to be conjugate symmetric is that its connection ${\mathop {\nabla }\limits ^{\alpha }}$ is equiaffine. If we recall from [39, p. 14], [48, pp. 57–58] that a torsion-free affine connection ${\mathop {\nabla }\limits ^{\alpha }}$ is called an equiaffine or Ricci-symmetric connection if ${\mathop {\nabla }\limits ^{\alpha }}\,{\mathop {\omega }\limits ^{\alpha }} =0$ for the volume form ${\mathop {\omega } \limits ^{\alpha }}:x\in M\rightarrow {\mathop {\omega } \limits ^{\alpha }}_{x} ={\mathop {\omega }\limits ^{\alpha }} (X_{1}, X_{2},\ldots ,X_{n}),$ where $X_1,X_2,\ldots ,X_n \in T_x M$ at each point $x\in M$, then we see that the Ricci tensor ${\mathop {Ric}\limits ^{\alpha }}$ of ${\mathop {\nabla }\limits ^{\alpha }}$ is symmetric. The converse is true.

Next, for $\alpha =1$ the $\alpha$-curvature tensor ${\mathop {Ric}\limits ^{\alpha }}$ and Ricci $\alpha$-curvature tensor ${\mathop {Ric}\limits ^{\alpha }}$ of the connection ${\mathop {\nabla }\limits ^{\alpha }}$ are called the curvature and Ricci tensors of a statistical manifold ($M, g, T$), respectively [24, 29, 30]. The scalar $\alpha$-curvature ${\mathop {s}\limits ^{\alpha }} :=\text{ trace }_g {\mathop {Ric}\limits ^{\alpha }}$ is called a scalar curvature of a statistical manifold ($M, g, T$) if $\alpha =1$. Moreover, the statistical manifold ($M, g, T$) is said to be of constant curvature if

$$\begin{aligned} {\mathop {R}\limits ^{1}}{_{jkl}^{i}} =\frac{1}{n(n-1)}{\mathop {s}\limits ^1}\cdot ( {g_{jl} \delta _k^i -g_{jk} \delta _l^i }), \end{aligned}$$

(2.7)

where ${\mathop {R}\limits ^1}(\partial _k,\partial _l) \partial _j ={\mathop {R}\limits ^1}{_{jkl}^{i}} \partial _i$ for $\partial _k =\partial /{\partial x^{k}}$. In particular, a statistical manifold ($M, g, T$) is said to be locally flat if ${\mathop {R}\limits ^{1}} =0$.

Finally we introduce the new class of statistical manifolds that we shall call as harmonic statistical manifolds. Before we present two differential operators of order 1. Namely, the operator $\delta ^{{*}}: \text{ C }^{\infty }S^{3}M\rightarrow C^{\infty }S^{4}M$ such that [9, p. 35]; [45]

$$\begin{aligned} ({\delta ^{{*}}T})_{kijl} =\nabla _k T_{ijl} +\nabla _i T_{jlk} +\nabla _j T_{lki} +\nabla _l T_{kij} \end{aligned}$$

and its formal adjoint differential operator $\delta : \text{ C }^{\infty }S^{4}M\rightarrow C^{\infty }S^{3}M$, which is defined by

$$\begin{aligned} ({\delta T})_{jk} =-\nabla _l T^{l}_{jk}. \end{aligned}$$

Then we can present the Laplacian $\Delta _{\mathrm{sym}} :C^{\infty }S^{3}M\rightarrow C^{\infty }S^{3}M$ acting on smooth covariant symmetric tensor fields which is defined as $\Delta _{\mathrm{sym}} :=\delta ^{{*}}\delta -\delta \delta ^{{*}}$ [9, pp. 52–54], [45]. The operator $\Delta _{\mathrm{sym}}$ is related to a variational problem as follows: If we define the “energy” of symmetric tensor field $T$ by $E(T)= 1/2\langle {T,\Delta _{\mathrm{sym}} T}\rangle$, then $\Delta _{\mathrm{sym}} T=0$ is the condition for a free extremal of $E(T)= 1/2\langle {T,\Delta _{\mathrm{sym}} T} \rangle$. Such tensor is called harmonic [45]. In accordance with this definition, we shall call ($M, g, T$) a harmonic statistical manifold if $\Delta _{\mathrm{sym}} T=0$.

In the Sect. 4.2, we shall consider a five-dimensional Riemannian manifold ($M, g$) with a nearly integrable SO(3)-structure as an example of a harmonic statistical manifold.

2.3 Overview of the theory of irreducible SO(3)-structures

It is well known that a Riemannian metric $g$ on an $n$-dimensional orientable manifold $M$ determines SO($n$)-structures, where the tangent space $T_{x}M$ at each point $x \in M$ behaves as a representation for SO($n$). An arbitrary subgroup $\text{ G }\subset SO(n)$ determines restricted Riemannian $G$-structure, i.e., the tangent space $T_{x}M$ must behave as a representation for $G$. Usually, the tangent space $T_{x}M$ is regarded as an irreducible representation of a subgroup $\text{ G }\subset SO(n)$. Following series of papers, some [2, 10, 13] consider an irreducible SO(3)-structure on a five-dimensional Riemannian manifold ($M, g$). In particular, it was shown that an irreducible SO(3)-structure on a five-dimensional Riemannian manifold ($M, g$) is a structure defined by means of a smooth covariant completely symmetric and trace free tensor field $T$of order 3 which satisfies on each chart $U({x^{1},\ldots ,x^{5}})\subset M$ the following condition:

$$\begin{aligned} T_{ijm} T_{kl}^{m} +T_{imk} T_{jl}^{m} +T_{mjk} T_{il}^{m} =g_{ij} g_{kl} +g_{ik} g_{jl} +g_{jk} g_{il}. \end{aligned}$$

(2.8)

For the tensor, $T\in C^{\infty }S_0^3 M$ the identities (2.8) after contraction with $g_{ij}$ and $T_m ^{ij}$, respectively, imply

$$\begin{aligned} T_{ikl} T_j^{kl}&= \frac{7}{2}g_{ij}.\end{aligned}$$

(2.9)

$$\begin{aligned} T_{il}^{m} T_{jn}^{l} T_{km}^{n}&= -\frac{3}{4}T_{ijk}. \end{aligned}$$

(2.10)

A five-dimensional Riemannian manifold ($M, g$) with an irreducible SO(3)-structure was obtained as ($M, g, T$). Further, authors in [10] define a characteristic ${\mathop {\nabla }\limits ^\Gamma }$ connection of ($M, g, T$) with skew-symmetric torsion tensor such that ${\mathop {\nabla }\limits ^\Gamma }\,g=0;\,{\mathop {\nabla }\limits ^\Gamma } T=0$ and prove that an irreducible SO(3)-structure ($M, g, T$) admits a characteristic connection ${\mathop {\nabla }\limits ^\Gamma }$ if it is nearly integrable, i.e., tensor field $T$ satisfies $\delta ^{{*}}T= 0$.

3 Conjugate symmetric and harmonic statistical manifolds

3.1 Conjugate connections on statistical manifolds

Let ($M, g, T$) be an $n$-dimensional statistical manifold. On each chart $U(x^{1},\ldots ,x^{n})\subset M$, the local components of the curvature tensors ${\mathop {R}\limits ^{\alpha }}$ of the connection ${\mathop {\nabla }\limits ^{\alpha }}$ and $R$ of the Levi-Civita connection $\nabla$ are related by the equation [18, p. 33]

$$\begin{aligned} {\mathop {R}\limits ^{\alpha }}{_{jkl}^{i}} =R^{i}_{jkl} \,+\frac{\alpha }{2}( {\nabla _l T_{jk}^i -\nabla _k T_{jl}^i} )+\frac{\alpha ^{2}}{4}( {T_{jl}^m T_{mk}^i -T_{jk} ^{m} T_{ml} ^{i}}), \end{aligned}$$

(3.1)

where ${\mathop {R}\limits ^{\alpha }}(X_k,X_l)X_j ={\mathop {R}\limits ^{\alpha }}{_{jkl}^{i}} X_i$ and $R(X_k,X_l)X_j =R_{jkl}^{i} X_i$ for $X_k =\partial /{\partial x^{k}}$. Contracting with respect to the indices $k$ and $i$ in (3.1), we obtain the equalities

$$\begin{aligned} {\mathop {R}\limits ^{\alpha }}_{jl} =R_{jl} +\frac{\alpha }{2}( {\nabla _l T_j -\nabla _k T_{jl}^k })+\frac{\alpha ^{2}}{4}({T_m T_{jl} ^{m}-T_{jk} ^{m} T_{lm} ^{k}}), \end{aligned}$$

(3.2)

which connect the Ricci curvature tensor ${\mathop {Ric}\limits ^{\alpha }}$ of the $\alpha$-connection ${\mathop {\nabla }\limits ^{\alpha }}$ and the Ricci curvature tensor $Ric$ of the Levi-Civita connection $\nabla$. In addition, we can rewrite the equalities in the form

$$\begin{aligned} {\mathop {R}\limits ^{-\alpha }\!}_{jl} =R_{jl} -\frac{\alpha }{2}( {\nabla _l T_j -\nabla _k T_{jl}^k })+\frac{\alpha ^{2}}{4}( {T_m T_{jl} ^{m}-T_{jk} ^{m} T_{lm} ^{k}}). \end{aligned}$$

(3.3)

Equality (3.3) connects the Ricci curvature tensor $Ric$ of the Levi-Civita connection $\nabla$ and the Ricci tensor ${\mathop {Ric}\limits ^{-\alpha }}$ of the connection ${\mathop {\nabla }\limits ^{-\alpha }}$ which is a conjugate to ${\mathop {\nabla }\limits ^{\alpha }}$ relative to the metric $g$. Using (3.2) and (3.3), we obtain the following identities:

$$\begin{aligned} {\mathop {R}\limits ^{\alpha }}_{jl} -{\mathop {R}\limits ^{-\alpha }}_{jl} =\alpha \,({\nabla _l T_j -\nabla _k T_{jl}^k }). \end{aligned}$$

(3.4)

Now we recall the definition of the Tchebychev form $\tau$ [48, p. 58]. Namely, for conjugate connections $\{{\mathop {\nabla }\limits ^{\alpha }}, g, {\mathop {\nabla }\limits ^{-\alpha }}\}$ the Tchebychev form $\tau$ has local components $T_{k}=g^{ij}T_{ijk}$ for $T_{ijk} ={\mathop {\Gamma }\limits ^{\alpha }}_{ij,k} -{\mathop {\Gamma }\limits ^{-\alpha }}_{ij,k}$. Therefore, if ${\mathop {R}\limits ^{\alpha }}_{jl} ={\mathop {R}\limits ^{-\alpha }}_{jl}$ then from (3.4) we have $\nabla _j T_l =\nabla _l T_j$ and hence ${\mathop {\nabla }\limits ^{\alpha }}$ is equiaffine. This shows that the Tchebychev form $\tau$ is locally an exact 1-form $d f$ for some smooth scalar potential function $f$for $\tau$.

On the other hand, the Christoffel symbols ${\mathop {\Gamma }\limits ^{\alpha }}{_{ij}^{k}}$ of an equiaffine connection ${\mathop {\nabla }\limits ^{\alpha }}$ satisfy the equalities [48, pp. 57–59]

$$\begin{aligned} {\mathop {\Gamma }\limits ^{\alpha }}{_{kj}^{k}} =\partial _j \left( {\text{ ln } \ {\mathop {\omega }\limits ^{\alpha }{}_{\!12\ldots n}}}\right) . \end{aligned}$$

But this time we know that the Christoffel symbols $\Gamma _{jk}^l$ of the Levi-Civita connection $\nabla$ satisfy the following condition: $\Gamma _{kj}^k =\partial _j ( {\text{ ln }\sqrt{\text{ det } g}})$, where $\omega =\sqrt{\text{ det }g}\,\mathrm{d}x^{1}\wedge \cdots \wedge \mathrm{d}x^{n}$ is the Riemannian volume form of $g$. Then using the above conditions for ${\mathop {\Gamma }\limits ^{\alpha }}{_{kj}^{k}}$ and $\Gamma _{kj}^k$, we can transform equalities (2.1) into the differential equations $\partial _k \text{ ln } \omega _{12 \ldots n} =\partial _k \text{ ln }\sqrt{\text{ det } g}+\frac{\alpha }{2} \partial _k f$, where $f$ is a potential function for $\tau$. From this we can see that ${\mathop {\omega }\limits ^{\alpha }}_{12\ldots n} =\sqrt{\text{ det } \tilde{g}}$, where $\tilde{g}=\text{ exp }( {\frac{C+\alpha f}{n}}) g$ for an arbitrary constant $C$.

Next, if we suppose that the Tchebychev form $\tau =T_k dx^{k}$is locally an exact 1-form, then from (3.2) and (3.3) we conclude that the $\alpha$-connection ${\mathop {\nabla }\limits ^{\alpha }}$ is equiaffine and its conjugate connection ${\mathop {\nabla }\limits ^{-\alpha }}$ is equiaffine, too. Next, multiplying by $g^{jl}$ on both sides of (3.2) yields

$$\begin{aligned} {\mathop {s}\limits ^{\alpha }} =s+\frac{\alpha ^{2}}{4}({ \Vert { \tau } \Vert ^{2}-\Vert { T }\Vert ^{2}} ), \end{aligned}$$

where $s=g^{jk}R_{jk}$ is the scalar curvature of $g$ and ${\mathop {s}\limits ^{\alpha }} =g^{jk}{\mathop {R}\limits ^{\alpha }}_{jk}$ is the $scalar\,\alpha$-curvature of ${\mathop {\nabla }\limits ^{\alpha }}$. Hence, we have ${\mathop {s}\limits ^{\alpha }} ={\mathop {s}\limits ^{-\alpha }}$ [48, p. 60] and if the Tchebychev form $\tau = 0$ then ${\mathop {s}\limits ^{\alpha }} \le s$. Finally, from the above considerations, we have the following.

Theorem 3.1

Let ($M, g, T$) be an $n$-dimensional statistical manifold. Assume that $\tau = \text{ trace }\,T$ is the Tchebychev form of conjugate connections $\{{\mathop {\nabla }\limits ^{\alpha }}, g, {\mathop {\nabla }\limits ^{-\alpha }}\}$, where ${\mathop {\nabla }\limits ^{\alpha }}$ is a connection of one-parameter family of affine $\alpha$-connections on M. Then the following propositions hold.

• 1. ${\mathop {s}\limits ^{-\alpha }} ={\mathop {s}\limits ^{\alpha }} = s+\frac{\alpha ^{2}}{4}( { \Vert { \tau } \Vert ^{2}-\Vert { T }\Vert ^{2}})$, where ${\mathop {s}\limits ^{\alpha }}$ and $\mathop s\limits ^{-\alpha }$ are scalar $\alpha$ -curvature and ($-\alpha$)-curvature of ${\mathop {\nabla }\limits ^{\alpha }}$ and ${\mathop {\nabla }\limits ^{-\alpha }}$, respectively.

• 2. If the Tchebychev form $\tau$ is locally an exact 1-form, then ${\mathop {\nabla }\limits ^{\alpha }}$ and ${\mathop {\nabla }\limits ^{-\alpha }}$ are equiaffine for an arbitrary $\alpha$.

• 3. If ${\mathop {Ric}\limits ^{\alpha }} ={\mathop {Ric}\limits ^{-\alpha }}$, then the Tchebychev form $\tau$ is locally an exact 1-form with potential function $f,{\mathop {\nabla }\limits ^{\alpha }}$ is an equiaffine connection and the volume form ${\mathop {\omega }\limits ^{\alpha }}=\sqrt{\mathrm{det} \tilde{g}}$ for the Riemannian metric $\tilde{g}=\mathrm{exp}( {\frac{C+\alpha f}{n}}) g$, where C is an arbitrary constant, which is conformal to g.

3.2 A conjugate symmetric statistical manifold

In this section, we consider a conjugate symmetric statistical manifold ($M, g, T$). This means that $F=\nabla T$ is a covariant completely symmetric tensor field of order 4, i.e.,

$$\begin{aligned} \nabla _k T_{lij} =\nabla _l T_{kij}, \end{aligned}$$

(3.5)

where $T_{kij}$ are local components of $T$.

First, for a conjugate symmetric statistical manifold ($M, g, T$), Theorem 3.1 implies the following corollary.

Corollary 3.2

Each linear connection ${\mathop {\nabla }\limits ^{\alpha }}$ of the one-parameter family of $\alpha$-connections on an $n$-dimensional conjugate symmetric statistical manifold $(M,g,T)$ is an equiaffine connection such that the volume form ${\mathop {\omega }\limits ^{\alpha }}$ associated with ${\mathop {\nabla }\limits ^{\alpha }}$ is defined by the equality ${\mathop {\omega }\limits ^{\alpha }} =\sqrt{\mathrm{det} \tilde{g}}$ for $\tilde{g}=\mathrm{exp}( {\frac{C+\alpha f}{n}}) g$, where C is an arbitrary constant.

Now let us consider a projectively flat equiaffine connection ${\mathop {\nabla }\limits ^{\alpha }}$ for a fixed number $\alpha$ on a conjugate symmetric statistical manifold ($M, g, T$). We recall that a torsion-free affine connection ${\mathop {\nabla }\limits ^{\alpha }}$ on manifold $M$ is called projectively flat if there exists a flat affine connection ${\nabla }^{\prime }$ on $M$ such that the pregeodesics (i.e., geodesics as curves without a distinguished parametrization) of ${\mathop {\nabla }\limits ^{\alpha }}$ and ${\nabla }^{\prime }$ coincide [35, pp. 138–142], [44, p. 386].

The $\alpha$-curvature tensor ${\mathop {R}\limits ^{\alpha }}$ of a projectively flat equiaffine connection ${\mathop {\nabla }\limits ^{\alpha }}$ has the form [35, pp. 138–142], [36, p. 169]

$$\begin{aligned} {\mathop {R}\limits ^{\alpha }}{_{jkl}^{i}} =\frac{1}{n-1} \,\left( {\delta _k^i {\mathop {R}\limits ^{\alpha }}_{jl} -\delta _l^i {\mathop {R}\limits ^{\alpha }}_{jk}}\right) . \end{aligned}$$

(3.6)

Therefore, using identities (2.6) and the definition of the Ricci tensor ${\mathop {Ric}\limits ^{\alpha }}$, from (3.6) we deduce ${\mathop {R}\limits ^{\alpha }}_{jk} =\frac{1}{n} {\mathop {s}\limits ^{\alpha }}\,g_{jk}$. On the other hand, the Ricci tensor ${\mathop {Ric}\limits ^{\alpha }}$ is a Codazzi tensor with respect to a projectively flat equiaffine connection ${\mathop {\nabla }\limits ^{\alpha }}$ [36, p. 169], i.e.,

$$\begin{aligned} {\mathop {\nabla }\limits ^{\alpha }}_{k} {\mathop {R}\limits ^{\alpha }}_{jl} = {\mathop {\nabla }\limits ^{\alpha }}_{j} {\mathop {R}\limits ^{\alpha }}_{lk}. \end{aligned}$$

(3.7)

Then substituting ${\mathop {R}\limits ^{\alpha }}_{jk} =\frac{1}{n} {\mathop {s}\limits ^{\alpha }} g_{jk}$ into (3.7) we obtain ${\mathop {s}\limits ^{\alpha }}=const$. Thus we have

$$\begin{aligned} {\mathop {R}\limits ^{\alpha }}{_{jkl}^{i}} =\frac{1}{n(n-1)} {\mathop {s}\limits ^{\alpha }} \,( {\delta _k^i g_{jl} -\delta _l^i g_{jk} }). \end{aligned}$$

In this case, we say that $({M,g,T})$ is a statistical manifold of constant $\alpha$-curvature. For the case $\alpha =1$ see [24, 29, 30].

It is well known that any Codazzi tensor $B$ of order 2 on a manifold $M$ with a projectively flat equiaffine connection ${\mathop {\nabla }\limits ^{\alpha }}$ has local components [36, p. 169]

$$\begin{aligned} {\mathop {B}\limits ^{\alpha }}_{ij} ={\mathop {\nabla }\limits ^{\alpha }} _i {\mathop {\nabla }\limits ^{\alpha }}_{j} f+\frac{f}{n-1} {\mathop {R}\limits ^{\alpha }}_{ij} \end{aligned}$$

for some smooth function $f$. On the other hand, we know that the metric tensor $g$ is a Codazzi tensor with respect to an arbitrary $\alpha$-connection ${\mathop {\nabla }\limits ^{\alpha }}$. Thus, we have

$$\begin{aligned} g_{ij} ={\mathop {\nabla }\limits ^{\alpha }}_i {\mathop {\nabla }\limits ^{\alpha }}_j f+\frac{f}{n( {n-1} )} {\mathop {s}\limits ^{\alpha }} \cdot g_{ij} \end{aligned}$$

for ${\mathop {s}\limits ^{\alpha }} ={\text{ const }}$. It follows that $g_{ij} =\frac{n(n-1)}{n(n-1)-{\mathop {s}\limits ^{\alpha }} \,\cdot f}\,{\mathop {\nabla }\limits ^{\alpha }}_{i} {\mathop {\nabla }\limits ^{\alpha }}_{j} f$. We have now proved the following theorem.

Theorem 3.3

Let ($M, g, T$) be a conjugate symmetric statistical manifold. If there exists a projectively flat $\alpha$-connection ${\mathop {\nabla }\limits ^{\alpha }}$ for some number $\alpha$, then $(M,g,T)$ is a statistical manifold of constant $\alpha$-curvature and its metric tensor g has local components $g_{ij} =\frac{n(n-1)}{n(n-1)-{\mathop {s}\limits ^{\alpha }} \,\cdot f}\,{\mathop {\nabla }\limits ^{\alpha }}_i {\mathop {\nabla }\limits ^{\alpha }}_{j}\, f$ for some smooth function f such that $({n(n-1)-{\mathop {s}\limits ^{\alpha }} \,\cdot f}) {\mathop {\nabla }\limits ^{\alpha }}_{i} {\mathop {\nabla }\limits ^{\alpha }}_{j}\,f$ is a positive definite symmetric 2-form on TM.

3.3 Compact statistical manifolds

First of all, we define a self-adjoint linear algebraic operator $\mathfrak R :S^{2}M\rightarrow S^{2}M$ acting on symmetric tensor fields of order 2 by the following identities:

$$\begin{aligned} \mathfrak R (B)_{ij} :=R_{kijl} B^{ij}. \end{aligned}$$

This operator is called the curvature operator or curvature operator of second kind [9, pp. 51–52], [26, 38, 40].

From the above definition, the curvature operator $\mathfrak R$ is a self-adjoint linear operator and hence all its eigenvalues are real numbers. This curvature operator is called negative or positive definite operator if its eigenvalues are negative or positive numbers, respectively.

It is well known [9, p. 45] that the vector space $S^{2} M$ of covariant symmetric tensor fields of order 2 is irreducible with respect to the action of the orthogonal group $O(n)$ at each point $x\in M$. We denote by $S_{0}^{2} M$ the vector space of traceless symmetric tensor fields of order 2 over ($M, g$). Then the following pointwise $O(n)$-irreducible decomposition holds: $S^{2}M=S_{0}^{2}M \oplus C^{\infty }M \cdot g$. We note that if the eigenvalues of $\mathfrak R$ restricted to $S_{0}^{2} M$ are $\lambda >0$, then the sectional curvatures at $x\in M$ are $\lambda >0$ [38, 40]. Thus, we say that $\mathfrak R$ is positive definite on $S_{0}^{2} M$, or simply $\mathfrak R \ge \lambda >0$, if all eigenvalues of $\mathfrak R$ restricted to $S_{0}^{2} M$ are positive. It should be noted that $\mathfrak R$ does not preserve the vector space $S_{0}^{2} M$ in general [9, p. 52]. Moreover, easy calculations give that ($M, g$) is Einstein (i.e., $Ric = n^{-1}\,s \cdot g$) if and only if $\mathfrak R$ maps $S_{0}^{2} M$ into itself at each point $x\in M$. In particular, $\mathfrak R$ is identical with $s/{n(n-1)}\times (the\,identity\,map)$ on $S_{0}^{2} \mathbf{S}^{n}$ for an $n$-dimensional Euclidean sphere $\mathbf{S}^{n}$ with standard metric $g_{0}$. Therefore, the curvature operator $\mathfrak R$ is positive on an $n$-sphere ($\mathbf{S}^{n}, g_{0}$).

Next we consider a compact conjugate symmetric statistical manifold ($M, g, T$) and show that there is a Weitzenböck formula for the skewness tensor $T$ of ($M, g, T$). The following lemma is simple computation.

Lemma 3.4

Let ($M, g, T$) be a compact oriented statistical manifold. Then the following integral formula holds:

$$\begin{aligned} \langle Ric(T),T\rangle +2\langle \mathfrak R (T),T \rangle +\frac{1}{12}\langle {\delta ^{{*}}T,\delta ^{{*}}T} \rangle -\frac{1}{3}\langle F,F \rangle -\langle {\delta T,\delta T}\rangle =0. \end{aligned}$$

(3.8)

Proof

Let $X$ be a vector field with local components $T_{jk}^l \nabla _l T^{ijk}-T_{jk}^i \nabla _l T^{ljk}$. For this vector field we have

$$\begin{aligned} \text{ div }X&= \nabla _i T_{jk}^l \nabla _l T^{ijk}+T_{jk}^l ( {\nabla _i \nabla _l T^{ijk}})-\nabla _i T_{jk}^i \nabla _l T^{ljk}-T_{jk}^i ( {\nabla _i \nabla _l T^{ljk}} )\nonumber \\&= R_{ij} T^{ikl}T_{kl}^j +2R_{ikjl} T^{ilm}T_m^{kj} +\nabla _i T^{jkl}\nabla _j T^{i}_{kl} -\nabla _i T^{ikl}\nabla _m T_{kl}^m\nonumber \\&= g( {Ric (T), T})+2g(\mathfrak R (T),T )\!+\!\frac{1}{12}g( {\delta ^{{*}}T,\delta ^{{*}}T}\! )\!-\!\frac{1}{3}g( {F,F})\!-\!g( {\delta T,\delta T}),\qquad \qquad \end{aligned}$$

(3.9)

where we used the Ricci identities [28, p. 145]

$$\begin{aligned} \nabla _i \nabla _j T^{ikl}-\nabla _j \nabla _i T^{ikl}=T^{mkl}R^{i}_{mij} +T^{iml}R^{k}_{mij} +T^{ikm}R^{l}_{mij}, \end{aligned}$$

(3.10)

and defined $g(Ric(T), T)$ and $g(\mathfrak R (T),T)$ by the equalities $g(Ric(T), T)=R_{ij} T^{ikl}T_{kl}^j$ and $g(\mathfrak R (T),T)=({R_{ikjl} T^{ilm}})\,T^{kj}_m$, respectively.

If we suppose that $M$ is a compact manifold, then applying Green’s theorem [28, p. 281] to (3.9) we obtain the integral formula (3.8). Here, we note that, to apply Green’s theorem, it is necessary to assume $M$ as orientable. If $M$ is not orientable, then we only need to consider an orientable double covering. The proof is complete. $\square$

Next, using Lemma 3.4 we prove the following “vanishing theorem”.

Theorem 3.5

Let ($M, g, T$) be a compact oriented statistical manifold. If the curvature operator $\mathfrak R$ is positive definite on $S_{0}^{2} M$, then ($M, g, T$) cannot be a conjugate symmetric statistical manifold with vanishing Tchebychev form. In particular, an $n$-dimensional ($n\ge 3$) Euclidean sphere ($\mathbf{S}^{n}, g_{0}$) cannot be a conjugate symmetric statistical manifold with vanishing Tchebychev form.

Proof

Let ($M, g, T$) be a conjugate symmetric statistical manifold then follows the equality $\delta ^{{*}}T=4F$, where $F$ is given by (2.5). On the other hand, from (3.5), we obtain $({\delta T})=-\nabla \tau$ for the Tchebychev form $\tau$ of ($M, g, T$). Therefore, if ($M, g, T$) is a compact conjugate symmetric statistical manifold with vanishing Tchebychev form $\tau$ then $T\in C^{\infty }S_0^3 M$ and we can rewrite (3.8) in the form

$$\begin{aligned} \langle Ric(T),T \rangle +2\langle \mathfrak R (T),T \rangle =-\langle F,F \rangle \le 0. \end{aligned}$$

(3.11)

If we suppose that the curvature operator $\mathfrak R$ is positive definite on $S_0^2 M$, i.e.,

$$\begin{aligned} R_{ijkl} B^{jk}B^{il}\ge \lambda B_{ij} B^{ij}>0 \end{aligned}$$

(3.12)

for an arbitrary symmetric tensor field $B=(B_{ij})$ which belongs to $S_0^2 M$ and some positive number $\lambda$, then

$$\begin{aligned} g(\mathfrak R (T),T)\ge \lambda \Vert T\Vert ^{2}>0. \end{aligned}$$

Denoting by $Y\circ X$ the symmetric tensor $X\circ Y:=1/2(X\otimes Y+Y\otimes X )$ for any local orthogonal unit vector fields $X$ and $Y$, we can represent (3.10) as $R_{ijkl} X^{i}Y^{j}X^{k}Y^{l}\ge \lambda >$ 0. This means that the sectional curvature $sec(X\wedge Y)\ge \lambda >0$ if the curvature operator $\mathfrak R \ge \lambda >0$ on $S_0^2 M$ [38, 40].

Next for the $n-1$ local unit vector fields $Y_1,\ldots ,Y_{n-1}$, orthogonal to $X$ and to each other, we have $sec (X\wedge Y_a )=R_{ijkl} Y_a^i X^{j}X^{k}Y_a^l \ge \lambda >0$ for all $a = 1,{\ldots }, n-1$, and hence $Ric\,(X,X)\ge (n-1)\lambda > 0$ [40]. Then we claim that

$$\begin{aligned} g(Ric(T), T)\ge (n-1) \lambda \Vert T\Vert ^2>0. \end{aligned}$$

So the left-hand side of (3.11) is positive, and this contradicts the inequality (3.11).

In particular, let ($M, g$) be a Riemannian manifold of constant sectional curvature then the Riemann curvature tensor has the form $R_{iljk} =K( {g_{ij} g_{lk} -g_{ik} g_{lj} })$. In this case (3.8) can be rewritten as

$$\begin{aligned} (n-3) K \langle {T, T}\rangle =-\langle {F,F}\rangle \le 0. \end{aligned}$$

(3.13)

For $n> 3, K> 0$ and $T\ne 0$ the left-hand side of (3.13) is positive that contradicts the inequality (3.13). In particular, for $n = 3$ from (3.13) we obtain $F = 0$. In this case, the Ricci identities (3.10) can be rewritten in the form $(n+1) K T_j^{kl} =0$ for $K> 0$ and $T\ne 0$, and this is a contradiction. This completes the proof of Theorem 3.5. $\square$

We shall now consider a harmonic statistical manifold ($M, g, T$) and show that the following theorem is true.

Theorem 3.6

Let ($M, g, T$) be a compact oriented statistical manifold. If the curvature operator $\mathfrak R$ is negative definite, then ($M, g, T$) cannot be a harmonic statistical manifold. In particular, an $n$-dimensional $(n\ge 3)$ compact hyperbolic manifold ($\mathbf{H}^{n}, g_{0})$ cannot be a harmonic statistical manifold.

Proof

Let ($M, g, T$) be a compact harmonic statistical manifold. In this case the formula (3.12) shows that

$$\begin{aligned} \langle Ric(T),T \rangle +2\langle \mathfrak R (T),T \rangle =\frac{11}{12}\langle {\delta ^{{*}}T,\delta ^{{*}}T} \rangle +\frac{1}{3}\langle {F,F} \rangle \ge 0, \end{aligned}$$

(3.14)

because $\langle {\Delta _{\mathrm{sym}} T,T} \rangle =\langle {\delta T,\delta T} \rangle -\langle {\delta ^{{*}}T,\delta ^{{*}}T} \rangle$. If we suppose that the curvature operator $\mathfrak R$ is negative definite, i.e.,

$$\begin{aligned} R_{ijkl} B^{jk}B^{il}\le -\mu \cdot B_{ij}B^{ij}<0 \end{aligned}$$

for an arbitrary symmetric tensor field $B=(B_{ij})$ and some positive number $\mu$, then $g(\mathfrak R (T),T)\le -\mu \cdot \Vert T\Vert ^{2}<0$ and hence $g(Ric(T), T)\le -(n-1) \mu \cdot \Vert T \Vert ^2<0$ [40]. In this case the left-hand side of (3.14) can be rewritten in the form

$$\begin{aligned} \langle Ric(T),T \rangle +2\langle \mathfrak R (T),T \rangle =-(n+1) \mu \langle {T, T} \rangle \le 0. \end{aligned}$$

So the left-hand side of (3.14) is negative that contradicts the inequality (3.14).

In particular, let ($M, g$) be a Riemannian manifold of constant sectional curvature [18, p. 84] then $R_{iljk} =K(g_{ij}g_{lk} -g_{ik} g_{lj})$. In this case (3.14) can be rewritten in the form

$$\begin{aligned} K((n-3)\,\langle {T, T} \rangle +2 \langle {\tau ,\tau } \rangle ) =\frac{11}{12}\langle {\delta ^{{*}}T,\delta ^{{*}}T}\rangle +\frac{1}{3}\langle {F,F}\rangle \ge 0. \end{aligned}$$

(3.15)

For $n > 3, K > 0$ and $T\ne 0$ the left-hand side of (3.15) is negative, and this contradicts the inequality (3.15). In particular, for $n = 3$ from (3.15) we obtain $F= 0$ and $\tau =0$. In this case the Ricci identities (3.8) can be rewritten in the form $(n+1) K T_j^{kl} =0$ for $K> 0$ and $T\ne 0$. As a result, we have a contradiction. This completes the proof of Theorem 3.5. $\square$

4 A five-dimensional Riemannian manifold with an irreducible SO(3)-structure

4.1 A conjugate symmetric statistical manifold with an irreducible SO(3)-structure

Let ($M, g, T$) be a five-dimensional statistical manifold with an irreducible SO(3)-structure, this means that the skewness tensor $T$ of ($M, g, T$) defines an irreducible SO(3)-structure on ($M, g$). This shows that the skewness tensor $T$ of ($M, g, T$) belongs to $S_0^3 M$ (i.e., the Tchebychev form $\tau$ of ($M, g, T$) is zero).

Consider a one-parameter group of affine torsion-free connections ${\mathop {\nabla }\limits ^{\alpha }}$ on ($M, g$) by Eq. (2.1). Then using (2.8) and (2.9) we get the equations

$$\begin{aligned} \nabla _l^{(\alpha )} T_{ijk}&= \nabla _l T_{ijk} + \frac{\alpha }{2}( {T_{ijm} T_{kl}^{m} +T_{imk} T_{jl}^{m} +T_{mjk} T_{il}^{\; m} })\nonumber \\&= \nabla _l T_{ijk} +\frac{\alpha }{2}( {g_{ij} g_{kl} +g_{ik} g_{jl} +g_{jk} g_{il} }). \end{aligned}$$

(4.1)

Then from Eq. (4.1), we obtain the following equality:

$$\begin{aligned} \Vert {\mathop {\nabla }\limits ^{\alpha }}T\Vert ^{2} =\Vert F\Vert ^{2}+\frac{105}{4} \alpha ^{2}, \end{aligned}$$

(4.2)

where $F$ is given by (2.5), $\Vert {{\mathop {\nabla }\limits ^{\alpha }} T} \Vert ^{2}=g( {{\mathop {\nabla }\limits ^{\alpha }} T, {\mathop {\nabla }\limits ^{\alpha }} T})$ and $\Vert F\Vert ^{2}=g(F,F)$. Then the tensor $T$ of an irreducible SO(3)-structure satisfies the following inequalities:

$$\begin{aligned} \Vert {{\mathop {\nabla }\limits ^{\alpha }} T}\Vert ^{2}\ge \Vert F\Vert ^{2}; \quad \Vert {\mathop {\nabla }\limits ^{\alpha }}T \Vert ^{2}\ge \frac{105}{4} \alpha ^{2}>0. \end{aligned}$$

(4.3)

On the other hand, from (3.2) we obtain the following identities:

$$\begin{aligned} {\mathop {R}\limits ^{\alpha }}_{jk} =R_{jk} -\frac{\alpha }{2}\nabla _m T_{jk} ^{m}-\frac{7\alpha ^{2}}{4}\cdot g_{jk}. \end{aligned}$$

(4.4)

In this case, ${\mathop {R}\limits ^{\alpha }}_{jk} ={\mathop {R}\limits ^{\alpha }}_{kj}$ and hence ${\mathop {\nabla }\limits ^{\alpha }}$ is an equiaffine connection for an arbitrary $\alpha$. Next from (4.4), we obtain ${\mathop {s}\limits ^{\alpha }} =s-{35}/4\cdot \alpha ^{2}$ and hence ${\mathop {s}\limits ^{\alpha }} \le s$ for the scalar $\alpha$-curvature ${\mathop {s}\limits ^{\alpha }}$ of ${\mathop {\nabla }\limits ^{\alpha }}$ and scalar curvature $s$ of $g$. The next theorem is an immediate consequence of Theorem 3.1 and its proof.

Theorem 4.1

Let a five-dimensional Riemannian manifold ($M, g$) with an irreducible SO(3)-structure be a statistical manifold ($M, g, T$), then the one-parameter family of affine $\alpha$-connections ${\mathop {\nabla }\limits ^{\alpha }}$ consisting of equiaffine connections and their volume forms ${\mathop {\omega }\limits ^{\alpha }}=\sqrt{\mathrm{det}({\mathrm{exp} C\cdot g})}$ do not depend on the parameter $\alpha$. Moreover, the scalar $\alpha$-curvature ${\mathop {s}\limits ^{\alpha }}$ of ${\mathop {\nabla }\limits ^{\alpha }}$ has the form ${\mathop {s}\limits ^{\alpha }} =s-{35}/4\cdot \alpha ^{2}$. $\square$

We consider a conjugate symmetric statistical manifold ($M, g, T$) such that the skewness tensor field $T$ defines an irreducible SO(3)-structure on ($M, g$). In this case, the skewness tensor field $T$ satisfies the following equations:

$$\begin{aligned} \nabla _k T_{lij} =\nabla _l T_{kij} ;\quad \nabla ^{k} T_{kij} =0, \end{aligned}$$

(4.5)

where $\nabla ^{k}=g^{kl}\nabla _l$. In view of what we have told above, we can formulate the following theorem.

Theorem 4.2

Let ($M, g, T$) be a five-dimensional statistical manifold with an irreducible SO(3)-structure and ${\mathop {\nabla }\limits ^{\alpha }}$ be a one-parameter family of affine $\alpha$-connections on M. Then ($M, g, T$) is conjugate symmetric if and only if ${\mathop {\nabla }\limits ^{\alpha }}$ T is a covariant completely symmetric tensor field of order 4. In particular, if ($M, g, T$) is a statistical manifold of constant $\alpha$-curvature, then it is a conjugate symmetric statistical manifold with Einstein metric g.

Proof

From (3.5), we obtain the following identities:

$$\begin{aligned} {\mathop {\nabla }\limits ^{\alpha }}_l T_{ijk} -{\mathop {\nabla }\limits ^{\alpha }}_i T_{ljk} =\nabla _l T_{ijk} -\nabla _i T_{ljk}. \end{aligned}$$

(4.6)

In turn, from (4.6) we conclude that a five-dimensional Riemannian manifold ($M, g$) with an irreducible SO(3)-structure is a conjugate symmetric statistical manifold if and only if the condition $\nabla ^{(\alpha )}T$ is a completely symmetric tensor field of order 4.

In addition, we suppose that ($M, g, T$) is a statistical manifold of constant $\alpha$-curvature for some $\alpha$ then from (2.3), (2.8) and the Ricci identities [18, p. 30]

$$\begin{aligned} {\mathop {\nabla }\limits ^{\alpha }}_k {\mathop {\nabla }\limits ^{\alpha }}_l g_{ij} - {\mathop {\nabla }\limits ^{\alpha }}_l {\mathop {\nabla }\limits ^{\alpha }}_k g_{ij} =-g_{im} {\mathop {R}\limits ^{\alpha }}{_{jlk}^{m}} -g_{mj} {\mathop {R}\limits ^{\alpha }}{_{ilk}^{m}}, \end{aligned}$$

(4.7)

we obtain (4.5). In addition, from (4.4) we have $R_{jk} =( {\frac{1}{5}{\mathop {s}\limits ^{\alpha }} +\frac{7}{8} \alpha ^{2}})\cdot g_{jk}$. This means that $g$ is an Einstein metric. The proof is complete. $\square$

Next we shall prove two “vanishing theorems” for conjugate symmetric statistical manifolds.

Theorem 4.3

Let ($M, g, T$) be a five-dimensional statistical manifold such that T be a structure tensor of an irreducible SO(3)-structure on ($M,g$). If the curvature operator $\mathfrak R \,(M, g)$ is positive definite on $S_0^2 M$, then ($M, g, T$) cannot be a (locally) conjugate symmetric. In particular, a five-dimensional Euclidian sphere ($\mathbf{S}^{5}, g_{0}$) cannot be a (locally) conjugate symmetric statistical manifold with an irreducible SO(3)-structure.

Proof

Let ($M, g, T$) be a conjugate symmetric statistical manifold. Then from the identity (2.8) by straightforward computation we get

$$\begin{aligned} 0&= \nabla _i \nabla _j (T^{ikl} T_{kl}^j )=(\nabla _i \nabla _j T^{ikl}) T_{kl}^j +({\nabla _j T^{ikl}}) ({\nabla _i T_{kl}^j })\nonumber \\&= ( {T^{mkl}R^{i}_{mij} \!+\!T^{iml}R^{k}_{mij} \!+\!T^{ikm}R^{l}_{mij} }) T_{kl}^j \!+\!\Vert F\Vert ^{2}\!=\!\frac{7}{2}s\!+\!2g( \mathfrak R (T), T)\!+\!\Vert F \Vert ^{2},\qquad \quad \end{aligned}$$

(4.8)

where $F$ is given by (2.5). If we suppose that the curvature operator $\mathfrak R$ is positive definite on $S_0^2 M$, then the scalar curvature $s$ of ($M, g$) is positive, too [40]. Moreover, in this case, we have $g(\mathfrak R (T),T)> 0$. Thus, we have the inequality $\frac{7}{2}s+2g(\mathfrak R (T),T)+\Vert F\Vert ^{2}>\text{0 }$ which contradicts to (4.8). The proof is complete. $\square$

The identities (3.1) for a conjugate symmetric statistical manifold ($M, g, T$) have the form

$$\begin{aligned} R_{ijkl} ={\mathop {R}\limits ^{\alpha }}_{ijkl} \,+\frac{\alpha ^{2}}{4}( { T_{mil} T_{jk} ^{m}-T_{mjl} T_{ik} ^{m}}), \end{aligned}$$

(4.9)

where ${\mathop {R}\limits ^{\alpha }}_{ijkl}:=g_{im} {\mathop {R}\limits ^{\alpha }}_{jkl}^m$. From (2.6) we conclude that symmetry properties of ${\mathop {R}\limits ^{\alpha }}_{ijkl}$ coincide with the symmetry properties of the Riemann curvature tensor $R_{ijkl}$. Therefore, we can define the $\alpha$-curvature operator ${\mathop {\mathfrak{R }}\limits ^{\alpha }}$ of an $\alpha$-connection ${\mathop {\nabla }\limits ^{\alpha }}$ as an analog of the curvature operator $\mathfrak R$ of ($M, g$). Then using (2.8), (2.9) and (2.10) from (4.9) we get $g(\mathfrak R (T),T) =g(\mathfrak{R }{\mathop {(T)}\limits ^{\alpha }},T)+\frac{595}{16}\alpha ^{2}$. If we suppose that there is an $\alpha$-connection ${\mathop {\nabla }\limits ^{\alpha }}$ such that its curvature operator ${\mathop {\mathfrak{R }}\limits ^{\alpha }}$ is positive semi-definite then $g(\mathfrak R (T),T)\ge \frac{595}{16}\alpha ^{2}>\text{0 }$. Thus, as a consequence of Theorem 4.3, we have the following corollary.

Corollary 4.4

Let ($M, g, T$) be a five-dimensional Riemannian manifold ($M, g$) with an irreducible SO(3)-structure. If there exists an $\alpha$-connection ${\mathop {\nabla }\limits ^{\alpha }}$ such that its curvature operator ${\mathop {\mathfrak{R }}\limits ^{\alpha }}$ is positive semi-definite then ($M, g, T$) is not conjugate symmetric.

Finally, we present topological properties of a five-dimensional conjugate symmetric statistical manifold with an irreducible SO(3)-structure. Namely, we have the following Theorem.

Theorem 4.5

Let ($M, g, T$) be a conjugate symmetric statistical manifold such that ($M, g$) is a five-dimensional compact, conformally flat Riemannian manifold and T is a structure tensor of an irreducible SO(3)-structure on ($M, g$). Then the fundamental group $\pi _1 (M)$ has exponential growth unless $F\equiv 0$. In particular, if $\pi _1(M)$ is almost solvable, then ($M, g$) is a flat manifold. Moreover, if F does not vanish anywhere on ($M, g$) then $\pi _1(M)$ is a non-amenable group.

Proof

The Riemannian curvature tensor $R$ of a five-dimensional conformal flat Riemannian manifold ($M, g$) has the form [18, p. 92]

$$\begin{aligned} R_{ijkl} =\frac{1}{3}( {g_{ik} R{ }_{lj}-g_{il} R_{jk} +g_{lj} R_{ik} -g_{jk} R_{il} })+\frac{s}{12}( {g_{jl} g_{ik} -g_{jk} g_{li} }). \end{aligned}$$

In this case from (4.8) we obtain $s=-\frac{4}{21}\Vert F\Vert ^{2}\le 0$, where $F$ is given by (2.5).

We know three things: First, if ($M, g$) is compact, conformally flat with non-positive scalar curvature $s$, then the fundamental group $\pi _1(M)$ has exponential growth unless $s\equiv 0$ [31]. This proposition gives the first result. Second, if the fundamental group $\pi _1 (M)$ of a compact conformal flat Riemannian manifold ($M, g$) with non-positive scalar curvature is almost solvable, then ($M, g$) is a flat manifold [31]. This proposition gives the second result. Third, the fundamental group $\pi _1(M)$ of a compact, locally conformally flat Riemannian manifold ($M, g$) with negative scalar curvature is a non-amenable group [46]. That gives the third result of the theorem. $\square$

4.2 Manifolds with nearly integrable SO(3)-structures

In this section, we consider a five-dimensional Riemannian manifold ($M, g$) with a nearly integrable SO(3)-structure as an example of a harmonic statistical manifold. In this case, we have the following theorem.

Theorem 4.6

A five-dimensional Riemannian manifold with a nearly integrable SO(3)-structure is a harmonic statistical manifold such that its skewness tensor defines the first integral of order 3 of the equations of geodesics.

Proof

Let $T$ be the tensor of a nearly integrable SO(3)-structure on a five-dimensional Riemannian manifold ($M, g$). If the equation $\delta ^{{*}}T= 0$ holds, then necessarily $\delta T= 0$ as is readily seen, and so $\Delta _{\mathrm{sym}} T :=\delta ^{{*}}\delta T-\delta \delta ^{{*}}T=0$, where $\Delta _{\mathrm{sym}}$ is a Laplacian on symmetric covariant tensor fields [9, pp. 52–54], [45]. In this case, the tensor field $T$ is harmonic [45] and hence, ($M,g,T$) is a harmonic statistical manifold.

On the other hand, a 1-dimensional immersed submanifold $\gamma$ of ($M, g$) is said to be a geodesic if there exists a parameterization $\gamma :x^{i}=x^{i}(t)$ for $t\in I\subset \mathbf{R}$ satisfying $\nabla _{\dot{x}} \dot{x}=0$. If each solution $x^{i}=x^{i}(t)$ of the equations $\nabla _{\dot{x}} \dot{x}=0$ of geodesics satisfies the condition $B({\dot{x},\ldots ,\dot{x}})={\text{ const }}$ for smooth covariant completely symmetric tensor field $B$ of order $p$ and $\dot{x}=\frac{d x^{k}}{d t}\cdot X_k$, then the equations $\nabla _{\dot{x}} \dot{x}=0$ are said to admit the first integral of the p-th order. The equation $\delta ^{{*}}B=0$ serves as a necessary and sufficient condition for this [18, pp. 128–129].

It is easy to check that a five-dimensional statistical manifold ($M, g, T$) whose skewness tensor $T$ is a structure tensor of a nearly integrable irreducible SO(3)-structure on ($M, g$) admits the first integral of order 3 of geodesics in the form $T(\dot{x},\dot{x},\dot{x})={\text{ const }}$. Thus, the theorem is valid. $\square$

Remark

For a harmonic symmetric tensor, it was said in [45] that it does not have any geometrical interpretation. It was also pointed out that symmetric tensors, whose covariant derivative vanishes, are clearly harmonic, the metric tensor $g$ being the most important example. These tensors are trivial examples of a harmonic symmetric tensor. Now we can say that the tensor $T$ of a nearly integrable SO(3)-structure on a five-dimensional connected Riemannian manifold ($M, g$) is a non-trivial example of a harmonic symmetric tensor.

Next, we prove a “vanishing theorems” for nearly integrable SO(3)-structures.

Theorem 4.7

Let the triplet ($M, g, T$) be a statistical manifold, where ($M, g$) be a five-dimensional Riemannian manifold and T be a structure tensor of an irreducible SO(3)-structure on ($M, g$). If the curvature operator $\mathfrak R$ of ($M, g$) is negative definite, then SO(3)-structure cannot be nearly integrable. In particular, there is no a nearly integrable irreducible SO(3)-structure on a five-dimensional hyperbolic manifold ($\mathbf{H}^{5}, g_{0})$.

Proof

We consider a five-dimensional statistical manifold ($M, g, T$) such that its skewness tensor $T$ defines a nearly integrable irreducible SO(3)-structure on ($M, g$). In this case, from the identity (2.8) by straightforward computation, we get

$$\begin{aligned} 0&= \nabla _i \nabla _j (T^{ikl} T_{kl}^j)=(\nabla _i \nabla _j T^{ikl}) T_{kl}^j +({\nabla _j T^{ikl}}) (\nabla _i T_{kl}^j)\nonumber \\&= ( {T^{mkl}R^{i}_{mij} +T^{iml}R^{k}_{mij} +T^{ikm}R^{l}_{mij} }) T_{kl}^j -\frac{1}{3}\Vert F\Vert ^{2}\nonumber \\&= \frac{7}{2}s+2g(\mathfrak R (T), T)-\frac{1}{3}\Vert F\Vert ^{2}. \end{aligned}$$

(4.10)

If we suppose that the curvature operator of second kind $\mathfrak R$ is negative definite, then $g(\mathfrak R (T),T) < 0$ and the scalar curvature $s$ must be negative, too. The above conditions give rise to a contradiction with the formula (4.10).

In particular, if we consider a Riemannian manifold ($M, g$) of constant curvature $K$, then from the formula (4.10) we can obtain $K\ge 0$. Hence there is no a nearly integrable irreducible SO(3)-structure on a five-dimensional hyperbolic manifold $(\mathbf{H}^{5}, g_{0})$ [55]. This completes the proof of the theorem. $\square$

Finally, we consider a topological property of a five-dimensional conformally flat ($M, g$) with a nearly integrable irreducible SO(3)-structure. Namely, we have the following corollary.

Corollary 4.9

Let ($M, g$) be a compact, locally conformally flat Riemannian manifold with a nearly integrable irreducible SO(3)-structure whose structure tensor T has a non-vanishing covariant derivative $\nabla T$ on M. Then the fundamental group $\pi _1(M)$ is hyperbolic in the sense of Gromov.

Proof

For a locally conformal flat Riemannian manifold ($M, g$) from (4.10), we obtain

$$\begin{aligned} s=\frac{4}{63}\Vert F\Vert ^{2}\ge 0. \end{aligned}$$

As a consequence of main theorem [25], we have that the fundamental group $\pi _1(M)$ of a compact, conformally flat manifold ($M, g$) with positive scalar curvature is hyperbolic in the sense of Gromov. This proposition gives our result. In addition, we say that there are other applications to geometry and topology of locally conformally flat manifolds with positive scalar curvature, and hence to geometry and topology of locally conformally flat Riemannian manifold with a nearly integrable irreducible SO(3)-structure [25]. In particular, this is a “vanishing theorem” for cohomology groups of such manifolds. $\square$