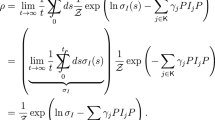Skip to main content
SpringerLink
Log in

An Ergodic Decomposition Defined by Transition Probabilities

  • Published:
Acta Applicandae Mathematicae Aims and scope Submit manuscript

Abstract

Our main goal in this paper is to prove that any transition probability P on a locally compact separable metric space (X,d) defines a Kryloff-Bogoliouboff-Beboutoff-Yosida (KBBY) ergodic decomposition of the state space (X,d). Our results extend and strengthen the results of Chap. 5 of Hernández-Lerma and Lasserre (Markov Chains and Invariant Probabilities, [2003]) and extend our KBBY-decomposition for Markov-Feller operators that we have obtained in Chap. 2 of our monograph (Zaharopol in Invariant Probabilities of Markov-Feller Operators and Their Supports, [2005]). In order to deal with the decomposition that we present in this paper, we had to overcome the fact that the Lasota-Yorke lemma (Theorem 1.2.4 in our book (op. cit.)) and two results of Lasota and Myjak (Proposition 1.1.7 and Corollary 1.1.8 of our work (op. cit.)) are no longer true in general in the non-Feller case.

In the paper, we also obtain a “formula” for the supports of elementary measures of a fairly general type. The result is new even for Markov-Feller operators.

We conclude the paper with an outline of the KBBY decomposition for a fairly large class of transition functions. The results for transition functions and transition probabilities seem to us surprisingly similar. However, as expected, the arguments needed to prove the results for transition functions are significantly more involved and are not presented here. We plan to discuss the KBBY decomposition for transition functions with full details in a small monograph that we are currently trying to write.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Barnsley, M.F.: Iterated function systems for lossless data compression. In: Barnsley, M.F., Saupe, D., Vrscay, E.R. (eds.) Fractals in Multimedia, pp. 33–63. Springer, New York (2002)

    Google Scholar 

  2. Barnsley, M.F., Demko, S.: Iterated function systems and the global construction of fractals. Proc. R. Soc. Lond. A 399, 243–275 (1985)

    MATH  MathSciNet  Google Scholar 

  3. Barnsley, M.F., Demko, S.G., Elton, J.H., Geronimo, J.S.: Invariant measures for Markov processes arising from iterated function systems with place-dependent probabilities. Ann. Inst. Henri Poincaré, Probab. Stat. 24, 367–394 (1988). Erratum: 25, 589–590 (1989)

    MATH  MathSciNet  Google Scholar 

  4. Barnsley, M.F., Elton, J.H.: A new class of Markov processes for image encoding. Adv. Appl. Prob. 20, 14–32 (1988)

    Article  MATH  MathSciNet  Google Scholar 

  5. Beboutoff, M.: Markov chains with a compact state space. Rec. Math. (Mat. Sbornik) N.S. 10(52), 213–238 (1942)

    MathSciNet  Google Scholar 

  6. Centore, P.M., Vrscay, E.R.: Continuity of attractors and invariant measures for iterated function systems. Can. Math. Bull. 37, 315–329 (1994)

    MATH  MathSciNet  Google Scholar 

  7. Cohn, D.L.: Measure Theory. Birkhäuser, Boston (1980)

    MATH  Google Scholar 

  8. Cornfeld, I.P., Fomin, S.V., Sinai, Y.G.: Ergodic Theory. Springer, Berlin (1982)

    MATH  Google Scholar 

  9. Costa, O.L.V., Dufour, F.: Invariant probability measures for a class of Feller Markov chains. Stat. Probab. Lett. 50, 13–21 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  10. Costa, O.L.V., Dufour, F.: Necessary and sufficient conditions for nonsingular invariant probability measures for Feller Markov chains. Stat. Probab. Lett. 53, 47–57 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  11. Costa, O.L.V., Dufour, F.: On the ergodic decomposition for a class of Markov chains. Stoch. Process. Appl. 115, 401–415 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  12. Edalat, A.: Power domains and iterated function systems. Inf. Comput. 124, 182–197 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  13. Hernández-Lerma, O., Lasserre, J.B.: Ergodic theorems and ergodic decomposition for Markov chains. Acta Appl. Math. 54, 99–119 (1998)

    Article  MATH  MathSciNet  Google Scholar 

  14. Hernández-Lerma, O., Lasserre, J.B.: Markov Chains and Invariant Probabilities. Birkhäuser, Basel (2003)

    MATH  Google Scholar 

  15. Heyer, H.: Probability Measures on Locally Compact Groups. Springer, Berlin (1977)

    MATH  Google Scholar 

  16. Iosifescu, M., Grigorescu, S.: Dependence with Complete Connections and Its Applications. Cambridge University Press, Cambridge (1990)

    MATH  Google Scholar 

  17. Iosifescu, M., Theodorescu, R.: Random Processes and Learning. Springer, New York (1969)

    MATH  Google Scholar 

  18. Jaroszewska, J.: Iterated function systems with continuous place dependent probabilities. Univ. Iagell. Acta Math., Fasc. 40, 137–146 (2002)

    MathSciNet  Google Scholar 

  19. Komorowski, T., Peszat, S., Szarek, T.: On ergodicity of some Markov processes (2008, submitted)

  20. Kryloff, N., Bogoliouboff, N.: La théorie générale de la mesure dans son application à l’étude des systèmes de la mécanique non linéaires. Ann. Math. 38, 65–113 (1937)

    Article  MathSciNet  Google Scholar 

  21. Lasota, A., Mackey, M.C.: Stochastic perturbation of dynamical systems: the weak convergence of measures. J. Math. Anal. Appl. 138, 232–248 (1989)

    Article  MATH  MathSciNet  Google Scholar 

  22. Lasota, A., Myjak, J.: Semifractals. Bull. Pol. Acad. Sci. Math. 44, 5–21 (1996)

    MATH  MathSciNet  Google Scholar 

  23. Lasota, A., Myjak, J.: Markov operators and fractals. Bull. Pol. Acad. Sci. Math. 45, 197–210 (1997)

    MATH  MathSciNet  Google Scholar 

  24. Lasota, A., Myjak, J.: Semifractals on Polish spaces. Bull. Pol. Acad. Sci. Math. 46, 179–196 (1998)

    MATH  MathSciNet  Google Scholar 

  25. Lasota, A., Myjak, J.: Fractals, semifractals, and Markov operators. Int. J. Bifurc. Chaos 9, 307–325 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  26. Lasota, A., Myjak, J.: Attractors of multifunctions. Bull. Pol. Acad. Sci. Math. 48, 319–334 (2000)

    MATH  MathSciNet  Google Scholar 

  27. Lasota, A., Myjak, J.: On a dimension of measures. Bull. Pol. Acad. Sci. Math. 50, 221–235 (2002)

    MATH  MathSciNet  Google Scholar 

  28. Lasota, A., Myjak, J., Szarek, T.: Markov operators with a unique invariant measure. J. Math. Anal. Appl. 276, 343–356 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  29. Lasota, A., Yorke, J.A.: Lower bound technique for Markov operators and iterated function systems. Random Comput. Dyn. 2, 41–77 (1994)

    MATH  MathSciNet  Google Scholar 

  30. Liggett, T.M.: Interacting Particle Systems. Springer, New York (1985)

    MATH  Google Scholar 

  31. Liggett, T.M.: Stochastic Interacting Systems: Contact, Voter and Exclusion Processes. Springer, Berlin (1999)

    MATH  Google Scholar 

  32. Lin, M.: Conservative Markov processes on a topological space. Isr. J. Math. 8, 165–186 (1970)

    Article  MATH  Google Scholar 

  33. de Melo, W., van Strien, S.: One-Dimensional Dynamics. Springer, Berlin (1993)

    MATH  Google Scholar 

  34. Morris, D.W.: Ratner’s Theorems on Unipotent Flows. University of Chicago Press, Chicago (2005)

    MATH  Google Scholar 

  35. Myjak, J., Szarek, T.: On Hausdorff dimension of invariant measures arising from non-contractive iterated function systems. Ann. Mat. 181, 223–237 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  36. Nicol, M., Sidorov, N., Broomhead, D.: On the fine structure of stationary measures in systems which contract-on-average. J. Theor. Probab. 15, 715–730 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  37. Norman, F.: Markov Processes and Learning Models. Academic Press, San Diego (1972)

    MATH  Google Scholar 

  38. Onicescu, O., Mihoc, G.: Sur les chaines de variables statistiques. Bull. Sci. Math. 59, 174–192 (1935)

    MATH  Google Scholar 

  39. Revuz, D.: Markov Chains. North-Holland, Amsterdam (1975)

    MATH  Google Scholar 

  40. Robinson, C.: Dynamical Systems—Stability, Symbolic Dynamics, and Chaos. CRC Press, Boca Raton (1995)

    MATH  Google Scholar 

  41. Stenflo, Ö.: A note on a theorem of Karlin. Stat. Probab. Lett. 54, 183–187 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  42. Stenflo, Ö.: Ergodic theorems for time-dependent random iteration of functions. In: Fractals and Beyond, Valletta, 1998, pp. 129–136. World Scientific, River Edge (1998)

    Google Scholar 

  43. Stenflo, Ö.: Uniqueness of invariant measures for place-dependent random iterations of functions. In: Barnsley, M.F., Saupe, D., Vrscay, E.R. (eds.) Fractals in Multimedia, pp. 13–32. Springer, New York (2002)

    Google Scholar 

  44. Stenflo, Ö.: Markov chains in random environments and random iterated function systems. Trans. Am. Math. Soc. 353, 3547–3562 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  45. Stenflo, Ö.: Uniqueness in g-measures. Nonlinearity 16, 403–410 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  46. Szarek, T.: Invariant measures for Markov operators with application to function systems. Stud. Math. 154, 207–222 (2003)

    MATH  MathSciNet  Google Scholar 

  47. Szarek, T.: Invariant Measures for Nonexpansive Markov Operators on Polish Spaces. Diss. Math. 425 (2003)

  48. Vrscay, E.R.: From fractal image compression to fractal-based methods in mathematics. In: Barnsley, M.F., Saupe, D., Vrscay, E.R. (eds.) Fractals in Multimedia, pp. 65–106. Springer, New York (2002)

    Google Scholar 

  49. Yosida, K.: Markov Processes with a stable distribution. Proc. Imp. Acad. Tokyo 16, 43–48 (1940)

    Article  MATH  MathSciNet  Google Scholar 

  50. Yosida, K.: Simple Markoff process with a locally compact phase space. Math. Jpn. 1, 99–103 (1948)

    MATH  MathSciNet  Google Scholar 

  51. Yosida, K.: Functional Analysis, 3rd edn. Springer, New York (1971)

    MATH  Google Scholar 

  52. Zaharopol, R.: Invariant Probabilities of Markov-Feller Operators and Their Supports. Birkhäuser, Basel (2005)

    MATH  Google Scholar 

  53. Zaharopol, R.: Invariant probabilities of convolution operators. Rev. Roum. Math. Pures Appl. 50, 387–405 (2005)

    MATH  MathSciNet  Google Scholar 

  54. Zaharopol, R.: Equicontinuity and the existence of attractive probability measures for some iterated function systems. Rev. Roum. Math. Pures Appl. 52, 259–286 (2007)

    MATH  MathSciNet  Google Scholar 

  55. Zaharopol, R.: Iterated function systems generated by strict contractions and place-dependent probabilities. Bull. Pol. Acad. Sci., Math. 48, 429–438 (2000)

    MATH  MathSciNet  Google Scholar 

  56. Zaharopol, R.: Fortet-Mourier norms associated with some iterated function systems. Stat. Probab. Lett. 50, 149–154 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  57. Zaharopol, R.: Attractive probability measures and their supports. Rev. Roum. Math. Pures Appl. 49, 397–418 (2004)

    MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Mathematical Reviews, 416 Fourth Street, Ann Arbor, MI, 48103, USA

    Radu Zaharopol

Authors
  1. Radu Zaharopol
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Radu Zaharopol.

Additional information

I am indebted to Sean Meyn for a discussion that we had in November 2004, which helped me to significantly improve the exposition in this paper, and to two anonymous referees for useful recommendations.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Zaharopol, R. An Ergodic Decomposition Defined by Transition Probabilities. Acta Appl Math 104, 47–81 (2008). https://doi.org/10.1007/s10440-008-9240-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10440-008-9240-4

Keywords

Mathematics Subject Classification (2000)

Search

Navigation