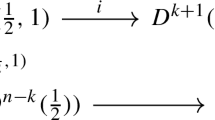Skip to main content
SpringerLink
Log in

Twists and Gromov hyperbolicity of riemann surfaces

  • Published:
Acta Mathematica Sinica, English Series Aims and scope Submit manuscript

Abstract

The main aim of this paper is to study whether the Gromov hyperbolicity is preserved under some transformations on Riemann surfaces (with their Poincaré metrics). We prove that quasiconformal maps between Riemann surfaces preserve hyperbolicity; however, we also show that arbitrary twists along simple closed geodesics do not preserve it, in general.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Gromov, M.: Hyperbolic Groups, Essays in Group Theory, Math. Sci. Res. Inst. Publ., 8, Springer, New York, 1987, 75–263

    Google Scholar 

  2. Bonk, M., Schramm, O.: Embeddings of Gromov hyperbolic spaces. Geom. Funct. Anal., 10(2), 266–306 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  3. Foertsch, T., Schroeder, V.: A product construction for hyperbolic metric spaces. Illinois J. Math., 49(3), 793–810 (2005)

    MATH  MathSciNet  Google Scholar 

  4. Naor, A., Peres, Y., Schramm, O., et al.: Markov chains in smooth Banach spaces and Gromov-hyperbolic metric spaces. Duke Math. J., 134(1), 165–197 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  5. Wenger, S.: Gromov hyperbolic spaces and the sharp isoperimetric constant. Invent. Math., 171(1), 227–255 (2008)

    Article  MATH  MathSciNet  Google Scholar 

  6. Balogh, Z. M., Buckley, S. M.: Geometric characterizations of Gromov hyperbolicity. Invent. Math., 153, 261–301 (2003)

    Article  MATH  MathSciNet  Google Scholar 

  7. Benoist, Y.: Convexes hyperboliques et fonctions quasisymétriques. Publ. Math. Inst. Hautes Études Sci., 97, 181–237 (2003)

    MATH  MathSciNet  Google Scholar 

  8. Bonk, M., Heinonen, J., Koskela, P.: Uniformizing Gromov hyperbolic spaces, Astérisque 270, 2001

  9. Breda, A. M., Sigarreta, J. M.: Symmetry and transitive properties of monohedral f-triangulations of the Riemannian sphere. Acta Mathematica Sinica, English Series, 25, 1609–1616 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  10. Hästö, P. A.: Gromov hyperbolicity of the j G and \( \tilde jG \) metrics. Proc. Amer. Math. Soc., 134, 1137–1142 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  11. Hästö, P. A., Lindén, H., Portilla, A., et al.: Gromov hyperbolicity of Denjoy domains with hyperbolic and quasihyperbolic metrics. J. Math. Soc. Japan, to appear

  12. Hästö, P. A., Portilla, A., Rodríguez, J. M., et al.: Gromov hyperbolic equivalence of the hyperbolic and quasihyperbolic metrics in Denjoy domains. Bull. London Math. Soc., 42(2), 282–294 (2010)

    Article  MATH  Google Scholar 

  13. Hästö, P. A., Portilla, A., Rodríguez, J. M., et al.: Uniformly separated sets and Gromov hyperbolicity of domains with the quasihyperbolicity metric. Medit. J. Math., DOI: 10.1007/s00009-010-0059-7

  14. Hästö, P. A., Portilla, A., Rodríguez, J. M., et al.: Comparative Gromov hyperbolicity results for the hyperbolic and quasiyperbolic metrics. Complex Var. Elliptic Equ., 55, 127–135 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  15. Karlsson, A., Noskov, G. A.: The Hilbert metric and Gromov hyperbolicity. Enseign. Math., 48, 73–89 (2002)

    MATH  MathSciNet  Google Scholar 

  16. Lindén, H.: Gromov hyperbolicity of certain conformal invariant metrics. Ann. Acad. Sci. Fenn. Math. 32(1), 279–288 (2007)

    MATH  MathSciNet  Google Scholar 

  17. Sun, M. F., Shen, Y. L.: On holomorphic sections in Teichmuller spaces. Acta Math. Sinica, English Series, 25, 2023–2034 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  18. Alvarez, V., Portilla, A., Rodríguez, J. M., et al.: Gromov hyperbolicity of Denjoy domains. Geom. Dedicata, 121, 221–245 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  19. Portilla, A., Rodríguez, J. M., Tourís, E.: Gromov hyperbolicity through decomposition of metric spaces II. J. Geom. Anal., 14, 123–149 (2004)

    MATH  MathSciNet  Google Scholar 

  20. Portilla, A., Rodríguez, J. M., Tourís, E.: The topology of balls and Gromov hyperbolicity of Riemann surfaces. Diff. Geom. Appl., 21, 317–335 (2004)

    Article  MATH  Google Scholar 

  21. Portilla, A., Rodríguez, J. M., Tourís E.: The role of funnels and punctures in the Gromov hyperbolicity of Riemann surfaces. Proc. Edinb. Math. Soc., 49, 399–425 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  22. Portilla, A., Rodríguez, J. M., Tourís, E.: A real variable characterization of Gromov hyperbolicity of flute surfaces. Osaka J. Math., to appear

  23. Portilla, A., Rodríguez, J. M., Tourís, E.: Structure Theorem for Riemannian surfaces with arbitrary curvature. Preprint

  24. Portilla, A., Tourís, E.: A characterization of Gromov hyperbolicity of surfaces with variable negative curvature. Publ. Mat., 53, 83–110 (2009)

    MATH  MathSciNet  Google Scholar 

  25. Rodríguez, J. M., Tourís, E.: Gromov hyperbolicity through decomposition of metric spaces. Acta Math. Hung., 103, 53–84 (2004)

    Article  Google Scholar 

  26. Rodríguez, J. M., Tourís, E.: A new characterization of Gromov hyperbolicity for Riemann surfaces. Publ. Mat., 50, 249–278 (2006)

    MATH  MathSciNet  Google Scholar 

  27. Rodríguez, J. M., Tourís, E.: Gromov hyperbolicity of Riemann surfaces. Acta Mathematica Sinica, English Series, 23, 209–228 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  28. Tourís, E.: Graphs and Gromov hyperbolicity of non-constant negatively curved surfaces. J. Math. Anal. Appl., to appear

  29. Ghys, E., de la Harpe, P.: Sur les Groupes Hyperboliques d’après Mikhael Gromov, Progress in Mathematics, Volume 83, Birkhäuser, 1990

  30. Basmajian, A.: Hyperbolic structures for surfaces of infinite type. Trans. Amer. Math. Soc., 336, 421–444 (1993)

    Article  MATH  MathSciNet  Google Scholar 

  31. Haas, A.: Dirichlet points, Garnett points and infinite ends of hyperbolic surfaces I. Ann. Acad. Sci. Fenn. Series AI, 21, 3–29 (1996)

    MATH  MathSciNet  Google Scholar 

  32. Haas, A., Susskind, P.: The geometry at infinity of a hyperbolic Riemann surface of infinite type. Geom. Dedicata, 130, 1–24 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  33. Buser, P.: Geometry and Spectra of Compact Riemann Surfaces, Birkhäuser, Boston, 1992

    MATH  Google Scholar 

  34. Chavel, I.: Eigenvalues in Riemannian Geometry, Academic Press, New York, 1984

    MATH  Google Scholar 

  35. Alvarez, V., Rodríguez, J. M.: Structure theorems for Riemann and topological surfaces. J. London Math. Soc., 69, 153–168 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  36. Douady, A., Earle, C.: Conformally natural extension of homeomorphisms of the circle. Acta Math., 157, 23–48 (1986)

    Article  MATH  MathSciNet  Google Scholar 

  37. Matsuzaki, K.: Quasiconformal mapping class groups having common fixed points on the asymptotic Teichm üller spaces. J. d’Analyse Math., 102, 1–28 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  38. Anderson, J. W.: Hyperbolic Geometry, Springer, London, 1999

    MATH  Google Scholar 

  39. Bers, L.: An Inequality for Riemann Surfaces. Differential Geometry and Complex Analysis, H. E. Rauch Memorial Volume, Springer-Verlag, 1985

  40. Beardon, A. F.: The geometry of discrete groups, Springer-Verlag, New York, 1983

    MATH  Google Scholar 

  41. Matsuzaki, K.: A countable Teichmüller modular group. Trans. Amer. Math. Soc., 357, 3119–3131 (2005)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, School of Education, Waseda University, Shinjuku, Tokyo, 169-8050, Japan

    Katsuhiko Matsuzaki

  2. Departamento de Matemáticas, Universidad Carlos III de Madrid, Avenida de la Universidad 30, 28911, Leganés, Madrid, Spain

    José M. Rodríguez

Authors
  1. Katsuhiko Matsuzaki
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. José M. Rodríguez
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Katsuhiko Matsuzaki.

Additional information

The second author is supported in part by two grants from Ministerio de Ciencia e Innovación (MTM 2009-07800 and MTM 2008-02829-E), Spain

Rights and permissions

Reprints and permissions

About this article

Cite this article

Matsuzaki, K., Rodríguez, J.M. Twists and Gromov hyperbolicity of riemann surfaces. Acta. Math. Sin.-English Ser. 27, 29–44 (2011). https://doi.org/10.1007/s10114-011-9693-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10114-011-9693-7

Keywords

MR(2000) Subject Classification

Search

Navigation