Abstract
In this paper, we consider the semi-continuous knapsack problem with generalized upper bound constraints on binary variables. We prove that generalized flow cover inequalities are valid in this setting and, under mild assumptions, are facet-defining inequalities for the entire problem. We then focus on simultaneous lifting of pairs of variables. The associated lifting problem naturally induces multidimensional lifting functions, and we prove that a simple relaxation in a restricted domain is a superadditive function. Furthermore, we also prove that this approximation is, under extra requirements, the optimal lifting function. We then analyze the separation problem in two phases. First, finding a seed inequality, and second, select the inequality to be added. In the first step we evaluate both exact and heuristic methods. The second step is necessary because the proposed lifting procedure is simultaneous; from where our class of lifted inequalities might contain an exponential number of these. We choose a strategy of maximizing the resulting violation. Finally, we test this class of inequalities using instances arising from electrical planning problems. Our tests show that the proposed class of inequalities is strong in the sense that the addition of these inequalities closes, on average, 57.70 % of the root integrality gap and 97.70 % of the relative gap while adding less than three cuts on average.




Similar content being viewed by others
Notes
A sufficient condition is that \(m_k\ge {\varGamma }\) for the two smallest \(\xi _k\) coefficients in \(C\).
i.e. the gap between the linear programming optimal value (with cuts and without the cuts) and the integer programming optimal solution value.
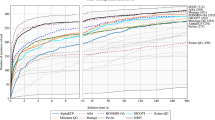
For Figs. 2a, and 3b each point \((x,y)\) of the plotted curves means that for the worst \(x\%\) of the instances, the given method closes at most \(y\%\) of absolute root integrality gap (left). For Figs. 2 and 3 each point \((x,y)\) of the plotted curves means that for the worst \(x\%\) of the instances, the given method concludes with a lower bound of \(y\%\) or less of the actual integer optimal solution value (right).
References
Aarts, E., Lenstra, J.K. (eds.): Local Search in Combinatorial Optimization, 1st edn. Wiley, New York (1997)
Atamtürk, A.: Sequence independent lifting for mixed-integer programming. Oper. Res. 52(3), 487–490 (2004)
Balas, E.: Facets of the knapsack polytope. Math. Program. 8, 146–164 (1975)
Balas, E., Jeroslow, R.: Canonical cuts on the unit hypercube. Math. Program. 23, 61–69 (1972)
Balas, E., Zemel, E.: Facets of the knapsack polytope from minimal covers. SIAM J. Appl. Math. 34, 119–148 (1978)
Carrion, M., Arroyo, J.M.: A computationally efficient mixed-integer linear formulation for the thermal unit commitment problem. IEEE Trans. Power Syst. 21, 1371–1378 (2006)
Dolan, E.D., Moré, J.J.: Benchmarking optimization software with performance profiles. Math. Program. 91(2), 201–213 (2002)
Easton, T., Hooker, K.: Simultaneously lifting sets of binary variables into cover inequalities for knapsack polytopes. Discrete Optim. 5(2), 254–261 (2008). (in Memory of George B. Dantzig)
Frangioni, A., Gentile, C., Lacalandra, F.: Tighter approximated milp formulations for unit commitment problems. IEEE Trans. Power Syst. 24, 105–113 (2009)
Gu, Z., Nemhauser, G., Savelsbergh, M.: Lifted flow cover inequalities for mixed 0–1 integer programs. Math. Program. 85, 439–468 (1999)
Gu, Z., Nemhauser, G., Savelsbergh, M.: Sequence independent lifting in mixed integer programming. J. Combin. Optim. 4, 109–129 (2000)
Hammer, P., Johnson, E., Peled, U.: Facet of regular 0–1 polytopes. Math. Program. 8(1), 179–206 (1975)
Hartvigsen, D., Zemel, E.: The complexity of lifted inequalities for the knapsack problem. Discrete Appl. Math. 39(2), 113–123 (1992)
Johnson, E.L., Padberg, M.W.: A note on the knapsack problem with special ordered sets. Oper. Res. Lett. 1, 18–22 (1981)
Kaparis, K., Letchford, A.N.: Cover inequalities. Wiley Encyclopedia of Operations Research and Management Science (2010)
Louveaux, Q., Wolsey, L.A.: Lifting, superadditivity, mixed integer rounding and single node flow sets revisited. Ann. OR 153, 47–77 (2007)
Nemhauser, G., Wolsey, L.: Integer and Combinatorial Optimization. Wiley, New York (1988)
Ott, A.L.: Evolution of computing requirements in the pjm market: past and future. In: Power and Energy Society General Meeting (2010)
Padberg, M.W., Van Roy, T.J., Wolsey, L.A.: Valid linear inequalities for fixed charge problems. Oper. Res. 33(4), 842–861 (1985)
Padberg, M.: (1, k)-Configurations and facets for packing problems. Math. Program. 18, 94–99 (1980)
Roy, T.V., Wolsey, L.: Valid inequalities for mixed 0–1 programs. Discrete Appl. Math. 14, 199–213 (1986)
Sherali, H., Lee, Y.: Sequential and simultaneous lifting of minimal cover inequalities for generalized upper bound constrained knapsack polytopes. SIAM J. Disc. Math. 8, 133–153 (1995)
Wolsey, L.: Valid inequalities for 0–1 knapsack and mips with generalized upper bound constraints. Discrete Appl. Math. 29, 251–261 (1988)
Wolsey, L.A.: Facets of linear inequalities in 0–1 variables. Math. Program. 8, 165–178 (1975)
Wolsey, L.A.: Valid inequalities and superadditivity for 0/1 integer programs. Math. Oper. Res. 2, 65–77 (1977)
Wood, A.J., Wollemberg, B.F.: Power Generation, Operation and Control, 2nd edn. Wiley, New York (1996)
Zemel, E.: Lifting the facets of zero-one polytopes. Math. Program. 15(1), 268–277 (1978)
Zeng, B.: Efficient Lifting Methods for Unstructured Mixed Integer Programs with Multiple Constraints. Ph.D. thesis, Purdue University, Industrial Engineering Department (2007)
Zeng, B., Richard, J.P.P.: Sequence independent lifting for 0–1 knapsack problems with disjoint cardinality constraints (2006)
Zeng, B., Richard, J.P.P.: A framework to derive multidimensional superadditive lifting functions and its applications. In: Integer Programming and Combinatorial Optimization, Lecture Notes in Computer Science, vol. 4513, pp. 210–224 (2007)
Acknowledgments
We thank the reviewers for their thorough review and highly appreciate the comments and suggestions, which significantly contributed to improving the quality of the publication.
Author information
Authors and Affiliations
Corresponding author
Additional information
This research was funded by FONDECYT Grant 1110024 and Millennium Nucleus Information and Coordination in Networks ICM/FIC RC13003.
Appendix: Extended proofs
Appendix: Extended proofs
1.1 Proof of Proposition 1
-
1.
\(\forall j \in M\), define \(\varvec{e_j}\) as the index vector of dimension \(n\) with a unique one in position \(j\). Let \(\varvec{0_n}\) be the zero vector of size \(n\). Let \(\overline{a}\) be the maximum contribution of any binary variable to the knapsack constraint, i.e. \(\overline{a}=\max _{j\in M}a_j\) and \(0<\epsilon <b-\overline{a}\). Then, \(\forall k \in M\), construct \(\varvec{p^k}=(\varvec{e_k},\varvec{0_n})\) and \(\varvec{q^k}=(\varvec{e_k},\epsilon \varvec{e_k})\). Define \(\varvec{s}=(\varvec{0_n},\varvec{0_n})\). The points \(\varvec{p^k}, \varvec{q^k}\) for \(k\in M\), and \(\varvec{s}\) belong to \(X_G\) because of Eq. (1) and \(\bar{a}<b\). These points are affinely independent because \(\forall k \in M, \varvec{p^k}-\varvec{s}\) (\(n\) points) and \(\varvec{q^k}-\varvec{s}\) (\(n\) points) are linearly independent. Since we have described \(2n+1\) affinely independent points in \(X_G\); we have shown that \(X_G\) is full-dimensional.\(\square \)
-
2.
To prove that \(y_k\ge 0\) is facet-defining, we use the same definitions as before, but eliminate element \(\varvec{q^k}\) from the set of valid points. Since we have described \(2n\) affinely independent points in \(X_G\) satisfying these inequalities at equality; we have shown that these inequalities are facet-defining.\(\square \)
-
3.
To prove that \(y_k\le x_k\) is facet-defining; we use the same definitions as in Proposition 1, but eliminate element \(\varvec{p^k}\) from the set of valid points and redefine \(\varvec{q^j}\) as \((\varvec{e_j},\varvec{e_j})\) for all \(j\in M\). Again, since we have described \(2n\) affinely independent points in \(X_G\) and these points satisfy our inequality at equality; we have shown that these inequalities are facet-defining.\(\square \)
-
4.
Note that \(\overline{a}_g\) is the maximum contribution to the knapsack constraint when we choose any binary variable and the minimum contribution in \(M_g\) at the same time. This allows us to prove that for each \(g\in G, \sum _{k\in M_g}x_k\le 1\) is facet-defining, by constructing the points \(\varvec{p}^k\) and \(\varvec{q}^k\), for each \(k\in M\) as follows:
$$\begin{aligned} \varvec{p^{k}} = \left\{ \begin{array}{ll} (\varvec{e_{k}}+\varvec{e_{k_{o}}},\varvec{0_n}) &{} \quad \forall k\notin M_g \\ (\varvec{e_{k}},\varvec{0_n}) &{} \quad \forall k \in M_g \end{array} \right. \end{aligned}$$and
$$\begin{aligned} \varvec{q^{k}} = \left\{ \begin{array}{ll} (\varvec{e_{k}}+\varvec{e_{k_{o}}},\epsilon \varvec{e_{k}}) &{} \quad \forall k\notin M_g \\ (\varvec{e_{k}},\epsilon \varvec{e_{k}}) &{} \quad \forall k \in M_g, \end{array} \right. \end{aligned}$$where \(k_o\in \hbox {argmin}_{k\in M_g}\{a_{k}\}\) and \(0<\epsilon <b-\overline{a}_g\). Since we have described \(2n\) affinely independent points in \(X_G\) and these points satisfy the inequality at equality; we have shown that these inequalities are facet-defining.\(\square \)
1.2 Proof of Theorem 1
The validity of inequality (3) was shown by Van Roy and Wolsey [21]. We prove that (3) is facet-defining for \(X_o:=X\cap \{x_i=0,i\notin C\}\) by constructing a set of \(2s\) affinely independent points in \(X_o\) satisfying it at equality, where \(s=|C|\). For this, define \(C_L=C{\setminus } C_U, C_k^+=\{j\in C_k:\xi _j>{\varGamma }\}\), and \(\bar{C}_k^+=C_k{\setminus } C_k^+\) for \(k=L,U\), and recall that \({\varGamma }=\xi (C)-b>0\) and our hypothesis is \(m(C_U^+)>{\varGamma }\). Let \(t_o\in \hbox {argmin}_{j \in C_U^+}\{\xi _j\}\) and assume that \(\frac{1}{0}=\infty \). Note that \(C_U^+\ne \emptyset \), and thus \(t_o\) exists. Let \(\varvec{e_j}\) be the index vector of dimension \(s\) with a unique one in position \(j\), for all \(j\in C\). Let \(\varvec{0_s}\) be the zero vector of size \(s, \varvec{1_s}\) be the one vector of size \(s, \varvec{1_{u^+}}:=\sum _{j\in C_U^+}\varvec{e_j}, \varvec{1_{\bar{u}^+}}:=\sum _{j\in \bar{C}_U^+}\varvec{e_j}\), and \(\varvec{1_u}=\varvec{1_{u^+}}+\varvec{1_{\bar{u}^+}}\). Then, construct \(\varvec{p^j}\) as
where \(y_{oj}=\frac{{\varGamma }-\xi _j}{m(C_U^+)}\). Now, construct \(\varvec{q^j}\) as
where
\(y_{\pm \epsilon }=\frac{{\varGamma }\pm \epsilon }{m(C_U^+)}\), and \(\varepsilon _j=\frac{\epsilon }{m_j}\) for \(j\in C_U\). Given the above definitions, it is easy to prove that points \(\varvec{p^j}\) and \(\varvec{q^j}\) belong to \(X_o\). Therefore, it is only necessary to evaluate the knapsack constraint of Eq. (1). Verifying the validity of the other constraints is straightforward. Let \(LHS_{KN}\) be the left-hand side of the knapsack constraint of Eq. (1).
-
Case 1 \(\varvec{p^j}\) with \(j\in C_L^+\)
$$\begin{aligned} LHS_{KN} = \xi (C)-a_j= b + {\varGamma }- a_j < b \end{aligned}$$ -
Case 2 \(\varvec{p^j}\) with \(j\in \bar{C}_L^+\)
$$\begin{aligned} LHS_{KN} = \xi (C)-a_j-y_{oj}m(C_U^+) =b + {\varGamma }-a_j - \frac{{\varGamma }-a_j}{m(C_U^+)} m(C_U^+) = b \end{aligned}$$ -
Case 3 \(\varvec{p^j}\) with \(j\in C_U^+\)
$$\begin{aligned} LHS_{KN} = \xi (C)-\xi _j= b + {\varGamma }- \xi _j < b \end{aligned}$$ -
Case 4 \(\varvec{p^j}\) with \(j\in \bar{C}_U^+\)
$$\begin{aligned} LHS_{KN} = \xi (C)-\xi _j-y_{oj}m(C_U^+) = b + {\varGamma }-\xi _j - \frac{{\varGamma }-\xi _j}{m(C_U^+)}m(C_U^+) = b \end{aligned}$$ -
Case 5 \(\varvec{q^j}\) with \(j\in C_L\)
$$\begin{aligned} LHS_{KN} \!=\! \xi (C)-\xi _{t_o}+\delta m_j = b+{\varGamma }- \xi _{t_o} + \delta m_j<b+{\varGamma }-\xi _{t_o}+\xi _{t_o}\!-\!{\varGamma }\!=\!b \end{aligned}$$ -
Case 6 \(\varvec{q^j}\) with \(j\in C_U^+\)
$$\begin{aligned} LHS_{KN} = \xi (C)- y_{+\epsilon }m(C_U^+) + \varepsilon _j m_j = b +\varepsilon _j m_j - \epsilon = b \end{aligned}$$ -
Case 7 \(\varvec{q^j}\) with \(j\in \bar{C}_U^+\)
$$\begin{aligned} LHS_{KN} = \xi (C)-y_{-\epsilon } m(C_U^+) - \varepsilon _j m_j = b -\varepsilon _j m_j + \epsilon = b \end{aligned}$$
By proceeding in the same manner but now evaluating \(\varvec{p^j}\) and \(\varvec{q^j}\) in Eq. (3), we can show that these points satisfy this inequality at equality. Remember that the left-hand side of (3) is \(\sum _{j\in C}\min \{1,\frac{\xi _j}{{\varGamma }}\}\left( x_j-1\right) +\sum _{j\in C_U}\frac{m_j}{{\varGamma }}\left( y_j-x_j\right) \), and its right-hand side is \(-\)1.
-
Case 1 \(\varvec{p^j}\) with \(j\in C_L^+\)
-
Case 3 \(\varvec{p^j}\) with \(j\in C_U^+\)
-
Case 5 \(\varvec{q^j}\) with \(j\in C_L\)
$$\begin{aligned} LHS = -\min \{1,\frac{\xi _j}{{\varGamma }}\} = -1 \end{aligned}$$ -
Case 2 \(\varvec{p^j}\) with \(j\in \bar{C}_L^+\)
-
Case 4 \(\varvec{p^j}\) with \(j\in \bar{C}_U^+\)
$$\begin{aligned} \begin{array}{rcl} LHS= & {} -\min \left\{ 1,\frac{\xi _j}{{\varGamma }}\right\} -y_{oj}\frac{m(C_U^+)}{{\varGamma }} = -\frac{\xi _j}{{\varGamma }}-\left( 1-\frac{\xi _j}{{\varGamma }}\right) = -1 \end{array} \end{aligned}$$ -
Case 6 \(\varvec{q^j}\) with \(j\in C_U^+\)
$$\begin{aligned} LHS=-y_{+\epsilon }\frac{m(C_U^+)}{{\varGamma }}+\varepsilon _j\frac{m_j}{{\varGamma }}=-\frac{{\varGamma }-\epsilon +\epsilon }{{\varGamma }}=-1 \end{aligned}$$ -
Case 7 \(\varvec{q^j}\) with \(j\in \bar{C}_U^+\)
$$\begin{aligned} LHS=-y_{-\epsilon }\frac{m(C_U^+)}{{\varGamma }}-\varepsilon _j\frac{m_j}{{\varGamma }}=-\frac{{\varGamma }+\epsilon -\epsilon }{{\varGamma }}=-1 \end{aligned}$$
Moreover, these points are affinely independent because \(\forall j \in C{\setminus }\{t_o\}, \varvec{p^j}-\varvec{p^{t_o}}\), and \(\varvec{q^j}-\varvec{p^{t_o}}\) are linearly independent. To show this, observe the structure of these points:
where, if elements \(x_{t_o}\) and \(y_{t_o}\) are placed in the last position in the columns, it is possible to obtain a upper-triangular matrix (using the first \(2s-1\) columns) whose diagonal elements are non-zero. Since \(2s\) affinely independent points in \(X_o\) have been described and these points satisfy inequality (3) at equality, it has been shown that these inequalities are facet-defining for \(conv\{X_o\}\).\(\square \)
Rights and permissions
About this article
Cite this article
Angulo, A., Espinoza, D. & Palma, R. Sequence independent lifting for mixed knapsack problems with GUB constraints. Math. Program. 154, 55–80 (2015). https://doi.org/10.1007/s10107-015-0902-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10107-015-0902-5