The more the merrier? On the optimality of market size restrictions

Abstract

This paper provides a novel rationale for the regulation of market size when heterogeneous firms compete. A regulator seeks to maximize total welfare by choosing the number of firms allowed to enter the market, e.g. by issuing a certain number of licenses. Opening up the market for more firms has a two-fold effect: it increases competition and thus welfare, but at the same time, it also attracts more cost-intensive firms, driving down average production efficiency. The regulator hence faces a trade-off between raising beneficial competition and detrimental costs. If goods are sufficiently substitutable, the latter effect can outweigh the former. It is then optimal to restrict the market size, rationalizing a limit to competition. This possibility result holds even in the absence of entry costs, search costs or increasing returns to scale, which previous literature required.

“You will agree, I’m sure, that there’s nothing more destructive than a monopoly.”

“Yes,” said Taggart, “on the one hand. On the other, there’s the blight of unbridled competition.”

“That’s true. That’s very true. The proper course is always, in my opinion, in the middle. So it is, I think, the duty of society to snip the extremes, now isn’t it?”

“Yes,” said Taggart, “it is.”

Ayn Rand, Atlas Shrugged

1 Introduction

Why do we observe markets where regulation restricts the number of firms allowed to compete, effectively creating oligopolies of a certain—at times seemingly arbitrary—size? After all, economic intuition suggests that in a market of profit-seeking firms, welfare is maximized by ensuring the highest possible degree of competition instead of limiting market entry. Even beyond the realm of economic theory, “competition is, in our system, a political and social desideratum” (McNulty 1968, p. 639).

In some markets existing in practice, however, competition is limited in that only a number of firms are allowed to enter. In 2016, the Greek government cut the number of TV licenses granted to privately owned broadcasters from eight to four – only to change it again to five licenses two years later.¹ Similarly, the German football league (Bundesliga) raised the amount of licenses issued for live pay-TV broadcasts of its matches in 2016, claiming an improvement not only for fans and viewers, but also for the league’s finances.² In New York City, the number of taxis in operation is regulated by the Taxi and Limousine Commission, which has barely varied the amount of “medallions” since the 1930s – despite the city having grown rapidly both in population and size, and despite the socially optimal number of such medallions estimated to be about 55% higher than its current value.³ Further examples include the number of licenses given out to telecommunication companies in spectrum auctions or to betting agencies in sport wagering.

Regulators are interested in both consumer surplus and industry profits in most markets, since the latter can (partly) be extracted using e.g. license auctions.⁴ Theory suggests that, unless specific market assumptions are made (which we discuss in the literature review), utmost competition is optimal: welfare is maximized by having as many firms as possible compete with one another. While there may be practical reasons to restrict the market size in specific settings (technical constraints, health concerns, etc.), from an economic perspective “the limit appears to be rather arbitrary” (Borenstein 1988, p. 357).

This paper explains why such restrictions to competition may indeed be optimal for a regulator seeking to maximize total welfare. To this end, it studies a simple market of constant marginal costs. We consider a two-stage model. First, the welfare-maximizing regulator specifies a market size, thus allowing a subset of finitely many interested firms to operate in a market. She does so e.g. by issuing licenses. Second, those firms to which access is granted compete à la Cournot. Crucially, firms differ in their marginal costs, with the costs unknown to the regulator. We show that raising market size by issuing more licenses has a two-fold effect: an increase in competition and a decrease in average production efficiency. We disentangle the two and study their welfare effects to prove that a more oligopolistic market can indeed be welfare-enhancing.

Intuitively, opening up the market gives rise to countervailing forces. On the one hand, it fosters competition. On the other hand, a greater market size also attracts less efficient firms: since firms with lower marginal costs generate higher profits, they enter the market first (as they place higher bids when licenses are auctioned off), while entrants arriving later produce at higher costs. To single out each effect, consider this market opening in two steps. First, add a new firm with production costs equal to the market average. This heats up competition while leaving production efficiency unchanged. As a result, prices are driven down, which is detrimental to firms but beneficial for consumers. Next, raise the entrant’s cost to the value expected by the regulator. Surprisingly, this cost increase does not necessarily harm firms as a whole: it is possible that an increase in average marginal costs, keeping the total number of firms fixed, raises total firm profits. The more efficient firms can exploit the inefficiencies of their high-cost rivals, taking over their market share. The additional firm profits overcompensate the loss for consumers caused by the increase in production costs. When more firms are admitted to a market, we therefore observe a trade-off due to the two—potentially countervailing—effects: fostering competition always increases welfare, while the simultaneous decrease in production efficiency has an ambiguous impact on welfare. The regulator has to consider both the competition and the cost effect in her choice of the market size.

Identifying the welfare-maximizing market size, we first consider the benchmark scenario of homogeneous firms. Here, the standard intuition applies as utmost competition is optimal. The regulator does not impose any restrictions and allows all firms to enter. But with firms being heterogeneous, the regulator may face the aforementioned trade-off between competition and production costs, depending on market characteristics. It can now become optimal in terms of welfare to enforce an oligopoly, granting market access only to a limited number of firms. This main finding is presented as a possibility result: market constellations can be such that competition is desirable only to some extent such that the usual notion of “the more the merrier” does not apply. After establishing this result analytically, the paper then employs numerical methods to study how the optimal regulatory policy varies with the market specifications.

The paper proceeds as follows: the existing literature is reviewed in Sect. 2, while Sect. 3 presents the formal model. In Sect. 4, we first analyze the market equilibrium given a fixed market size. Subsequently, we solve the regulator’s problem of choosing the optimal market size to maximize expected total welfare. Section 5 shows that the results are robust to changes to the model: we analyze both Cournot and Bertrand competition, i.e. firms setting either prices or quantities; heterogeneity is studied both in marginal costs and in quality levels; a regulator interested in auction revenue is considered. Here we also present an auction implementing entry of firms in the order of their efficiency as well as superior mechanisms. All proofs are in the Appendix. Accompanying Mathematica code is available upon request.

2 Related literature

This paper adds to previous work on the optimality of market size restrictions. In the literature, different causes have been identified as to why a finite market size may be optimal. Building on the recent work of Peivandi and Vohra (2021), we can sort these different causes into four different categories. The first two refer to the market participants’ mode of relationship, while the latter two to refer to their heterogeneity.

First, market participants can interact by means of competition. If they compete within a given market, total welfare maximization usually mandates aggravating the competition. However, there are notable exceptions to the dictum that “increasing competition will increase welfare” (Stiglitz 1981, p.184). Specific market characteristics can cause an excess of competition within the market. Seminal papers mention increasing returns to scale (Schumpeter 1942), R &D spillovers (Stiglitz 1981; Spence 1984), entry costs (Mankiw and Whinston 1986) as well as fixed or quadratic production cost components (von Weizsäcker 1980; Suzumura and Kiyono 1987). More recently, Amir et al. (2014) extended the model of Mankiw and Whinston (1986) and identified a “business stealing” condition as a source of inefficient entry under symmetric competition. Instead of competition being ‘internal’ within a given market, it can also be ‘external’ in that it takes place between market-makers. Here, regulation of the respective market sizes may be optimal. For example, Malamud and Rostek (2017) show that fragmenting a large centralized market into smaller ones may allows for a more efficient distribution of systemic risk, increasing total welfare.

Turning to the second mode of interaction, congestion can arise. Here, an increased presence of participants on one side of the market causes negative externalities to other members of the same side. Hence, an over-joining of users can be detrimental to welfare (Yang et al. 2017). An increase in thickness may cause the regulator to favor fragmentation. At times, markets themselves will unravel and fragment, reducing the time or cost required by each transaction (Roth and Xing 1994).

Alternatively, the finiteness of a market’s size may be optimal not due to the mode of interaction between the market participants, but due to their heterogeneity. The third category comprises work where such heterogeneity is in the agents’ information. Kawakami (2017) studies a securities trading with asymmetrically informed traders and shows that an increase traders has two-fold effect. On the one hand, it allows for beneficial risk-sharing, while on the other, it reduces hedging effectiveness. The net effect may be negative, causing finite market size to be welfare optimal. Similarly, Glode and Opp (2020) studies traders with asymmetric expertise regarding the valuation of assets. Again, fragmenting a market to reduce the size of its participants may increase efficiency: the authors show how decentralized over-the-counter markets dominate a centralized market by providing increased incentives to acquire information.

Fourth and finally, agents can be heterogeneous in their fundamental characteristics. Peivandi and Vohra (2021) suggest differences in preference “for order size, anonymity, and likelihood of execution” (p. 164). It is this fourth category that the present paper adds to. However, it derives the optimality of a finite market from heterogeneity in the producers’ marginal costs. In doing so, we extend the model of a differentiated duopoly à la Singh and Vives (1984) to the case of an arbitrary number of competing firms. This yields a model similar to the one of Ledvina and Sircar (2012). However, they focus on the case of unregulated entry and analyze which firms want to be active. This paper, on the other hand, considers the question which firms should be active from a welfare perspective: if entry is attractive for all firms, how many of them should a regulator allow to operate in order to maximize total surplus? We therefore endogenize market size, which the authors take as given.

We have thus located the paper in the more general literature on optimal market size regulation. We analyze the change in welfare caused by the entry of an additional firm of given (though potentially uncertain) production efficiency. A different strand of literature similarly analyzes the effect of a firm’s efficiency on total welfare—but instead of raising the number of firms, it raises the production costs of a specific firm. Salop and Scheffman (1983) shows that while the affected firm itself obviously suffers, the competitor may in fact benefit. The net effect on total firm profits may in fact be positive (Kimmel 1992). Lahiri and Ono (1988) [henceforth LO] add consumers to the welfare analysis. They show that “helping” a small firm, e.g. by boosting its efficiency, may in fact lower total welfare. Our model differs mainly in three aspects. First, we allow for a differentiated market, while goods are necessarily homogeneous in the case of LO. Second, we explicitly study how market characteristics (degree of substitutability, quality of goods, size of the firm pool) affect the necessity for a regulator to intervene. Third, in the setup of LO, the regulator is perfectly informed about the firms’ costs. As Baron and Myerson (1982) argue, “[t]his assumption is unlikely to be met in reality, since the firm would be expected to have better information about costs than would the regulator” (p. 911). We therefore model asymmetric information between firms and regulator. In particular, we assume firms’ costs are random variables whose realization is unknown to the regulator. Returning to the issue of market size regulation, we then show how the findings of LO affect optimal regulatory intervention.

3 Model

We study a two-stage Bayesian game. In the first stage, a welfare-maximizing regulator specifies the market size. She does so by choosing the number of firms allowed to enter, e.g. via issuing licenses. In the second stage, those firms that are granted access compete in a Cournot style. Firms are heterogeneous in their marginal costs, where we refer to a firm with lower costs as “more efficient” throughout this paper.

Regulator: The regulator faces a pool of $m\in {\mathbb {N}}$ firms that seek to enter a market. She chooses the market size $n\le m$, specifying the number of firms allowed to enter. Her goal is to maximize total welfare, given by consumer surplus and firm profits. The regulator is unaware of each firm’s marginal costs. She knows only the cost distribution and the fact that given a market size n, the n firms with the lowest costs are going to enter the market.

We discuss this form of market regulation and information structure in more detail in Sect. 5.2. It is shown that the regulator could benefit from more sophisticated mechanisms allocating permits to enter the market. We will argue that entry of the most efficient firms—instead of selecting specific firms—is a political desideratum rather than an economic one in many regulatory settings. We construct a mechanism and equilibrium strategies implementing this goal, ensuring that more efficient firms indeed enter the market first.

In addition, we follow the empirical observation that the regulator can grant or deny market access to firms, but that she cannot prevent the firms’ strategic profit-maximizing behavior once in the market. The socially optimal solution would require a regulation of production levels and prices to their first-best levels, which in practice is not available to the regulator in competitive markets. Our results extend to the case where the regulator’s objective is not to maximize total welfare but rather the sum of consumer surplus and auction revenue generated from selling licenses for market entry, as shown in Sect. 5.3.

Firms: There are $m\in {\mathbb {N}}$ firms seeking to participate in the market. Each firm $i\in \{1,\ldots ,m\}$ produces quantity $q_i$ of a good at marginal cost $c_i$. Firms sell their goods at price $p_i$ given by the consumers’ inverse demand. A firm’s profit function is given by $\pi _i(p_i,q_i)=q_i(p_i-c_i)$.

Firms are heterogeneous in marginal costs $c_i$. Their costs (or “types”) are i.i.d. draws from U[0, 1]. Denote a cost profile by $\varvec{c}=(c_1,\ldots ,c_m)\in {[0,1]}^m$. Firms know each others’ types, while the regulator does not. This information structure is motivated by producers having more detailed insights into the technical requirements of the specific market and the cost structure of their rivals than a government does.⁵ It allows us to focus on the regulator’s optimal policy facing both imperfect competition and imperfect information without the additional complexity of firm’s strategic choices made under uncertainty. We later identify conditions such that indeed all firms find it profitable to engage in competition.

After the regulator has specified a market size $n\le m$ in the first stage, the n firms with the lowest marginal costs enter, e.g. by having obtained a license. In the second stage, they engage in competition à la Cournot and choose their production levels $\varvec{q}\equiv (q_1,\ldots ,q_n)$.⁶

Consumers: There is a unit mass of consumers with a standard utility function: utility is quadratic in the goods $\varvec{q}$, while it is linear and separable in the numeraire good. With prices denoted by $\varvec{p}\equiv (p_1,\ldots ,p_n)$, consumers choose a bundle $\varvec{q}$ maximizing $U(\varvec{q})-\sum _{i=1}^n p_i q_i$, yielding the inverse demand function $\varvec{p}(\varvec{q})$. We extend the standard model of a differentiated duopoly à la Singh and Vives (1984) to an oligopolistic setting, writing

$$\begin{aligned} U(\varvec{q})=\alpha \sum _{i=1}^n q_i -\frac{1}{2}\bigg (\sum _{i=1}^n q_i^2+\gamma \sum _{i=1}^n\sum _{j\ne i}q_i q_j\bigg ). \end{aligned}$$

(1)

Here, $\gamma$ specifies the degree of product differentiation: for $\gamma =0$, goods are independent, while for $\gamma =1$ they are perfect substitutes. We allow for arbitrary $\gamma \in {[0,1]}$. The factor $\alpha$ can be interpreted as a common quality of all goods, which is sufficiently large such that market entry is profitable for all firms (see Lemma 1 below).⁷

4 Analysis

The analysis is structured as follows: Sect. 4.1 derives the market equilibrium and conducts a welfare analysis given a market size n and a cost profile $\varvec{c}$. Subsequently, Sect. 4.2 determines optimal market size $n^*$ chosen by a regulator facing uncertainty about the cost profile. Section 4.3 considers comparative statics.

4.1 Welfare in the market

Starting from the consumers’ optimization problem, backward induction yields equilibrium production levels of

$$\begin{aligned} q_i=\lambda \Big [\alpha (2-\gamma )-[2+\gamma (n-2)]c_i+\gamma \sum _{j\ne i}c_j\Big ], \end{aligned}$$

(2)

and prices given by

$$\begin{aligned} p_i=\lambda \Big [\alpha (2-\gamma )+[2+\gamma (n-2)-\gamma ^2(n-1)]c_i+\gamma \sum _{j\ne i}c_j\Big ], \end{aligned}$$

(3)

with $\lambda =\{(2-\gamma )[2+\gamma (n-1)]\}^{-1}>0$. Omitting solutions where all production levels and prices are zero, the equilibrium is unique, which follows from applying the Poincaré-Hopf index theorem Vives (1999 p. 48). A key driver in the inefficiency of excessive entry explored in this paper will stem from new entrants’ decreasing production efficiency, i.e. their increase in marginal costs. Therefore, the effect of a firm’s marginal costs on the equilibrium is of interest:

Claim 1

When the marginal costs of a firm increase, ...

• a) production decreases for this firm and increases for all its competitors,

• b) all prices increase.

The equilibrium resembles the results of Ledvina and Sircar (2012). We extend their analyses by also identifying conditions ensuring that production is profitable for every firm: since we are interested in the regulator’s choice of the optimal market size, we want to focus on the case where each firm finds market entrance attractive. That is, we want to ensure that each firm wants to enter the market but should not necessarily do so from a welfare perspective. The following lemma derives a lower bound on the quality ensuring positive supply levels and prices.

Lemma 1

A sufficient condition for prices and quantities given by eqs. (2) and (3) to be weakly positive is $\alpha \ge m$.

Throughout this paper, we hence assume that $\alpha \ge m$ is satisfied.⁸ If this condition is met, it can be shown that for any market size n and any cost profile $\varvec{c}$, the entry of an additional firm causes all incumbents to lower their production levels irrespective of the new rival’s costs, while the total production level increases. Note also the (standard) finding that firms with lower marginal costs have higher profits when participating in the market. This rationalizes our assumption that more efficient firms enter the market first, e.g. because they accept higher prices for licenses permitting such entry, or because they place higher bids in an auction setting.⁹ We present an auction implementing this entry in Sect. 5.2.

We now turn to the welfare analysis, continuing to consider a given profile of marginal costs $\varvec{c}$. With consumer surplus ${\mathcal {W}}_\text {C}(n)=U(\varvec{q})-\sum _{i=1}^n p_i q_i$ and firm profits ${\mathcal {W}}_\text {F}(n)=\sum _{i=1}^n\pi _i$, the first set of observations comes at no surprise:

Claim 2

When the marginal costs of a firm increase, ...

• a) profits decrease for this firm and increase for all its competitors,

• b) consumer surplus decreases.

The effect on total welfare, however, is more intricate. For a clearer picture, we distinguish between three channels through which a change in firm i’s marginal costs affects total welfare given by ${\mathcal {W}}_\text {tot}={\mathcal {W}}_\text {C}+{\mathcal {W}}_\text {F}$, inferring the sign of each channel from Claim 2. First, consumers suffer from an increase in marginal costs and their surplus decreases. Second, firm i’s profit is driven down, and third, the profits of i’s competitors rise. Hence, we have

(4)

with an explicit form of total welfare and its derivative given in the Appendix, see eq. (6). Given the opposing directions of these three channels, what is the sign of the overall effect? In standard Cournot settings with homogeneous firms, total welfare unambiguously decreases in marginal production costs, as will be shown below in Lemma 2. With heterogeneous costs, however, we find:

Proposition 1

When a firm’s marginal costs increase, total welfare may increase.

This result extends the seminal finding of Lahiri and Ono (1988)—i.e. that “helping minor firms [sometimes] reduces welfare”—to differentiated markets.¹⁰

When does the positive competitor effect outweigh the negative consumer and firm effects? As shown in the proof, this is the case if, first, firm i is relatively inefficient even before the increase in its costs ($c_i$ large), and hence it has a small market share ($q_i$ small). Second, (most of) its competitors need to be relatively efficient, i.e. their costs $c_j$ should be low. Both can be seen from writing a firm’s profit as $\pi _j=q_j(p_j-c_j)=[q_j(\varvec{c})]^2$. First, the (beneficial) competitor effect equals $2q_j\frac{{\text {d\!}}{}q_j}{{\text {d\!}}{}c_i}$, thus raising welfare and increasingly doing so if firm j is efficient and i is inefficient, as such a cost profile drives up $q_j$ according to eq. (2) and the derivative $\frac{{\text {d\!}}{}q_j}{{\text {d\!}}{}c_i}$ is independent of the costs. Second, the (detrimental) firm effect is given by $2q_i\frac{{\text {d\!}}{}q_i}{{\text {d\!}}{}c_i}$, which is small (in absolute terms) if firm i produces sparsely. This, again, is the case if i itself is inefficient while its rivals are efficient. Combining both effects, we find:

Corollary 1

When a firm’s marginal costs increase, total firm profits may increase.

Regarding the consumer effect, the detrimental impact on consumers stemming from an increase in firm i’s costs is smaller if consumption of this good was low initially, i.e. if $c_i$ was small and hence $q_i$ as well. The negative consequences hence diminish if the firm whose costs increase is among the less efficient ones, reiterating the dynamics above. To conclude, Proposition 1 shows that there are specific cost profiles where an increase in a firm’s marginal costs can cause a somewhat surprising increase in total welfare. More specifically, this is the case if the firm whose costs increase is relatively small and inefficient, while the opposite is true for its rivals.

4.2 Regulating the market size

This section derives the paper’s key results: it shows that it can be welfare-optimal to restrict the market size, thus justifying the forms of regulation observed in practice and described in the introduction. An increase in competition may in fact decrease total welfare, as Proposition 2 proves. Crucially, this possibility result hinges on the heterogeneity of firms: with homogeneous firms, a benchmark analysis shows that the standard intuition of more competition being optimal does apply and welfare is always maximized by having all firms compete.

We take the perspective of the welfare-maximizing regulator, specifying the market size. Given a size n, she knows that the n most efficient firms will enter into competition¹¹ – but she has to form expectations about the costs of the firms seeking to compete. Formally, her optimization problem is to find the optimal number of firms $n^*\in \{1,\ldots ,m\}$ maximizing ${{\,\textrm{E}\,}}_{\varvec{c}}[{\mathcal {W}}_{\text {tot}}(n)]$.

For the benchmark case of homogeneity, let all m firms share the same marginal costs $c_i=c\in {[0,1]}$. Their goods may still be differentiated, i.e. we still allow for any degree of product differentiation $\gamma \in {[0,1]}$ as well as any quality level $\alpha$ satisfying the condition stated in Lemma 1. The results presented here hold both if the regulator observes c and if she is unaware of the costs realized, knowing only that they are uniformly distributed.

Lemma 2

If firms are homogeneous, it is always welfare-optimal to allow all firms to enter the market.

The result confirms the standard intuition of more competition providing more welfare. Allowing more firms to enter the market has a known two-fold effect: first, it reduces each firm’s profits by lowering both production levels and prices, and second, it raises consumer surplus by providing a larger variety of goods available at lower prices. In a setting of homogeneous firms, the latter effect outweighs the former: the benefit to consumers from having more competition overcompensates the loss to each firm. In consequence, total welfare is maximized by allowing all firms to enter. The regulator always finds it optimal to grant market access to all firms. There is no rationale to limit the market size.

Having considered a setting with symmetric firms, we now turn to a more realistic market with heterogeneous firms whose production costs may differ, with the regulator only knowing their distribution. This can drastically change the optimal policy for market regulation. Total welfare may now be maximized by restricting market access, providing a rationale for the seemingly arbitrary restriction of market access sometimes observed in practice.

Proposition 2

If firms are heterogeneous, it can be welfare-optimal to limit competition.

When the regulator opens up the market by allowing more firms to enter, there are two forces affecting total welfare: a competition effect and a cost effect. The competition effect resembles the observation made in the setting of homogeneous firms: firms suffer from a decrease in prices and production levels, while consumers benefit from a larger variety of goods at lower prices. The net impact of this competition effect is positive, as observed in the previous benchmark. The cost effect, however, was not present before and stems from the firms’ heterogeneity:

Since firms enter the market in the order of their efficiency, a newly arriving firm is known to be less efficient than those already in the market. This does not only change the number of firms (as captured by the competition effect) but also changes the cost distribution, as it increases expected average production costs. Intuitively, we can single out the latter change by first adding a firm whose marginal costs are equal to the current market average (changing only the market size and thus creating a competition effect) and then decreasing the entrant’s costs to the expected value given by the $(n+1)$-th order statistic. In this second step, we effectively reduce the efficiency of a single firm and can hence recall Proposition 1, stating that such a reduction may in fact raise welfare.

When we now combine this cost effect (driving down welfare) with the competition effect (working the opposite way), Proposition 2 shows that the net impact can be negative. It may thus be beneficial to limit the market size, restricting the number of entrants to a value $n^*<m$. Note that this finding is a possibility result: obtaining the welfare-maximizing number of firms is not analytically possible in most cases and can only be numerically approximated.¹²

4.3 The effect of market characteristics

Interaction within the market as well as regulation of the market depend on exogenous parameters. In particular, the optimal market size $n^*$ is driven by quality level $\alpha$, degree of product differentiation $\gamma$, and size m of the firm pool. In this section, we analyze their interplay. Observations stemming from the numerical simulations will be referred to as results, as opposed to the lemmata and propositions above, which were proven analytically.

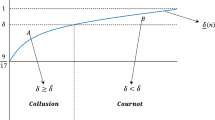
For each of the market characteristics, we plot $n^*$ as a function of the market characteristics $\alpha , \gamma , m$. In each plot, a solid bold line indicates the “demarcation line”. Here, $n^*=m$ becomes optimal. The line thus separates two parameter ranges: to one side of the line, the regulator optimally allows all firms to enter. This area is colored in orange, depicted by the diagonal plane in Figs. 1 and 3 and by the horizontal plane in Fig. 2. The regulator would even prefer a larger market size ($n>m$) but is restricted to the number of firms m available in the pool. The area to the other side of the demarcation line is colored in blue. Here, we observe the effect this paper rationalizes: the regulator finds it optimal to restrict access by setting some interior value $n^*<m$.

Quality level $\alpha$: The quality level captures the utility consumers derive from the consumption of some good before considering decreasing marginal benefits or substitution effects. If this quality grows, consumers increasingly benefit from large consumption levels. As more firms enter the market, the expected level of total production Q increases, affecting total welfare both positively and negatively: positively via the quality $\alpha$ derived from consumption and negatively via decreasing marginal benefits as well as via substitution effects. In addition, total firm profits may decrease. For high levels of $\alpha$, the positive effect receives greater weight. The market can thus be opened further than if $\alpha$ was small. Only if the number of firms in the market becomes too large, the negative effects dominate and a restriction is optimal.

Fig. 1

Optimal market size $n^*$ as a function of pool size m and quality level $\alpha$

This intuition is visualized by Fig. 1: take a fixed degree of product differentiation $\gamma$ (we turn to the effect of $\gamma$ below). For small sizes of the firm pool size m , it is optimal to have all firms enter the market, irrespective of the quality level $\alpha$. This is seen in the diagonal plane left of the solid line, where we have $n^*=m$ and the regulator would prefer to have even more firms compete. At some point, however, increasing the market size—and thus total production—brings increasingly negative effects, such that $n^*<m$ becomes optimal. This tipping point is indicated by the solid line, to the right of which a restriction of the market size is optimal. Now we increase the quality level $\alpha$ and thus the welfare gain from total production Q: the tipping point moves further up and the regulator wants more firms to enter. We therefore find:

Result 1

Restricting the market size is optimal if the quality of goods is sufficiently low.

Fig. 2

Optimal market size $n^*$ as a function of pool size m and product differentiation $\gamma$

Degree of Product Differentiation $\gamma$: Consumers’ utility derived from goods is diminished by substitutability effects. With a growing number of firms, this detrimental impact on welfare increases: there is a larger level of total production, and a larger number of different goods between which substitution effects arise. If $\gamma$ is sufficiently large, this outweighs the beneficial effects of an increase of competition caused by opening up the market. We then expect the optimal policy not to allow entry for all firms.

Figure 2 illustrates this finding. The diagonal plane again indicates where the regulator fully opens the market and would prefer to have even more firms in competition. As the number of available firms m increases, however, we reach a tipping point indicated by the solid line, beyond which more competition lowers welfare. This point is reached earlier for larger values of $\gamma$: substitution effects dominate and only a fraction of those firms in the pool is allowed to enter the market. If goods are too differentiated or the pool size is too small, the regulator will not want to limit competition. We summarize this finding:

Result 2

Restricting the market size is optimal if the goods are sufficiently substitutable.

For an interplay of $\gamma$ and $\alpha$ on the optimal regulatory policy, consider Fig. 3. If the goods are sufficiently differentiated ($\gamma$ small) and have sufficiently high quality ($\alpha$ large), there is no restriction to competition. This is observed in the left, flat part of the graph, where access is granted to all firms. As differentiation or quality decrease, the benefits of more competition shrink and we reach the point where restriction starts becoming optimal. The less differentiated the goods are (i.e. the larger $\gamma$), the lower is the quality threshold below which the regulator limits market access. Conversely, the higher the quality of the goods (i.e. the larger $\alpha$), the more substitutability is required for such a limiting policy to be optimal.

Pool Size m: An increase in the number m of firms seeking to enter affects the regulator’s policy through two channels. First, it simply allows her to open up the market further, as she is constrained in her choice to set $n\le m$. Second, for a fixed choice of n, the pool size also determines the cost distribution of those n (out of m) most efficient firms entering the market. Since the firms’ costs are i.i.d. draws from a uniform distribion on the unit interval, the i-th most efficient firm has expected costs of $\frac{i}{m+1}$. As the pool size—and hence the sample size—increases by an additional firm, this expectation changes to $\frac{i}{m+2}$. Similarly, the expected efficiency of all other firms grows.

This effect can equivalently be explained in terms of statistical sampling: the average costs of the n most efficient firms decrease in the sample size m. Only if this size is sufficiently large does the regulator prefer to have a subset of the sample compete rather than all firms. The advantage of a cost-efficient yet oligopolistic market then outweighs a more competitive alternative with higher average costs:

Result 3

Restricting the market size is optimal if many firms seek to compete.

Fig. 3

Optimal market size $n^*$ as a function of quality level $\alpha$ and product diff. $\gamma$

The result is visualized by Figs. 1 and 2. For low values of m, the regulator wants all firms to engage in competition. Increasing m hence increases the optimal market size $n^*$, as observed in the diagonal plane in both plots. At some point, however, the detrimental cost effect from additional entry outweighs beneficial competition effect and the regulator starts restricting access. But even then, a further increase in m has a positive effect on $n^*$: instead of a flat plane beyond the “tipping point” indicated by the solid line, we still observe a growth of $n^*$, even though it remains below m. This growth stems from the fact that as the pool size increases to $m+1$, the expected efficiency of the m original firms rises—both of those that were operating in the market and of those still seeking entry. The cost effect driving down welfare is reduced vis-à-vis the competition effect and the regulator allows some additional firms to enter.

5 Robustness and extensions

This section extends the key result of the paper—i.e., that it can be welfare-enhancing to restrict market entry due to firm heterogeneity—to variations of the market modeling. First, we show that our finding carries over to firms competing à la Bertrand instead of Cournot (Sect. 5.1). We then discuss the assumptions on market entry and information structure. To this end, we devise an auction implementing an entry of firms in the order of efficiency (Sect. 5.2) and consider regulator interested in the revenue generated from auctioning off the licenses rather than in industry profits per se, therefore maximizing consumer surplus plus auction revenue. Again, our results carry over (Sect. 5.3). The same holds true of firms are heterogeneous in quality levels $\alpha _i$ instead of production costs $c_i$ (Lemma A2 in the Appendix).

5.1 Bertrand competition

In a Bertrand market, firms choose their prices expecting consumers’ consumption levels. In analogy to the Cournot case, we can derive equilibrium prices $\varvec{p}$ and quantities $\varvec{q}$ as a function of the cost profile $\varvec{c}$. Given the complexity of the expressions, we defer their presentation to the Appendix.

Again we want to ascertain that, first, all firms want to participate in the market but should not necessarily do so from a total welfare perspective and, second, that more efficient firms enter the market first, i.e. that firms with lower marginal costs generate higher profits. We find that a lower bound on the quality level $\alpha$ satisfies both requirements:

Lemma 3

If firms compete in a Bertrand market and the quality $\alpha$ of their goods is sufficiently high, there exists an equilibrium in which each firm entering the market obtains a profit which is strictly positive and decreasing in marginal costs.

More formally speaking, Lemma 3 states that for any pool size $m\in {\mathbb {N}}_+$ and any degree of product differentiation $\gamma \in {[0,1)}$, there exists a lower bound ${\underline{\alpha }}(m,\gamma )$ on the quality such that, if $\alpha >{\underline{\alpha }}$, there is an equilibrium with $(\varvec{q},\varvec{p})\in {\mathbb {R}}_+^{2n}$ and $\pi _i>0$ as well as $\frac{{\text {d\!}}{}\pi _i}{{\text {d\!}}{}c_i}\le 0$ $\forall i$ for every market size $n\in \{1,\ldots ,m\}$ and every cost profile $\varvec{c}\in {[0,1]}^m$.¹³

Having ensured that all firms find market participation profitable, and that they enter in the order of their efficiency, we now turn to the regulator’s problem. She specifies the market size, seeking to maximize expected total welfare. In particular, we ask whether she may again find it optimal to limit market access by setting a market size n smaller than the firm pool size m. The answer is positive:

Proposition 3

If firms compete à la Bertrand, it can be welfare-optimal to limit competition.

The qualitative finding of firm heterogeneity rationalizing restrictions to market size therefore extends to a Bertrand setting. While the computations are more involved given the more complex market equilibrium $(\varvec{q},\varvec{p})$, the intuition is the same as in the Cournot case, see the discussion in Sect. 4.2.

5.2 Market entry and information structure

So far, we have assumed that firms enter the market in the order of their production costs, with more efficient firms entering first. This section rationalizes our assumption by providing both empirical evidence as well as an auction implementing such entry.

To start with, recall the information structure of the Bayesian game. Our model takes seriously the intuition of Baron and Myerson (1982) that the regulator is unlikely to know the firms’ production costs and hence only is aware of their distribution. In this regard, we depart from the perfect information setting of Lahiri and Ono (1988). The firms, on the other hand, know their rivals’ cost, as commonly assumed in the regulation of competitive markets, see e.g. Vickers (1995).

This raises two questions. First, couldn’t the regulator extract the information held by the firms? And second, couldn’t she make use of this information to design a more efficient entry mechanism? The answer to both questions is affirmative. Regarding the first, the regulator could easily and costlessly extract this information using a “shoot the liar mechanism”, see Crémer and McLean (1988): each firm is asked for a report on the whole cost profile and deviations from unanimity are penalized. This would, second, also allow for a more efficient form of regulation, as an entry in the order of production costs is not necessarily optimal ex post: from Proposition 1 we have learned that total welfare can increase if a small firm becomes less efficient. Hence, it may be socially preferable to replace one of the n most efficient firms by a less efficient competitor. An optimal mechanism regulating entry would thus first seek to elicit the realized cost profile and then select individual firms to enter.

In the light of these observations, why do we nevertheless focus on an entry of the firms with lowest production costs? The focus is motivated by a perception governing many procurement processes and license auctions in practice: when striving for efficient outcomes, “[e]fficiency [is] understood as putting the licenses into the hands of the bidders with the best business plans” (Binmore and Klemperer 2002, p. C79). In consequence, an auction does not necessarily aim at maximizing total welfare ex post—instead, it merely “ensures that the object is allocated to that bidder that values it most” (van Damme 2002, p. 7). But this is not only an empirical observation: on the theory side, Biglaiser and Crémer (2020) similarly assume that those market participants with the highest expected gain are the ones to move first.

When determining the optimal market size to be set by the regulator, this paper thus effectively considers the the above policy practice as a constraint. Recall that in the present model, a firm’s profit decreases in its marginal costs irrespective of the rivals active in the market (Claim 2). Hence, more efficient firms have a higher valuation of market entry irrespective of the firms they are going to compete with. An incentive-compatible auction will thus induce higher bids from these firms. We now devise an auction which causes the firms with lowest costs to enter in equilibrium. It thus satisfies the requirement of granting access only to those with highest valuations and meets a political rather than a purely economic objective.

Lemma 4

Consider n sequential second-price single-unit open-bid auctions with a cap of one item per bidder. There is an equilibrium in bidding strategies where each of the n most efficient firms wins one of the units for any cost profile.

The equilibrium involves grim trigger strategies where on the equilibrium path, firms bid their valuation truthfully. Efficient firms need to be deterred from postponing their bids in hope of acquiring a license in later rounds at a cheaper price. This is achieved by having a selection of the remaining firms to start bidding the monopoly profit if a deviation occurred, thus punishing the deviant while also ensuring that overbidding is unattractive for the less efficient firms. For details, see the proof.¹⁴

The allocation generated by this auction does not only satisfy the political desideratum of granting entry to the most efficient firms, but it also is robust to aftermarket trade. If firms can sell their licenses ex post to rivals who failed to place winning bids, incumbents will look for a rival with a license valuation higher than their own. But since all rivals not yet holding a license are less efficient, their valuation will necessarily be lower as long as the profile of competitors is unchanged. Bilateral exchange between firms therefore does not lead to a reallocation, although a centralized clearing house with side transfers could. Note that the desired allocation can also be implemented using a uniform-price auction selling all licenses at the same market-clearing price. This does, however, generate a much lower auction revenue.

5.3 A regulator interested in auction revenue

Given the auction just introduced, we now consider a regulator who seeks to maximize consumer surplus plus auction revenue instead of total welfare. Considering that only a fraction of the industry profits can be extracted, is it still optimal to restrict the market size?

From Lemma 4 we know that firms bid their valuation truthfully in equilibrium. Given the second-price auction, revenue in the first round thus is given by the profits of the second most efficient firm, in the second round by the third most efficient firm, and so forth to the n-th round, where the revenue is equal to the profit the $(n+1)$-th most efficient firm would generate if it entered the market instead of the n-th most efficient one. Using strategies from Lemma 4, we can solve the regulator’s new problem of choosing the market size $n^*$ which maximizes the expected sum of consumer surplus and auction revenue. We find that it can again be optimal to restrict the market size:

Proposition 4

If the regulator maximizes expected consumer surplus plus auction revenue, it can be welfare-optimal to limit competition.

6 Conclusion

This paper presents a novel explanation for restrictions to the market size. We show that a regulator seeking to maximize total welfare may find it optimal to grant market access only to a limited number of firms, e.g. by issuing a certain number of licenses. Considering a two-stage Bayesian game, the regulator faces a finite number of firms seeking to enter a market. Firms differ in their marginal costs, with the regulator knowing only the distribution. In the first stage, the regulator specifies a market size, allowing the most efficient firms to enter. In the second stage, these firms engage in Cournot competition.

If firms are homogeneous, the standard notion of more competition being better does apply. That is, the regulator finds it welfare-optimal to allow unrestricted market entrance. But if firms are heterogeneous, this paper rationalizes regulation. In particular, expected welfare can be maximized by choosing a certain oligopolistic market size.

We identified a two-fold effect driving this possibility result. When the market is opened up and more firms are admitted, there is a change to, first, the degree of competition and, second, the distribution of production costs in the market. The competition effect harms firms and benefits consumers, with a positive net effect on total welfare. The cost effect is ambiguous: new entrants are less efficient and hence drive up average production costs, but firm profits and total welfare may nevertheless rise—despite a decrease in consumer surplus. This stems from the observation that if a small firm becomes less efficient, its more efficient rivals may take over some of its market share. The loss to this small firm is overcompensated by the additional profit of its competitors, causing overall firm profits and total welfare to increase.

When the regulator opens up the market, she thus faces a trade-off between beneficial competition and a potential decrease in production efficiency. The latter effect may outweigh the former, such that she optimally limits the number of firms allowed to operate. This result does not hinge on the existence of entry costs, search costs or increasing returns to scale, which previous literature required.

The two-fold effect and the optimality of regulation are robust to changes to the model. We looked at both Cournot and Bertrand competition, at firms being heterogeneous either in their production costs or in the quality of their goods, and at a regulator interested in the revenue generated from auctioning off licenses for market entry. In our modeling, we took seriously the policy practice of preferring more cost-efficient firms to enter the market first. While this approach is not welfare-optimal, it mirrors many real-world instances of regulatory practice. Our approach thus shows that in cases where it is either not desirable or not possible to select individual firms to enter, restrictions to the market size may be necessary, and limits to competition may be adequate.

Appendix: Proofs

Proof of Claim 1

We first briefly derive the market equilibrium given by eqs. (2) and (3). Consumers’ optimization problem yields an inverse demand given by $p_i=\alpha -q_i-\gamma \sum _{j\ne i} q_j$. Firms maximize their profits by setting production levels, considering those of their rivals, yielding $q_i=\frac{1}{2}(\alpha -c_i-\gamma \sum _{j\ne i}q_j)$. Summing over all firms and rearranging yields a firm’s chosen quantity as a function of the cost profile, as stated in eq. (2), as well as the resulting prices from eq. (3). Now the claim directly follows from differentiating these equilibrium values with respect to marginal costs. Let $i,j\in \{1,\ldots ,n\}$. From eq. (2), we obtain

$$\begin{aligned} \frac{{\text {d\!}}{}q_i}{{\text {d\!}}{}c_j} ={} {\left\{ \begin{array}{ll} -\lambda [2+\gamma (n-2)] &{}<0 \text { if } i=j \\ \lambda \gamma &{}\ge 0 \text { if } i\ne j \end{array}\right. } \end{aligned}$$

proving part a). Differentiating eq. (3) yields

$$\begin{aligned} \frac{{\text {d\!}}{}p_i}{{\text {d\!}}{}c_j} ={} {\left\{ \begin{array}{ll} \lambda [2+\gamma (n-2)-\gamma ^2(n-1)] &{}>0 \text { if } i=j \\ \lambda \gamma &{}\ge 0 \text { if } i\ne j \end{array}\right. } \end{aligned}$$

and thus proves part b).

Proof of Lemma 1

The Lemma states that:

$$\begin{aligned} \alpha \ge m \Rightarrow p_i, q_i\ge 0\quad \forall i,n,m,\varvec{c},\gamma \,\text { s.t. } 1\le i\le n\le m, (\varvec{c},\gamma )\in {[0,1]}^{m+1}. \end{aligned}$$

(5)

Since $p_i\ge q_i$, it suffices to show positivity of $q_i$. The denominator of eq. (2) is strictly positive while the numerator is decreasing in $c_k$ for $k=i$ and increasing for $k\ne i$. Hence, consider the cost profile $c_i=1, c_j=0$. The numerator becomes $\alpha (2-\gamma )-[2+\gamma (n-2)]$, which decreases in $\gamma$ for $n\ge 2$ (for $n=2$, $q_i$ is trivially positive). Hence, let $\gamma =1$. We have $q_i\ge \{(2-\gamma )[2+\gamma (n-1)]\}^{-1}(\alpha -n)$. Since $n\le m$, weak positivity is ensured if $\alpha \ge m$. Note that positivity is strict unless $\alpha =m=n, \gamma =1, c_i=1, c_j=0$.

Proof of Claim 2

The profits of firm i given a profile of costs $\varvec{c}$ equal

$$\begin{aligned} \pi _i= q_i(p_i-c_i)= q_i\lambda \{\alpha (2-\gamma )+[2+\gamma (n-2)-\gamma ^2(n-1)-\lambda ^{-1}]c_i+\gamma \smash {\sum _{j\ne i}c_j}\}= q_i^2. \end{aligned}$$

Differentiation with respect to marginal costs thus yields $\frac{{\text {d\!}}{}\pi _i}{{\text {d\!}}{}c_j}=2q_i\frac{{\text {d\!}}{}q_i}{{\text {d\!}}{}c_j}$. Recall from Lemma 1 that for $\alpha \ge m$ we have $q_i\ge 0\,\forall i$. Invoke Claim 1 for the sign of $\frac{{\text {d\!}}{}q_i}{{\text {d\!}}{}c_j}$. Combining, we obtain

$$\begin{aligned} \frac{{\text {d\!}}{}\pi _i}{{\text {d\!}}{}c_i}\le 0,\quad \frac{{\text {d\!}}{}\pi _i}{{\text {d\!}}{}c_j}\ge 0\text { if } i\ne j, \end{aligned}$$

which proves part a) of the claim.

For part b), we need to show that the derivative of consumer surplus with respect to any firm’s marginal costs is negative. We introduce the following notation: write consumer surplus ${\mathcal {W}}_\text {C}(n)=\eta ^\text {C}_1+\eta ^\text {C}_2\sum _{i=1}^n c_i+\eta ^\text {C}_3\sum _{i=1}^n c_i^2+\eta ^\text {C}_4\sum _{i=1}^n\sum _{j\ne i}c_i c_j$. Later, we will use analogous (though of course not necessarily equal) coefficients for firm profits and total welfare. Using this notation, we must prove that $\frac{{\text {d\!}}{}{\mathcal {W}}_\text {C}}{{\text {d\!}}{}c_i}=\eta _2^\text {C}+2\eta _3^\text {C}c_i+2\eta _4^\text {C}\sum _{j\ne i}c_j\le 0\ \forall i$. We find the following coefficients:

$$\begin{aligned} \eta _1^\text {C} ={}&\frac{\lambda ^2}{2} \alpha ^2(2-\gamma )^2[\gamma (n-1)+1]n{} & {} >0 \\ \eta _2^\text {C} ={}&-\lambda ^2 \alpha (2-\gamma )^2[\gamma (n-1)+1]{} & {} <0 \\ \eta _3^\text {C} ={}&\frac{\lambda ^2}{2}\{\gamma [-\gamma ^2(n-2)(n-1)+\gamma (n-7)n+7\gamma +4n-8]+4\}{} & {} >0 \\ \eta _4^\text {C} ={}&\frac{\lambda ^2}{2}\gamma ^2[\gamma (n-1)-n+2]{} & {} \gtreqless 0 \end{aligned}$$

Hence, the sign of $\eta _4^\text {C}$ is ambiguous. Since the derivative is linear in $c_j$, we consider the case of both $c_j=0$ and $c_j=1$. For $\eta _4^\text {C}>0$, we have

$$\begin{aligned} \frac{{\text {d\!}}{}{\mathcal {W}}_\text {C}}{{\text {d\!}}{}c_i}\le {}&\eta _2^\text {C}+2\eta _3^\text {C}+2\eta _4^\text {C}(n-1)\\ {}={}&-\lambda ^2(\alpha -1)(2-\gamma )^2[1+\gamma (n-1)]\le 0. \end{aligned}$$

If, on the other hand, $\eta _4^\text {C}\le 0$, it holds true that

$$\begin{aligned} \frac{{\text {d\!}}{}{\mathcal {W}}_\text {C}}{{\text {d\!}}{}c_i}\le {}&\eta _2^\text {C}+2\eta _3^\text {C}\\&=\, \lambda ^2 \{4[1+\gamma (n-2)]+\gamma ^2[7-2\gamma +n\{3\gamma -7+n(1-\gamma )\}]\\ {}&\quad -\alpha (2-\gamma )^2[1+\gamma (n-1)]\} \\&\le \, {-\lambda ^2}(n-1)\{4+\gamma [\{4-\gamma (5-2\gamma )\}n+\gamma (7-2\gamma )-8]\} \\&\le \, 0, \end{aligned}$$

where we have used that $\alpha \ge n$ at the second inequality. This proves that consumer surplus ${\mathcal {W}}_\text {C}$ is weakly decreasing in every firm’s marginal costs.

Proof of Proposition 1

Using eqs. (2), (3) as well as $\lambda$ from above, we find

(6)

We want to show the existence of cases where $\frac{{\text {d\!}}{}{\mathcal {W}}_\text {tot}}{{\text {d\!}}{}c_i}>0$. In analogy to the notation introduced in the proof of Claim 2, we write ${\mathcal {W}}_\text {tot}(n)=\eta _1+\eta _2\sum _{i=1}^n c_i+\eta _3\sum _{i=1}^n c_i^2+\eta _4\sum _{i=1}^n\sum _{j\ne i}c_i c_j$. Hence, we have $\eta _1,\eta _3>0$, while $\eta _2,\eta _4\le 0$. With $\frac{{\text {d\!}}{}{\mathcal {W}}_\text {tot}}{{\text {d\!}}{}c_i}=\eta _2+2\eta _3 c_i+2\eta _4\sum _{j\ne i}c_j$, we therefore consider the cost profile $c_i=1$, $c_j=0\ \forall j\ne i$ and assume $\gamma =1$, yielding

Since we need $\alpha \ge m$ by Lemma 1 and $n\le m$ by assumption, we consider $n=\alpha$. Here, the derivative takes the form $n^2-1$, which is strictly positive unless there is a monopoly. We thus have identified market situations where, given the model at hand, an increase in some firm’s marginal costs can cause total welfare to rise.

Proof of Lemma 2

The lemma claims that:

$$\begin{aligned} \text {If } c_i=c\in {[0,1]}\ \forall i: \mathop {\text {arg max}}\limits _{n\in \{1,\ldots ,m\}} {{\,\textrm{E}\,}}_c[{\mathcal {W}}_{\text {tot}}(n)]=m\quad \forall \alpha , \gamma , m \text { s.t. } 0\le \gamma \le 1\le m\le \alpha . \end{aligned}$$

First consider the case where the costs are not known by the regulator. With $c_i=c\sim U{[0,1]}\ \forall i$, we have ${{\,\textrm{E}\,}}[c_i]=\frac{1}{2}$, ${{\,\textrm{E}\,}}[c_i^2]=E[c_i c_j]=\frac{1}{3}$. Plugging this into eq. (6) for total welfare yields

$$\begin{aligned} {{\,\textrm{E}\,}}_c[{\mathcal {W}}_{\text {tot}}] ={}&{{\,\textrm{E}\,}}_c[\eta _1+\eta _2\sum _{i=1}^n c_i+\eta _3\sum _{i=1}^n c_i^2+\eta _4\sum _{i=1}^n\sum _{j\ne i}c_i c_j] \\ ={}&\eta _1 + \frac{1}{2}n\eta _2+\frac{1}{3}[n\eta _3+n(n-1)\eta _4] \\ ={}&\frac{n[1+3\alpha (\alpha -1)][3+\gamma (n-1)]}{6[2+\gamma (n-1)]^2}. \end{aligned}$$

To analyze the effect of an increase in the market size n on expected welfare, we take the derivative:

$$\begin{aligned} \frac{{\text {d\!}}{}}{{\text {d\!}}{}n}{{\,\textrm{E}\,}}_c[{\mathcal {W}}_{\text {tot}}] ={}&\frac{[1+3\alpha (\alpha -1)]\{6+\gamma [n(1-\gamma )+\gamma -5]\}}{6[2+\gamma (n-1)]^2}, \end{aligned}$$

which is strictly positive $\forall \alpha ,\gamma ,n,m \text { s.t. } 0\le \gamma \le 1\le n\le m\le \alpha$. Next, consider the case where the costs are $c_i=c\ \forall i$ with c being public. We have

$$\begin{aligned} {\mathcal {W}}_{\text {tot}} ={}&\eta _1+n \eta _2 c+[n\eta _3 +n(n-1) \eta _4] c^2 \\ ={}&\frac{n(\alpha -c)^2[3+\gamma (n-1)]}{2[2+\gamma (n-1)]^2}, \\ \Rightarrow \ \frac{{\text {d\!}}{}}{{\text {d\!}}{}n}{\mathcal {W}}_{\text {tot}} ={}&\frac{(\alpha -c)^2\{6+\gamma [n(1-\gamma )+\gamma -5]\}}{2[2+\gamma (n-1)]^3}, \end{aligned}$$

which again is weakly positive $\forall \alpha ,\gamma ,n,m,c \text { s.t. } 1\le n\le m\le \alpha , (c,\gamma )\in {[0,1]}^2$ and equal to zero only for the polar case of a monopoly with $\alpha =c=1$ (recall that $n\le \alpha$).

Proof of Proposition 2

The proposition states that:

Recall the term for total welfare given by ${\mathcal {W}}_\text {tot}(n)=\eta _1+\eta _2\sum _{i=1}^n c_i+\eta _3\sum _{i=1}^n c_i^2+\eta _4\sum _{i=1}^n\sum _{j\ne i}c_i c_j$, with the coefficients in eq. (6). We now need to compute the summations over expected marginal costs.

Lemma A1

The regulator’s expectations of the firms’ marginal costs are given by:

$$\begin{aligned} \sum _{k=1}^n{{\,\textrm{E}\,}}[c_{(k)}]&{}= \frac{n(n+1)}{2(m+1)} \\ \sum _{k=1}^n{{\,\textrm{E}\,}}[c_{(k)}^2]&{}= \frac{n(n+1)(n+2)}{3(m+1)(m+2)} \\ \sum _{k=1}^n\sum _{l\ne k}{{\,\textrm{E}\,}}[c_{(k)}c_{(l)}]&{}= \frac{n(n-1)(n+1)(n+2)}{4(m+1)(m+2)} \end{aligned}$$

Proof of Lemma A1

We are interested in the expected costs of the n most efficient firms out of m, with firm costs $c_1,\ldots ,c_m$ being an i.i.d. sample from U[0, 1]. That is, we need to compute expectations for the first n order statistics. Incorporating the cases of heterogeneity in both costs ($c_i$) and quality ($\alpha _i$), we follow standard convention in that we write X for the random variable (“cost/quality type”) and denote by $X_{(i:m)}$ the i-th order statistic of a sample with size m. That is, the variables are ordered such that

$$\begin{aligned} X_{(1:m)}\le \ldots \le X_{(n:m)} \le \ldots \le X_{(m:m)}. \end{aligned}$$

To simplify notation, we write $X_{(i)}$ whenever the sample size is m. Realizations are denoted by lowercase letters.

Distributions: Let F(x) be the cumulative distribution function (cdf) of the unordered random variables $X_k$ and $F_{(i)}(x)$ the cdf of the i-th order statistic $X_{(i)}$. Verbally, the latter denotes the probability that at least i of the m (unordered) $X_k$ are less than or equal to x:

$$\begin{aligned} F_{(i)}(x)=\sum _{k=i}^m \left( {\begin{array}{c}m\\ k\end{array}}\right) [F(x)]^k[1-F(x)\big ]^{m-k}. \end{aligned}$$

(7)

Differentiating and rearranging yields the probability density function (pdf):

$$\begin{aligned} f_{(i)}(x) =\frac{{\text {d\!}}{}}{{\text {d\!}}{}x}F_{(i)}(x)=m f(x)\left( {\begin{array}{c}m-1\\ i-1\end{array}}\right) [F(x)]^{i-1}[1-F(x)]^{m-i} \end{aligned}$$

(8)

For the distribution $X\sim U{[0,1]}$, the pdf of the i-th order statistic is given by:

$$\begin{aligned} f_{(i)}(x)=\frac{m!}{(i-1)!(m-i)!}x^{i-1}(1-x)^{m-i}. \end{aligned}$$

(9)

Since we will encounter “mixed terms” when computing expected types, we also need to consider the joint pdf of $X_{(i)}, X_{(j)}$. For $i\le j$ and $x_i\le x_j$, it holds that

$$\begin{aligned} f_{(i,j)}(x_i,x_j)=\frac{m!}{(i-1)!(j-i-1)!(m-j)!}x_i^{i-1}(x_j-x_i)^{j-i-1}(1-x_j)^{m-j}.\nonumber \\ \end{aligned}$$

(10)

Expected values: To compute expectations of the order statistics $x_{(i)}$ as well as squared and mixed terms, we make use of the Beta function given by $B(i,j)= \frac{(i-1)!(j-1)!}{(i+j-1)!}$ for $i,j\in {\mathbb {N}}_+$. This yields the expected marginal cost of the i-th most cost efficient firm:

$$\begin{aligned} {{\,\textrm{E}\,}}[x_{(i)}]&{}= \int _0^1 x f_{(i)}(x) {\text {d\!}}{}x \\&{}= \frac{1}{B(i,m-i+1)}\int _0^1x^{(i+1)-1}(1-x)^{(m-i+1)-1}{\text {d\!}}{}x\\&{}= \frac{B(i+1,m-i+1)}{B(i,m-i+1)} \\&{}= \frac{i}{m+1} \end{aligned}$$

Similarly, we find:

$$\begin{aligned} {{\,\textrm{E}\,}}[x_{(i)}^2]&{}= \frac{i(i+1)}{(m+1)(m+2)} \\ {{\,\textrm{E}\,}}[x_{(i)}x_{(j)}]&{}= \frac{i(j+1)}{(m+1)(m+2)}\quad \text {for } i<j \end{aligned}$$

Summing over the first n out of m order statistics—that is, the n most efficient firms—yields the expressions stated in the lemma.

Pairing eq. (6) with Lemma A1, we find:

$$\begin{aligned} {{\,\textrm{E}\,}}_{\varvec{c}}[{\mathcal {W}}_{\text {tot}}] ={}&\eta _1+\eta _2\sum _{i=1}^n{{\,\textrm{E}\,}}_{\varvec{c}}[c_i]+\eta _3\sum _{i=1}^n{{\,\textrm{E}\,}}_{\varvec{c}}[c_i^2]+\eta _4\sum _{i=1}^n\sum _{j\ne i}{{\,\textrm{E}\,}}_{\varvec{c}}[c_i c_j] \nonumber \\ ={}&\eta _1 + \eta _2\frac{n(n+1)}{2(m+1)}+\eta _3\frac{n(n+1)(n+2)}{3(m+1)(m+2)}+\eta _4\frac{n(n-1)(n+1)(n+2)}{4(m+1)(m+2)} \nonumber \\ ={}&n\cdot \{24(2-\gamma )^2(m+1)(m+2)[2+\gamma (n-1)]^2\}^{-1}\nonumber \\*&\cdot \bigg [12\alpha (2-\gamma )^2(m+2)[3+\gamma (n-1)][\alpha (m+1)-(n+1)] \nonumber \\&{}+4 (n+1) (n+2) \{12+ \gamma [\gamma (13-2 \gamma )+(3-\gamma )n[4+\gamma (n-3)]-24]\} \nonumber \\&{}-3 \gamma (n-1) (n+1) (n+2) \{8+\gamma [\gamma +(3-\gamma )n-6]\}\bigg ], \end{aligned}$$

(11)

for arbitrary parameters satisfying $0\le \gamma \le 1\le n\le m\le \alpha$. The numerator is a polynomial of fifth degree in n, the denominator one of second degree. We hence cannot find a general solution for the welfare-maximizing value of n by the Abel-Ruffini Theorem. To prove existence of interior solutions, consider the case of $\alpha =m=10, \gamma =1$. We obtain ${{\,\textrm{E}\,}}_{\varvec{c}}[{\mathcal {W}}_{\text {tot}}]=[n(n+2)(156\,965+n\{(2n+7)n-1\,430\})][3\,168(n+1)^2]^{-1}$, which can be maximized numerically.¹⁵ There are five values for $n^*$ satisfying $\frac{{\text {d\!}}{}}{{\text {d\!}}{}n}{{\,\textrm{E}\,}}_{\varvec{c}}[{\mathcal {W}}_{\text {tot}}]|_{n^*}=0$. Ignoring the solutions $n^*_1<0$, $n^*_2>m$ as well as complex values $n^*_3$, $n^*_4$ s.t. ${\text {Im}}(n^*_3)$, ${\text {Im}}(n^*_4)\ne 0$, we obtain the interior optimum $n^*_5\approx 5.38$, noting that $1<n^*_5<m$. Computing the second order derivative, it is readily verified that $n^*_5$ is indeed a maximizer.

To verify that consumers benefit from an increase in competition, consider $\frac{{\text {d\!}}{}}{{\text {d\!}}{}n}{{\,\textrm{E}\,}}_{\varvec{c}}[{\mathcal {W}}_{\text {C}}(n)]$ and note that it is increasing in both $\alpha$ and m. Hence, let $\alpha =m=n$ to see that the derivative is positive for all $0\le \gamma \le 1\le n$. $\square$

Proof of Lemma 3

We first derive consumer demand $q_i$ given prices $\varvec{p}$. Differentiating and summing over all firms yields $q_i=\xi _1-\xi _2 p_i+\xi _3 \sum _{j\ne i}p_j$ with coefficients

$$\begin{aligned} \xi '=\{(1-\gamma )[1+\gamma (n-1)]\}^{-1},\quad \xi _1=\xi '\alpha (1-\gamma ),\quad \xi _2=\xi '[1+\gamma (n-2)],\quad \xi _3=\xi '\gamma . \end{aligned}$$

Firms anticipate this demand when setting their prices. Firm i chooses $p_i$ to maximize its profit given by $\pi _i=q_i(p_i-c_i)=(\xi _1-\xi _2 p_i+\xi _3\sum _{j\ne i}p_j)(p_i-c_i)$. Summing the respective first order conditions over all firms and rearranging yields a price $p_i=\xi _4+\xi _5 c_i+\xi _6 \sum _{j\ne i}c_j$ with coefficients

$$\begin{aligned} \xi ''&{}=\{[2+\gamma (n-3)][2+\gamma (2n-3)]\}^{-1},\quad&\xi _4&{}=\xi ''\alpha (1-\gamma )[2+\gamma (2n-3)], \\ \xi _5&{}=\xi ''[1+\gamma (n-2)][2+\gamma (n-2)],&\xi _6&{}=\xi ''\gamma [1+\gamma (n-2)]. \end{aligned}$$

Combining both, we obtain consumer demand given $\varvec{c}$, given by $q_i=\xi _7-\xi _8 c_i+\xi _9 \sum _{j\ne i}c_j$ with coefficients

$$\begin{aligned} \xi _7=\xi '\xi _4[1+\gamma (n-2)],\quad{} & {} \xi _8=\xi ''\xi _2\{2+\gamma (-6+5\gamma +n[3+\gamma (n-2)])\},\\ {}{} & {} \xi _9=\xi '\xi _6[1+\gamma (n-2)]. \end{aligned}$$

We now turn to positivity of the equilibrium. Note that all coefficients $\xi _1,\ldots ,\xi _9$ are weakly positive. Since $\xi _4>0$ (recall that $\gamma <1$ in the Bertrand setting), prices are trivially strictly positive. To ensure positivity of demand, note that $q_i\ge \xi _7-\xi _8\,\forall \varvec{c}$. Hence, a sufficient condition is $\frac{\xi _7}{\xi _8}>1$, which is equivalent to

$$\begin{aligned} \alpha \ge \frac{2+\gamma (-6+5\gamma +n[3+\gamma (n-2)])}{(1-\gamma )[2+\gamma (2n-3)]}\equiv \alpha ^*(n,\gamma ). \end{aligned}$$

Note that $\frac{\partial \alpha ^*}{\partial n}\ge 0\,\forall \gamma \in {[0,1)}$. Since the model requires $n\le m$, it suffices to have $\alpha >\alpha ^*(m,\gamma )\equiv {\underline{\alpha }}$. We hence conclude that

$$\begin{aligned} \forall m\in {\mathbb {N}}_+,\gamma \in {[0,1)}\ \exists \,{\underline{\alpha }}>0 \text { s.t.:}\quad&\alpha>{\underline{\alpha }} \Rightarrow p_i, q_i>0\nonumber \\&\forall i,n,\varvec{c}\text { s.t. } 1\le i\le n\le m, \varvec{c}\in {[0,1]}^m, \nonumber \\ \text {with}\quad&{\underline{\alpha }}=\frac{2+\gamma (-6+5\gamma +m[3+\gamma (m-2)])}{(1-\gamma )[2+\gamma (2m-3)]}. \end{aligned}$$

(12)

We make three remarks. First, let $\alpha ={\underline{\alpha }}$. All firms still post strictly positive prices and have strictly positive demand, unless the polar case $c_i=1, c_j=0\,\forall j\ne i$ and $n=m$ occurs. Here, the demand for firm i goes to zero. Second, note there is no finite lower bound on $\alpha$ ensuring positive quantities for all degrees of product differentiation, as opposed to the case of Cournot competition. This follows from $\frac{\partial {\underline{\alpha }}}{\partial \gamma }\ge 0$ and $\lim _{\gamma \rightarrow 1}{\underline{\alpha }}=\infty$. Third, sufficiency in a Bertrand setting neither implies nor requires sufficiency in a Cournot setting. That is, $\alpha \ge {\underline{\alpha }}$ does not necessarily imply $\alpha \ge m$ or vice versa. This is readily seen from ${\underline{\alpha }}|_{\gamma =0}=1$ and $\lim _{\gamma \rightarrow 1}{\underline{\alpha }}=\infty \,\forall m\in {\mathbb {N}}_+$.

Next, we turn to firm profits. In particular, we want to ascertain that $\pi _i=q_i(p_i-c_i)$ is positive for every firm and every profile of costs. We have already identified a condition ensuring that $q_i>0$. Hence, we only need to check whether $p_i\ge c_i$ holds for all $\varvec{c}\in {[0,1]}^m$. Recalling the equilibrium price above, this is equivalent to

$$\begin{aligned} \xi _4+(\xi _5-1)c_i+\xi _6\sum _{j\ne i}c_j\ge 0. \end{aligned}$$

Noting that $\xi _5-1\le 0$ and $\xi _6\ge 0$, this expression is bounded below by setting $c_i=1$ and $c_j=0\,\forall j\ne i$. The requirement thus is satisfied if $\frac{\xi _4}{1-\xi _5}\ge 1$, which holds true if $\alpha \ge {{\underline{\alpha }}}$. To see that each firm’s profit decreases in its marginal costs, consider

$$\begin{aligned} \frac{{\text {d\!}}{}\pi _i}{{\text {d\!}}{}c_i}&{}=\frac{{\text {d\!}}{}q_i}{{\text {d\!}}{}c_i}(p_i-c_i)+q_i(\frac{{\text {d\!}}{}p_i}{{\text {d\!}}{}c_i}-1)\\&{}=-\xi _8(p_i-c_i)+q_i(\xi _5-1)\le 0, \end{aligned}$$

using $\xi _8\ge 0$, $p_i-c_i\ge 0$, $q_i\ge 0$ and $\xi _5-1\le 0$ from above for $\alpha \ge {{\underline{\alpha }}}$.

Proof of Proposition 3

The proposition states that:

The proof mirrors the Cournot case. Only differences are the market equilibrium $(\varvec{q},\varvec{p})$, where prices and quantities are now as derived in Lemma 3, as well as the quality threshold ${{\underline{\alpha }}}$ now defined by eq. (12). We compute total welfare given a profile of costs $\varvec{c}$:

(13)

Using the expected order statistics from Lemma A1, we obtain

$$\begin{aligned} {{\,\textrm{E}\,}}_{\varvec{c}}[{\mathcal {W}}_{\text {tot}}^{\text {B}}] ={}&\{24(1-\gamma ) (m+1) (m+2)[2+\gamma (n-3)]^2[1+\gamma (n-1)][2+\gamma (2 n-3)]^2\}^{-1}\nonumber \\*&\cdot n[1+\gamma (n-2)]\bigg [3n^6\gamma ^4+n^5\gamma ^3 (30-31 \gamma )\nonumber \\&{}+ n^4\gamma ^2(\gamma (64 \gamma +48 \alpha (\gamma -1) (m+2)-175)+99) \nonumber \\&{}- n^3\gamma \Big \{\gamma \big (\gamma (69-151 \gamma )+48 \alpha ^2 (\gamma -1) \gamma (m+1) (m+2)\nonumber \\&{}+48 \alpha (\gamma -1) (6 \gamma -5) (m+2)+192\big )-120\Big \} \nonumber \\&{}+n^2 \Big \{\gamma \big (\gamma ((965-379 \gamma ) \gamma -723)+48 \alpha ^2 (\gamma -1) \gamma (7 \gamma -5) (m+1) (m+2)\nonumber \\&{}+12 \alpha (\gamma -1) (\gamma (29 \gamma -60)+28) (m+2)+96\big )+48\Big \}\nonumber \\&{}-3 n \Big \{(4 \gamma -3) (\gamma (\gamma (7 \gamma +20)-40)+16)+4 \alpha ^2 (\gamma -1) \nonumber \\&{}\gamma (3 \gamma -2) (19 \gamma -14) (m+1) (m+2) -4 \alpha (\gamma -1) (3 \gamma -2) (\gamma (7 \gamma +3)-6) (m+2)\Big \}\nonumber \\&{}+2 (3 \gamma -2) \Big \{\gamma (\gamma (58 \gamma -129)+96)+6 \alpha ^2 (\gamma -1) (3 \gamma -2) \nonumber \\ {}&{}(4 \gamma -3) (m+1) (m+2) -6 \alpha (\gamma -1) (3 \gamma -2) (4 \gamma -3) (m+2)-24\Big \}\bigg ]. \end{aligned}$$

(14)

Again, no explicit solution exists for general parameters $0\le \gamma \le 1\le n\le m\le \alpha$. To show the existence of an interior solution $1<n^*<m$, we considers the case $\alpha =90$, $\gamma =0.9$ and $m=20$. Computing the extreme values numerically and excluding all those which are either below 1, above m or whose imaginary part is nonzero, we obtain $n^*\approx 16.898$, with the second order derivative verifying that it is a maximum indeed.

Proof of Lemma 4

We introduce the following notation. We refer to the firms by the order statistics of their marginal costs $c_{(1)}\le \ldots \le c_{(m)}$, such that the index (i) signifies the i-th most efficient firm. Denote by $[\varvec{c}]^n$ the set of subsets with n elements, i.e. the possible combinations of firms which can be active in a market of size n. $\pi _{(i)}(\varvec{c}_n)$ is the profit of firm i in a market where the firms $\varvec{c}_n\in [\varvec{c}]^n$ compete. Note that this profit is equal to zero if $c_{(i)}\notin \varvec{c}_n$ (i.e. if the firm is not active in the market) and that it is strictly positive otherwise by Lemma 1 apart from the polar case discussed in Footnote 8, when it is zero. Recall that this polar case as well as a cost realization with $c_{(i)}=c_{(j)}$ for $i\ne j$ have zero probability mass due to m being finite and can be covered using a tie breaking rule. Finally, for each of the $k\in \{1,\ldots ,n\}$ sequential auctions, denote by $w(k)\in \{1,\ldots ,m\}$ the firm winning this auction, identified by the firm’s order statistic (i). Since bids are public and a second-price auction is played each round, a bidding strategy for round k can be contingent on $w(k')$ for all $k'<k$.

We now construct a profile of bidding strategies as follows: in equilibrium, the k-th auction will be won by the k-th most efficient firm, i.e. $w(k)=k$ for all $k\in \{1,\ldots ,n\}$, such that the n most efficient firms will indeed each hold one item (“license”) each. In each round k, we therefore check whether for all past auctions $k'<k$ the $k'$-th round was won by the $k'$-th most efficient firm, i.e. whether $w(k')=k'$ for all $k'<k$.

On-path strategies: Let all firms $i\le n$ bid their on-equilibrium market valuation given by $\pi _{(i)}(c_{(1)},\ldots ,c_{(n)})$, while the remaining firms $i>n$ bid the off-equilibrium valuation of them entering the market instead of firm (n), i.e. instead of the least efficient active firm: $\pi _{(i)}(c_{(1)},\ldots ,c_{(n-1)},c_{(i)})$. Finally, since there is a cap of one item per bidder, firms having won an item (i.e. in round k all firms i s.t. $\exists k'<k$ with $w(k')=i$) in a previous round bid zero in the future.

Recall that a firm’s profit decreases in its marginal costs and hence for all $\varvec{c}_n$ we have $\pi _{(i)}(\varvec{c}_n)\ge \pi _{(j)}(\varvec{c}_n)$ if and only if $i\le j$. For the same reason it holds that for all ${\textbf{c}}$ and $i>n$: $\pi _{(n)}(c_{(1)},\ldots ,c_{(n)})\ge \pi _{(i)}(c_{(1)},\ldots ,c_{(n-1)},c_{(i)})$. Note that in the latter statement we compare two different profiles of active firms while in the first the firms are the same. Combining these inequalities we conclude that, ceteris paribus, a more efficient firm always has a higher valuation and places a higher bid if it follows the strategies above. Using these strategies, the k-th auction will be won by the k-th most efficient firm and the desired outcome is implemented.

Off-path strategies: We now need to ensure that more efficient firms have no incentive to initially lower their bids in order to wait for rounds when only very inefficient rivals are still bidding, providing them with much lower second-highest bids of their rivals. The most efficient firm, for example, could obtain the n-th license at a price much lower than the first license without running the risk of not obtaining a license at all. To avoid this, we now define bids for the case where in some past round $k'$ a different firm than the $k'$-th most efficient has placed the highest bid, i.e. if in round $k\ \exists k'<k \text { s.t.\ } w(k')\ne k'$. Using the notation introduced above, $\pi _{(1)}(c_{(1)})$ denotes the monopoly profit of the most efficient firm. Given a cost profile $\varvec{c}$, this value is an upper limit for the possible profits across firms i and market market sizes n.¹⁶ Now for every round after some $k'\ne w(k')$, all firms with $i< n$ who do not yet hold a license bid $\pi _{(1)}(c_{(1)})$, while all firms with $i>n$ bid zero. Firm n bids the monopoly profit if the deviant is among the more efficient firms, i.e. if $w(k')<n$, while it bids zero otherwise, i.e. if a firm that was not supposed to enter the market in equilibrium has done so.

This grim trigger strategy deters deviations in two ways. First, it prevents more efficient firms ($i<n$) from postponing their bids in order to win later, cheaper rounds. If they do not acquire their item in the round they are supposed to, they will only be able to do so at a price equal to the monopoly profit, making a later entry unattractive. Second, it also prevents overbidding of less efficient firms ($i>n$). Initially, none of these firms have an incentive to acquire a license: in any round $k\le n$, a firm $i>n$ would be paying a price $\pi _{(k)}(c_{(1)},\ldots ,c_{(n)})$, which is greater than its own profit if the profile of competitors remained unchanged. However, if all firms—instead of only the remaining $n-k$ most efficient ones—would then move to monopoly bids, the deviating firm i could hope that a tie breaking rule will cause less efficient firms ($j>n$) to enter, increasing its own profit beyond the price initially paid. The off-path strategies above ensure that if a less efficient firm enters the market it will nevertheless only face the $n-1$ most efficient rivals, making overbidding unattractive.

Proof of Proposition 4

Using strategies and notation from Lemma 4, we can compute the total auction revenue R and compare it to total firm profits:

$$\begin{aligned} R(n)&{}=\sum _{i=2}^n\pi _{(i)}(c_{(1)},\ldots ,c_{(n)})+\pi _{(n+1)}(c_{(1)},\ldots ,c_{(n-1)},c_{(n+1)})\nonumber \\&{}={\mathcal {W}}_{\text {F}}-\pi _{(1)}(c_{(1)},\ldots ,c_{(n)})+\pi _{(n+1)}(c_{(1)},\ldots ,c_{(n-1)},c_{(n+1)}). \end{aligned}$$

(15)

Now the proposition can be written as follows:

Recall that for all firms i and all cost profiles $\varvec{c}$ it holds that $\pi _i(\varvec{c})=[q_i(\varvec{c})]^2$. We can therefore rewrite the profit of the most efficient firm by separating the effect of $c_1$, $(c_2,\ldots ,c_{n-1})$ and $c_n$:

$$\begin{aligned}&\pi _{(1)}(c_{(1)},\ldots ,c_{(n)})\nonumber \\&=\lambda ^2\left\{ \alpha ^2(2-\gamma )^2-2\alpha (2-\gamma )[2+\gamma (n-2)]c_{(1)}+2\alpha (2-\gamma )\gamma c_{(n)}\right. \nonumber \\&\quad {}+2\alpha (2-\gamma )\gamma \sum _{i=2}^{n-1}c_{(i)}+[2+\gamma (n-2)]^2 c_{(1)}^2+\gamma ^2 c_{(n)}^2+\gamma ^2\sum _{i=2}^{n-1}c_{(i)}^2\nonumber \\&\quad {}-2[2+\gamma (n-2)]\gamma c_{(1)}c_{(n)}-2[2+\gamma (n-2)]\gamma c_{(1)}\smash {\sum _{i=2}^{n-1}}c_{(i)}\nonumber \\&\quad \left. {}+2\gamma ^2c_{(n)}\sum _{i=2}^{n-1}c_{(i)}+\gamma ^2\sum _{i=2}^{n-1}\sum _{j=2, j\ne i}^{n-1}c_{(i)}c_{(j)}\right\} \end{aligned}$$

(16)

The bid of the $(n+1)$-th most efficient firm is the second highest in the n-th auction and hence is paid by the n-th most efficient firm for obtaining the last license. Firm $(n+1)$ bids the profit it would generate if it was to enter the market instead of firm (n), the other rivals’ costs remaining the same. We directly obtain $\pi _{(n+1)}(c_{(1)},\ldots ,c_{(n-1)},c_{(n+1)})$ from eq. (16) by replacing $c_{(1)}$ with $c_{(n+1)}$ and $c_{(n)}$ with $c_{(1)}$. This allows us to write the total auction revenue:

(17)

Adding consumer surplus and using the order statistics, we obtain:

(18)

for arbitrary parameters satisfying $0\le \gamma \le 1\le n\le m\le \alpha$. Just as in the proof of Proposition 2, we observe that the numerator is a polynomial of fifth degree in n, the denominator one of second degree. We hence cannot find a general solution for the welfare-maximizing value of n by the Abel-Ruffini Theorem. To prove existence of interior solutions, again consider the case of $\alpha =m=10, \gamma =1$. We obtain ${{\,\textrm{E}\,}}_{\varvec{c}}[{\mathcal {W}}_{\text {C}}+R(n)]=(2n^5+11n^4-1\,368n^3+148\,465n^2+308\,146n+5712)(3\,168(n+1)^2)^{-1}$, which can be maximized numerically. There are five values for $n^*$ satisfying $\frac{{\text {d\!}}{}}{{\text {d\!}}{}n}{{\,\textrm{E}\,}}_{\varvec{c}}[{\mathcal {W}}_{\text {C}}(n)+R(n)]|_{n^*}=0$. Ignoring the solutions $n^*_1<0$, $n^*_2>m$ as well as complex values $n^*_3$, $n^*_4$ s.t. ${\text {Im}}(n^*_3)$, ${\text {Im}}(n^*_4)\ne 0$, we obtain the interior optimum $n^*_5\approx 5.10$, noting that $1<n^*_5<m$. Computing the second order derivative, it is readily verified that $n^*_5$ is indeed a maximizer.

Lemma A2

If firms are heterogeneous in quality, it can be welfare-optimal to limit competition.

Proof of Lemma A2

Let each firm’s quality level $\alpha _i$ be separable into a common “base” quality $\alpha$ and a private margin $x_i\sim U{[0,1]}$, such that $\alpha _i=\alpha -x_i$. We denote a quality profile by $\varvec{x}=(x_1,\ldots ,x_m)$. With such individual values for quality, consumer utility is given by

$$\begin{aligned} U(\varvec{q})=\sum _{i=1}^n \alpha _i q_i -\frac{1}{2}\bigg (\sum _{i=1}^n q_i^2+\gamma \sum _{i=1}^n\sum _{j\ne i}q_i q_j\bigg ). \end{aligned}$$

(19)

For simplicity, we assume all firms’ marginal cost to be zero, i.e. $c_i=0\,\forall i$. Häckner (2000) derives firm prices and consumer demand under Cournot competition:

$$\begin{aligned} p_i=q_i=\frac{[\gamma (n-2)+2]\alpha _i-\gamma \sum _{j\ne i}\alpha _j}{(2-\gamma )[\gamma (n-1)+2]} \end{aligned}$$

(20)

We need to ascertain that all firms find market entrance profitable. We can ensure positivity of prices and quantities by requiring a sufficiently high base quality $\alpha$. A sufficient condition is $\alpha \ge m$, i.e.:

$$\begin{aligned} \alpha \ge m \Rightarrow p_i, q_i\ge 0\quad \forall i,n,m,\gamma \,\text { s.t. } 1\le i\le n\le m, (\varvec{x},\gamma )\in {[0,1]}^{m+1}. \end{aligned}$$

(21)

To see this, We first look at the denominator of eq. (20). For $n=1$, its positivity is trivial. For $n>1$, the denominator is concave in $\gamma$ and positive for $\gamma \in \{0,1\}$, thus for all $\gamma \in {[0,1]}$. The numerator is increasing in $\alpha _k$ for $k=i$ and decreasing for $k=j\ne i$. By assumption, we know $\alpha \ge m$ and $x_i\in {[0,1]}$. Hence

$$\begin{aligned}{}[\gamma (n-2)+2]\alpha _i-\gamma \smash {\sum _{j\ne i}}\alpha _j&{}= (2-\gamma )\alpha -[\gamma (n-2)+2]x_i+\gamma \smash {\sum _{j\ne i}}x_j \\&{}\ge (2-\gamma )\alpha -[\gamma (n-2)+2] \\&{}\ge (2-\gamma )m-[\gamma (m-2)+2] \\&{}\ge 0, \end{aligned}$$

with equality only if $n=m=\alpha$. We can now state Lemma A2 formally:

Using $\alpha _i=\alpha -x_i$, we can rewrite the production levels $q_i$ in the market equilibrium given by eq. (20). Recall the definition $\lambda =\{(2-\gamma )[2+\gamma (n-1)]\}^{-1}$ to obtain

$$\begin{aligned} q_i=\lambda \Big [\alpha (2-\gamma )-[2+\gamma (n-2)]x_i+\gamma \sum _{j\ne i}x_j\Big ], \end{aligned}$$

(22)

which mirrors the equilibrium output in the case of cost heterogeneity from eq. (2) with costs $c_i$ replaced by marginal quality $x_i$. Total welfare in the case of quality heterogeneity is given by

$$\begin{aligned} {\mathcal {W}}_{\text {tot}}^{\alpha _i} =U(\varvec{q})&{}=\alpha \sum _{i=1}^n q_i -\frac{1}{2}\sum _{i=1}^n q_i^2-\frac{\gamma }{2}\sum _{i=1}^n\sum _{j\ne i}q_i q_j -\sum _{i=1}^n q_i x_i, \end{aligned}$$

(23)

which in turn mirrors the expression for total welfare in the case of cost heterogeneity from eq. (6). But since $x_i$ and $c_i$ are both i.i.d. draws from U[0, 1], the distributions of their order statistics coincide. Hence, all results for the expected value ${{\,\textrm{E}\,}}_{\varvec{c}}[{\mathcal {W}}_{\text {tot}}]$ directly carry over to ${{\,\textrm{E}\,}}_{\varvec{x}}[{\mathcal {W}}_{\text {tot}}^{\alpha _i}]$ and we can apply Proposition 2. That is, from the regulator’s perspective, expected total welfare is given by eq. (11) and the same interior maxima exist.