Investigation on the rudder force of a ship in large drifting conditions with the MMG model

Abstract

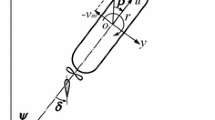
The rudder force, including interference with a ship hull in large drifting conditions, is investigated in this study by captive model tests using a ship model with steering. In the tests, hydrodynamic forces acting on the ship, the rudder normal force, and propeller thrust are measured while changing the hull drift angle $\beta$ in the range of $-180^{\circ } \sim 180^{\circ }$, which encompasses forward, lateral, and astern motion. The hull and rudder interaction coefficients, thrust deduction factor, inflow velocity to propeller, and inflow velocity to rudder are obtained in large drifting conditions using the measured hydrodynamic force data. The Maneuvering Model Group (MMG) model (Yasukawa and Yoshimura, 2015) is applicable for estimating the rudder force when the absolute value of $\beta$ is smaller than $45^\circ$. In this region, the hydrodynamic force parameters for the inflow velocity to the rudder are nearly constant for any hull drift angle. However, in the region where the absolute value of $\beta$ is greater than nearly $45^\circ$, the MMG model cannot be applied. For improving the formula for the longitudinal inflow velocity component to the rudder ($u_R$), we propose a new formula that is applicable to any hull drift angle.

Appendix: Derivation of an equation of \(u_R\)

In this appendix, we derive an equation for $u_R$ that is applicable for any hull drift angle. As shown in Fig. 21, there exist two flow fields around the rudder. One is the area where the propeller slip stream makes contact, and the other is the area where the propeller slip stream does not make contact. Here, the representative longitudinal components of the inflow velocity to the rudder area where the propeller slip stream makes contact and does not make contact are denoted $u_{RP}$ and $u_{R0}$, respectively. Similarly, the lateral inflow components are denoted as $v_{RP}$ and $v_{R0}$.

Fig. 21

Schematic diagram of flow around the rudder

The representative longitudinal component of the inflow velocity to the rudder $u_R$ is assumed to be expressed as,

$$\begin{aligned} u_R= \,\,& {} (A_{RP}/A_R) u_{RP} + (A_{R0}/A_R)u_{R0}. \end{aligned}$$

(29)

Here, $A_{RP}$ is the rudder area where the propeller slip stream makes contact and $A_{R0}$ is the rudder area where the propeller slip stream does not make contact. $A_R$ is the rudder total area expressed as the sum of $A_{RP}$ and $A_{R0}$. The $u_R$ is expressed by weighting two velocity components $u_{RP}$ and $u_{R0}$ with respect to the respective rudder areas. If the rectangular rudder is assumed, $(A_{RP}/A_R)$ can be approximated as $(D_P/H_R)$ where $D_P$ is the propeller diameter and $H_R$ is the rudder span length. Therefore, $u_R$ is expressed in the following form:

$$\begin{aligned} u_R & \simeq (D_P/H_R) u_{RP} + (1-D_P/H_R)u_{R0} \nonumber \\&= \eta u_{RP} + ( 1 - \eta ) u_{R0}, \end{aligned}$$

(30)

where $\eta$ is $D_P/H_R$. We consider Eq. (30) as a basic form of $u_R$.

The $u_{R0}$ is expressed as

$$\begin{aligned} u_{R0} =(1-w_R)u, \end{aligned}$$

(31)

where $w_R$ is the wake fraction at the rudder position. Note that $w_R$ should be zero for $|\beta | > 90^\circ$. According to the MMG model [1], $u_{RP}$ is expressed as,

$$\begin{aligned} u_{RP} = u_{R0} + k_x \varDelta u, \end{aligned}$$

(32)

where $k_x \varDelta u$ represents a velocity increase due to the propeller slip stream. $\varDelta u$ is defined in terms of the difference between $u_{\infty }$ and the inflow velocity to propeller $u_P$ ($\varDelta u = u_{\infty } - u_P$), where $u_{\infty }$ is the velocity at the infinite aft position.

The $u_{\infty }$ is expressed as [1]

$$\begin{aligned} u_{\infty } = u_P{\text {sgn}}(u) \sqrt{1 + \displaystyle \frac{8K_T}{\pi J_P^2}}. \end{aligned}$$

(33)

Substituting Eqs. (31) and (33) into Eq. (32) yields the following:

$$\begin{aligned} u_{RP}= \,\,& {} (1-w_R)u + k_x u_P\left\{ {\text {sgn}}(u) \sqrt{1 + \displaystyle \frac{8K_T}{\pi J_P^2}} -1\right\} . \end{aligned}$$

(34)

Furthermore, substituting Eqs. (31) and (34) into Eq. (30) yields the following:

$$\begin{aligned} u_R= \,\,& {} u_P\varepsilon _n \left\{ \eta \kappa _n \left( {\text {sgn}}(u) \sqrt{1 + \displaystyle \frac{8K_T}{\pi J_P^2}} -1\right) + 1 \right\} , \end{aligned}$$

(35)

where $\varepsilon _n=(1-w_R)/(1-w_P)$, and $\kappa _n=k_x/\varepsilon _n$. By moving $u_P$ to the left-hand side of the equation, the boosting ratio of the inflow velocity $u_R/u_P$ is obtained as follows:

$$\begin{aligned} \frac{u_R}{u_P} = \varepsilon _n \left\{ \eta \kappa _n \left( {\text {sgn}}(u) \sqrt{1 + \frac{8K_T}{\pi J_P^2}} - 1 \right) + 1\right\} . \end{aligned}$$

(36)