A novel nonlinear isolated rooftop tuned mass damper-inerter (IR-TMDI) system for seismic response mitigation of buildings

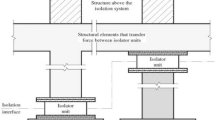
Abstract

This paper conceptualizes a novel passive vibration control system comprising a tuned mass damper-inerter (TMDI) contained within a seismically isolated rooftop and investigates numerically its effectiveness for seismic response mitigation of building structures. The working principle of the proposed isolated rooftop tuned mass damper-inerter (IR-TMDI) system relies on the yielding of typical elastomeric isolators (e.g. lead rubber bearings) under severe earthquake ground motions to create a flexible rooftop which, in turn, increases the efficacy of the TMDI for seismic vibrations suppression. Herein, a nonlinear mechanical model is considered to explore the potential of IR-TMDI whereby the primary building structure is taken as linear damped single-mode system, while the Bouc–Wen model is used to capture the nonlinear/hysteretic behaviour of the rooftop isolators. An equivalent linear system (ELS), derived through statistical linearization, is used to expedite the optimal IR-TMDI tuning for different isolated rooftop properties, inertance, and primary structure natural period. To this aim, white noise excitations with different intensities as well as Kanai–Tajimi excitations for different soil conditions are considered. It is found that tuning for maximizing TMDI seismic energy dissipation is more advantageous than tuning for minimizing primary structure displacement or acceleration response since it lowers deflection and force demands to the isolators and to the inerter. Further, significant primary structure displacement and acceleration reductions are achieved as the effective rooftop flexibility increases through reduction of the nominal strength of the isolators, which verifies the intended working principle of the IR-TMDI. This is also confirmed through response history analyses to the nonlinear model under benchmark recorded ground motions. Moreover, for IR-TMDI with sufficiently flexible isolators, improved seismic structural performance with concurrent reduced deflection and force demands at the isolators is shown for all considered stochastic excitations as the inertance scales-up, which is readily achievable technologically. Thus, it is concluded that the IR-TMDI mitigates effectively structural seismic response without requiring the inerter to span several floors, as suggested in previous studies, thus extending the TMDI applicability to both existing and low-rise new-built structures.

Appendix 1: Energy dissipation index derivation

In this Appendix, the energy-based performance criterion in Eq. (24) is derived. To this aim, the equations of motion of the ELS in Eq. (16) are first written as

$$m_{s} \ddot{x}_{s} + c_{s} \dot{x}_{s} + k_{s} x_{s} - \left( {c_{i} \left( {\dot{x}_{is} - \dot{x}_{s} } \right) + \alpha k_{i} \left( {x_{is} - x_{s} } \right) + \left( {1 - \alpha } \right)F_{y} y} \right) - b\left( {\ddot{x}_{d} - \ddot{x}_{s} } \right) = - m_{s} \ddot{x}_{g}$$

(28a)

$$m_{i} \ddot{x}_{is} \; + c_{i} \left( {\dot{x}_{is} - \dot{x}_{s} } \right) + \alpha k_{i} \left( {x_{is} - x_{s} } \right) + \left( {1 - \alpha } \right)F_{y} z - \left( {c_{d} \left( {\dot{x}_{d} - \dot{x}_{is} } \right) + k_{d} \left( {x_{d} - x_{is} } \right)} \right) = - m_{i} \ddot{x}_{g}$$

(28b)

$$m_{d} \ddot{x}_{d} + c_{d} \left( {\dot{x}_{d} - \dot{x}_{is} } \right) + k_{d} \left( {x_{d} - x_{is} } \right)\; + b\left( {\ddot{x}_{d} - \ddot{x}_{s} } \right) = - m_{d} \ddot{x}_{g}$$

(28c)

Next, the so-called equations of relative energy balance are derived by multiplying Eq. (28a) by $\dot{x}_{s}$, Eq. (28b) by $\dot{x}_{is}$ and Eq. (28c) by $\dot{x}_{d}$ and integrating over time to yield (e.g. [54])

$$E_{{m_{s} }} \left( t \right) + E_{{c_{s} }} \left( t \right) + E_{{k_{s} }} \left( t \right) - E_{{c_{i,s} }} \left( t \right) - E_{{k_{i,s} }} \left( t \right) - E_{{h_{i,s} }} \left( t \right) - E_{b,s} \left( t \right) = E_{{g_{s} }} \left( t \right)$$

(29a)

$$E_{{m_{i} }} \left( t \right) + E_{{c_{i} }} \left( t \right) + E_{{h_{i} }} \left( t \right) + E_{{k_{i} }} \left( t \right) - E_{{c_{d,is} }} \left( t \right) - E_{{k_{d,is} }} \left( t \right) = E_{{g_{i} }} \left( t \right)$$

(29b)

$$E_{{m_{d} }} \left( t \right) + E_{{c_{d} }} \left( t \right) + E_{{k_{d} }} \left( t \right) + E_{b} \left( t \right) = E_{{g_{d} }} \left( t \right)$$

(29c)

In Eq. (29a), $E_{{m_{s} }}$, $E_{{c_{s} }}$, and $E_{{k_{s} }}$ are the kinetic energy, viscous damping energy, and elastic strain energy of the primary structure, respectively, given by

$$E_{{m_{s} }} \left( t \right) = m_{s} \int_{0}^{t} {\ddot{x}_{s} } \dot{x}_{s} {\text{d}}t, \, E_{{c_{s} }} \left( t \right) = c_{s} \int_{0}^{t} {\dot{x}_{s}^{2} {\text{d}}t,{\text{ and}}} \;\; \, E_{{k_{s} }} \left( t \right) = k_{s} \int_{0}^{t} {x_{s} \dot{x}_{s} {\text{d}}t} { ,}$$

(30)

$E_{{c_{i,s} }}$, $E_{{k_{i,s} }}$, and $E_{{h_{i,s} }}$ are the viscous damping energy, strain energy, and hysteretic dissipated energy transferred from the isolation system to the primary structure, respectively, given by

$$\begin{aligned} E_{{c_{i,s} }} \left( t \right) & = - c_{i} \int_{0}^{t} {\dot{x}_{s}^{2} {\text{d}}t + c_{i} \int_{0}^{t} {\dot{x}_{is} \dot{x}_{s} {\text{d}}t} } ,\quad E_{{k_{i,s} }} \left( t \right) = - \alpha k_{i} \int_{0}^{t} {x_{s} \dot{x}_{s} {\text{d}}t} + \alpha k_{i} \int_{0}^{t} {x_{is} \dot{x}_{s} {\text{d}}t} ,\quad {\text{and}}\quad \\ E_{{h_{i,s} }} \left( t \right) & = \left( {1 - \alpha } \right)k_{i} u_{y} \int\limits_{0}^{t} {y\dot{x}_{s} } {\text{d}}t, \\ \end{aligned}$$

(31)

$E_{b,s}$ is the energy transferred from the inerter to the primary structure, given by

$$E_{b,s} \left( t \right) = - b\int_{0}^{t} {\ddot{x}_{s} \dot{x}_{s} {\text{d}}t} + b\int_{0}^{t} {\ddot{x}_{d} \dot{x}_{s} {\text{d}}t} ,$$

(32)

and $\, E_{{g_{s} }}$ is the seismic excitation energy entering the primary structure, given by

$$\, E_{{g_{s} }} \left( t \right) = - m_{s} \int_{0}^{t} {\ddot{x}_{g} \dot{x}_{s} {\text{d}}t}$$

(33)

Further, in Eq. (29b), $E_{{m_{i} }}$, $E_{{c_{i} }}$, $E_{{k_{i} }}$, and $E_{{h_{i} }}$ are the kinetic energy, viscous damping energy, elastic strain energy, and hysteretic energy dissipation of the isolated rooftop, respectively, given by

$$\begin{aligned} E_{{m_{i} }} \left( t \right) & = m_{i} \int_{0}^{t} {\ddot{x}_{is} \dot{x}_{is} {\text{d}}t} ,\quad E_{{c_{i} }} \left( t \right) = c_{i} \int_{0}^{t} {\dot{x}_{is}^{2} {\text{d}}t} - c_{i} \int_{0}^{t} {\dot{x}_{s} \dot{x}_{is} {\text{d}}t} ,\quad \\ E_{{k_{i} }} \left( t \right) & = \, \alpha k_{i} \int_{0}^{t} {x_{is} \dot{x}_{is} {\text{d}}t} - \alpha k_{i} \int_{0}^{t} {x_{s} \dot{x}_{is} {\text{d}}t} \quad {\text{and }}E_{{h_{i} }} \left( t \right) = \left( {1 - \alpha } \right)k_{i} u_{y} \int\limits_{0}^{t} {y\dot{x}_{is} } {\text{d}}t, \\ \end{aligned}$$

(34)

$E_{{c_{d,is} }}$ and $E_{{k_{d,is} }}$ are the damping element energy and spring energy transferred from the TMDI to the isolated rooftop, respectively, given by

$$E_{{c_{d,is} }} \left( t \right) = - c_{d} \int_{0}^{t} {\dot{x}_{is}^{2} {\text{d}}t} + c_{d} \int_{0}^{t} {\dot{x}_{d} \dot{x}_{is} {\text{d}}t} \quad {\text{and}}\quad E_{{k_{d,is} }} \left( t \right) = - k_{d} \int_{0}^{t} {x_{is} \dot{x}_{is} {\text{d}}t} { + }k_{d} \int_{0}^{t} {x_{d} \dot{x}_{is} {\text{d}}t} ,$$

(35)

and $\, E_{{g_{i} }}$ is the seismic excitation energy entering the isolated floor system, given by

$$E_{{g_{i} }} \left( t \right) = - m_{i} \int_{0}^{t} {\ddot{x}_{g} \dot{x}_{is} {\text{d}}t}$$

(36)

In addition, in Eq. (29b), $E_{{m_{d} }}$, $E_{{c_{d} }}$, $E_{{k_{d} }}$, and $E_{b}$ are the kinetic energy, viscous damping energy, elastic strain energy, and inerter energy of the TMDI, respectively, given by

$$\begin{aligned} E_{{m_{d} }} \left( t \right) & = m_{d} \int_{0}^{t} {\ddot{x}_{d} \dot{x}_{d} {\text{d}}t} {,}\quad E_{{c_{d} }} \left( t \right) = c_{d} \int_{0}^{t} {\dot{x}_{d}^{2} {\text{d}}t} - c_{d} \int_{0}^{t} {\dot{x}_{is} \dot{x}_{d} {\text{d}}t} ,\quad \\ E_{{k_{d} }} \left( t \right) & = k_{d} \int_{0}^{t} {x_{d} \dot{x}_{d} {\text{d}}t} - k_{d} \int_{0}^{t} {x_{is} \dot{x}_{d} {\text{d}}t} ,\quad {\text{and}}\;\;\;E_{b} \left( t \right) = b\int_{0}^{t} {\ddot{x}_{d} \dot{x}_{d} {\text{d}}t} - b\int_{0}^{t} {\ddot{x}_{s} \dot{x}_{d} {\text{d}}t} , \\ \end{aligned}$$

(37)

and $\, E_{{g_{d} }}$ is the seismic excitation energy entering the TMDI, given by

$$E_{{g_{d} }} \left( t \right) = - \int_{0}^{t} {m_{d} \ddot{x}_{g} \dot{x}_{d} {\text{d}}t} .$$

(38)

Assuming Gaussian stationary stochastic seismic excitation and taking the mathematical expectation in both sides of Eqs. (29), the following set of equations are derived in a small increment of time $\Delta t$ under ergodic conditions (see also [37]

$$\begin{aligned} E\left[ {\Delta E_{{g_{s} }} } \right] & = E\left[ {\Delta E_{{m_{s} }} } \right] + E\left[ {\Delta E_{{c_{s} }} } \right] + E\left[ {\Delta E_{{k_{s} }} } \right] - E\left[ {\Delta E_{{c_{i,s} }} } \right] - E\left[ {\Delta E_{{k_{i,s} }} } \right] - E\left[ {\Delta E_{{h_{i,s} }} } \right] - E\left[ {\Delta E_{b,s} } \right] \\ E\left[ {\Delta E_{{g_{i} }} } \right] & = E\left[ {\Delta E_{{m_{i} }} } \right] + E\left[ {\Delta E_{{c_{i} }} } \right] + E\left[ {\Delta E_{{h_{i} }} } \right] + E\left[ {\Delta E_{{k_{i} }} } \right] - E\left[ {\Delta E_{{c_{d,is} }} } \right] - E\left[ {\Delta E_{{k_{d,is} }} } \right] \\ E\left[ {\Delta E_{{g_{d} }} } \right] & = E\left[ {\Delta E_{{m_{d} }} } \right] + E\left[ {\Delta E_{{c_{d} }} } \right] + E\left[ {\Delta E_{{k_{d} }} } \right] + E\left[ {\Delta E_{b} } \right] \\ \end{aligned}$$

(39)

In Eq. (39), the following incremental energy terms vanish $E\left[ {\Delta E_{{m_{s} }} } \right] = E\left[ {\Delta E_{{m_{i} }} } \right] = E\left[ {\Delta E_{{m_{d} }} } \right] = E\left[ {\Delta E_{{k_{s} }} } \right] = 0$ due to the property $E\left[ {u\dot{u}} \right] = 0$ which holds for any Gaussian temporal stochastic process u (e.g. [42]). Further, it can be shown that $E\left[ {\Delta E_{{k_{i} }} } \right] - E\left[ {\Delta E_{{k_{i,s} }} } \right] + E\left[ {\Delta E_{{k_{d} }} } \right] - E\left[ {\Delta E_{{k_{d,is} }} } \right] + E\left[ {\Delta E_{b} } \right] - E\left[ {\Delta E_{b,s} } \right] = 0$ by making use of the previous property together with the coordinate transformations x_i = x_is − x_s and x_k = x_d − x_is and some algebraic manipulation. To this end, by summing the three expressions in Eq. (39), the total expected incremental seismic input energy in the ELS system $\, E\left[ {\Delta E_{{{\text{Total}}}} } \right]$ is found as

$$\begin{aligned} \, E\left[ {\Delta E_{{{\text{Total}}}} } \right] &= E\left[ {\Delta E_{{g_{s} }} } \right] + \, E\left[ {\Delta E_{{g_{i} }} } \right] + E\left[ {\Delta E_{{g_{d} }} } \right] \\&= c_{s} \sigma_{{\dot{x}_{s} }}^{2} \Delta t + c_{d} \sigma_{{\dot{x}_{k} }}^{2} \Delta t + c_{i} \sigma_{{\dot{x}_{i} }}^{2} \Delta t + \left( {1 - \alpha } \right)k_{i} u_{y} \sigma_{{y\dot{x}_{i} }}^{2} \Delta t, \hfill \\ \end{aligned}$$

(40)

by noting that

$$\begin{aligned} & E\left[ {\Delta E_{{c_{s} }} } \right] = c_{s} E\left[ {\dot{x}_{s}^{2} } \right]\Delta t = c_{s} \sigma_{{\dot{x}_{s} }}^{2} \Delta t,\quad E\left[ {\Delta E_{{c_{i} }} } \right] - E\left[ {\Delta E_{{c_{i,s} }} } \right] = c_{i} E\left[ {\left( {\dot{x}_{s}^{{}} - \dot{x}_{is}^{{}} } \right)^{2} } \right]\Delta t = c_{i} \sigma_{{\dot{x}_{i} }}^{2} \Delta t, \\ & E\left[ {\Delta E_{{c_{d} }} } \right] - E\left[ {\Delta E_{{c_{d,is} }} } \right] = c_{d} E\left[ {\left( {\dot{x}_{d}^{{}} - \dot{x}_{is}^{{}} } \right)^{2} } \right]\Delta t = c_{d} \sigma_{{\dot{x}_{k} }}^{2} \Delta t,\quad {\text{and}} \\ & E\left[ {\Delta E_{{h_{i} }} } \right] - E\left[ {\Delta E_{{h_{i,s} }} } \right] = \left( {1 - \alpha } \right)k_{i} u_{y} E\left[ {y\left( {\dot{x}_{is} - \dot{x}_{s} } \right)} \right]\Delta t = \left( {1 - \alpha } \right)k_{i} u_{y} \sigma_{{y\dot{x}_{i} }}^{2} \Delta t \\ \end{aligned}$$

(41)

Finally, the energy dissipation index (EDI) defined by Pietrosanti et al. [37] as the ratio of the energy dissipated by the TMDI damping element over the total input energy is given as

$${\text{EDI}} = \frac{{E\left[ {\Delta E_{{c_{d} }} } \right] - E\left[ {\Delta E_{{c_{d,is} }} } \right]}}{{E\left[ {\Delta E_{{{\text{Total}}}} } \right]}}$$

(42)

for the herein considered ELS. Then, Eq. (24) follows from Eqs. (40–42).