Nonlinear analysis of sandwich plate with FG porous core and RD-CNTCFRC face sheets under transverse patch loading

Abstract

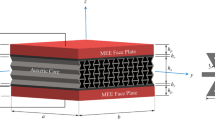
Nonlinear bending analysis of a sandwich plate with randomly distributed carbon nanotube and carbon fiber-reinforced composite (RD-CNTCFRC) face sheets and functionally graded (FG) porous core subjected to transverse patch loading is performed in the present work. The mechanical properties of the hybrid matrix, which is formed after mixing of single-walled carbon nanotubes and polymer epoxy, are estimated using Eshelby–Mori–Tanaka techniques. Subsequently, the rule of mixture technique is employed to compute the mechanical properties of RD-CNTCFRC face sheets. The mechanical properties of a functionally graded porous core are determined considering both the open-cell and closed-cell metal foam. Utilizing the mechanical properties of RD-CNTCFRC face sheets and FG porous core, the effective properties of RD-CNTCFRC porous sandwich plate are estimated. The sandwich plate is modeled based on higher-order shear deformation theory in conjunction with von Kármán geometric nonlinearity, and subsequently minimization of potential energy is employed to obtain the partial differential equations (PDEs). PDEs are solved using Galerkin’s method and reduced to nonlinear algebraic equations (NAEs). Later, these NAEs are solved via Newton–Raphson method to analyze the nonlinear bending behavior of the RD-CNTCFRC porous sandwich plate using various parameters which can help in suitable design of sandwich plates.

Appendices

Appendix A

The Fourier series expansion is adopted to derive the generalized analytical expansion for the transverse patch loading in both x- and y-directions, which is multiplied later to form a square patch loading (pressure). Transverse patch loading is applied at different locations and with different concentrations to analyze the RD-CNTCFRC porous sandwich plate for nonlinear bending behavior. The unloaded part of the plate’s width is considered as zero in the domain. As per Fig. 

Fig. 12

Generalized case of transverse patch loading in the x and y-direction

12, the functions for the general case of transverse patch loading are stated in Eqs. (85–87), where ‘${x}_{c}$’ and ‘${y}_{c}$’ are the center of loading from mid of the width in both ‘x’ and ‘y’ direction, respectively. The final form of the generalized patch loading applied as pressure on the top of the plate’s surface is obtained by multiplying the individual equations for transverse patch loading derived for x-direction and y-direction separately.

The generalized transverse loading along x-direction is given as:

$$q\left( x \right) \, = 0 - \frac{a}{2} < x < x_{c} - \frac{{a_{1} }}{2},$$

(85)

$$q\left( x \right){ } = q_{0} \cdot x_{c} - \frac{{a_{1} }}{2} < x < x_{c} + \frac{{a_{1} }}{2},$$

(86)

$$q\left( x \right) \, = 0\;x_{c} + \frac{{a_{1} }}{2} < x < \frac{a}{2}$$

(87)

where ${a}_{1}$ denotes the length of the patch loading area in the x-direction. The above transverse loading along the x-direction is expressed in the total length of the plate via Fourier series:

$$q\left( x \right){ } = { }\frac{{a_{0} }}{2} + \mathop \sum \limits_{i = 1}^{\infty } a_{i} {\text{cos}}\zeta_{i} x + \mathop \sum \limits_{i = 1}^{\infty } b_{i} {\text{sin}}\zeta_{i} x$$

(88)

where

$$\begin{aligned} a_{0} { } & = { }\frac{2}{a}\mathop \int \limits_{ - a/2}^{a/2} q_{0} {\text{d}}y, \\ a_{0} { } & = { }\frac{2}{a}\mathop \int \limits_{ - a/2}^{{x_{c} - \frac{{a_{1} }}{2}}} q_{0} {\text{d}}x + { }{ }\frac{2}{a}\mathop \int \limits_{{x_{c} - \frac{{a_{1} }}{2}}}^{{x_{c} + \frac{{a_{1} }}{2}}} q_{0} {\text{d}}x + { }\frac{2}{a}\mathop \int \limits_{{x_{c} + \frac{{a_{1} }}{2}}}^{\frac{a}{2}} q_{0} {\text{d}}x \\ & = { }\frac{2}{a}\mathop \int \limits_{{x_{c} - \frac{{a_{1} }}{2}}}^{{x_{c} + \frac{{a_{1} }}{2}}} q_{0} {\text{d}}x, \\ a_{0} { } & = { }\frac{2}{a}q_{0} a_{1}. \\ \end{aligned}$$

(89)

Again,

$$\begin{aligned} a_{i} { } & = { }\frac{2}{a}\mathop \int \limits_{ - a/2}^{\frac{a}{2}} q_{0} {\text{cos}}\zeta_{i} x {\text{d}}x = { }\frac{2}{a}\mathop \int \limits_{ - a/2}^{{x_{c} - \frac{{a_{1} }}{2}}} q_{0} {\text{cos}}\zeta_{i} x {\text{d}}x + { }\frac{2}{a}\mathop \int \limits_{{x_{c} - \frac{{a_{1} }}{2}}}^{{x_{c} + \frac{{a_{1} }}{2}}} q_{0} {\text{cos}}\zeta_{i} x {\text{d}}x + { }\frac{2}{a}\mathop \int \limits_{{x_{c} + \frac{{a_{1} }}{2}}}^{\frac{a}{2}} q_{0} {\text{cos}}\zeta_{i} x {\text{d}}x \\ & = { }\frac{2}{a}\mathop \int \limits_{{x_{c} - \frac{{a_{1} }}{2}}}^{{x_{c} + \frac{{a_{1} }}{2}}} q_{0} {\text{cos}}\zeta_{i} x{\text{d}}x ,\\ a_{i} & = { }\frac{{2q_{0} }}{{a\beta_{i} }}\left[ {{\text{sin}}\zeta_{i} \left( {x_{c} + \frac{{a_{1} }}{2}} \right) - {\text{sin}}\zeta_{i} \left( {x_{c} - \frac{{a_{1} }}{2}} \right)} \right], \\ \end{aligned}$$

(90)

Similarly,

$$\begin{aligned} b &_{i} { } = { }\frac{2}{a}\mathop \int \limits_{ - a/2}^{\frac{a}{2}} q_{0} {\text{sin}}\zeta_{i} x{\text{d}}x \\ & = { }\frac{2}{a}\mathop \int \limits_{ - a/2}^{{x_{c} - \frac{{a_{1} }}{2}}} q_{0} {\text{sin}}\zeta_{i} x{\text{ d}}x + { }\frac{2}{a}\mathop \int \limits_{{x_{c} - \frac{{a_{1} }}{2}}}^{{x_{c} + \frac{{a_{1} }}{2}}} q_{0} {\text{sin}}\zeta_{i} x dx + { }\frac{2}{a}\mathop \int \limits_{{x_{c} + \frac{{a_{1} }}{2}}}^{\frac{a}{2}} q_{0} {\text{sin}}\zeta_{i} x {\text{d}}x \\ & = { }\frac{2}{a}\mathop \int \limits_{{x_{c} - \frac{{a_{1} }}{2}}}^{{x_{c} + \frac{{a_{1} }}{2}}} q_{0} {\text{sin}}\zeta_{i} x {\text{d}}x, \\ b_{i} & = { } - \frac{{2q_{0} }}{{a\beta_{i} }}\left[ {{\text{cos}}\zeta_{i} \left( {x_{c} + \frac{{a_{1} }}{2}} \right) - {\text{cos}}\zeta_{i} \left( {x_{c} - \frac{{a_{1} }}{2}} \right)} \right]. \\ \end{aligned}$$

(91)

Thus, using (88), (89), (90), and (91), we get

$$q\left( x \right) = { }\frac{{q_{0} a_{1} }}{a} + \mathop \sum \limits_{i = 1}^{\infty } \frac{{2q_{0} }}{{a\zeta_{i} }}\left[ {{\text{sin}}\zeta_{i} \left( {x_{c} + \frac{{a_{1} }}{2}} \right) - {\text{sin}}\zeta_{i} \left( {x_{c} - \frac{{a_{1} }}{2}} \right)} \right]{\text{cos}}\zeta_{i} x - \mathop \sum \limits_{i = 1}^{\infty } \frac{{2q_{0} }}{{a\zeta_{i} }}\left[ {{\text{cos}}\zeta_{i} \left( {x_{c} + \frac{{a_{1} }}{2}} \right) - {\text{cos}}\zeta_{i} \left( {x_{c} - \frac{{a_{1} }}{2}} \right)} \right]{\text{sin}}\zeta_{i} x,$$

(92)

Similarly, the generalized transverse loading along y-direction is expressed as:

$$q\left( y \right) = { }\frac{{q_{0} b_{1} }}{b} + \mathop \sum \limits_{j = 1}^{\infty } \frac{{2q_{0} }}{{b\xi_{j} }}\left[ {{\text{sin}}\xi_{j} \left( {y_{c} + \frac{{b_{1} }}{2}} \right) - {\text{sin}}\xi_{j} \left( {y_{c} - \frac{b}{2}} \right)} \right]{\text{cos}}\xi_{j} y - \mathop \sum \limits_{j = 1}^{\infty } \frac{{2q_{0} }}{{b\xi_{j} }}\left[ {{\text{cos}}\xi_{j} \left( {y_{c} + \frac{{b_{1} }}{2}} \right) - {\text{cos}}\xi_{j} \left( {y_{c} - \frac{{b_{1} }}{2}} \right)} \right]{\text{sin}}\xi_{j} y.$$

(93)

Thus, the final generalized patch loading on the total area of the plate in the transverse direction of the RD-CNTCFRC plate is defined using Eqs. (92) and (93),

$$\begin{aligned} & q\left( {x, y} \right) = \left( \frac{{q_{0} a_{1} }}{a} + \mathop \sum \limits_{i = 1}^{\infty } \frac{{2q_{0} }}{{a\zeta_{i} }}\left[ {\sin \zeta_{i} \left( {x_{c} + \frac{{a_{1} }}{2}} \right) - \sin \zeta_{i} \left( {x_{c} - \frac{{a_{1} }}{2}} \right)} \right]\cos \zeta_{i} x \right. \\ & \quad \left. - \mathop \sum \limits_{i = 1}^{\infty } \frac{{2q_{0} }}{{a\zeta_{i} }}\left[ {\cos \zeta_{i} \left( {x_{c} + \frac{{a_{1} }}{2}} \right) - \cos \zeta_{i} \left( {x_{c} - \frac{{a_{1} }}{2}} \right)} \right]\sin \zeta_{i} x \right) \\ & \quad \times \left( \frac{{q_{0} b_{1} }}{b} + \mathop \sum \limits_{j = 1}^{\infty } \frac{{2q_{0} }}{{b\xi_{j} }}\left[ {\sin \xi_{j} \left( {y_{c} + \frac{{b_{1} }}{2}} \right) - \sin \xi_{j} \left( {y_{c} - \frac{b}{2}} \right)} \right]\cos \xi_{j} y \right.\\ &\quad -\left. \mathop \sum \limits_{j = 1}^{\infty } \frac{{2q_{0} }}{{b\xi_{j} }}\left[ {\cos \xi_{j} \left( {y_{c} + \frac{{b_{1} }}{2}} \right) - \cos \xi_{j} \left( {y_{c} - \frac{{b_{1} }}{2}} \right)} \right]\sin \xi_{j} y \right) ,\\ \end{aligned}$$

(94)

where, $\zeta_{i} = \frac{2\pi i}{a}$ and $\xi_{j} = \frac{2\pi j}{b}$.

The final expression for generalized transverse patch loading becomes

$$q\left( {x,y} \right) = \left( {\frac{{q_{0} ab}}{{a_{1} b_{1} }}} \right) \times \left( {\begin{array}{*{20}c} {\frac{{a_{1} }}{a} + \mathop \sum \limits_{i = 1}^{\infty } \frac{1}{\pi i} \left( {\sin \zeta_{i} \left( {x_{c} + \frac{{a_{1} }}{2}} \right) - {\text{sin}}\zeta_{i} \left( {x_{c} - \frac{{a_{1} }}{2}} \right)} \right)cos\zeta_{i} x } \\ { - \mathop \sum \limits_{i = 1}^{\infty } \frac{1}{\pi i} \left( {\cos \zeta_{i} \left( {x_{c} + \frac{{a_{1} }}{2}} \right) - {\text{cos}}\zeta_{i} \left( {x_{c} - \frac{{a_{1} }}{2}} \right)} \right)sin\zeta_{i} x} \\ \end{array} } \right) \times \left( {\begin{array}{*{20}c} {\frac{{b_{1} }}{b} + \mathop \sum \limits_{j = 1}^{\infty } \frac{1}{\pi j} \left( {\sin \xi_{j} \left( {y_{c} + \frac{{b_{1} }}{2}} \right) - {\text{sin}}\xi_{j} \left( {y_{c} - \frac{{b_{1} }}{2}} \right)} \right)cos\xi_{j} y } \\ { - \mathop \sum \limits_{j = 1}^{\infty } \frac{1}{\pi j} \left( {\cos \xi_{j} \left( {y_{c} + \frac{{b_{1} }}{2}} \right) - {\text{cos}}\xi_{j} \left( {y_{c} - \frac{{b_{1} }}{2}} \right)} \right)sin\xi_{j} y} \\ \end{array} } \right)$$

(95)

where $\left( {\frac{ab}{{a_{1} b_{1} }}} \right)$ is multiplied to keep the same amount of total load in all the cases, although the size of patch loading is varied by varying the values of $a_{1}$ and $b_{1}$.

Appendix B

The nonlinear governing partial differential equations of the RD-CNTCFRC porous sandwich plate in terms of displacements (u⁰, v⁰, w⁰) and rotation ($\phi_{x}^{0}$, $\phi_{y}^{0}$) variables are given below:

$$A_{11} u_{,xx}^{0} + A_{66} u_{,yy}^{0} + \left( {A_{12} + A_{66} } \right)v_{,xy}^{0} - \left\{ {B_{11} w_{,xxx}^{0} + \left( {B_{12} + 2B_{66} } \right)w_{,xyy}^{0} } \right\} + \left( {C_{11} \phi_{x,xx}^{0} + C_{66} \phi_{x,yy}^{0} } \right) + \left( {C_{12} + C_{66} } \right)\phi_{y,xy}^{0} + \left( {A_{11} w_{,xx}^{0} + A_{66} w_{,yy}^{0} } \right)w_{,x}^{0} + \left( {A_{12} + A_{66} } \right)w_{,y}^{0} w_{,xy}^{0} 0 = 0,$$

(96)

$$\left( {A_{12} + A_{66} } \right)u_{,xy}^{0} + A_{66} v_{,xx}^{0} + A_{22} v_{,yy}^{0} - \left\{ {B_{22} w_{,yyy}^{0} + \left( {B_{12} + 2B_{66} } \right)w_{,xxy}^{0} } \right\} + \left( {C_{12} + C_{66} } \right)\phi_{x,xy}^{0} + \left( {C_{22} \phi_{y,yy}^{0} + C_{66} \phi_{y,xx}^{0} } \right) + \left( {A_{22} w_{,yy}^{0} + A_{66} w_{,xx}^{0} } \right)w_{,y}^{0} + \left( {A_{12} + A_{66} } \right)w_{,x}^{0} w_{,xy}^{0} = 0,$$

(97)

$$\begin{aligned} & B_{11} \left\{ {u_{,xxx}^{0} + w_{,x}^{0} w_{,xxy}^{0} + \left( {w_{,xx}^{0} } \right)^{2} } \right\} + B_{12} \left\{ {v_{,xxy}^{0} + u_{,xxy}^{0} + w_{,x}^{0} w_{,xyy}^{0} + w_{,y}^{0} w_{,xxy}^{0} + 2\left( {w_{,xy}^{0} } \right)^{2} } \right\} \\ & \quad + B_{22} \left\{ {v_{,yyy}^{0} + w_{,y}^{0} w_{,yyy}^{0} + \left( {w_{,yy}^{0} } \right)^{2} } \right\} \\ & \quad + 2B_{66} \left\{ {u_{,xyy}^{0} + v_{,xxy}^{0} + w_{,x}^{0} w_{,xxy}^{0} + w_{,yy}^{0} w_{,xx}^{0} + w_{,y}^{0} w_{,xxy}^{0} + \left( {w_{,xy}^{0} } \right)^{2} } \right\} - D_{11} w_{,xxxx}^{0} \\ & \quad - 2D_{12} w_{,xxyy}^{0} - D_{22} w_{,yyyy}^{0} - 4D_{66} w_{,xxyy}^{0} + E_{11} \phi_{x,xxx}^{0} + E_{12} \left( {\phi_{x,xyy}^{0} + \phi_{y,xxy}^{0} } \right) \\ & \quad + E_{22} \phi_{y,yyy}^{0} + 2E_{66} \left( {\phi_{x,xyy}^{0} + \phi_{y,xxy}^{0} } \right) \\ & \quad + w_{,xx}^{0} \left( {A_{11} q_{1} + A_{12} r_{1} + B_{11} q_{2} + B_{12} r_{2} + C_{11} q_{3} + C_{12} r_{3} } \right) \\ & \quad + w_{,x}^{0} \left( {A_{11} q_{1,x} + A_{12} r_{1,x} + B_{11} q_{2,x} + B_{12} r_{2,x} + C_{11} q_{3} ,x + C_{12} r_{3,x} } \right) - n_{xx,x} w_{,x}^{0} \\ & \quad + 2w_{,xy}^{0} \left( {A_{66} s_{1} + B_{66} s_{3} + C_{66} s_{3} } \right) + w_{,x}^{0} \left( {A_{66} s_{1,y} + B_{66} s_{3,y} + C_{66} s_{3,y} } \right) \\ & \quad + w_{,y}^{0} \left( {A_{66} s_{1,x} + B_{66} s_{3,x} + C_{66} s_{3,x} } \right) \\ & \quad + w_{,yy}^{0} \left( {A_{12} q_{1} + A_{22} r_{1} + B_{12} q_{2} + B_{22} r_{2} + C_{12} q_{3} + C_{22} r_{3} } \right) \\ & \quad + w_{,y}^{0} \left( {A_{12} q_{1,y} + A_{22} r_{1,y} + B_{12} q_{2,y} + B_{22} r_{2,y} + C_{12} q_{3,y} + C_{22} r_{3,y} } \right) = Q\left( {x,y} \right) \\ \end{aligned}$$

(98)

where $q_{1} = u_{,x}^{0} + 0.5\left( {w_{,x}^{0} } \right)^{2} ;r_{1} = v_{,x}^{0} + 0.5\left( {w_{,y}^{0} } \right)^{2} ;s_{1,x} = u_{,x}^{0} + v_{,x}^{0} + w_{,x}^{0} w_{,y}^{0}$; $q_{2} = - w_{,xx}^{0}; r_{2} = - w_{,yy}^{0};\,s_{2} =2w_{,xy}^{0}$; $q_{3} = \phi_{x,x}^{0} ;r_{3} = \phi_{y,y}^{0} ;s_{3} = \phi_{x,y}^{0} + \phi_{y,x}^{0};$

$$\begin{aligned} & \left( {C_{12} + C_{66} } \right)u_{,xy}^{0} + \left( {C_{22} v_{,yy}^{0} + C_{66} v_{,xx}^{0} } \right) + \left( {C_{12} + C_{66} } \right)w_{,x}^{0} w_{,xy}^{0} + \left( {C_{22} w_{,yy}^{0} + C_{66} w_{,xx}^{0} } \right)w_{,y}^{0} - E_{22} w_{,yyy}^{0} \\ & \quad - \left( {E_{12} + 2E_{66} } \right)w_{,xxy}^{0} + F_{66} \phi_{y,xx}^{0} + F_{22} \phi_{y,yy}^{0} + \left( {F_{12} + F_{66} } \right)\phi_{x,xy}^{0} - H_{44} \phi_{y}^{0} = 0 ,\\ \end{aligned}$$

(99)

$$\begin{aligned} & \left( {C_{11} u_{,xx}^{0} + C_{66} u_{,yy}^{0} } \right) + \left( {C_{12} + C_{66} } \right)v_{,xy}^{0} + \left( {C_{11} w_{,xx}^{0} + C_{66} w_{,yy}^{0} } \right)w_{,x}^{0} + \left( {C_{12} + C_{66} } \right)w_{,y}^{0} w_{,xy}^{0} \\ & \quad - E_{11} w_{,xxx}^{0} - \left( {E_{12} + 2E_{66} } \right)w_{,xyy}^{0} + F_{11} \phi_{x,xx}^{0} + F_{66} \phi_{x,yy}^{0} + \left( {F_{12} + F_{66} } \right)\phi_{y,xy}^{0} - H_{55} \phi_{x}^{0} = 0. \\ \end{aligned}$$

(100)