Wave boundary control method for vibration suppression of large net structures

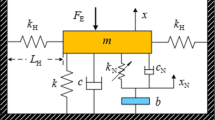
Abstract

Large net structures used in engineering can easily get into vibration under external excitations; however, the corresponding vibration control strategy still remains challenging. In this paper, a wave boundary control (WBC) strategy is proposed for the vibration suppression of large net structures. The stability of the controlled structures is confirmed by using inverse Fourier transform, transfer function analysis, and numerical simulation. When WBC controllers are set at all boundaries and excitations come from the boundaries, dynamic responses for all the strings of the net structures can quickly reduce to zero without any residual vibration. The effects of different observations, controls, and distributions of sensors on the control laws are discussed. As an application, a method for reducing the number of controllers for large net structures is finally proposed. The research provides theoretical guidance for vibration control of large net structures.

Appendices

Appendix A

Wave boundary control laws for the model in Fig. 3a:

$$\begin{aligned} U_C =\frac{U_1 }{2G^{2}},\quad U_D =\frac{U_1 }{2G^{2}}. \end{aligned}$$

(A.1)

The traveling waves at the boundary points can be expressed as

$$\begin{aligned} \left\{ {\begin{array}{l} u_{A1} =\frac{U_1 (1-2G^{2})}{2-2G^{2}},\quad u_{A2} =\frac{U_1 }{2-2G^{2}}, \\ u_{B1} =\frac{U_1 }{2-2G^{2}},\quad u_{B1} =-\frac{U_1 }{2-2G^{2}}, \\ u_{c1} =0,\quad u_{C2} =\frac{U_1 }{2G^{2}}, \\ u_{D1} =0,\quad u_{D2} =\frac{U_1 }{2G^{2}}. \\ \end{array}} \right. \end{aligned}$$

(A.2)

Thus, the frequency responses for Fig. 3a can be obtained as

$$\begin{aligned} \left\{ {\begin{array}{l} V_1 (x,\omega )=\frac{\hbox {e}^{-ikx}(1-2G^{2}+\hbox {e}^{2ikx})U_1 }{2(1-G^{2})}, \\ V_2 (x,\omega )=\frac{\hbox {e}^{-ikx}(1-\hbox {e}^{2ikx})U_1 }{2(1-G^{2})}, \\ V_3 (x,\omega )=\frac{\hbox {e}^{ikx}U_1 }{2G^{2}},\quad V_4 (x,\omega )=\frac{\hbox {e}^{ikx}U_1 }{2G^{2}}, \\ \end{array}} \right. \end{aligned}$$

(A.3)

$$\begin{aligned} V_3 (x,t)=V_4 (x,t)=\frac{1}{2}U_1 \left( {t-2\frac{l}{c}+\frac{x}{c}} \right) . \end{aligned}$$

(A.4)

Similarly, for the three controllers model in Fig. 4a, the control laws are

$$\begin{aligned} U_B =\frac{U_1 }{2G^{2}-1},\quad U_C =\frac{U_1 }{2G^{2}-1},\quad U_D =\frac{U_1 }{2G^{2}-1}. \end{aligned}$$

(A.5)

The traveling waves at boundary points for Fig. 4a can be expressed as

$$\begin{aligned} \left\{ {\begin{array}{l} u_{A1} =-\frac{2G^{2}U_1 }{1-2G^{2}},\quad u_{A2} =\frac{U_1 }{1-2G^{2}}, \\ u_{B1} =0,\quad u_{B1} =-\frac{U_1 }{1-2G^{2}}, \\ u_{c1} =0,\quad u_{C2} =-\frac{U_1 }{1-2G^{2}}, \\ u_{D1} =0,\quad u_{D2} =-\frac{U_1 }{1-2G^{2}}, \\ \end{array}} \right. \end{aligned}$$

(A.6)

The time responses for the three controllers model in Fig. 4a are as follows:

$$\begin{aligned} \left\{ {\begin{array}{l} V_1 (x,t)=-\mathop \sum \limits _{n=0}^\infty {\frac{1}{2^{n+1}}} U_1 \left( {t-\frac{2\left( {n+1} \right) l}{c}+\frac{x}{c}} \right) +\mathop \sum \limits _{n=0}^\infty {\frac{1}{2^{n}}U_1 \left( {t-\frac{2nl}{c}-\frac{x}{c}} \right) }, \\ V_2 (x,t)=V_3 (x,t)=V_4 (x,t)=\mathop \sum \limits _{n=0}^\infty {\frac{1}{2^{n+1}}} U_1 \left( {t-\frac{2\left( {n+1} \right) l}{c}+\frac{x}{c}} \right) . \\ \end{array}} \right. \end{aligned}$$

(A.7)

The time responses for the four controllers model in Fig. 4b are as follows:

$$\begin{aligned} \left\{ {\begin{array}{l} V_1 (x,t)=-\frac{i}{4kT}\left[ f_1 \left( {t-\frac{3l}{2c}+\frac{x}{c}} \right) -2f_1 \left( {t-\frac{l}{2c}+\frac{x}{c}} \right) \right] , \\ V_2 (x,t)=V_3 (x,t)=V_4 (x,t)=\frac{i}{4kT}f_1 \left( {t-\frac{3l}{2c}+\frac{x}{c}} \right) , \\ \end{array}} \right. \end{aligned}$$

(A.8)

$$\begin{aligned} \left\{ {\begin{array}{l} \alpha =(8+40G^{2}+97G^{4}+130G^{6}+96G^{8}+32G^{10}), \\ \beta =8+32G^{2}+53G^{4}+44G^{6}+16G^{8}, \\ \gamma =8+36G^{2}+60G^{4}+48G^{6}+16G^{8}, \\ \eta =8G+20G^{3}+20G^{5}+8G^{7}, \\ \lambda =12G+35G^{3}+38G^{5}+16G^{7}, \\ \psi =4G+16G^{3}+20G^{5}+8G^{7}. \\ \end{array}} \right. \end{aligned}$$

(A.9)

Appendix B

The total energy of the net structure is the sum of the kinetic and the energy of each string.

The energy of the string is as follows:

$$\begin{aligned} E(t)=\frac{1}{2}\int _0^L {(\rho \left| {V_t (x,t)} \right| ^{2}+T\left| {V_x (x,t)} \right| ^{2})} \hbox {d}x. \end{aligned}$$

(B.1)

The space interval [0, L] is the length of the string and discretized into m equally spaced panels. By applying the trapezoidal rule to each panel, the approximation to the energy of this string (Eq. (B.1)) at time $t_{q}$ becomes

$$\begin{aligned} E(t_q )=\frac{1}{2}\sum _{p=0}^{(m-1)L} {\int _{p \Delta x}^{(p +1)\Delta x} {(\rho \left| {V_t (x,t_q )} \right| ^{2}+T\left| {V_x (x,t_q )} \right| ^{2})} \hbox {d}x}. \end{aligned}$$

(B.2)

After a summation of $E(t_q )$ for all strings, we can obtain the total energy of the net structure at time $t_{q}$.

Appendix C

The expression of the displacement of an arbitrary point on the string (see Fig. 1 or Fig. 8b) corresponding to different observations is shown in Table 2.

Table 2 Displacement of an arbitrary point on the string corresponding to different observations