Performance characterization of a decoupled tri-axis micro angular rate sensor

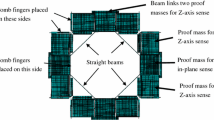
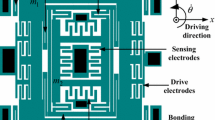
Abstract

A novel tri-axis micro-gyroscope is proposed in this paper. Three orthogonal axis angular rates, pitch, yaw, and row, can be detected simultaneously by the designed micro-gyroscope. The coupling effect of the tri-axis angular rates due to Coriolis response and nonlinearity of the high frequency modes can be efficiently reduced by the decoupled mechanical structure design. Firstly, an 8 × 8 linearized state-space representation with a holonomic constraint is established and the relative stability is investigated. Secondly, the mathematical description and discussion upon performance indices for micro-gyroscope such as sensitivity, nonlinearity, and resolution are addressed. In addition, the quality factor is verified to be a significant design parameter for the micro-gyroscope. The performance and stability of the gyroscope could be degraded by choosing inappropriate quality factors. Moreover, the trade-off among performance indices for the gyroscope is theoretically evaluated and discussed. Finally, in order to meet most performance specifications, a pole/zero mapping design method is proposed to satisfy either large bandwidth or high resolution requirements.

Appendix 1: Equations of motions

In order to simplify the analysis on the motion of each mechanical component, three coordinate frames are defined at first. The inertia frame is denoted as 〈X, Y, Z〉. Chip frame (body axis) is defined as coordinate attached on the substrate of the micro gyroscope and denoted as 〈x, y, z〉. Ring frame is defined as coordinate attached on the outer-ring and denoted as 〈U, V, W〉. According to Euler’s rotation theorem, any rotation may be described by three angles. In this paper 〈θ, ψ, ϕ〉 (pitch-roll-yaw) convention is used to describe the attitude of Chip frame corresponding to Inertia frame. To integrate motions of all mechanical components into the same coordinate so that the system dynamics of the proposed gyroscope can be uniquely described, the coordinate transforms among frames, 〈X, Y, Z〉, 〈x, y, z〉 and 〈U, V, W〉 are required. The coordinate transformation of the Chip Frame with respect to Inertia Frame is shown in Fig. 14. The rotational matrix for each rotation can be noted as

$$ R_{1} = \left[ {\begin{array}{*{20}c} {\cos \phi } & {\sin \phi } & 0 \\ { - \sin \phi } & {\cos \phi } & 0 \\ 0 & 0 & 1 \\ \end{array} } \right] $$

(27)

$$ R_{2} = \left[ {\begin{array}{*{20}c} {\cos \theta } & 0 & { - \sin \theta } \\ 0 & 1 & 0 \\ {\sin \theta } & 0 & {\cos \theta } \\ \end{array} } \right] $$

(28)

$$ R_{3} = \left[ {\begin{array}{*{20}c} 1 & 0 & 0 \\ 0 & {\cos \psi } & {\sin \psi } \\ 0 & {\sin \psi } & {\cos \psi } \\ \end{array} } \right] $$

(29)

Fig. 14

Coordinate Transform Between Chip-frame and Inertia-frame (a) yaw angle, (b) pitch angle, (c) row angle

Hence, the rotational matrix of body axis corresponding to inertial frame can be obtained by

$$ R = R_{3} R_{2} R_{1} = \left[ {\begin{array}{*{20}c} {r_{{11}} } & {r_{{12}} } & {r_{{13}} } \\ {r_{{21}} } & {r_{{22}} } & {r_{{23}} } \\ {r_{{31}} } & {r_{{32}} } & {r_{{33}} } \\ \end{array} } \right] $$

(29a)

where r ₁₁ = cos θ cos ϕ, r ₁₂ = cos θ sin ϕ, r ₁₃ = −sin θ, r ₂₁ = sin ψ sin θ cos ϕ − cos ψ sin ϕ, r ₂₂ = sin ψ sin θ sin ϕ + cos ψ sin ϕ, r ₂₃ = cos θ sin ψ, r ₃₁ = cos ψ sin θ sin ϕ + sin ψ sin ϕ, r ₃₂ = cos ψ sin θ sinϕ − sin ψ cos ϕ, r ₃₃ = cos θ cos ψ.

Assuming that the angular acceleration of the Chip-frame is zero, the angular velocity of the body frame (i.e., Chip-frame) can be expressed as

$$ \begin{aligned} \underline \omega = & \omega _{x} \hat e_{x} + \omega _{y} \hat e_{y} + \omega _{z} \hat e_{z} \\ = & ( - \dot \phi \sin \theta + \dot \psi )\hat e_{x} + (\dot \phi \cos \theta \sin \psi + \dot \theta \cos \psi )\hat e_{y} + (\dot \phi \cos \theta \cos \psi + \dot \theta \sin \psi )\hat e_{z} \\ \end{aligned} $$

(30)

Based on the coordinate transforms stated above and the motion analysis on each mechanical component, the angular velocities of the outer-ring and inner-disk can be rewritten, respectively as follows:

$$ \begin{aligned} \underline \Upomega _{O} = & \underline \omega + \underline \omega _{{O/C}} \\ = & ( - \dot \phi \sin \theta + \dot \psi )\hat e_{x} + (\dot \phi \cos \theta \sin \psi + \dot \theta \cos \psi )\hat e_{y} + (\dot \phi \cos \theta \cos \psi + \dot \theta \sin \psi )\hat e_{z} \\ \quad + \dot \phi _{A} \hat e_{y} + \dot \beta _{d} \hat e_{W} \\ \end{aligned} $$

(31)

$$ \begin{aligned} \underline \Upomega _{i} = & \underline \Upomega _{O} + \underline \omega _{{i/O}} \\ = & ( - \dot \phi \sin \theta + \dot \psi )\hat e_{x} + (\dot \phi \cos \theta \sin \psi + \dot \theta \cos \psi )\hat e_{y} + (\dot \phi \cos \theta \cos \psi + \dot \theta \sin \psi )\hat e_{z} \\ \quad + \dot \phi _{A} \hat e_{y} + \dot \beta _{d} \hat e_{W} + \dot \varphi _{v} \hat e_{v} \\ \end{aligned} $$

(32)

The coordinate transform of the Chip-frame with respect to Ring-frame is shown in Fig. 15. The transformation between Chip-frame and Ring-frame can be describe by following rotation matrix

$$ \begin{aligned} \left\{ {\begin{array}{*{20}c} {\hat e_{x} } \\ {\hat e_{y} } \\ {\hat e_{z} } \\ \end{array} } \right\} = & \left[ {\begin{array}{*{20}c} 1 & 0 & 0 \\ 0 & {\cos \phi _{A} } & { - \sin \phi _{A} } \\ 0 & {\sin \phi _{A} } & {\cos \phi _{A} } \\ \end{array} } \right]\left[ {\begin{array}{*{20}c} {\cos \beta _{d} } & { - \sin \beta _{d} } & 0 \\ {\sin \beta _{d} } & {\cos \beta _{d} } & 0 \\ 0 & 0 & 1 \\ \end{array} } \right]\left\{ {\begin{array}{*{20}c} {\hat e_{U} } \\ {\hat e_{V} } \\ {\hat e_{W} } \\ \end{array} } \right\} \\ = & \left[ {\begin{array}{*{20}c} {\cos \beta _{d} } & { - \sin \beta _{d} } & 0 \\ {\cos \phi _{A} \sin \beta _{d} } & {\cos \phi _{A} \cos \beta _{d} } & { - \sin \phi _{A} } \\ {\sin \phi _{A} \sin \beta _{d} } & {\sin \phi _{A} \cos \beta _{d} } & {\cos \phi _{A} } \\ \end{array} } \right]\left\{ {\begin{array}{*{20}c} {\hat e_{U} } \\ {\hat e_{V} } \\ {\hat e_{W} } \\ \end{array} } \right\} \\ \end{aligned} $$

(33)

Fig. 15

Coordinate transform of the chip frame with respect to ring frame

Based on the coordinate transforms stated above and the motion analysis on each mechanical component, the angular velocities of the outer-ring and inner-disk can be rewritten, respectively as follows:

$$ \begin{aligned} \underline \Upomega _{O} = & \underline \omega + \underline \omega _{{O/C}} \\ = & ( - \dot \phi \sin \theta + \dot \psi + \dot \beta _{d} \cos \phi _{A} )\hat e_{x} + (\dot \phi \cos \theta \sin \psi + \dot \theta \cos \psi + \dot \phi _{A} )\hat e_{y} \\ \quad + (\dot \phi \cos \theta \cos \psi + \dot \theta \sin \psi + \dot \beta _{d} \sin \phi _{A} )\hat e_{z} \\ \end{aligned} $$

(34)

$$ \begin{aligned} \underline \Upomega _{i} = & \underline \Upomega _{O} + \underline \omega _{{i/O}} \\ = & ( - \dot \phi \sin \theta + \dot \psi + \dot \beta _{d} \cos \phi _{A} + \dot \varphi _{v} \cos \beta _{d} \sin \phi _{A} )\hat e_{x} + (\dot \phi \cos \theta \sin \psi + \dot \theta \cos \psi + \dot \phi _{A} \\ \quad + \dot \varphi _{v} \sin \beta _{d} )\hat e_{y} + (\dot \phi \cos \theta \cos \psi + \dot \theta \sin \psi + \dot \beta _{d} \sin \phi _{A} + \dot \varphi _{v} \cos \beta _{d} \cos \phi _{A} )\hat e_{z} \\ \end{aligned} $$

(35)

The position vector of DTMP can be described as:

$$ \underline r _{p} = \underline R + \underline r _{{P/C}} $$

(36)

where $\underline R$ is the position vector of the center of gyroscope relative to the inertia frame. Since the DTPM is constrained to move on x–y plane, the component along z-axis of position vector is zero (i.e., r _z  = 0). Hence, the velocity of DTPM can be expressed as:

$$ \underline V _{p} = \underline {\dot r} _{{P/C}} + \underline \omega \times \underline r _{{P/C}} = (r_{z} \omega _{y} - r_{y} \omega _{z} )\hat e_{x} + (\dot r_{y} - r_{z} \omega _{x} )\hat e_{y} + (\dot r_{z} + r_{y} \omega _{x} )\hat e_{z} $$

(37)

It is noted that without loss of generosity to investigate the capability of a gyroscope it has been assumed $\underline {\dot R} = \underline 0 .$ The kinetic energy of the gyroscope system can be obtained by summation of the three inertia modules: outer-ring, inner-disk and proof mass.

$$ T = T_{{\text{O}}} + T_{{\text{i}}} + T_{{\text{P}}} $$

(38)

where T _O is the kinetic energy of outer-ring. T _i and T _P are the kinetic energy of inner-disk and DTPM, respectively. They can be individually expressed as follows:

$$ \begin{aligned} T_{{\text{o}}} = & (J_{{\text{o}}} /2)\underline \Upomega _{o} \cdot \underline \Upomega _{o} \\ = & (J_{{\text{o}}} /2)\left[ {\dot \varphi ^{2} + \dot \psi ^{2} + \dot \theta ^{2} + \dot \varphi _{A}^{2} + \dot \beta _{d}^{2} - 2\dot \varphi \dot \psi \sin \theta - 2\dot \varphi \dot \beta _{d} (\sin \theta \cos \varphi _{A} + \cos \theta \cos \psi \sin \varphi _{A} )} \right. \\ \left. {\quad + 2\dot \psi \dot \beta _{d} \cos \varphi _{A} + 2\dot \varphi \dot \varphi _{A} \cos \theta \sin \psi + 2\dot \theta \dot \varphi _{A} \cos \psi + 2\dot \theta \dot \beta _{d} \sin \psi \sin \varphi _{A} } \right] \\ \end{aligned} $$

(39)

$$ \begin{aligned} T_{{\text{i}}} = & \frac{1}{2}J_{{\text{i}}} \underline \Upomega _{i} \cdot \underline \Upomega _{i} \\ = & \frac{{J_{{\text{i}}} }}{2}\left[ {\dot \phi ^{2} + \dot \psi ^{2} + \dot \theta ^{2} + \dot \phi _{A}^{2} + \dot \beta _{d}^{2} + \dot \varphi _{v}^{2} - 2\dot \phi \dot \psi \sin \theta - 2\dot \phi \dot \beta _{d} (\sin \theta \cos \phi _{A} + \cos \theta \cos \psi \sin \phi _{A} )} \right. \\ \quad + 2\dot \psi \dot \beta _{d} \cos \phi _{A} + 2\dot \phi \dot \phi _{A} \cos \theta \sin \psi + 2\dot \theta \dot \phi _{A} \cos \psi + 2\dot \theta \dot \beta _{d} \sin \psi \sin \phi _{A} \\ \quad - 2\dot \phi \dot \varphi _{v} (\sin \theta \cos \beta _{d} \sin \phi _{A} + \cos \theta \sin \psi \sin \beta _{d} + \cos \theta \cos \psi \cos \beta _{d} \cos \phi _{A} ) \\ \quad \left. { + 2\dot \psi \dot \varphi _{v} \cos \beta _{d} \sin \phi _{A} - 2\dot \theta \dot \varphi _{v} (\cos \psi \sin \beta _{d} + \sin \psi \cos \beta _{d} \cos \phi _{A} ) + 2\dot \phi _{A} \dot \varphi _{v} \sin \beta _{d} } \right] \\ \end{aligned} $$

(40)

$$ \begin{aligned} T_{{\text{p}}} = & \frac{1}{2}M_{{{\text{eq}}}} \underline V _{p} \cdot \underline V _{p} \\ = & \frac{1}{2}M_{{{\text{eq}}}} \left[ {\dot r_{y}^{2} + \dot r_{z}^{2} + r_{y}^{2} (\dot \phi ^{2} \cos ^{2} \theta \cos ^{2} \psi + \dot \theta ^{2} \sin ^{2} \psi - 2\dot \phi \dot \theta \cos \theta \cos \psi \sin \psi )} \right. \\ \quad + r_{z}^{2} (\dot \phi ^{2} \cos ^{2} \theta \sin ^{2} \psi + \dot \theta ^{2} \cos ^{2} \psi + 2\dot \phi \dot \theta \cos \theta \cos \psi \sin \psi ) \\ \quad + \left( {r_{y}^{2} + r_{z}^{2} } \right)\left( {\dot \psi ^{2} + \dot \phi ^{2} \sin ^{2} \theta - 2\dot \psi \dot \phi \sin \theta } \right) \\ \quad - 2r_{y} r_{y} (\dot \phi ^{2} \cos ^{2} \theta \cos \psi \sin \psi + \dot \phi \dot \theta \cos \theta (\cos ^{2} \psi - \sin ^{2} \psi ) \\ \quad \left. { - \dot \theta ^{2} \cos \psi \sin \psi + 2(\dot r_{y} r_{y} - \dot r_{y} r_{y} )(\dot \psi - \dot \phi \sin \theta )} \right] \\ \end{aligned} $$

(41)

where J _o and J _i are polar moments of inertia of outer-ring and inner-disk, respectively. M _eq is equivalent mass of translation proof mass. Secondly, by assuming that the limited elongations of all springs are both in the elastic region, the potential energy of the tri-axis micro gyroscope can be expressed as:

$$ V = V_{{\phi _{A} }} + V_{{\beta _{d} }} + V_{{\varphi _{v} }} + V_{{r_{y} }} + V_{{r_{z} }} $$

(42)

where

$$ V_{{\phi _{A} }} = \frac{1}{2}k_{{\phi _{A} }} \phi _{A} ^{2} $$

(43)

$$ V_{{\beta _{d} }} = \frac{1}{2}k_{{\beta _{d} }} \beta _{d} ^{2} $$

(44)

$$ V_{{\varphi _{v} }} = \frac{1}{2}k_{{\varphi _{v} }} \varphi _{v}^{2} $$

(45)

$$ V_{{r_{y} }} = \frac{1}{2}k_{{r_{y} }} r_{y}^{2} $$

(46)

$$ V_{{r_{z} }} = \frac{1}{2}k_{{r_{z} }} r_{z}^{2} $$

(47)

$V_{{\varphi _{A} }}$ and $V_{{\beta _{d} }}$ are the potential energy of the torsional spring S1, corresponding to angular displacements ϕ _A and β _d , respectively. $V_{{\varphi _{v} }}$ is potential energy of torsional spring S2. $V_{{r_{y} }}$ and $V_{{r_{z} }}$ are the potential energy of the springs S3 and S4, respectively. In addition to the conservative energy, energy dissipation of the mechanical energy conducted by viscous damping forces is considered as well. There are two major types of frictions involved for the micro device whose size scale is down to 10⁻⁴–10⁻⁷ m. Namely, one is Couette damping and the other is squeeze-film damping. Consequently, the Rayleigh’s dissipation function is employed to describe the interested energy dissipation as follows:

$$ R = R_{{\phi _{A} }} + R_{{\beta _{d} }} + R_{{\varphi _{v} }} + R_{{r_{y} }} + R_{{r_{z} }} $$

(48)

where

$$ R_{{\phi _{A} }} = \frac{1}{2}C_{{\phi _{A} }} \dot \phi _{A}^{2} $$

(49)

$$ R_{{\beta _{d} }} = \frac{1}{2}C_{{\beta _{d} }} \dot \beta _{d}^{2} $$

(50)

$$ R_{{\varphi _{v} }} = \frac{1}{2}C_{{\varphi _{v} }} \dot \varphi _{v}^{2} $$

(51)

$$ R_{{r_{y} }} = \frac{1}{2}C_{{r_{y} }} \dot r_{y}^{2} $$

(52)

$$ R_{{r_{z} }} = \frac{1}{2}C_{{r_{z} }} \dot r_{z}^{2} $$

(53)

Resulting from squeeze film damping, $R_{{\phi _{A} }}$ and $R_{{\varphi _{v} }}$ represent the dissipation functions regarding the outer-ring rotation about A-axis and inner-disk rotation about V-axis, respectively. Due to Couette damping, $R_{{\beta _{d} }}$ denotes the dissipation functions about drive direction. On other hands, $R_{{r_{y} }}$ and $R_{{r_{z} }}$ are the dissipation functions of DTPM translation about A-axis and B-axis, respectively. Finally, the kinetic energy, potential energy and dissipation function are substituted into Lagrange’s equation, i.e.,

$$ \frac{{\text{d}}}{{{\text{d}}t}}\left( {\frac{{\partial L}}{{\partial \dot q_{i} }}} \right) - \left( {\frac{{\partial L}}{{\partial q_{i} }}} \right) + \frac{{\partial R}}{{\partial \dot q_{i} }} = (\tau _{i} )_{n} $$

(54)

where L is Lagragian which is defined as L = T−V. q _i is the generalized coordinate. $\dot q_{i}$ is the generalized velocity. (τ _i ) _n is the component of non-conservative force (or moment) in the direction of generalized coordinate q _i . Assume ϕ, ψ and θ are small and $\dot \varphi ,$ $\dot \psi ,$ and $\dot \theta$ is constant. Moreover, ϕ_A, ϕ _v , and β _d are much small then ϕ, ψ and θ. Therefore the motion equations can be simplified as:

$$ \ddot \beta _{d} + \frac{{\omega _{{\beta _{d} }} }}{{Q_{{\beta _{d} }} }}\dot \beta _{d} + \omega _{{\beta _{d} }}^{2} \beta _{d} = \dot \phi \dot \phi _{A} - J_{R} \left( {\dot \varphi _{v} \dot \theta + \dot \phi _{A} \dot \varphi _{v} } \right) + \tilde M_{{\beta _{d} }} (t) - \left( {4\ell /J_{{\text{O}}} + J_{{\text{i}}} } \right)F_{{r_{z} }} $$

(55)

$$ \ddot \phi _{A} + \frac{{\omega _{{\phi _{A} }} }}{{Q_{{\phi _{A} }} }}\dot \phi _{A} + \omega _{{\varphi _{A} }}^{2} \phi _{A} = - \dot \phi \dot \beta _{d} + J_{R} \left( {\dot \psi \dot \varphi _{v} + \dot \beta _{d} \dot \varphi _{v} } \right) $$

(56)

$$ \ddot \varphi _{v} + \frac{{\omega _{{\varphi _{v} }} }}{{Q_{{\varphi _{v} }} }}\dot \varphi _{v} + \omega _{{\phi _{v} }}^{2} \varphi _{v} = \dot \psi \dot \phi _{A} - \dot \theta \dot \beta _{d} - \dot \theta \dot \psi - \dot \phi _{A} \dot \beta _{d} $$

(57)

$$ \ddot r_{y} + \frac{{\omega _{{r_{y} }} }}{{Q_{{r_{y} }} }}\dot r_{y} + \omega _{{r_{y} }}^{2} r_{y} = 2\dot r_{z} \dot \psi $$

(58)

$$ \ddot r_{z} + \frac{{\omega _{{r_{z} }} }}{{Q_{{r_{z} }} }}\dot r_{z} + \omega _{{r_{z} }}^{2} r_{z} = - 2\dot r_{y} \dot \psi + F_{{r_{z} }} /M_{{eq}} $$

(59)

where $\omega _{{\beta _{d} }} = \sqrt {k_{\beta } /(J_{{\text{o}}} + J_{i} )} ,$ $\omega _{{\phi _{A} }} = \sqrt {k_{{\phi _{A} }} /\left( {J_{0} + J_{i} } \right)} ,$ $\omega _{{\phi _{v} }} = \sqrt {k_{\varphi } /J_{i} } ,$ $\omega _{{r_{y} }} = \sqrt {k_{{_{{r_{y} }} }} /M_{{eq}} }$ and $\omega _{{r_{z} }} = \sqrt {k_{{_{{r_{z} }} }} /M_{{{\text{eq}}}} }$ are the natural frequencies in directions β _d , ϕ _A , φ _v , r _A and r _B , respectively. $Q_{{\beta _{d} }} = (J_{{\text{o}}} + J_{{\text{i}}} )\omega _{{\beta _{d} }} /C_{{\beta _{d} }} ,$ $Q_{{\phi _{A} }} = (J_{{\text{o}}} + J_{{\text{i}}} )\omega _{{\phi _{A} }} /C_{{\phi _{A} }} ,$ $Q_{{\varphi _{v} }} = J_{{\text{i}}} \omega _{{\varphi _{v} }} /C_{{\varphi _{v} }} ,$ $Q_{{r_{y} }} = M_{{{\text{eq}}}} \omega _{{r_{y} }} /C_{{r_{y} }}$ and $Q_{{r_{z} }} = M_{{eq}} \omega _{{r_{z} }} /C_{{r_{z} }}$ are the associated quality factors. $F_{{r_{z} }}$ is the constraint force between ribs and DTPM.