An analytical method for vibration analysis of multi-span Timoshenko beams under arbitrary boundary conditions

Abstract

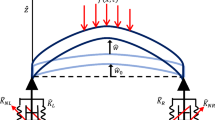
An analytical method for analyzing free vibration of multi-span Timoshenko beams under arbitrary boundary conditions is proposed in this paper. Based on the theoretical model of the Timoshenko beam, linear displacement springs and rotational springs are introduced to simulate the boundary and support forces of multi-span Timoshenko beams. By modifying the springs’ stiffness, different boundary conditions and inter-span coupling conditions can be simulated. To develop the vibration calculation models based on the energy method, the improved Fourier cosine series with four sine series are introduced to represent the displacement functions in order to eliminate the discontinuities or jumps in the solution processes. With the Rayleigh–Ritz method, the Lagrange equations of structures are solved to obtain free vibration characteristics. Using a three-span beam and a four-span beam as examples, this method is applied to calculate the natural frequencies of structures with circular and rectangular cross sections. The correctness and accuracy of the method are verified by comparing the solutions with the results of existing literature. On this basis, the influences of boundary conditions, span ratio and span number on the vibration characteristics of multi-span Timoshenko beams are discussed and analyzed.

Appendix

According to the above theoretical derivation process, if the span number is N, and the highest number of terms in the improved Fourier series is M, then the dimension unknown coefficient vector A in Eq. (19) is [2(M + 5) × N] × 1, the specific formula is expressed as follows:

$${\mathbf{A}} = \left\{ {A_{i,0} ,A_{i,1} , \cdots ,A_{i,m} , \cdots ,A_{i,M} ,A_{i,1}^{a} ,A_{i,2}^{a} ,A_{i,3}^{a} ,A_{i,4}^{a} ,B_{i,0} ,B_{i,1} , \cdots ,B_{i,m} , \cdots ,B_{i,M} ,B_{i,1}^{a} ,B_{i,2}^{a} ,B_{i,3}^{a} ,B_{i,4}^{a} } \right\}$$

(20)

Similarly, for an N-span Timoshenko beam, its overall mass matrix ${\mathbf{M}}$ and stiffness matrix ${\mathbf{K}}$ are both [2(M + 5) × N] × [2(M + 5) × N] order matrices. Thus, the specific formulas of the overall mass matrix ${\mathbf{M}}$ and stiffness matrix ${\mathbf{K}}$ in Eq. (19) are expressed as follows:

$${\mathbf{K}} = {\mathbf{K}}_{P} + {\mathbf{K}}_{S} + {\mathbf{K}}_{C}$$

(21)

where ${\mathbf{K}}_{P}$, ${\mathbf{K}}_{S}$ and ${\mathbf{K}}_{C}$ are all [2(M + 5) × N] × [2(M + 5) × N] order matrices.

$${\mathbf{K}}_{P} = \left[ {\begin{array}{*{20}l} {{\mathbf{K}}_{1,P} } \hfill & 0 \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & \ddots \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & {{\mathbf{K}}_{i,P} } \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & 0 \hfill & {{\mathbf{K}}_{N,P} } \hfill \\ \end{array} } \right]_{N \times N}$$

(22)

where ${\mathbf{K}}_{i,P}$ is 2(M + 5) × 2(M + 5) order matrices, its specific formula is expressed as follows:

$${\mathbf{K}}_{i,P} = \left[ {\begin{array}{*{20}l} {K_{11}^{i,P} } \hfill & {K_{12}^{i,P} } \hfill & {K_{13}^{i,P} } \hfill & {K_{14}^{i,P} } \hfill \\ {K_{21}^{i,P} } \hfill & {K_{22}^{i,P} } \hfill & {K_{23}^{i,P} } \hfill & {K_{24}^{i,P} } \hfill \\ {K_{31}^{i,P} } \hfill & {K_{32}^{i,P} } \hfill & {K_{33}^{i,P} } \hfill & {K_{34}^{i,P} } \hfill \\ {K_{41}^{i,P} } \hfill & {K_{42}^{i,P} } \hfill & {K_{43}^{i,P} } \hfill & {K_{44}^{i,P} } \hfill \\ \end{array} } \right]$$

(23)

$$K_{11}^{i,P} = GA\beta \lambda_{i,r} \lambda_{i,k} \int_{0}^{{l_{i} }} {\sin \lambda_{i,r} x_{i} \sin \lambda_{i,k} x_{i} {\text{d}}x_{i} }$$

(24)

$$K_{22}^{i,P} = GA\beta \lambda_{i,s} \lambda_{i,j} \int_{0}^{{l_{i} }} {\cos \lambda_{i,s} x_{i} \cos \lambda_{i,j} x_{i} {\text{d}}x_{i} }$$

(25)

$$\begin{aligned} K_{33}^{i,P} & = EI\lambda_{i,r} \lambda_{i,k} \int_{0}^{{l_{i} }} {\sin \lambda_{i,r} x_{i} \sin \lambda_{i,k} x_{i} {\text{d}}x_{i} } \\ & \quad + GA\beta \int_{0}^{{l_{i} }} {\cos \lambda_{i,r} x_{i} \cos \lambda_{i,k} x_{i} {\text{d}}x_{i} } \\ \end{aligned}$$

(26)

$$\begin{aligned} K_{44}^{i,P} & = EI\lambda_{i,s} \lambda_{i,j} \int_{0}^{{l_{i} }} {\cos \lambda_{i,s} x_{i} \cos \lambda_{i,j} x_{i} {\text{d}}x_{i} } \\ & \quad + GA\beta \int_{0}^{{l_{i} }} {\sin \lambda_{i,s} x_{i} \sin \lambda_{i,j} x_{i} {\text{d}}x_{i} } \\ \end{aligned}$$

(27)

$$K_{12}^{i,P} = GA\beta \left( { - \lambda_{i,r} } \right)\lambda_{i,j} \int_{0}^{{l_{i} }} {\sin \lambda_{i,r} x_{i} \cos \lambda_{i,j} x_{i} {\text{d}}x_{i} }$$

(28)

$$K_{13}^{i,P} = GA\beta \lambda_{i,r} \int_{0}^{{l_{i} }} {\sin \lambda_{i,r} x_{i} \cos \lambda_{i,k} x_{i} {\text{d}}x_{i} }$$

(29)

$$K_{14}^{i,P} = GA\beta \lambda_{i,r} \int_{0}^{{l_{i} }} {\sin \lambda_{i,r} x_{i} \sin \lambda_{i,j} x_{i} {\text{d}}x_{i} }$$

(30)

$$K_{21}^{i,P} = GA\beta \lambda_{i,s} \left( { - \lambda_{i,k} } \right)\int_{0}^{{l_{i} }} {\cos \lambda_{i,s} x_{i} \sin \lambda_{i,k} x_{i} {\text{d}}x_{i} }$$

(31)

$$K_{23}^{i,P} = GA\beta \left( { - \lambda_{i,s} } \right)\int_{0}^{{l_{i} }} {\cos \lambda_{i,s} x_{i} \cos \lambda_{i,k} x_{i} {\text{d}}x_{i} }$$

(32)

$$K_{24}^{i,P} = GA\beta \left( { - \lambda_{i,s} } \right)\int_{0}^{{l_{i} }} {\cos \lambda_{i,s} x_{i} \sin \lambda_{i,j} x_{i} {\text{d}}x_{i} }$$

(33)

$$K_{31}^{i,P} = GA\beta \lambda_{i,k} \int_{0}^{{l_{i} }} {\cos \lambda_{i,r} x_{i} \sin \lambda_{i,k} x_{i} {\text{d}}x_{i} }$$

(34)

$$K_{32}^{i,P} = GA\beta \left( { - \lambda_{i,j} } \right)\int_{0}^{{l_{i} }} {\cos \lambda_{i,r} x_{i} \cos \lambda_{i,j} x_{i} {\text{d}}x_{i} }$$

(35)

$$\begin{aligned} K_{34}^{i,P} & = EI\left( { - \lambda_{i,r} } \right)\lambda_{i,j} \int_{0}^{{l_{i} }} {\sin \lambda_{i,r} x_{i} \cos \lambda_{i,j} x_{i} {\text{d}}x_{i} } \\ & \quad + GA\beta \int_{0}^{{l_{i} }} {\cos \lambda_{i,r} x_{i} \sin \lambda_{i,j} x_{i} {\text{d}}x_{i} } \\ \end{aligned}$$

(36)

$$K_{41}^{i,P} = GA\beta \lambda_{i,k} \int_{0}^{{l_{i} }} {\sin \lambda_{i,s} x_{i} \sin \lambda_{i,k} x_{i} {\text{d}}x_{i} }$$

(37)

$$K_{42}^{i,P} = GA\beta \left( { - \lambda_{i,j} } \right)\int_{0}^{{l_{i} }} {\sin \lambda_{i,s} x_{i} \cos \lambda_{i,j} x_{i} {\text{d}}x_{i} }$$

(38)

$$\begin{aligned} K_{43}^{i,P} & = EI\lambda_{i,s} \left( { - \lambda_{i,k} } \right)\int_{0}^{{l_{i} }} {\cos \lambda_{i,s} x_{i} \sin \lambda_{i,k} x_{i} {\text{d}}x_{i} } \\ & \quad + GA\beta \int_{0}^{{l_{i} }} {\sin \lambda_{i,s} x_{i} \cos \lambda_{i,k} x_{i} {\text{d}}x_{i} } \\ \end{aligned}$$

(39)

$${\mathbf{K}}_{S} = \left[ {\begin{array}{*{20}l} {{\mathbf{K}}_{1,S} } \hfill & 0 \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & \ddots \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & {{\mathbf{K}}_{i,S} } \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & 0 \hfill & {{\mathbf{K}}_{N,S} } \hfill \\ \end{array} } \right]$$

(40)

where ${\mathbf{K}}_{i,S}$ is 2(M + 5) × 2(M + 5) order matrices, its specific formula is expressed as follows:

$${\mathbf{K}}_{i,S} = \left[ {\begin{array}{*{20}l} {K_{11}^{i,S} } \hfill & 0 \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & {K_{22}^{i,S} } \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & {K_{33}^{i,S} } \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & 0 \hfill & 0 \hfill \\ \end{array} } \right]$$

(41)

$$K_{11}^{i,S} = B_{i} \left( {\tilde{k}_{i,0} + \left( { - 1} \right)^{r + k} \tilde{k}_{i,l} } \right)$$

(42)

$$K_{22}^{i,S} = B_{i} \left( {\lambda_{i,s} \lambda_{i,j} \tilde{K}_{i,0} + \left( { - 1} \right)^{s + j} \lambda_{i,s} \lambda_{i,j} \tilde{K}_{i,l} } \right)$$

(43)

$$K_{33}^{i,S} = B_{i} \left( {\tilde{K}_{i,0} + \left( { - 1} \right)^{r + k} \tilde{K}_{i,l} } \right)$$

(44)

$${\mathbf{\rm K}}_{C} = \left[ {\begin{array}{*{20}l} \ddots \hfill & {{\mathbf{K}}_{i,C}^{11} } \hfill & {{\mathbf{K}}_{i,C}^{12} } \hfill \\ {{\mathbf{K}}_{i,C}^{21} } \hfill & {{\mathbf{K}}_{i,C}^{22} + {\mathbf{K}}_{i + 1,C}^{11} } \hfill & {{\mathbf{K}}_{i + 1,C}^{12} } \hfill \\ {{\mathbf{K}}_{i + 1,C}^{21} } \hfill & {{\mathbf{K}}_{i + 1,C}^{22} } \hfill & \ddots \hfill \\ \end{array} } \right]_{N \times N}$$

(45)

where ${\mathbf{K}}_{i,C}^{11}$, ${\mathbf{K}}_{i,C}^{12}$, ${\mathbf{K}}_{i,C}^{21}$, ${\mathbf{K}}_{i,C}^{22}$ are all 2(M + 5) × 2(M + 5) order matrices, its specific formula are expressed as follows:

$${\mathbf{K}}_{i,C}^{11} = \left[ {\begin{array}{*{20}l} {K_{11,11}^{i,C} } \hfill & 0 \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & {K_{11,22}^{i,C} } \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & {K_{11,33}^{i,C} } \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & 0 \hfill & 0 \hfill \\ \end{array} } \right]$$

(46)

$$K_{11,11}^{i,C} = B_{i} \left( { - 1} \right)^{r + k} k_{i,i + 1}$$

(47)

$$K_{11,22}^{i,C} = B_{i} \lambda_{i,s} \lambda_{i,j} \left( { - 1} \right)^{s + j} K_{i,i + 1}$$

(48)

$$K_{11,33}^{i,C} = B_{i} \left( { - 1} \right)^{r + k} K_{i,i + 1}$$

(49)

$${\mathbf{K}}_{i,C}^{12} = \left[ {\begin{array}{*{20}l} {K_{12,11}^{i,C} } \hfill & 0 \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & {K_{12,22}^{i,C} } \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & {K_{12,33}^{i,C} } \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & 0 \hfill & 0 \hfill \\ \end{array} } \right]$$

(50)

$$K_{12,11}^{i,C} = - B_{i} \left( { - 1} \right)^{s} k_{i,i + 1}$$

(51)

$$K_{12,22}^{i,C} = - B_{i} \lambda_{i,s} \lambda_{i,j} \left( { - 1} \right)^{s} K_{i,i + 1}$$

(52)

$$K_{12,33}^{i,C} = - B_{i} \left( { - 1} \right)^{s} K_{i,i + 1} \left( {A.34} \right)$$

(53)

$${\mathbf{K}}_{i,C}^{22} = \left[ {\begin{array}{*{20}l} {K_{22,11}^{i,C} } \hfill & 0 \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & {K_{22,22}^{i,C} } \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & {K_{22,33}^{i,C} } \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & 0 \hfill & 0 \hfill \\ \end{array} } \right]$$

(54)

$$K_{22,11}^{i,C} = B_{i} k_{i,i + 1}$$

(55)

$$K_{22,22}^{i,C} = B_{i} \lambda_{i,s} \lambda_{i,j} K_{i,i + 1}$$

(56)

$$K_{22,33}^{i,C} = B_{i} K_{i,i + 1}$$

(57)

$${\mathbf{K}}_{i,C}^{21} = \left( {{\mathbf{K}}_{i,C}^{12} } \right)^{T}$$

(58)

$${\mathbf{M}} = \left[ {\begin{array}{*{20}l} {{\mathbf{M}}_{1} } \hfill & 0 \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & \ddots \hfill & 0 \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & {{\mathbf{M}}_{i} } \hfill & 0 \hfill \\ 0 \hfill & 0 \hfill & 0 \hfill & {{\mathbf{M}}_{N} } \hfill \\ \end{array} } \right]$$

(59)

$${\mathbf{M}}_{i} = \left[ {\begin{array}{*{20}l} {M_{11}^{i} } \hfill & {M_{12}^{i} } \hfill & {M_{13}^{i} } \hfill & {M_{14}^{i} } \hfill \\ {M_{21}^{i} } \hfill & {M_{22}^{i} } \hfill & {M_{23}^{i} } \hfill & {M_{24}^{i} } \hfill \\ {M_{31}^{i} } \hfill & {M_{32}^{i} } \hfill & {M_{33}^{i} } \hfill & {M_{34}^{i} } \hfill \\ {M_{41}^{i} } \hfill & {M_{42}^{i} } \hfill & {M_{43}^{i} } \hfill & {M_{44}^{i} } \hfill \\ \end{array} } \right]$$

(60)

$$M_{11}^{i} = \rho A\int_{0}^{{l_{i} }} {\cos \lambda_{i,r} x_{i} \cos \lambda_{i,k} x_{i} {\text{d}}x_{i} }$$

(61)

$$M_{22}^{i} = \rho A\int_{0}^{{l_{i} }} {\sin \lambda_{i,s} x_{i} \sin \lambda_{i,j} x_{i} {\text{d}}x_{i} }$$

(62)

$$M_{33}^{i} = \rho I\int_{0}^{{l_{i} }} {\cos \lambda_{i,r} x_{i} \cos \lambda_{i,k} x_{i} {\text{d}}x_{i} }$$

(63)

$$M_{22}^{i} = \rho I\int_{0}^{{l_{i} }} {\sin \lambda_{i,s} x_{i} \sin \lambda_{i,j} x_{i} {\text{d}}x_{i} }$$

(64)

$$M_{12}^{i} = \rho A\int_{0}^{{l_{i} }} {\cos \lambda_{i,r} x_{i} \sin \lambda_{i,j} x_{i} {\text{d}}x_{i} }$$

(65)

$$M_{21}^{i} = \rho A\int_{0}^{{l_{i} }} {\sin \lambda_{i,s} x_{i} \cos \lambda_{i,k} x_{i} {\text{d}}x_{i} }$$

(66)

$$M_{34}^{i} = \rho I\int_{0}^{{l_{i} }} {\cos \lambda_{i,r} x_{i} \sin \lambda_{i,j} x_{i} {\text{d}}x_{i} }$$

(67)

$$M_{43}^{i} = \rho I\int_{0}^{{l_{i} }} {\sin \lambda_{i,s} x_{i} \cos \lambda_{i,k} x_{i} {\text{d}}x_{i} }$$

(68)

$$M_{13}^{i} = M_{14}^{i} = M_{23}^{i} = M_{24}^{i} = M_{31}^{i} = M_{32}^{i} = M_{41}^{i} = M_{42}^{i} = 0$$

(69)

where $r,k = 1,2, \ldots ,M$; $s,j = 1,2,3,4$; $i = 1,2, \ldots ,N$.