The thermoelastic contact problem of one-dimensional hexagonal quasicrystal layer with interfacial imperfections

Abstract

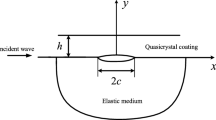
The coupled thermo-mechanical contact between a rotating sphere made of one-dimensional hexagonal quasicrystal materials, and a layer-substrate system with interfacial imperfections, is investigated by applying the discrete convolution-fast Fourier transform (DC-FFT) algorithm. The effects of dislocation-like interface defects, friction coefficient, coating thickness and rotation velocity on the thermoelastic field are discussed. The effect of vertical imperfection on the phason normal displacement is opposite to that of horizontal imperfections. The discontinuity of the horizontal displacement plays a limiting role on the effect of the vertical discontinuities on the phason normal stress. The peak value of the normal phason displacement decreases, and the peak point obviously moves in the negative direction of the x-axis with the increase of friction coefficient, while the change of the phason normal stress is negligible. The numerical results may provide theoretical help for the design of quasicrystal coatings.

Appendices

Appendix A

This appendix provides the constants closely related to the material constants of 1D hexagonal QC and the correlation coefficients in thermoelastic solutions of transversely isotropic substrate.

(1) The correlation coefficients in thermoelastic solutions of transversely isotropic substrate.

$$\begin{aligned} s_{0}^{{\prime }} & = \sqrt {{{c_{66}^{{\prime }} } \mathord{\left/ {\vphantom {{c_{66}^{{\prime }} } {c_{44}^{{\prime }} }}} \right. \kern-\nulldelimiterspace} {c_{44}^{{\prime }} }}} \\ s_{3}^{{\prime }} & = \sqrt {{{\kappa_{11}^{{\prime }} } \mathord{\left/ {\vphantom {{\kappa_{11}^{{\prime }} } {\kappa_{33}^{{\prime }} }}} \right. \kern-\nulldelimiterspace} {\kappa_{33}^{{\prime }} }}} \\ \end{aligned}$$

(A-1)

$s_{j}^{\prime } ,(j = 1,2)$ are the roots of the equation

$$a_{0}^{\prime } s^{\prime 4} - b_{0}^{\prime } s^{\prime 2} + c_{0}^{\prime } = 0$$

(A-2)

with positive real part, in which

$$\begin{aligned} a^{\prime}_{0} & = c^{\prime}_{33} c^{\prime}_{44} \\ b^{\prime}_{0} & = c^{\prime}_{11} c^{\prime}_{33} + c^{\prime2}_{44} - \left( {c^{\prime}_{13} + c^{\prime}_{44} } \right)^{2} \\ c^{\prime}_{0} & = c^{\prime}_{11} c^{\prime}_{44} \\ a^{\prime}_{1} & = \beta^{\prime}_{1} c^{\prime}_{44} \\ b^{\prime}_{1} & = - \beta^{\prime}_{3} \left( {c^{\prime}_{13} + c^{\prime}_{44} } \right) + \beta^{\prime}_{1} c^{\prime}_{33} \\ \end{aligned}$$

$$\begin{aligned} a^{\prime}_{2} & = \beta^{\prime}_{1} \left( {c^{\prime}_{13} + c^{\prime}_{44} } \right) - \beta^{\prime}_{3} c^{\prime}_{11} \\ b^{\prime}_{2} & = - \beta^{\prime}_{3} c^{\prime}_{44} \\ \lambda^{\prime}_{kj} & = - a^{\prime}_{k} + b^{\prime}_{k} s^{\prime2}_{j} ,\left( {k = 1,2,j = 1,2,3} \right) \\ \end{aligned}$$

$$\begin{aligned} \lambda^{\prime}_{33} & = a^{\prime}_{0} s^{\prime4}_{3} - b^{\prime}_{0} s^{\prime2}_{3} + c^{\prime}_{0} \\ \eta_{1j} & = {{\lambda^{\prime}_{2j} s^{\prime}_{j} } \mathord{\left/ {\vphantom {{\lambda^{\prime}_{2j} s^{\prime}_{j} } {\lambda^{\prime}_{1j} }}} \right. \kern-\nulldelimiterspace} {\lambda^{\prime}_{1j} }} \\ \eta_{2j} & = c^{\prime}_{33} \eta_{1j} s^{\prime}_{j} + c^{\prime}_{13} + \beta^{\prime}_{3} \eta_{4j} \\ \eta_{3j} & = c^{\prime}_{44} \left( {s^{\prime}_{j} - \eta_{1j} } \right) \\ \eta_{41} & = \eta_{42} = 0 \\ \eta_{43} & = {{\lambda^{\prime}_{33} } \mathord{\left/ {\vphantom {{\lambda^{\prime}_{33} } {\lambda^{\prime}_{31} }}} \right. \kern-\nulldelimiterspace} {\lambda^{\prime}_{31} }} \\ \varsigma_{j} & = c^{\prime}_{11} m^{2} + c^{\prime}_{12} n^{2} + c^{\prime}_{{{1}3}} \alpha^{2} \eta_{1j} s^{\prime}_{j} + \beta^{\prime}_{1} \alpha^{2} \eta_{4j} \\ \end{aligned}$$

(2) The constants closely related to the material constants of 1D hexagonal QCs.

$$s_{0} = \sqrt {{{c_{66} } \mathord{\left/ {\vphantom {{c_{66} } {c_{44} }}} \right. \kern-\nulldelimiterspace} {c_{44} }}} ,\quad s_{4} = \sqrt {{{\kappa_{11} } \mathord{\left/ {\vphantom {{\kappa_{11} } {\kappa_{33} }}} \right. \kern-\nulldelimiterspace} {\kappa_{33} }}} .$$

(A-3)

The constants $s_{i} \left( {i = 1,2,3} \right)$ are the eigen-value with a positive real part of the following characteristic equation

$$n_{1} s^{6} - n_{2} s^{4} + n_{3} s^{2} - n_{4} = 0$$

(A-4)

where

$$\begin{aligned} n_{1} & = - c_{44} \left( {R_{2}^{2} - c_{33} K_{1} } \right) \\ n_{2} & = - c_{33} \left[ {\left( {R_{1} + R_{3} } \right)^{2} - c_{44} K_{2} } \right] + K_{1} \left[ {c_{11} c_{33} + c_{44}^{2} - \left( {c_{13} + c_{44} } \right)^{2} } \right] \\ & \quad - R_{2} \left[ {2c_{44} R_{3} + c_{11} R_{2} - 2\left( {c_{13} + c_{14} } \right)\left( {R_{1} + R_{3} } \right)} \right] \\ n_{3} & = - c_{44} \left[ {\left( {R_{1} + R_{3} } \right)^{2} - c_{11} K_{1} } \right] + K_{2} \left[ {c_{11} c_{33} + c_{44}^{2} - \left( {c_{13} + c_{44} } \right)^{2} } \right] \\ & \quad - R_{3} \left[ {2c_{11} R_{2} + c_{44} R_{3} - 2\left( {c_{13} + c_{44} } \right)\left( {R_{1} + R_{3} } \right)} \right] \\ n_{4} & = - c_{11} \left( {R_{3}^{2} - c_{44} K_{2} } \right) \\ \end{aligned}$$

The constants involved in the general solutions (12, 13) are defined as

$$\begin{aligned} \alpha_{1i} & = - \frac{{\lambda_{2i} s_{i} }}{{\lambda_{1i} }},\quad \alpha_{2i} = - \frac{{\lambda_{3i} s_{i} }}{{\lambda_{1i} }},\quad \alpha_{31} = \alpha_{32} = \alpha_{33} = 0,\quad \alpha_{34} = - \frac{{\lambda_{44} }}{{\lambda_{14} }} \\ \gamma_{1i} & = c_{33} \alpha_{1i} s_{i} + R_{2} \alpha_{2i} s_{i} + c_{13} - \beta_{3} \alpha_{3i} \\ \gamma_{2i} & = R_{2} \alpha_{1i} s_{i} + K_{1} \alpha_{2i} s_{i} + R_{1} \\ \gamma_{3i} & = c_{11} m^{2} + c_{12} n^{2} + c_{13} \alpha^{2} \alpha_{1i} s_{i} + R_{1} \alpha^{2} \alpha_{2i} s_{i} - \beta_{1} \alpha^{2} \alpha_{3i} \\ \upsilon_{1i} & = c_{44} \left( {\alpha_{1i} - s_{i} } \right) + R_{3} \alpha_{2i} \\ \upsilon_{2i} & = R_{3} \left( {\alpha_{1i} - s_{i} } \right) + K_{2} \alpha_{2i} \\ \end{aligned}$$

(A-5)

in which

$$\begin{aligned} \lambda_{ji} & = a_{j} - b_{j} s_{i}^{2} + c_{j} s_{i}^{4} ,\quad \left( {i = 1,2,3,4;\quad j = 1,2,3} \right) \\ \lambda_{44} & = n_{1} s_{4}^{6} - n_{2} s_{4}^{4} + n_{3} s_{4}^{2} - n_{4} \\ \end{aligned}$$

with the auxiliary a_j, b_j and c_j being

$$\begin{aligned} a_{1} & = \beta_{1} \left( {c_{44} K_{2} - R_{3}^{2} } \right) \\ b_{1} & = \beta_{1} \left( {c_{44} K_{1} + c_{33} K_{2} - 2R_{2} R_{3} } \right) + \beta_{3} \left[ {R_{3} \left( {R_{1} + R_{3} } \right) - K_{2} \left( {c_{13} + c_{44} } \right)} \right] \\ c_{1} & = \beta_{1} \left( {c_{33} K_{1} - R_{2}^{2} } \right) + \beta_{3} \left[ {R_{2} \left( {R_{1} + R_{3} } \right) - K_{1} \left( {c_{13} + c_{44} } \right)} \right] \\ a_{2} & = \beta_{1} \left[ {R_{3} \left( {R_{1} + R_{3} } \right) - K_{2} \left( {c_{13} + c_{44} } \right)} \right] + \beta_{3} c_{11} K_{2} \\ b_{2} & = \beta_{1} \left[ {R_{2} \left( {R_{1} + R_{3} } \right) - K_{1} \left( {c_{13} + c_{44} } \right)} \right] + \beta_{3} \left[ {c_{11} K_{1} + c_{44} K_{2} - \left( {R_{1} + R_{3} } \right)^{2} } \right] \\ c_{2} & = \beta_{3} c_{44} K_{1} \\ a_{3} & = \beta_{1} \left( {c_{13} R_{3} - c_{44} R_{1} } \right) - \beta_{3} c_{11} R_{3} \\ b_{3} & = \beta_{1} \left[ {R_{2} \left( {c_{13} + c_{44} } \right) - c_{33} \left( {R_{1} + R_{3} } \right)} \right] + \beta_{3} \left[ {c_{13} R_{3} - c_{11} R_{2} + R_{1} \left( {c_{13} + c_{44} } \right)} \right] \\ c_{3} & = - \beta_{3} c_{44} R_{2} \\ \end{aligned}$$

Appendix B

The expressions of $\chi_{i} ,\xi_{j} ,D_{jk}$ in Eqs. (12, 13, 16) are given as follows

$$\begin{aligned} \chi_{{u_{x} 0}} & = inA_{0} (m,n)e^{{ - \alpha s_{0} z}} + in\overline{{A_{0} }} (m,n)e^{{\alpha s_{0} z}} \\ \chi_{{u_{x} k}} & = - imA_{k} (m,n)e^{{ - \alpha s_{k} z}} - im\overline{{A_{k} }} (m,n)e^{{\alpha s_{k} z}} \\ \chi_{{u_{y} 0}} & = - imA_{0} (m,n)e^{{ - \alpha s_{0} z}} - im\overline{{A_{0} }} (m,n)e^{{\alpha s_{0} z}} \\ \chi_{{u_{y} k}} & = - inA_{k} (m,n)e^{{ - \alpha s_{k} z}} - in\overline{{A_{k} }} (m,n)e^{{\alpha s_{k} z}} \\ \chi_{{u_{z} k}} & = - \alpha \alpha_{1k} A_{k} (m,n)e^{{ - \alpha s_{k} z}} + \alpha \alpha_{1k} \overline{{A_{k} }} (m,n)e^{{\alpha s_{k} z}} \\ \chi_{{w_{z} k}} & = - \alpha \alpha_{2k} A_{k} (m,n)e^{{ - \alpha s_{k} z}} + \alpha \alpha_{2k} \overline{{A_{k} }} (m,n)e^{{\alpha s_{k} z}} \\ \chi_{{\sigma_{xx} 0}} & = - 2mnc_{66} A_{0} (m,n)e^{{ - \alpha s_{0} z}} - 2mnc_{66} A_{0} (m,n)e^{{\alpha s_{0} z}} \\ \chi_{{\sigma_{xx} k}} & = \gamma_{3k} A_{k} (m,n)e^{{ - \alpha s_{k} z}} \; + \gamma_{3k} \overline{{A_{k} }} (m,n)e^{{\alpha s_{k} z}} \\ \chi_{{\sigma_{zz} k}} & = \alpha^{2} \gamma_{1k} A_{k} (m,n)e^{{ - \alpha s_{k} z}} + \alpha^{2} \gamma_{1k} \overline{{A_{k} }} (m,n)e^{{\alpha s_{k} z}} \\ \chi_{{\sigma_{zx} 0}} & = - in\alpha c_{44} s_{0} A_{0} (m,n)e^{{ - \alpha s_{0} z}} + in\alpha c_{44} s_{0} \overline{{A_{0} }} (m,n)e^{{\alpha s_{0} z}} \\ \chi_{{\sigma_{zx} k}} & = - im\alpha \upsilon_{1k} A_{k} (m,n)e^{{ - \alpha s_{k} z}} + im\alpha \upsilon_{1k} \overline{{A_{k} }} (m,n)e^{{\alpha s_{k} z}} \\ \chi_{{\sigma_{zy} 0}} & = im\alpha c_{44} s_{0} A_{0} (m,n)e^{{ - \alpha s_{0} z}} - im\alpha c_{44} s_{0} \overline{{A_{0} }} (m,n)e^{{\alpha s_{0} z}} \\ \chi_{{\sigma_{zy} k}} & = - in\alpha \upsilon_{1k} A_{k} (m,n)e^{{ - \alpha s_{k} z}} + in\alpha \upsilon_{1k} \overline{{A_{k} }} (m,n)e^{{\alpha s_{k} z}} \\ \chi_{{H_{zx} 0}} & = - in\alpha R_{3} s_{0} A_{0} (m,n)e^{{ - \alpha z_{0} }} + in\alpha R_{3} s_{0} \overline{{A_{0} }} (m,n)e^{{\alpha z_{0} }} \\ \chi_{{H_{zx} k}} &= - im\alpha \upsilon_{2k} A_{k} (m,n)e^{{ - \alpha z_{k} }} + im\alpha \upsilon_{2k} \overline{{A_{k} }} (m,n)e^{{\alpha z_{k} }} \\ \chi_{{H_{zy} 0}} &= im\alpha R_{3} s_{0} A_{0} (m,n)e^{{ - \alpha z_{0} }} - im\alpha R_{3} s_{0} \overline{{A_{0} }} (m,n)e^{{\alpha z_{0} }} \\ \chi_{{H_{zy} k}} &= - in\alpha \upsilon_{2k} A_{k} (m,n)e^{{ - \alpha z_{k} }} + in\alpha \upsilon_{2k} \overline{{A_{k} }} (m,n)e^{{\alpha z_{k} }} \\ \end{aligned}$$

(B-1)

$$\begin{aligned} \chi_{{H_{zz} k}} & = \alpha^{2} \gamma_{2k} A_{k} (m,n)e^{{ - \alpha s_{k} z}} + \alpha^{2} \gamma_{2k} \overline{{A_{k} }} (m,n)e^{{\alpha s_{k} z}} \\ \chi_{Tk} & = 0,(k = 1,2,3) \\ \chi_{T4} & = \alpha^{2} \alpha_{34} A_{4} (m,n)e^{{ - \alpha s_{4} z}} + \alpha^{2} \alpha_{34} \overline{{A_{4} }} (m,n)e^{{\alpha s_{4} z}} \\ k & = 1,2,3,4 \\ \xi_{{u^{\prime}_{z} j}} & = - \alpha \eta_{1j} B_{j} (m,n)e^{{ - \alpha s^{\prime}_{j} z^{\prime}}} \\ \xi_{{u^{\prime}_{x} 0}} & = inB_{0} (m,n)e^{{ - \alpha s^{\prime}_{0} z^{\prime}}} \\ \xi_{{u^{\prime}_{x} j}} & = - imB_{j} (m,n)e^{{ - \alpha s^{\prime}_{j} z^{\prime}}} \\ \xi_{{u^{\prime}_{y} 0}} & = - imB_{0} (m,n)e^{{ - \alpha s^{\prime}_{0} z^{\prime}}} \\ \xi_{{u^{\prime}_{y} j}} & = - inB_{j} (m,n)e^{{ - \alpha s^{\prime}_{j} z^{\prime}}} \\ \xi_{{\sigma^{\prime}_{xx} 0}} & = - 2mnc_{66} B_{0} (m,n)e^{{ - \alpha s^{\prime}_{0} z^{\prime}}} \\ \xi_{{\sigma^{\prime}_{xx} j}} & = \varsigma_{j} B_{j} (m,n)e^{{ - \alpha s^{\prime}_{j} z^{\prime}}} \\ \xi_{{\sigma^{\prime}_{zz} j}} & = \alpha^{2} \eta_{2j} B_{j} (m,n)e^{{ - \alpha s^{\prime}_{j} z^{\prime}}} \\ \xi_{{\sigma^{\prime}_{zx} 0}} & = - in\alpha c^{\prime}_{44} s^{\prime}_{0} B_{0} (m,n)e^{{ - \alpha s^{\prime}_{0} z^{\prime}}} \\ \xi_{{\sigma^{\prime}_{zx} j}} & = im\alpha \eta_{3j} B_{j} (m,n)e^{{ - \alpha s^{\prime}_{j} z^{\prime}}} \\ \xi_{{\sigma^{\prime}_{zy} 0}} & = im\alpha c^{\prime}_{44} s^{\prime}_{0} B_{0} (m,n)e^{{ - \alpha s^{\prime}_{0} z^{\prime}}} \\ \xi_{{\sigma^{\prime}_{zy} j}} & = in\alpha \eta_{3j} B_{j} (m,n)e^{{ - \alpha s^{\prime}_{j} z^{\prime}}} \\ \xi_{{T^{\prime}j}} & = 0,(j = 1,2) \\ \xi_{{T^{\prime}3}} & = \alpha^{2} \eta_{43} B_{3} (m,n)e^{{ - \alpha s_{3} z^{\prime}}} \\ j & = 1,2,3 \\ \end{aligned}$$

(B-2)

where the coefficients $s_{k} ,\alpha_{ij} ,\gamma_{ij} ,\upsilon_{ij} ,s^{\prime}_{j} ,\eta_{ij} ,\varsigma_{k}$ are given in Appendix A.

$$\begin{aligned} D_{1k} & = t_{x} A_{k} (m,n)e^{{ - \alpha s_{k} h}} + t_{x} \overline{{A_{k} }} (m,n)e^{{\alpha s_{k} h}} + B_{k} ,\quad (k = 1,2) \\ D_{13} & = t_{x} A_{3} (m,n)e^{{ - \alpha s_{3} h}} + t_{x} \overline{{A_{3} }} (m,n)e^{{\alpha s_{3} h}} \\ D_{2k} & = t_{z} \alpha_{1k} A_{k} (m,n)e^{{ - \alpha s_{k} h}} - t_{z} \alpha_{1k} \overline{{A_{k} }} (m,n)e^{{\alpha s_{k} h}} - \eta_{1k} B_{k} ,\quad (k = 1,2) \\ D_{23} & = t_{z} \alpha_{13} A_{3} (m,n)e^{{ - \alpha s_{3} h}} - t_{z} \alpha_{13} \overline{{A_{3} }} (m,n)e^{{\alpha s_{3} h}} \\ D_{3k} & = - \alpha_{2k} A_{k} (m,n)e^{{ - \alpha s_{k} h}} + \alpha_{2k} \overline{{A_{k} }} (m,n)e^{{\alpha s_{k} h}} ,\quad (k = 1,2,3) \\ D_{4k} & = \gamma_{1k} A_{k} (m,n)e^{{ - \alpha s_{k} h}} + \gamma_{1k} \overline{{A_{k} }} (m,n)e^{{\alpha s_{k} h}} - \eta_{2k} B_{k} ,\quad (k = 1,2) \\ D_{43} & = \gamma_{13} A_{3} (m,n)e^{{ - \alpha s_{3} h}} + \gamma_{13} \overline{{A_{3} }} (m,n)e^{{\alpha s_{3} h}} \\ D_{5k} & = - \upsilon_{1k} A_{k} (m,n)e^{{ - \alpha s_{k} h}} + \upsilon_{1k} \overline{{A_{k} }} (m,n)e^{{\alpha s_{k} h}} - \eta_{3k} B_{k} ,\quad (k = 1,2) \\ D_{53} & = - \upsilon_{13} A_{3} (m,n)e^{{ - \alpha s_{3} h}} + \upsilon_{13} \overline{{A_{3} }} (m,n)e^{{\alpha s_{3} h}} \\ D_{6k} = & \gamma_{2k} A_{k} (m,n) + \gamma_{2k} \overline{{A_{k} }} (m,n),\quad (k = 1,2,3) \\ D_{7k} & = - \upsilon_{1k} A_{k} (m,n) + \upsilon_{1k} \overline{{A_{k} }} (m,n),\quad (k = 1,2,3) \\ D_{8k} & = \gamma_{1k} A_{k} (m,n) + \gamma_{1k} \overline{{A_{k} }} (m,n),\quad (k = 1,2,3) \\ \end{aligned}$$

(B-3)

The coefficients in the Eqs. (17) are given as follows

$$\begin{aligned} h_{pk} & = w_{{\left( {p + 1} \right)k}} - \frac{{w_{{\left( {p + 1} \right)4}} }}{{w_{14} }}w_{1k} e^{{ - \alpha \left( {s_{k} + s_{1} } \right)h}} ,\quad \left( {p = 1,2,3;k = 1,2,3} \right) \\ h_{p4} & = w_{{\left( {p + 1} \right)5}} - \frac{{w_{{\left( {p + 1} \right)4}} }}{{w_{14} }}w_{15} e^{{ - \alpha s_{1} h}} ,\quad \left( {p = 1,2,3} \right) \\ w_{1r} & = t_{2j} - \frac{{t_{25} }}{{t_{15} }}t_{1j} ,\quad \left( {r = j = 1,2,3,4} \right)w_{15} = t_{26} - \frac{{t_{25} }}{{t_{15} }}t_{16} , \\ w_{lr} & = t_{{\left( {l + 1} \right)j}} - \frac{{t_{{\left( {l + 1} \right)5}} }}{{t_{15} }}t_{1j} e^{{ - \alpha \left( {s_{k} + s_{2} } \right)h}} ,\quad \left( {l = 2,3,4;r = j = k = 1,2,3} \right) \\ w_{l4} & = t_{{\left( {l + 1} \right)4}} - \frac{{t_{{\left( {l + 1} \right)5}} }}{{t_{15} }}t_{14} e^{{\alpha \left( {s_{1} - s_{2} } \right)h}} ,\quad \left( {l = 2,3,4} \right) \\ w_{l5} & = t_{{\left( {l + 1} \right)6}} - \frac{{t_{{\left( {l + 1} \right)5}} }}{{t_{15} }}t_{16} e^{{ - \alpha s_{2} h}} ,\quad \left( {l = 2,3,4} \right) \\ t_{lj} & = g_{lj} + \frac{{g_{l6} \alpha_{2k} }}{{\alpha_{23} }},\quad \left( {k = j = 1,2,3;l = 1,2} \right) \\ t_{lj} & = g_{lj} - \frac{{g_{l6} \alpha_{2k} }}{{\alpha_{23} }},\quad \left( {l = 1,2;k = 1,2;j = k + 3} \right) \\ t_{l6} & = g_{l7} - \frac{{g_{l6} }}{{\alpha_{23} }}g_{3} ,\quad \left( {l = 1,2} \right) \\ t_{3j} & = \gamma_{2k} + \frac{{\gamma_{23} }}{{\alpha_{23} }}\alpha_{2k} e^{{ - \alpha \left( {s_{k} + s_{3} } \right)h}} ,\quad \left( {k = j = 1,2,3} \right) \\ t_{3j} & = \gamma_{2k} - \frac{{\gamma_{23} }}{{\alpha_{23} }}\alpha_{2k} e^{{\alpha \left( {s_{k} - s_{3} } \right)h}} ,\quad \left( {k = 1,2;j = k + 3} \right) \\ t_{36} & = g_{6} - \frac{{\gamma_{23} }}{{\alpha_{23} }}g_{3} e^{{ - \alpha s_{3} h}} \\ t_{4j} & = - \upsilon_{1k} + \frac{{\upsilon_{13} }}{{\alpha_{23} }}\alpha_{2k} e^{{ - \alpha \left( {s_{k} + s_{3} } \right)h}} ,\quad \left( {k = j = 1,2,3} \right) \\ t_{4j} & = \upsilon_{1k} - \frac{{\upsilon_{13} }}{{\alpha_{23} }}\alpha_{2k} e^{{\alpha \left( {s_{k} - s_{3} } \right)h}} ,\quad \left( {k = 1,2;j = k + 3} \right) \\ t_{46} & = g_{7} - \frac{{\upsilon_{13} }}{{\alpha_{23} }}g_{3} e^{{ - \alpha s_{3} h}} \\ t_{5j} & = \gamma_{1k} + \frac{{\gamma_{13} }}{{\alpha_{23} }}\alpha_{2k} e^{{ - \alpha \left( {s_{k} + s_{3} } \right)h}} ,\quad \left( {k = j = 1,2,3} \right) \\ t_{5j} & = \gamma_{1k} - \frac{{\gamma_{13} }}{{\alpha_{23} }}\alpha_{2k} e^{{\alpha \left( {s_{k} - s_{3} } \right)h}} ,\quad \left( {k = 1,2;j = k + 3} \right) \\ \end{aligned}$$

(B-4)

$$\begin{aligned} t_{56} & = g_{8} - \frac{{\gamma_{13} }}{{\alpha_{23} }}g_{3} e^{{ - \alpha s_{3} h}} \\ {\text{g}}_{1j} & = \gamma_{1k} - \frac{{\eta_{21} \left( {t_{z} \alpha_{1k} - \eta_{12} t_{x} } \right)}}{{\eta_{11} - \eta_{12} }} - \frac{{\eta_{22} \left( {t_{z} \alpha_{1k} - \eta_{11} t_{x} } \right)}}{{\eta_{12} - \eta_{11} }},\left( {k = j = 1,2,3} \right) \\ {\text{g}}_{1j} & = \gamma_{1k} - \frac{{\eta_{21} \left( { - t_{z} \alpha_{1k} - \eta_{12} t_{x} } \right)}}{{\eta_{11} - \eta_{12} }} - \frac{{\eta_{22} \left( { - t_{z} \alpha_{1k} - \eta_{11} t_{x} } \right)}}{{\eta_{12} - \eta_{11} }},\left( {k = 1,2,3;j = k + 3} \right) \\ {\text{g}}_{2j} & = - \upsilon_{1k} - \frac{{\eta_{31} \left( {t_{z} \alpha_{1k} - \eta_{12} t_{x} } \right)}}{{\eta_{11} - \eta_{12} }} - \frac{{\eta_{32} \left( {t_{z} \alpha_{1k} - \eta_{11} t_{x} } \right)}}{{\eta_{12} - \eta_{11} }},\left( {k = j = 1,2,3} \right) \\ {\text{g}}_{2j} & = \upsilon_{1k} - \frac{{\eta_{31} \left( { - t_{z} \alpha_{1k} - \eta_{12} t_{x} } \right)}}{{\eta_{11} - \eta_{12} }} - \frac{{\eta_{32} \left( { - t_{z} \alpha_{1k} - \eta_{11} t_{x} } \right)}}{{\eta_{12} - \eta_{11} }},\left( {k = 1,2,3;j = k + 3} \right) \\ g_{17} & = g_{4} + \frac{{\eta_{21} \left( {\eta_{12} g_{1} - g_{2} } \right)}}{{\eta_{11} - \eta_{12} }} + \frac{{\eta_{22} \left( {\eta_{11} g_{1} - g_{2} } \right)}}{{\eta_{12} - \eta_{11} }} \\ g_{27} & = g_{5} + \frac{{\eta_{31} \left( {\eta_{12} g_{1} - g_{2} } \right)}}{{\eta_{11} - \eta_{12} }} + \frac{{\eta_{32} \left( {\eta_{11} g_{1} - g_{2} } \right)}}{{\eta_{12} - \eta_{11} }} \\ g_{1} & = B_{3} - \frac{{t_{x} \eta_{43} }}{{\alpha_{34} }}B_{3} \\ g_{2} & = (\eta_{13} - \frac{{t_{z} \alpha_{14} \kappa^{\prime}_{33} \eta_{43} s^{\prime}_{3} }}{{\kappa_{33} \alpha_{34} s_{4} }})B_{3} \\ g_{3} & = \frac{{\alpha_{24} \kappa^{\prime}_{33} \eta_{43} s^{\prime}_{3} }}{{\kappa_{33} \alpha_{34} s_{4} }}B_{3} \\ g_{4} & = (\eta_{23} - \frac{{\gamma_{14} \eta_{43} }}{{\alpha_{34} }})B_{3} g_{5} = (\eta_{33} + \frac{{\upsilon_{14} \kappa^{\prime}_{33} \eta_{43} s^{\prime}_{3} }}{{\kappa_{33} \alpha_{34} s_{4} }})B_{3} \\ g_{6} & = - \gamma_{24} (A_{4} + \overline{{A_{4} }} ) \\ g_{7} & = \upsilon_{14} (A_{4} - \overline{{A_{4} }} ) + \frac{{imf\mathop p\limits^{ \approx } }}{{\alpha^{3} }} \\ g_{8} & = - \gamma_{14} (A_{4} + \overline{{A_{4} }} ) - \frac{{\mathop p\limits^{ \approx } }}{{\alpha^{2} }} \\ \end{aligned}$$