Skip to main content
SpringerLink
Log in

Predicting dynamic response of large amplitude free vibrations of cantilever tapered beams on a nonlinear elastic foundation

  • Original
  • Published:
Archive of Applied Mechanics Aims and scope Submit manuscript

Abstract

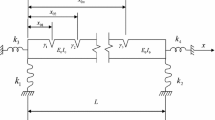
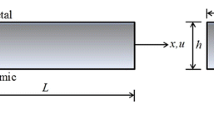
The purpose of this paper is to investigate the nonlinear vibration behavior of single and double tapered cantilever beams on the nonlinear foundation and present an alternative analytical method to solve the issue. Unlike other literatures, the admissible lateral displacement function satisfying the geometric boundary conditions of a single or double tapered cantilever beam is derived by using Rayleigh-Ritz method. Analytical approximate solutions in closed and explicit form are obtained by combining the Lagrange method with the Galerkin and Newton linearization method. Compared with the exact (numeric) results, the accuracy of these approximate solutions is presented. The effects of different parameters such as vibration amplitude, and foundation stiff parameters on nonlinear frequencies and displacement response of the tapered beams are easily analyzed, with the brief expressions of the present analytical approximate solutions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8

Similar content being viewed by others

Abbreviations

x :

The coordinate of the beam neutral axis

y :

The coordinate of the vertical axis

u :

The axial shortening along the beam neutral axis

v :

The transverse deflection v along the vertical axis y

E :

Young’s modulus of the beam

\(\rho \) :

Tensity of the beam

I :

\(={bh^{3}}/{12}\) second moment of the beam cross-section

\(I_0 ,\,I_1 \) :

Moment of inertia at the small end and the large end, respectively

b :

Beam width

h :

Beam thickness

\(L_1 \) :

Beam length

\(b_1 ,\,b_0 \) :

The width of beam at the large end and the small end, respectively

\(h_1 ,\,h_0 \) :

The thickness of beam at the large end and the small end, respectively

\(A_1 \) :

\(=b_1 h_1 ,\) cross-section area of beam at the large end

\(A_0 \) :

\(=b_0 h_0 \) cross-section area of beam at the small end

\(\theta \) :

The angle formed by the tangent from neutral axis to the x axis

\(\tilde{\varphi }\left( s \right) \) :

Single deflection mode

\(q\left( t \right) \) :

An unknown time modulation of the assumed deflection mode

V :

The potential energy of the system

T :

The kinetic energy

\(\kappa \) :

Curvature of the beam neutral axis

L :

The Lagrangian function of the beam

\(\xi \) :

\(=s/{L_1 }\)

\(\varphi \left( \xi \right) \) :

The non-dimensional deflection mode

\(C_1 -C_4 \) :

Arbitrary constants to be determined by Rayleigh-Ritz method

\(\alpha \) :

Taper ratio of the beam

\(\tilde{F}_f \) :

The reaction of the elastic foundation on the beam

\(\tilde{k}_L ,\, \tilde{k}_{\mathrm{NL}} \) :

Linear and nonlinear elastic foundation coefficients, respectively

\(\tilde{k}_s \) :

The coefficient of shear stiffness of the elastic foundation

\(\tilde{\beta }_i ,i=0,1,2,3,4,5\) :

The coefficients of the Lagrangian function of the beam

\(\beta _i ,i=0,1,2,3,4,5\) :

The dimensionless coefficients in Eq. (19)

\(\Theta ,k_s , k_L ,k_{\textit{NL}} \) :

The dimensionless coefficients in Eq. (27)

\(\varepsilon _i ,i=1,2,3,4,5\) :

the dimensionless coefficients in Eq. (31)

\(\tau \) :

\(=t\sqrt{\Omega \Theta }\)

\(\omega \) :

\(=\sqrt{\Omega },\) the dimensionless frequency of nonlinear oscillation

\(\varpi _{\mathrm{La}} , \varpi _L \) :

The approximate and exact fundamental frequency, respectively

\(\omega _{\mathrm{La}} , \omega _L \) :

The dimensionless approximate and exact frequency, respectively

a :

The amplitude of the motion

\(q_i , \omega _i , \Omega _i\), i=1,2 :

The \(i\hbox {th}\) analytical approximation to q,\(\omega \),\(\Omega \)

\(\Delta q_1 ,\, \Delta \Omega _1 \) :

The corrections to \(q_1 (\tau )\) and \(\Omega _1 \left( a \right) \), respectively

\(z_1 \) :

Coefficient to be determined in method of harmonic balance

\(\omega _{\mathrm{e}}\) :

The exact frequency obtained by the improved shooting method

\(\omega _N \) :

The nonlinear vibration frequency of the beam

\(\omega _{\mathrm{LGA}}, \omega _{\mathrm{LGE}}, \omega _{\mathrm{LRR}}\) :

Experimental results from Georgian [29], and the results obtained by Rao and Rao [31], respectively

References

  1. Kienzler, R., Altenbach, H., Ott, I.: Theories of Plates and Shells: Critical Review and New Applications, vol. 16. Springer, Berlin (2004)

    MATH  Google Scholar 

  2. Mohammadimehr, M., Monajemi, A., Moradi, M.: Vibration analysis of viscoelastic tapered micro-rod based on strain gradient theory resting on visco-pasternak foundation using DQM. J. Mech. Sci. Technol. 29(6), 2297–2305 (2015)

    Article  Google Scholar 

  3. Huo, Y., Wang, Z.: Dynamic analysis of a rotating double-tapered cantilever Timoshenko beam. Arch. Appl. Mech. 86(6), 1147–1161 (2016)

    Article  MATH  Google Scholar 

  4. Attarnejad, R., Shahba, A., Eslaminia, M.: Dynamic basic displacement functions for free vibration analysis of tapered beams. J. Vib. Control 17(14), 2222–2238 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  5. Swaddiwudhipong, S., Liu, Z.S.: Response of laminated composite plates and shells. Compos. Struct. 37(1), 21–32 (1997)

    Article  Google Scholar 

  6. Schneider, P., Kienzler, R., Böhm, M.: Modeling of consistent second-order plate theories for anisotropic materials. ZAMM-J. Appl. Math. Mech./Z. Angew. Math. Mech. 94(1–2), 21–42 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  7. Schneider, P., Kienzler, R.: Comparison of various linear plate theories in the light of a consistent second-order approximation. Math. Mech. Solids 20(7), 871–882 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  8. Awrejcewicz, J., Kurpa, L., Shmatko, T.: Investigating geometrically nonlinear vibrations of laminated shallow shells with layers of variable thickness via the R-functions theory. Compos. Struct. 125, 575–585 (2015)

    Article  Google Scholar 

  9. Chauhan, P.K., Khan, I.: Review on analysis of functionally graded material beam type structure. Int. J. Adv. Mech. Eng. 4(3), 299–306 (2014)

    Google Scholar 

  10. Bambill, D.V., Rossit, C.A., Rossi, R.E., Felix, D.H., Ratazzi, A.R.: Transverse free vibration of non uniform rotating Timoshenko beams with elastically clamped boundary conditions. Meccanica 48(6), 1289–1311 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  11. Rajasekaran, S.: Buckling and vibration of axially functionally graded nonuniform beams using differential transformation based dynamic stiffness approach. Meccanica 48(5), 1053–1070 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  12. He, P., Liu, Z.S., Li, C.: An improved beam element for beams with variable axial parameters. Shock Vib. 20(4), 601–617 (2013)

    Article  Google Scholar 

  13. Baghani, M., Mazaheri, H., Salarieh, H.: Analysis of large amplitude free vibrations of clamped tapered beams on a nonlinear elastic foundation. Appl. Math. Model. 38(3), 1176–1186 (2014)

    Article  MathSciNet  Google Scholar 

  14. Fang, J., Zhou, D.: Free vibration analysis of rotating axially functionally graded-tapered beams using Chebyshev-Ritz method. Mater. Res. Innov. 19, 1255–1262 (2015)

    Google Scholar 

  15. Mao, Q.B.: AMDM for free vibration analysis of rotating tapered beams. Struct. Eng. Mech. 54(3), 419–432 (2015)

    Article  Google Scholar 

  16. Ma, J., Peng, J., Gao, X., Xie, L.: Effect of soil-structure interaction on the nonlinear response of an inextensional beam on elastic foundation. Arch. Appl. Mech. 85(2), 273–285 (2015)

    Article  Google Scholar 

  17. Tarnowski, W., Krzyzynski, T., Maciejewski, I., Oleskiewicz, R.: Poly-optimization: a paradigm in engineering design in mechatronics. Arch. Appl. Mech. 81(2), 141–156 (2011)

    Article  MATH  Google Scholar 

  18. Akgoz, B., Civalek, O.: Buckling analysis of linearly tapered micro-columns based on strain gradient elasticity. Struct. Eng. Mech. 48(2), 195–205 (2013)

    Article  MATH  Google Scholar 

  19. Sadeghi, A.: A new investigation for double tapered atomic force microscope cantilevers by considering the damping effect. ZAMM-Z. Angew. Math. Mech. 95(3), 283–296 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  20. Fang, W., Lu, C., Zi, S.L., Jing, L., Ai, Q.L., Xu, M.Z.: Finite element simulation and theoretical analysis of fiber-optical switches. Sens. Actuator A-Phys 96(s 2–3), 167–178 (2002)

    Google Scholar 

  21. Lai, H.Y., Chen, C.K., Hsu, J.C.: Free vibration of non-uniform Euler-Bernoulli beams by the Adomian modified decomposition method. CMES-Comput. Model. Eng. Sci. 34(1), 87–115 (2008)

    MathSciNet  MATH  Google Scholar 

  22. Mehraeen, S., Jagannathan, S., Corzine, K.A.: Energy harvesting from vibration with alternate scavenging circuitry and tapered cantilever beam. IEEE Trans. Ind. Electron. 57(3), 820–830 (2010)

    Article  Google Scholar 

  23. Shahba, A., Rajasekaran, S.: Free vibration and stability of tapered Euler-Bernoulli beams made of axially functionally graded materials. Appl. Math. Model. 36(7), 3088–3105 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  24. Durmaz, S., Demirbag, S.A., Kaya, M.O.: Approximate solutions for nonlinear transverse vibrations of elastically restrained tapered beams. Int. J. Comput. Math. 89(7), 901–915 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  25. Danesh, M., Farajpour, A., Mohammadi, M.: Axial vibration analysis of a tapered nanorod based on nonlocal elasticity theory and differential quadrature method. Mech. Res. Commun. 39(1), 23–27 (2012)

    Article  MATH  Google Scholar 

  26. Wang, C.Y.: Vibration of a tapered cantilever of constant thickness and linearly tapered width. Arch. Appl. Mech. 83(1), 171–176 (2013)

    Article  MATH  Google Scholar 

  27. Wang, C.Y., Wang, C.M.: Analytical solutions for catenary domes. J. Eng. Mech. 141(2), 06014019 (2014)

    Article  Google Scholar 

  28. Rosenberg, R.M.: Nonlinear oscillations. Appl. Mech. Rev. 14, 837–841 (1961)

    MathSciNet  Google Scholar 

  29. Georgian, J.C.: Discussion:“vibration frequencies of tapered bars and circular plates”(Conway, HD, Becker, ECH, and Dubil, JF, 1964, ASME J. Appl. Mech., 31, pp. 329–331). J. Appl. Mech 32(1), 234–235 (1965)

    Article  Google Scholar 

  30. Wagner, H.: Large-amplitude free vibrations of a beam. J. Appl. Mech. 32(4), 887–892 (1965)

    Article  MathSciNet  Google Scholar 

  31. Rao, B.N., Rao, G.V.: Large amplitude vibrations of a tapered cantilever beam. J. Sound Vib. 127(1), 173–178 (1988)

    Article  Google Scholar 

  32. Dugush, Y.A., Eisenberger, M.: Vibrations of non-uniform continuous beams under moving loads. J. Sound Vib. 254(5), 911–926 (2002)

    Article  Google Scholar 

  33. Shahba, A., Attarnejad, R., Semnani, S.J., Gheitanbaf, H.H.: New shape functions for non-uniform curved Timoshenko beams with arbitrarily varying curvature using basic displacement functions. Meccanica 48(1), 159–174 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  34. Raj, A., Sujith, R.I.: Closed-form solutions for the free longitudinal vibration of inhomogeneous rods. J. Sound Vib. 283(3), 1015–1030 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  35. Auciello, N.M., Nole, G.: Vibrations of a cantilever tapered beam with varying section properties and carrying a mass at the free end. J. Sound Vib. 214(1), 105–119 (1998)

    Article  Google Scholar 

  36. Abrate, S.: Vibration of non-uniform rods and beams. J. Sound Vib. 185(4), 703–716 (1995)

    Article  MathSciNet  MATH  Google Scholar 

  37. Sato, K.: Transverse vibrations of linearly tapered beams with ends restrained elastically against rotation subjected to axial force. Int. J. Mech. Sci. 22(2), 109–115 (1980)

    Article  MathSciNet  MATH  Google Scholar 

  38. Karimpour, S., Ganji, S., Barari, A., Ibsen, L.B., Domairry, G.: Nonlinear vibration of an elastically restrained tapered beam. Sci. China Phys. Mech. 55(10), 1925–1930 (2012)

    Article  Google Scholar 

  39. Abdel-Jaber, M.S., Al-Qaisia, A.A., Abdel-Jaber, M., Beale, R.G.: Nonlinear natural frequencies of an elastically restrained tapered beam. J. Sound Vib. 313(3–5), 772–783 (2008)

    Article  Google Scholar 

  40. Sakiyama, T.: A method of analyzing the bending vibration of any type of tapered beams. J. Sound Vib. 101(2), 267–270 (1985)

    Article  Google Scholar 

  41. Katsikadelis, J.T., Tsiatas, G.C.: Non-linear dynamic analysis of beams with variable stiffness. J. Sound Vib. 270(4), 847–863 (2004)

    Article  Google Scholar 

  42. Vo, T.P., Lee, J.: Geometrical nonlinear analysis of thin-walled composite beams using finite element method based on first order shear deformation theory. Arch. Appl. Mech. 81, 419–435 (2011)

    Article  MATH  Google Scholar 

  43. Sapountzakis, E.J., Panagos, D.G.: Nonlinear analysis of beams of variable cross section, including shear deformation effect. Arch. Appl. Mech. 78, 687–710 (2008)

    Article  MATH  Google Scholar 

  44. Baltacıoğlu, A.K., Civalek, Ö., Akgöz, B., Demir, F.: Large deflection analysis of laminated composite plates resting on nonlinear elastic foundations by the method of discrete singular convolution. Int. J. Press. Vessels Pip. 88(8), 290–300 (2011)

    Article  Google Scholar 

  45. Akgöz, B., Civalek, Ö.: A new trigonometric beam model for buckling of strain gradient microbeams. Int. J. Mech. Sci. 81, 88–94 (2014)

    Article  Google Scholar 

  46. Kounadis, A.N., Mallis, J., Sbarounis, A.: Postbuckling analysis of columns resting on an elastic foundation. Arch. Appl. Mech. 75, 395–404 (2006)

    Article  MATH  Google Scholar 

  47. Wu, B.S., Sun, W.P., Lim, C.W.: An analytical approximate technique for a class of strongly non-linear oscillators. Int. J. Nonlinear. Mech. 41(6), 766–774 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  48. Lenci, S., Clementi, F., Mazzilli, C.: Simple formulas for the natural frequencies of non-uniform cables and beams. Int. J. Mech. Sci. 77, 155–163 (2013)

    Article  Google Scholar 

  49. Clementi, F., Demeio, L., Mazzilli, C.E.N., Lenci, S.: Nonlinear vibrations of non-uniform beams by the MTS asymptotic expansion method. Contin. Mech. Thermodyn. 27(4–5), 703–717 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  50. Shames, I.H.: Energy and Finite Element Methods in Structural Mechanics. CRC Press, New York (1985)

    MATH  Google Scholar 

  51. Yu, Y.P., Wu, B.S., Lim, C.W.: Numerical and analytical approximations to large post-buckling deformation of MEMS. Int. J. Mech. Sci. 55(1), 95–103 (2012)

    Article  Google Scholar 

Download references

Acknowledgements

The work described in this paper is supported by the Science and Technology Developing Plan Project of Jilin Province (Grant No. 20160520021JH), the National Natural Science Foundation of China (Grant No. 11402095), and Innovative Project of Scientific Forefront and Interdisciplinary of Jilin University (Grant No. 2013ZY14).

Author information

Authors and Affiliations

  1. College of Construction Engineering, Jilin University, Changchun, 130021, People’s Republic of China

    Yongping Yu, Hongzhi Zhang & Youhong Sun

  2. School of Mathematics, Jilin University, Changchun, 130012, People’s Republic of China

    Weipeng Sun

Authors
  1. Yongping Yu
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hongzhi Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Youhong Sun
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Weipeng Sun
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Weipeng Sun.

Appendix

Appendix

The coefficients of \(B_i \) and \(D_i \left( {i=0,1,2} \right) \) are given by

$$\begin{aligned} B_0= & {} 16a\varepsilon _1 +12\varepsilon _3 a^{3}+10\varepsilon _5 a^{5}-\Omega _1 \left( {16a+8\varepsilon _2 a^{3}+6\varepsilon _4 a^{5}} \right) \\ B_1= & {} -\left( {16a+8\varepsilon _2 a^{3}+6\varepsilon _4 a^{5}} \right) \\ B_2= & {} 16\varepsilon _1 +24\varepsilon _3 a^{2}+25\varepsilon _5 a^{4}+\Omega _1 \left( {-16+5\varepsilon _4 a^{4}} \right) \\ D_0= & {} 4\varepsilon _3 a^{3}+5\varepsilon _5 a^{5}-\Omega _1 \left( {8\varepsilon _2 a^{3}+7\varepsilon _4 a^{5}} \right) \\ D_1= & {} -\left( {8\varepsilon _2 a^{3}+7\varepsilon _4 a^{5}} \right) \\ D_2= & {} -16\varepsilon _1 -12\varepsilon _3 a^{2}-5\varepsilon _5 a^{4}+\Omega _1 \left( {144+56\varepsilon _2 a^{2}+31\varepsilon _4 a^{4}} \right) \end{aligned}$$

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Yu, Y., Zhang, H., Sun, Y. et al. Predicting dynamic response of large amplitude free vibrations of cantilever tapered beams on a nonlinear elastic foundation. Arch Appl Mech 87, 751–765 (2017). https://doi.org/10.1007/s00419-016-1221-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00419-016-1221-x

Keywords

Search

Navigation