On measuring axiom violations due to each tax instrument applied in a real-world personal income tax

Abstract

In their seminal paper, Kakwani and Lambert (Eur J Polit Econ 14:369–380, 1998) state three Axioms an equitable tax system should respect. By proposing a measurement system based on re-ranking indexes of taxes, tax rates and post-tax incomes, they show how to evaluate the negative influences that Axiom violations exert on the redistributive effect of a tax. By considering each element of a real-world personal income tax, i.e. deductions and tax credits as well as statutory tax rates, in this study we take a theoretical step further by decomposing the magnitude of the three Axiom violations produced by all these tax elements. We propose two complementary strategies. The first one is a ‘stepwise’ decomposition computing the effect of each element of the tax on the redistributive effect when they are sequentially applied; the second strategy is an ‘overall and simultaneous’ decomposition always evaluating the effect of small changes in deductions, tax rates and tax credits with respect to the pre-tax income distribution, once all the three tax instruments have been simultaneously applied. These strategies can be more suitable and effective in measuring the loss of the redistributive effect produced by each tax element because of axiom violations. We also show that they can give different information on the existing inequities of the tax. We finally emphasize the goodness of our approach by applying it to a real world personal income tax.

1 Introduction

As Kakwani and Lambert (1998) point out, the redistributive effect achieved by a real-world tax system has to be performed respecting social equity principles. In this respect, the two basic commands of social equity are the equal treatment of equals and the appropriately unequal treatment of unequals. Real world taxes violate these two commands, and the magnitude of their violations depends on several factors, primarly the structure of the tax and the different tax instruments, such as deductions, tax credits and statutory marginal tax rates; as a consequence, the choice of the tax parameters plays a crucial role (Morini and Pellegrino 2018; Pellegrino et al. 2019). Moreover, as Aronson et al. (1994) stress, equity violations should be considered for given specifications of the utility or income relationship.

As it is well known, the overall redistributive effect of a tax, and mainly a personal income tax, is quantified by the difference between the Gini coefficient for pre-tax incomes and the corresponding one observed for post-tax incomes. This overall effect measures the magnitude of the income inequality reduction due to the application of the tax. Kakwani and Lambert (1998) suggest the potential equity of the tax coincides with the redistributive effect that could be achieved were all (vertical and horizontal) inequities abolished by feasible rearrangements of tax parameters able to keep unchanged either the tax revenue or the tax structure. Clearly, the assessment of the potential equity requires a definition of an equitable tax system. These authors also propose an exhaustive approach to measure inequity in taxation. According to their methodology, an equitable tax system should respect three Axioms: taxes should increase monotonically with respect to people’s ability to pay (Axiom 1); richer taxpayers should pay taxes at higher rates (Axiom 2); no re-ranking should occur in people’s living standards (Axiom 3).

Violations of each one of the three Axioms provide the means to characterise the type of inequity present in an income tax system. The benchmark is a perfectly equitable tax system in which all three Axioms are satisfied. The reference distribution could be the pre-tax income one or the living standard one. To properly apply this approach, Kakwani and Lambert (1998) stress that nominal incomes and taxes should be transformed “by means of an equivalence scale, chosen to represent the value judgement of the social policy”. Without a proper “mapping from a household’s size and money income to its per capita living standards” the evaluation of the role of tax rates, allowances, deductions and tax credits would be misleadingly evaluated. Of course, as the Authors recall, different equivalence scales will in general lead to different assessments; however, as they observe, the alternative way would be to accept “that the tax system must by definition be treating families of different sizes equitably with respect to each other”.¹ Moreover, by considering the net tax liability distribution, the tax rate distribution and, finally, the post-tax income distribution, Kakwani and Lambert (1998) suggest that the extent of each overall Axiom violations can be measured by the Atkinson-Kakwani-Plotnick re-ranking index of each distribution. By applying these re-ranking indexes as well as considering the Kakwani (1977) progressivity index and the Kakwani (1984) decomposition of the redistributive effect, Kakwani and Lambert (1998) evaluate the implicit or potential equity in the tax system reachable in the absence of inequities.

In this paper we maintain the original Kakwani and Lambert (1998) definition of equity in income taxation by means of the three Axioms and we take a theoretical step further by measuring the magnitude of each Axiom violations produced by each tax element (deductions, tax credits and statutory marginal tax rates). We propose two complementary strategies to decompose each Axiom violations; these methodologies seem to be suitable to explore which features of the tax contribute the most to the removal of a real-world tax from a perfectly equal one. The first one is a ‘stepwise’ decomposition computing the effect of each element of the tax (namely deductions, tax rates and tax credits) on the redistributive effect when they are sequentially applied; the second strategy is an ‘overall and simultaneous’ decomposition always evaluating the effect of small changes in deductions, tax rates and tax credits with respect to the pre-tax income distribution, once the three tax instruments have been simultaneously applied. In our opinion these strategies can be more suitable and effective in measuring the loss of the redistributive effect produced by each tax element because of axioms violations, and they can also give different information on the existing inequities of the tax.

Our methodologies differ from the existing literature and focus on two innovations. While tax credits exert a direct effect on the net tax liability, deductions exert an indirect one since their application influences the tax base definition that in turn affects the tax saving in presence of several marginal tax rates. We then do not compare the inequality of the pre-tax distribution with the tax base one, but we compute the effect of deductions by comparing the gross tax liability when calculated on the tax base with the hypothetical one obtained by applying the tax rates to the whole pre-tax income. In so doing we properly isolate the impact that deductions exert on the net tax liability. We apply these new approaches to a simple theoretical distribution of incomes in order to both assess their potentialities and show how the ‘step by step’ decomposition and the ‘overall’ one can lead to contrasting results. Finally, we also apply these methodologies to a real-world personal income tax.

The structure of the remainder of the paper is as follows. Section 2 first introduces the notation used throughout; Sect. 3 summarises the existing literature and presents the three Axioms proposed by Kakwani and Lambert (1998) when the whole tax structure is considered. Section 4 presents our methodology explaining how Axiom violations can be decomposed by tax components. Section 5 first presents the data and the microsimulation model employed in the empirical analysis (Sect. 5.1); it then shows the results when considering the Italian personal income tax (Sect. 5.2). Section 6 concludes.

2 Starting definitions

Considering a generic personal income tax, we start by defining all the variables influencing the transition from pre- to post-tax income. A population of N income earners, with $i=(1,\dots ,N)$, is considered. Let $x_i$ be the taxpayer i’s gross income. The corresponding taxable income $b_i$ is given by the difference between gross income $x_i$ and all taxpayer i’s allowances and deductions $d_{i}$, so that $b_i=x_i-d_i$.²

Let $f(b_i)$ be the gross tax liability produced by applying the marginal tax rates³ and $r(b_i)=\frac{f(b_i)}{b_i}$. We can express the gross tax liability as $s_i= r(b_i)b_i$, where $r(b_i)$ is the average tax rate corresponding to the taxable income $b_i$. Were the marginal tax rates applied to $x_i$ instead to $b_i$, the resulting tax liability would be $v_i= r(x_i)x_i$, being $r(x_i)$ the average tax rate corresponding to the taxable income $x_i$. When the rate schedule is not linear and the taxpayer can benefit from deductions, $r(x_i)$ is greater than $r(b_i)$. The difference between $v_i$ and $s_i$ depends on both $d_i$ and $r(x_i) - r(b_i)$, so that $v_i-s_i= (r(x_i)- r(b_i))x_i+ r(b_i)d_i$. Finally, the net tax liability $t_i$ is equal to the gross tax liability $s_i$ minus all the tax credits $c_i$ from which taxpayer i can benefit. It follows that the net tax liability is computed as $t_i = r(b_i)(x_i-d_i)-c_i$.

We denote by $X=(x_1,\dots ,x_N)$ the gross income distribution ordered in non decreasing order. Similarly, we call $D=(d_1,\dots ,d_N)$ the distribution of tax allowances and deductions, $B=X-D=(b_1,\dots ,b_N)$ the tax base distribution, $S=(s_1,\dots ,s_N)$ the distribution of gross tax liabilities, and $V=(v_1,\dots ,v_N)$ the distribution of gross tax liabilities were the rate schedule applied to the X distribution instead of the B one; finally, we denote by $C=(c_1,\dots ,c_N)$ the overall tax credit distribution, by $T=(t_1,\dots ,t_N)$ the net tax liability distribution, and by $Z=X-T=(z_1,\dots ,z_N)$ the post-tax distribution.

Let’s consider an attribute $E=(\epsilon _1,\dots ,\epsilon _N)$ in which quantities are ordered in non decreasing order ($\epsilon _{i-1} \le \epsilon _{i}$ $\forall i$). To evaluate the inequality within these distributions, we employ the Gini (1914) coefficient $G_E={2\mu _E}^{-1}cov\big (E,F(E)\big )$, where $\mu _E$ is the average value of the considered distribution, cov represents the covariance, and F(E) is the cumulative distribution function (Kakwani 1980; Jenkins 1988).

Let’s consider another attribute $H=(\eta _1,\dots ,\eta _N)$ related to the same set of N statistical units. The attribute H can be characterised by a different distribution than the attribute E, so that F(H) does not necessarily coincide with F(E). As a consequence, for every possible pair of statistical units (i, j), it is not granted that $\eta _i$ and $\eta _j$ are similarly ranked as $\epsilon _i$ and $\epsilon _j$. We define $C_{H|E}=2{\mu _H}^{-1}cov\big (H,F(E)\big )$ the concentration coefficient, so that $C_{H|E}$ is evaluated for the attribute H when the values of H are ranked according to the non decreasing order of the attribute E. The difference between $G_H$ and $C_{H|E}$ measures to which extent the non decreasing ordering of the attribute H differs from the non decreasing ordering of the attribute E, when dealing with the same set of N statistical units.

Following the existing literature (Kakwani 1977; Reynolds and Smolensky 1977; Lambert 2001), the overall redistributive effect is measured by $RE=G_X-G_Z=RS-R_{Z|X}$, where $RS=G_X-C_{Z|X}$ is the Reynolds–Smolensky index and $R_{Z|X}=G_Z-C_{Z|X}$ is the Atkinson–Plotnick–Kakwani index, which computes the extension of the re-ranking of post-tax incomes with respect to pre-tax ones (Atkinson 1980; Plotnick 1981; Kakwani 1984).⁴ Similarly, the overall degree of tax progressivity is measured using the Kakwani index $K=C_{T|X}-G_X$. As it is well known, RS and K are linked by the overall average tax rate $\theta =\frac{\sum _{i=1}^N t_i}{\sum _{i=1}^N x_i}$, since $RS=\frac{\mu _T}{\mu _Z}K$ and $\frac{\mu _T}{\mu _Z}=\frac{\theta }{1-\theta }$.

3 Considering axiom violations for the whole tax structure

3.1 Non-technical explanation

As observed, the overall redistributive effect of a (personal income) tax RE measures the magnitude of the income inequality reduction due to the application of the tax. It is quantified by the difference between the Gini coefficient for the pre-tax incomes and the corresponding one observed for the post-tax incomes, that is $RE=G_X-G_Z$. For each pre-tax income, the corresponding post tax one primarily depends on the tax structure. This means that in the transition from the pre- to the post-tax distribution the tax should be performed respecting social equity principles. In this respect, the two basic commands of social equity are the equal treatment of equals and the appropriately unequal treatment of unequals.

In their seminal study, Kakwani and Lambert (1998) define three Axioms that should be respected by an equitable income tax: the tax liability should increase monotonically with respect to people’s ability to pay (Axiom 1); richer taxpayers should pay taxes at higher rates (Axiom 2); no re-ranking should occur in people’s living standards (Axiom 3). In technical notation it means that for each observed pair of values $x_i \ge x_j$, the first Axiom requires that $t_i \ge t_j$ (minimal progression), the second Axiom demands $\tilde{t_i}=\frac{t_i}{x_i} \ge \frac{t_j}{x_j}=\tilde{t_j}$, where $\tilde{t_i}=\frac{t_i}{x_i}$ is taxpayer i’s average tax rate (progressive principle), and Axiom 3 requests $z_i \ge z_j$ (no re-ranking). The first two Axioms are related to vertical equity, that is the unequal treatment of unequals, whilst the last one is related to horizontal equity.

Unfortunately, real world taxes violate these two commands, that is the three Axioms. Kakwani and Lambert (1998) suggest the potential equity of the tax $RE^P$ coincides with the redistributive effect that could be achieved were all (vertical and horizontal) inequities abolished by feasible rearrangements of tax parameters able to keep unchanged either the tax revenue or the tax structure. In order to obtain the actual redistributive effect RE, the magnitude of each Axiom violations ($AV_1, AV_2, AV_3$) has to be subtracted by the potential redistributive effect $RE^P$. Following this approach, the overall redistributive effect RE can be decomposed as:

$$\begin{aligned} RE=RE^P-AV_1-AV_2-AV_3. \end{aligned}$$

(1)

3.2 Technical explanation

Employing the re-ranking indexes of taxes $R_{T|X}=G_T-C_{T|X}$, tax rates $R_{\tilde{T}|X}=G_{\tilde{T}}-C_{\tilde{T}|X}$ (with $\tilde{T}=\frac{T}{X}=\tilde{t_1},\dots , \tilde{t_N}$), and post-tax incomes $R_{Z|X}=G_Z-C_{Z|X}$, the authors measure⁵ the negative influences of each Axiom violations. More precisely, Kakwani and Lambert (1998) quantify the overall Axiom 1 violations as:

$$\begin{aligned} AV_1=\frac{\mu _T}{\mu _Z}R_{T|X}, \end{aligned}$$

(2)

whereas gross violations of Axiom 2 can be quantified as⁶

$$\begin{aligned} AV_2=\frac{\mu _T}{\mu _Z}R_{\tilde{T}|X}, \end{aligned}$$

(3)

net violations of Axiom 2 as

$$\begin{aligned} AV_{2N}=\frac{\mu _T}{\mu _Z}\Big (R_{\tilde{T}|X}-R_{T|X}\Big )=AV_2-AV_1, \end{aligned}$$

(4)

and violations of Axiom 3 as

$$\begin{aligned} AV_3=R_{Z|X}. \end{aligned}$$

(5)

In particular, the overall potential redistributive effect $RE^P$ can be evaluated as

$$\begin{aligned} RE^P=\frac{\mu _T}{\mu _Z} \Bigg ( K + \Big (R_{\tilde{T}|X}-R_{T|X}\Big ) +R_{T|X}\Bigg ). \end{aligned}$$

(6)

By adding the tax re-ranking index to the Kakwani (1977) progressivity index, they evaluate the potential equity that the tax system would reach in the absence of Axiom 1 violations. Analogously, by adding the tax-rate re-ranking index to the Kakwani progressivity index, they estimate the potential equity which the tax system would reach in the absence of Axiom 2 violations, that is to say, in the absence of the progressive principle violations. Finally, the usual Atkinson–Plotnick–Kakwani index evaluates Axiom 3 violations.

3.3 A stylized example

Suppose the existence of four individuals representing a collectivity. Each individual is the only person with a positive income within each household. Their nominal pre-tax incomes are, respectively, 500, 600, 700 and 1.000 euros. The tax strucure is very simple: a deduction $d_i$, with $i=(1,2,3,4)$, equal to 100 euros is applied to everyone; the rate schedule is as follows: 20% up to 600 euros, 30% between 600 and 750 euros, 40% between 750 an 900 euros and 50% above 900 euros; since each individual belongs to a household with different characteristics, tax credits are different: 25 euros for the poorest taxpayer, 15 for the second one, 7 for the third and 150 for the richest one. Table 1 shows how each taxpayer evaluates the transition from the pre- to the post-tax income.

Table 1 The personal income tax

Since we image the household composition be different among the four households, in order to compute equivalent values we employ an equivalence scale as shown in Table 2.

Table 2 Equivalent values

As can be noted, given equivalent pre-tax incomes ordered in non decreasing order, deductions and tax bases as well as post-tax incomes are still ordered in non decreasing order, whilst the opposite occurs for gross tax liabilities, tax credits, net tax liabilities and average tax rates.

In particular, $\mu _T=53.23512$, $\mu _Z=359.26488$, $K=0.11140$, $R_{T|X}=0.01311$ and $R_{\tilde{T}|X}=0.03244$. From Eq. (6) we get $RE^P=0.02326$. From Eq. (1) we know that $RE^P-RE=AV_1+AV_2+AV_3$, with $RE=G_X-G_Z=0.14394-0.12743=0.01651$. Equation (5) tells us that $AV_3=0$, since no reranking occurs in the transition from the pre- to the post-tax incomes. All inequities are then due to Axiom 1 and 2 violations: $AV_1=0.00194$ and $AV_2=0.00481$.

In this simple example, Kakwani and Lambert (1998) methodology underlines that inequities depend on the distribution of tax liabilities and average tax rates, when compared with the pre-tax income distribution. Why is this? Since all tax instruments (deductions, statutory marginal tax rates and tax credits) influence each taxpayer’s net tax liability, which of them contribute the most to Axiom 1 and 2 violations? Which parameters of the tax should be changed in order to reduce Axiom violations? We try to answer these questions in the following part of the paper.

4 Decomposing axiom violations

As observed, the personal income tax structure is composed by three main tax instruments: deductions, statutory marginal tax rates and tax credits. The transition from the pre- to the post-tax income depends on all of them. In particular, deductions and tax credits modify the gross tax liability that would be generated were only the statutory marginal tax rates applied to the pre-tax income. Motivations for introducing deductions and tax credits in addition to the statutory marginal tax rates are numerous and not necessarily consequent to the realization of a tax that takes into account in such a way the rationale of the ability to pay and the principles of horizontal and vertical equity. In the next subparagraphs we try to explain their effect, proposing a ‘step by step’ as well as an ‘overall’ decomposition.

4.1 The ‘step by step’ decomposition

4.1.1 Non-technical explanation

Being interested in evaluating the fairness of the tax, one can imagine that deductions first, and tax credits subsequently, correct the effect of the tax liability that would have been obtained by applying only the rate schedule. In principle, the rate schedule should be the tax instrument allowing tax progressivity, and in particular vertical equity, were all taxpayers homogeneous, that is with the same personal characteristics. On the contrary, in order to properly achieve fairness, deductions and tax credits have to be applied in order to take into account the different and taxpayer-specific abilities to pay, since taxpayers are heterogeneous.

Evaluating the effect of the statutory marginal tax rates is not problematic, since they can be applied to each taxpayer’s pre-tax income. Similarly for tax credits: their effect can be measured by simply subtracting them from the gross tax liability in order to obtain the net tax liability. The same does not hold for deductions, since marginal tax rates that increase with income cause a non-linear effect of deductions on each taxpayer’s net tax liability. As a consequence, understanding how deductions behave is not obvious. A reasonable way to take into account their effect is to apply statutory marginal tax rates twice: a first time to the pre-tax income; a second time to the tax base, that is the pre-tax income minus the deductions.

We first evaluate the effect due to the rate schedule V itself (i.e. the transition from distribution X to distribution $X-V$); we then calculate the effect due to deductions and allowances D (i.e. the transition from distribution V to distribution S, which also explains the transition from distribution X to distribution $X-S$); finally, we consider the effect due to tax credits C (i.e. the transition from distribution S to distribution T). Since the structure of a real-world tax may be different (and specifically it is) from a perfectly equal one, primarily if the structure of each tax component is not consistent with the adopted equivalent scale, our approach is able to assess the incremental effect of Axiom violations due to the rate schedule and deductions as well as tax credits.

Following this line of reasoning, we propose a first approach, that we call ‘Step by Step’, in order to evaluate $AV_1$, $AV_2$ and $AV_3$, as shown in Eqs. (2), (3) and (5): in the first step we compute these equations by applying the rate schedule to the pre-tax income distribution (Eqs. (8), (13) and (18)); the second step is to evaluate the variation of each Axiom violations by applying the rate schedule to the tax base distribution (Eqs. (9), (14) and (19)). In so doing we measure the corrective effect due to deductions (Eqs. (10), (15) and (20)). As a consequence, the variation of each Axiom violations due to tax credits can be simply measured by subtracting each overall Axiom violations to the part of Axiom violations due to both rate schedule and deductions (Eqs. (11), (16) and (21)).

Note that this approach is dynamic. Starting from a hypotetical tax structure composed by only statutory marginal tax rates, applying or not applying other tax mechanisms, such as income splitting or family quotient, we move on to a more complex tax structure which takes into account income components (in case of deductions) as well as other items of expenditure (in case of tax credits) which, depending on the household caracteristics, are fair to be deducted from the pre-tax income or from the gross tax liability.

An intuitive explanation of the ‘step by step’ decomposition can help the reader understand the tecnical details presented in the next subsection. Focusing on Axiom 1 violation due to V (Eq. (8)), suppose that the ordering of V and X is the same. If this is the case, no reranking occurs since $G_V=C_{V|X}$ and $R_{V|X}=0$, so that no Axiom 1 violation can be registered. Now, suppose that the ordering of V is different with respect the one observed for X. In this case $G_V>C_{V|X}$, and the magnitude of $AV_1^V$ depends on both $R_{V|X}$ and the ratio $\frac{\mu _V}{\mu _{X-V}}$. Ceteris paribus, the higher $\frac{\mu _V}{\mu _{X-V}}$, the higher $AV_1^V$. On the contrary, for a given $\frac{\mu _V}{\mu _{X-V}}$, the higher the re-ranking between distributions X and V, the higher $AV_1^V$. All the other relevant equations have the same explanation.

4.1.2 Technical details

Our goal is to decompose the indexes defined by Eqs. (2), (3) and (5), which quantify each of the overall Axiom violations, into the three parts highlighting the effect of statutory marginal tax rates, deductions, and tax credits, respectively.

For each Axiom, we show how its overall violations can be decomposed by the parts due to V, S, D, and C (for a generalization of this effect when several rate shedules are applied see Appendix A1, A2 and A3). Starting from Axiom 1, we state that

$$\begin{aligned} AV_1=AV_1^{V}+AV_1^{D}+AV_1^{C}. \end{aligned}$$

(7)

In particular,

$$\begin{aligned} AV_1^{V}= & {} \frac{\mu _V}{\mu _{X-V}}R_{V|X}, \end{aligned}$$

(8)

$$\begin{aligned} AV_1^{S}= & {} \frac{\mu _S}{\mu _{X-S}}R_{S|X}, \end{aligned}$$

(9)

$$\begin{aligned} AV_1^{D}= & {} AV_1^{S}-AV_1^{V}, \end{aligned}$$

(10)

$$\begin{aligned} AV_1^{C}= & {} AV_1-AV_1^{S}. \end{aligned}$$

(11)

Having generically defined $\tilde{E}=\frac{E}{X}$ and $R_{\tilde{E}|X}=G_{\tilde{E}}-C_{\tilde{E}|X}$, we similarly evaluate the overall gross violations of Axiom 2 as follows:

$$\begin{aligned} AV_2=AV_2^{V}+AV_2^{D}+AV_2^{C}, \end{aligned}$$

(12)

where

$$\begin{aligned} AV_2^{V}= & {} \frac{\mu _{V}}{\mu _{X-V}}R_{\tilde{V}|X}, \end{aligned}$$

(13)

$$\begin{aligned} AV_2^{S}= & {} \frac{\mu _{S}}{\mu _{X-S}}R_{\tilde{S}|X}, \end{aligned}$$

(14)

$$\begin{aligned} AV_2^{D}= & {} AV_2^{S}-AV_2^{V}, \end{aligned}$$

(15)

$$\begin{aligned} AV_2^{C}= & {} AV_2-AV_2^{S}. \end{aligned}$$

(16)

For what concerns⁷ Axiom 3,

$$\begin{aligned} AV_3=G_{Z}-C_{Z|X}, \end{aligned}$$

(17)

where

$$\begin{aligned} AV_3^{V}= & {} G_{X-V}-C_{(X-V)|X}, \end{aligned}$$

(18)

$$\begin{aligned} AV_3^{S}= & {} G_{X-S}-C_{(X-S)|X}, \end{aligned}$$

(19)

$$\begin{aligned} AV_3^{D}= & {} AV_3^{S}-AV_3^{V}, \end{aligned}$$

(20)

$$\begin{aligned} AV_3^{C}= & {} AV_3-AV_3^{S}. \end{aligned}$$

(21)

4.1.3 A stylized example

By considering the example of Paragraph 3.3, we can observe that in Table 2 the ordering of V is different from the ordering of X: for example, the bottom equivalent income is $x_1=300$ to which is associated $v_1=82.50$, but $x_2=350>x_1$ and $v_2=70<v_1$. As a consequence, $R_{V|X}$ is expected to be positive. We can compute both $G_V$ and $C_{V|X}$. They are 0.12339 and 0.10262, respectively, so that $R_{V|X}=0.02077$. In addition to $R_{V|X}$, to estimate $AV_1^V$ we have to consider the magnitude of $\frac{\mu _V}{\mu _{X-V}}$, that is 0.28013. We get $AV_1^V=0.00582$.

We can now consider the application of the deductions D and repeat the same calculations on the distributions S and X. We get $R_{S|X}=0.02164$ and $\frac{\mu _S}{\mu _{X-S}}=0.21564$, so that $AV_1^S=0.00467$. Note that $AV_1^S<AV_1^V$: this relation depends on the low increase of the reranking terms when V and S are considered ($R_{V|X}<R_{S|X}$) and the remarkable decrease of the corresponding ratios $\frac{\mu _V}{\mu _{X-V}}>\frac{\mu _S}{\mu _{X-S}}$.

Finally, focusing on tax credits C, their trend is fluctuating: to $x_1=300$ is associated $c_1=45$, to $x_2=350$ is associated $c_2=8.75$, to $x_3=400$ is associated $c_3=20$ and to $x_4=600$ is associated $c_4=6$. Given this distribution, the contribution due to C is negative, saying that tax credits help containing Axiom 1 violation: $AV_1^{C}=AV_1-AV_1^{S}=-0.00272$. Note that $AV_1=0.00194=AV_1^V+AV_1^D+AV_1^C=0.00582-0.00115-0.00272$, showing a large violation due to V, and an impact that is reduced thanks to both D and C.

The same can be observed for Axiom 2 violation: $AV_2=0.00481 =AV_2^V+AV_2^D+AV_2^C=0.03319 - 0.00582 - 0.02256$.

4.2 The ‘overall and symultaneous‘ or ‘ex post’ approach

4.2.1 Non-technical explanation

The decompositions in Sect. 4.1 show how the ‘step by step’ or ‘ex ante’ application of tax instruments sequentially modifies the redistributive effect RE and influences Axiom violations. This strategy measures the incremental effect of the redistributive effect due to deductions, deductions and the rate schedule, and, finally, deductions and the rate schedule as well as tax credits (i.e. the whole tax structure).

In this Paragraph, we consider the end of the story and focus on the ‘overall and simultaneous’ or ‘ex post’ decomposition. This allows us to measure Axiom violations due to each tax component given the application of all the other tax components. Such a strategy can be also useful in evaluating the fairness or the goodness of tax reform, since this metodology helps us understanding the effect of changes in deductions or tax rates or tax credits, once the three tax instruments have been simultaneously applied. For example, it could be of interest to understand the effect that statutory marginal tax rates exert when combined with the application of deductions and tax credits; or it could be of interest to understand the effect that tax credits exert when combined with the application of deductions and statutory marginal tax rates. Generally speaking, it can be more relevant understanding the final effect produced by a specific tax instrument when interacting with all the other ones.

An intuitive explanation of the ‘ex post’ decomposition can now be presented. We define Axiom 1 violation due to V as $AAV_1^V$; similarly for the other indexes: $AAV_1^S$, $AAV_1^D$ and so on. Let’s focus on $AAV_1^V$. As stated by Eq. (2), Axiom 1 violations occur if the ordering of distribution T is different from the ordering of distribution X. In this case the contribution of each of the three tax instruments (V, D and C) to Axiom 1 violations depends not only on how distribution V, distribution $V-S$ and distribution C relate with distribution X as well as distribution T, but also on their sizes relative to distribution T and distribution X. If the ordering of distribution T is equal to the ordering of distribution X, then this axiom violation cannot be registered, whatever the ordering of distributions V, $V-S$ and C is.

Now, let’s consider a situation in which Axiom 1 violations are detected, due to a different ordering of distribution T with respect to distribution X. Suppose that the ordering of V is still equal to the ordering of X, but it is remarkably different from the ordering of T. In this case, $AAV_1^V$ is negative. Finally, suppose that the ordering of V is equal to the ordering of T, but it is remarkably different from the ordering of X. In this case, $AAV_1^V$ is positive. Generally speaking, the magnitude of the sign of $AAV_1^V$ depends on how different the orderings are: ceteris paribus, the more the ordering of V is similar to the ordering of X, the more $AAV_1^V$ is negative; conversely, the more the ordering of V is similar to the ordering of T, the more $AAV_1^V$ is positive. This is the information we get from Eq. (25), presented in the next subsection.

The rationable behind all the other equations for what concerns the three Axioms, shown in the tecnical details section, is similar. Focusing on Axiom 1, since deductions (tax credits) have to be subtracted from the pre-tax incomes (gross tax liabilities), the explanation of Eqs. (27) and (28) is the opposite of the explanation of Eq. (25): the more the ordering of $V-S$ (C) is similar to the ordering of X, the more $AAV_1^D$ ($AAV_1^C$) is positive; conversely, the more the ordering of $V-S$ (C) is similar to the ordering of T, the more $AAV_1^S$ ($AAV_1^C$) is negative. Analogous interpretetions apply, ceteris paribus, for what concerns the effect of the statutory marginal tax rates, deductions and tax credits on Axiom 2 and 3 violations.

Following this line of reasoning, we basically focus on the final equilibrium generated by the joint interaction of the three tax instruments. Differently from the ‘Step by Step’ decomposition, due to action and feedback effects of the tax instruments, the final equilibrium is not easily predictable in advance. This imply that nothing can be ‘a priori’ said on the sign of the composition of Axiom violations, as we discuss in greater detail in Sects. 5.2.2 and 5.2.3.

4.2.2 Technical details

For what concerns Axiom 1, we start again from Eq. (2): $AV_1=\frac{\mu _T}{\mu _Z}R_{T|X}$. Remembering the strategy originally proposed by Rao (1969), also employed by Podder (1993a, 1993b) and Podder and Chatterjee (2002), we decompose $G_T$ and $C_{T|X}$ as follows:

$$\begin{aligned} G_T= & {} \frac{\mu _V}{\mu _T}C_{V|T}-\frac{\mu _{V-S}}{\mu _T}C_{(V-S)|T}-\frac{\mu _C}{\mu _T} C_{C|T} \end{aligned}$$

(22)

$$\begin{aligned} C_{T|X}= & {} \frac{\mu _V}{\mu _T}C_{V|X}-\frac{\mu _{V-S}}{\mu _T}C_{(V-S)|X}-\frac{\mu _C}{\mu _T} C_{C|X}. \end{aligned}$$

(23)

By employing the Gini correlation coefficient $r_{E|H}=\frac{C_{E|H}}{G_E}$, which evaluates the rank of E with respect to the rank of H, the following Eqs. hold for Axiom 1 (for a generalization of this effect when several rate shedules are applied see Appendix B1, B2 and B3):

$$\begin{aligned} AV_1= & {} AAV_1^V+AAV_1^D+AAV_1^C=AAV_1^S+AAV_1^C\nonumber \\= & {} \frac{\mu _T}{\mu _Z}\Bigg (\frac{\mu _V}{\mu _T}\Big (r_{V|T}-r_{V|X}\Big )G_V -\frac{\mu _{V-S}}{\mu _T}\Big (r_{(V-S)|T}-r_{(V-S)|X}\Big )G_{V-S} -\frac{\mu _C}{\mu _T}\Big (r_{C|T}-r_{C|X}\Big )G_C\Bigg ). \end{aligned}$$

(24)

In particular,

$$\begin{aligned} AAV_1^{V}= & {} \frac{\mu _V}{\mu _{Z}}(r_{V|T}-r_{V|X})G_V, \end{aligned}$$

(25)

$$\begin{aligned} AAV_1^{S}= & {} AAV_1^{V}+AAV_1^{D}, \end{aligned}$$

(26)

$$\begin{aligned} AAV_1^{D}= & {} -\frac{\mu _{V-S}}{\mu _{Z}}\Big (r_{(V-S)|T}-r_{(V-S)|X}\Big )G_{(V-S)}, \end{aligned}$$

(27)

$$\begin{aligned} AAV_1^{C}= & {} -\frac{\mu _C}{\mu _{Z}}\Big (r_{C|T}-r_{C|X}\Big )G_C=AV_1-AAV_1^{S}. \end{aligned}$$

(28)

Continuing with the second Axiom,

$$\begin{aligned} AV_2= & {} \frac{\mu _T}{\mu _Z}R_{\tilde{T}|X}=AAV_2^V+AAV_2^D+AAV_2^C=AAV_2^S+AAV_2^C\nonumber \\= & {} \frac{\mu _T}{\mu _Z}\Bigg (\frac{\mu _{\tilde{V}}}{\mu _{\tilde{T}}} \Big (r_{\tilde{V}|\tilde{T}}-r_{\tilde{V}|X}\Big )G_{\tilde{V}} -\frac{\mu _{{\tilde{V-S}}}}{\mu _{\tilde{T}}}\Big (r_{(\tilde{V-S})|\tilde{T}} -r_{(\tilde{V-S})|X}\Big )G_{\tilde{V-S}}-\frac{\mu _{\tilde{C}}}{\mu _{\tilde{T}}} \Big (r_{\tilde{C}|\tilde{T}}-r_{\tilde{C}|X}\Big )G_{\tilde{C}}\Bigg ). \end{aligned}$$

(29)

In particular,

$$\begin{aligned} AAV_2^{V}= & {} \frac{\mu _T}{\mu _Z}\frac{\mu _{\tilde{V}}}{\mu _{\tilde{T}}}\Big (r_{\tilde{V}|\tilde{T}}-r_{\tilde{V}|X}\Big )G_{\tilde{V}}, \end{aligned}$$

(30)

$$\begin{aligned} AAV_2^{S}= & {} AAV_2^{V}+AAV_2^{D}, \end{aligned}$$

(31)

$$\begin{aligned} AAV_2^{D}= & {} -\frac{\mu _T}{\mu _Z}\frac{\mu _{{\tilde{V-S}}}}{\mu _{\tilde{T}}}\Big (r_{(\tilde{V-S})|\tilde{T}}-r_{(\tilde{V-S})|X}\Big )G_{\tilde{V-S}}, \end{aligned}$$

(32)

$$\begin{aligned} AAV_2^{C}= & {} -\frac{\mu _T}{\mu _Z}\frac{\mu _{\tilde{C}}}{\mu _{\tilde{T}}}\Big (r_{\tilde{C}|\tilde{T}}-r_{\tilde{C}|X}\Big )G_{\tilde{C}}. \end{aligned}$$

(33)

Finally, focusing on the last Axiom, we start by considering the decompositions of $G_Z$ and $C_Z$:

$$\begin{aligned} G_Z= & {} \frac{\mu _{(X-V)}}{\mu _Z}C_{(X-V)|Z}+\frac{\mu _{(V-S)}}{\mu _Z}C_{(V-S)|Z}+\frac{\mu _{C}}{\mu _Z}C_{C|Z}, \end{aligned}$$

(34)

$$\begin{aligned} C_{Z|X}= & {} \frac{\mu _{(X-V)}}{\mu _Z}C_{(X-V)|X}+\frac{\mu _{(V-S)}}{\mu _Z}C_{(V-S)|X}+\frac{\mu _{C}}{\mu _Z}C_{C|X}. \end{aligned}$$

(35)

Computing their difference, $AV_3$ follows:

$$\begin{aligned} \begin{aligned} AV_3&=\frac{\mu _{X-V}}{\mu _Z}\Big (r_{(X-V)|Z}-r_{(X-V)|X}\Big )G_{X-V}\\&\quad +\frac{\mu _{V-S}}{\mu _Z}\Big (r_{(V-S)|Z}-r_{(V-S)|X}\Big )G_{V-S}+\frac{\mu _C}{\mu _Z}\Big (r_{C|Z}-r_{C|X}\Big )G_C. \end{aligned} \end{aligned}$$

(36)

In particular,

$$\begin{aligned} AAV_3^V= & {} \frac{\mu _{X-V}}{\mu _Z}\Big (r_{(X-V)|Z}-r_{(X-V)|X}\Big )G_{X-V}, \end{aligned}$$

(37)

$$\begin{aligned} AAV_3^{S}= AAV_3^{V}+AAV_3^{D}, \end{aligned}$$

(38)

$$\begin{aligned} AAV_3^{D}= & {} \frac{\mu _{V-S}}{\mu _Z}\Big (r_{(V-S)|Z}-r_{(V-S)|X}\Big )G_{V-S}, \end{aligned}$$

(39)

$$\begin{aligned} AAV_3^{C}= & {} \frac{\mu _C}{\mu _Z}\Big (r_{C|Z}-r_{C|X}\Big )G_C. \end{aligned}$$

(40)

More technically, the above Eqs. undeline the importance of the Gini correlations among the distributions in explaining Axiom violations. For what concerns Axiom 1, the contribution to its violation due to V (Eq. 25) depends on four items: first, $(r_{V|T}-r_{V|X})$, that is the difference between the Gini correlation of V and T, that is $r_{V|T}=\frac{C_{V|T}}{G_V}$, and the Gini correlation between V and X, that is $r_{V|X}=\frac{C_{V|X}}{G_V}$; second, the magnitude of $G_V$; third, the ratio between the mean value of V and the mean value of T; fourth the overall ratio (see Eq. 24) between the mean value of T and the mean value of Z. Focusing on the Gini correlations, a cograduation of V closer to T than to X determines a positive contribution. For what concerns $V-S$, that is the gross tax liability reduction due to deductions D, and C, the opposite occurs: in this situation, it is the cograduation of $V-S$ (C) closer to X than to T that determines a positive contribution to Axiom violations. A similar discussion applies to Axiom 2.

The third Axiom considers instead the Gini correlations of $X-V$, $V-S$ and C with respect to Z and X. A cograduation of distribution $X-V$ closer to Z than to X determines a positive contribution to this Axiom violation. Focusing on $V-S$ and C, since both of them are a component of the post-tax income Z, it is a cograduation of distribution $V-S$ (C) closer to Z than to X that determines a positive contribution to this Axiom violation.

If no reranking between X and T occurs, it has to be observed that $r_{V|T}=r_{V|X}$, $r_{(V-S)|T}=r_{(V-S)|X}$ and $r_{C|T}=r_{C|X}$, so that none of V, $V-S$ and C can contribute to the Axiom violation regardless of the sign of the Gini correlation. A similar discussion applies to Axiom 2 and 3 when, respectively, no reranking occurs between X and $\tilde{T}$ and between X and Z.

4.2.3 A stylized example

We again focus on the stylized example of Paragraph 3.3, and we show how the ‘ex post’ approach works, and why it can give different information with respect to the ‘step by step’ decomposition.

In Table 2 it is essential to observe that the ordering of T is different from the ordering of X; moreover, focusing on Axiom 1, we can also observe that the ordering of V is different from both the ordering of X and the ordering of T. In order to evaluate the effect of V (Eq. (25)), we first compute the Gini correlation coefficients: $r_{V|X}=0.83167$ and $r_{V|T}=0.71944$, from which we can conclude that the ordering of V is more different with respect to T than it is with respect to X. As a consequence, $r_{V|T}-r_{V|X}<0$; since $G_V=0.12339>0$ as well as $\frac{\mu _V}{\mu _Z}=\frac{90.26786}{359.26488}>0$, $AAV_1^V$ is negative ($-$ 0.00348).

From Eq. (27), we get $AAV_1^D=0.00151$ and, from Eq. (26), $AAV_1^S=-0.00197$. Focusing on Eq. (27), $r_{{(V-S)}|T}=0.64459$ and $r_{{(V-S)}|X}=0.84547$; since $-\frac{\mu _{V-S}}{\mu _z}=-\frac{17.09524}{359.26488}<0$ and $G_{V-S}=0.15773>0$ as well as $r_{{(V-S)}|T}-r_{{(V-S)}|T}<0$, it follows that $AAV_1^D>0$. Considering $AAV_1^C$, it is positive and equal to 0.00391: looking to Eq. (28), since $G_C>0$ and $-\frac{\mu _C}{\mu _Z}<0$, the sign depends on $r_{C|T}-r_{C|X}=-1-(-0.82456)=-0.17544)$.

Note that the contributions of the three tax instruments to Axiom 1 violation commented here are opposite of those observed for the ‘Ex ante’ decomposition (see Paragraph 4.1.3): $AAV_1^V<0$, whilst $AV_1^V>0$; $AAV_1^D>0$ and $AV_1^D<0$; $AAV_1^C>0$ and $AV_1^C<0$. A similar discussion holds for Axiom 2 violation.

5 Empirical analysis

5.1 Data

As input data, we use the static and non-behavioural microsimulation model developed by Pellegrino (2007) about 15 years ago and constantly updated. The model can estimate the most important taxes and contributions that characterise the Italian fiscal system. Here, we employ the microsimulation model module concerning the personal income tax updated to the 2014 fiscal year.⁸

The microsimulation model employs, as input data, those provided by the Bank of Italy (2015) in its Survey on Household Income and Wealth (BI-SHIW), published in 2015 with regard to the 2014 fiscal year. The BI-SHIW contains information on the household income and wealth of 8,156 households and 19,366 individuals. The sample is representative of the Italian population, composed of about 24.7 million households and 60.8 million individuals.

Considering individual taxpayers, the results for the gross income distribution, the distribution of all tax variables, and the overall tax revenue are close to the Department of Finance (2016) official statistics. Moreover, the inequality indexes for both taxpayers and equivalent households are also similar to those evaluated by the Department of Finance’s official microsimulation model (Di Nicola et al. 2015). The instrument employed in this study is then suitable for the type of empirical analysis we propose.

Starting from taxpayer distributions, we derive the corresponding equivalent household ones by applying the equivalent scale given by the square root of the number of components. In the following subsection, the results are presented and discussed according to these equivalent household distributions.

5.2 Results

5.2.1 Basic indexes

Table 3 shows all the basic as well as composed inequality indexes involved in our decompositions; it also presents the average values of the tax variables. The Gini coefficient for the pre-tax distribution $G_X$ is equal to 0.42089, whereas the concentration coefficient for the post-tax one $C_{Z|X}$ is 0.37035. As a consequence, the overall Reynolds–Smolensky index RS is equal to 0.05054. This is the value we would like to decompose by isolating the effect of the most important tax components.

The Gini coefficient for the post-tax distribution $G_Z$ equals 0.37097, meaning that the overall redistributive effect RE is 0.04992, which is lower than RS because of the re-ranking of net incomes in the transition from the pre- to the post-tax values (measured by Axiom 3, which is the Atkinson–Plotnick–Kakwani index $R_{Z|X}=G_Z-C_Z=AV_3=0.00062$). The concentration coefficient for net tax liabilities $C_{T|X}$ is 0.63954, and the Kakwani index $K=C_{T|X}-G_X$ is equal to 0.21865, $\frac{\mu _T}{\mu _Z}=\frac{\theta }{1-\theta }$ to 0.23113, and $\theta$ to 0.18774. As observed for net incomes, $G_T$=0.64626 is also greater than $C_{T|X}$; their difference $R_{T|X}=0.00673$ evaluates the importance of the re-ranking of the net tax liability distribution because of the tax.⁹

As shown in Sect. 4, deductions affect the transition from the V distribution to the S one, whereas tax credits influence the transition from the S distribution to the T one. We focus on the impact of the related indexes later; here, we start by noting their values. For what concerns the two gross tax liability distributions, $G_V=0.48343$, whereas $G_S=0.47901$; the corresponding concentration coefficients are $C_{V|X}=0.48245$, $C_{S|X}=0.47732$. Moreover, $G_{V-S}=0.74211$, $G_{X-V}=0.39786$, and $G_{X-S}=0.40147$, whereas $C_{(V-S)|X}=0.56811$, $C_{(X-V)|X}=0.39768$, and $C_{(X-S)|X}=0.40123$. Finally, discussing the basic indexes specifically employed for Axiom 2, $G_{\tilde{T}}=0.42087$, $C_{\tilde{T}|X}=0.39600$, $G_{\tilde{V}}=0.07271$. The Gini coefficient for tax credits is $G_C=0.22783$, whereas the corresponding concentration coefficients are lower: $C_{C|X}=0.04587$ and $C_{C|T}=0.02299$.

All the inequality decompositions we propose depend on the average values of the distributions to which they refer: average gross income $\mu _X$ is 21,615.47 euros, whereas $\mu _V=5,918.43$, $\mu _{S}=5,583.88$, $\mu _{V-S}=334.56$, $\mu _{X-V}=15,697.04$, $\mu _{X-S}=16,031.60$, $\mu _{C}=1,525.82$, $\mu _{T}=4,058.06$, $\mu _Z$ is 17,557.41, $\mu _{\tilde{V}}=0.24437$, $\mu _{\tilde{V-S}}=0.01333$, $\mu _{\tilde{C}}=0.11098$, and $\mu _{\tilde{T}}=0.12006$.

We can now start analysing the core structure of our methodologies. First, we present the ‘step by step’ analysis (Sect. 5.2.2) and then the ‘overall and simultaneous’ decompositions (Sect. 5.2.3).

Both methodologies consider $RE^P=0.05784$, whilst $AV_1=0.00155$, $AV_2=0.00575$ and $AV_3=0.00062$.

Table 3 Inequality indexes values

5.2.2 The ‘step by step’ analysis

Focusing on Axiom 1, Table 4 shows the marginal effects of V, D, S, and C in determining $AV_1$. In particular, $AV_1^V$ is equal to 0.00037, lower than $AV_1^S=0.00059$. If we compare the situation with and without deductions, it follows that D positively contributes to increase Axiom 1 violations. A similar picture emerges if tax credits C are added into the analysis: $AV_1=0.00155$ is greater than $AV_1^S=0.00059$, stemming from the positive effect of C in increasing Axiom 1 violations ($AV_1^C=0.00097$).

Table 5 shows the corresponding marginal effects of V, D, S, and C in determining $AV_2$. In particular, $AV_2^V$ is equal to 0.00221, much lower than $AV_2^S=0.00572$. This is due to the positive contribution of D in increasing $AV_2$ since $AV_2^D$ is positive and equal to 0.00351. Again, a similar picture emerges if tax credits C are considered: $AV_2=0.00575$ is only marginally greater than $AV_2^S=0.00572$, stemming from the positive but negligible effect of tax credits C in increasing Axiom violations ($AV_2^C=0.00003$). Finally, $AV_3^V$ is equal to 0.00019, lower than $AV_1^S=0.00024$; $AV_3=0.00062$ is greater than $AV_1^S$.

As a consequence, again both D and C positively contribute to the Axiom 3 violations: $AV_3^D=0.00005$ and $AV_3^C=0.00038$ (Table 6).

Table 4 Violations of Axiom 1—‘step by step’

Table 5 Violations of Axiom 2—‘step by step’

Table 6 Violations of Axiom 3—‘step by step’

5.2.3 The ‘overall and simultaneous’ or ‘ex post’ analysis

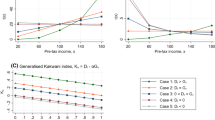
Table 7 presents the decomposition of Axiom 1, whereas Tables 8 and 9 show those of Axioms 2 and 3, respectively.

These tables contain the actual values and values as a percentage of RS as well as $AV_1$, $AV_2$, and $AV_3$, respectively. More precisely, $AV_1$ is 3.08% of RS (Table 7, second row). Deductions D and tax credits C positively contribute to the Axiom 1 violations by 0.83% of RS ($AAV_1^D$) and 3.93% of RS ($AAV_1^C$), respectively: their effects are thus detrimental to $AV_1$ and they are offset by the rate schedule ($-1.69\%$ of RS if $AAV_1^{V}$ is considered and $-0.86\%$ of RS if $AV_1^{S}$ is instead contemplated). Regarding the composition of $AV_1$ (Table 7, third row), tax credits C contribute the most to the overall violation: $AAV_1^{C}$ represents 127.90% of $AV_1$, whereas $AAV_1^{D}$ is 26.93% of $AV_1$. Together, they reach 154.83%, meaning that the rate schedule ($AAV_1^V$) overcomes these unpleasant outcomes by $-54.83\%$ of $AV_1$.

$AV_2$ is 11.37% of RS (Table 8, second row). All the elements of the tax positively contribute to Axiom 2 violations: V by 2.28% of RS ($AAV_2^V$), D by 1.29% ($AAV_2^D$), and C by 7.81% ($AAV_2^C$). Focusing on the composition of $AV_2$ (Table 8, third row), tax credits C contribute the most to the overall violation: $AAV_2^{C}$ represents 68.64% of $AV_2$, $AAV_2^{S}$ 31.35%, $AAV_2^{V}$ 20.03%, and $AAV_2^{D}$ 11.32%.

For what concerns the third Axiom, its overall violations are a low percentage (1.22%) of RS (Table 9, second row). As observed for Axiom 1, deductions D and tax credits C also positively contribute to these Axiom violations: $AAV_3^D$ is $0.22\%$ of RS and $AAV_3^C$ is $1.38\%$ of RS. On the contrary, the rate schedule counterpoises these effects: $AAV_3^V=-0.38\%$ and $AAV_3^S=-0.16\%$. This is particularly noticeable by looking at the composition of $AV_3$ (Table 9, third row): tax credits contribute $112.88\%$ of $AV_3$ to the ‘ex post’ effect, whereas deductions D account for only $18.22\%$ of $AV_3$; these percentages are reduced by the negative contributions of the tax rate structure ($-$ 31.10 and $-$ 12.88%, respectively).

Table 7 Violations of Axiom 1—‘ex post’

Table 8 Violations of Axiom 2—‘ex post’

Table 9 Violations of Axiom 3—‘ex post’

5.2.4 Comparing the two methodologies

Comparing Tables 4, 5, and 6 with Tables 7, 8, and 9 shows that the decompositions of Axiom violations are considerably different if the ‘ex ante’ or the ‘ex post’ approach is considered. Not only the contributions of components are different in the two approaches, but also their signs can change.

Focusing on Axiom 1 and Axiom 3, the ‘ex post’ analysis even shows negative signs for the rate schedule contributions V and S to the corresponding overall Axiom violations. In particular, their negative effect partially compensates for the positive effect due to deductions D and tax credits C. In addition, the magnitude of the positive contributions due to D and C differs between the two approaches; the ‘ex ante’ contributions are about half those of the ‘ex post’ ones.

On the contrary, focusing on Axiom 2, the ‘ex post’ contribution due to D is far lower (about one-fifth) than the corresponding ‘ex ante’ one. The opposite happens if tax credits C are examined: in particular, the ‘ex ante’ methodology shows that the marginal contribution of tax credits is not only low, but also the lowest among all the tax components, whereas the situation is reversed if the ‘ex post’ methodology is considered: in this case, the contribution of C is remarkably high.¹⁰

Focusing on the ‘step by step’ analysis, in the actual Italian tax system the re-ranking generated by the application of the rate schedule to distribution X is lower than the one generated by the application of the rate schedule to $X-D$; moreover, after the application of tax credits C, the re-ranking still increases. This explains the values reported in Tables 4, 5 and 6. We recall that the starting contribution to each Axiom violation is the one registered for statutory marginal tax rates (see Eqs. (8), (13) and (18)).

Having evaluated the Axiom violation that would be obtained by applying statutory marginal tax rates to the tax base $X-D$, the effect due to deductions D is evaluated by difference (see Eqs. (10), (15) and (20)). Similarly, having evaluated the Axiom violation due to the net tax liabilty T, also the effect due to tax credits C is evaluated by difference (see Eqs. (11), (16) and (21)).

For what concerns the ‘ex post’ analysis, Axiom 1 is violated since the ordering of T differs from the ordering of X. By employing Eq. (24), we can compute the contribution of the three components, that is $AAV_1^V$, $AAV_1^D$ and $AAV_1^C$. The contribution to the Axiom violation due to the statutory tax rates $AAV_1^V$ depends on two Gini correlations: the one between distribution V and distribution T, and, in relative terms and regardless of the sign of the Gini correlation, the one between distribution V and distribution X. In the present Italian system, the latter is greater than the former, so that, once deductions and tax credits have been applied, distribution V smooths the violation; conversely, being the Gini correlations both of $V-S$ and C greater with respect to X than to T, due to the negative sign, Axiom violation arises.

In case of Axiom 2 (see Eq. (29)), the contributions of the three components are positive, being also the Gini correlation of $\tilde{V}$ greater when evaluated with respect to $\tilde{T}$ than to X.

Finally, since the Gini correlation of $X-V$ with respect to Z is lower than the one with respect to X, the contribution of V moderates Axiom 3 violation; conversely, the contribution of distributions D and C is positive, because the Gini correlations of $V-S$ and of C are greater with respect to Z than with respect to X.

5.2.5 An overview of the Italian personal income tax through the new methodologies

The Italian personal income tax establishes its overall redistributive effect through the rate schedule and tax credits for taxpayers’ kind of income and dependent individuals within the household as well as tax credits and deductions for items of expenditure. From the progressivity point of view, tax credits and deductions for items of expenditures play a marginal role,¹¹ whilst the rate schedule and the tax credits for earned incomes and dependent individuals within the household represent 41% and 65% of it, respectively (Barbetta et al. 2018).

In this respect, our analysis based on Axiom violations gives details to the policy maker for implementing a tax reform. By considering equivalent incomes, the present tax strucure reveals a significant horizontal and vertical unfairness: the application of the rate schedule to nominal incomes per se produces horizontal and vertical equity problems, and the actual system of tax credits and deductions not only is not able to overcome these unpleasant outcomes, but also it makes them worse. In particular, the ‘ex post’ analysis even reveals that the contribution of the rate schedule rectifies the negative impact of deductions D and tax credits C in violation Axiom 1 and 3.

Summing up, our methodology clearly emphasises that, in order to achieve fairness, tax credits and deductions are structured in a non-harmonious way with respect to both the magnitude of the marginal tax rates and the adoption of a reasonable equivalence scale. The rate schedule is unique and it is applied to all taxpayers, whilst tax credits, that depend on the kind of work and the number of dependent individuals within the household as well as the items of expenditure, are more differentiated than it would be necessary on equity ground. These conclusions should help ignite the public and political debate on the need for tax reform. For example, a way to lighten the unpleasent outcomes due to Axiom violations would be a transition from the actual tax structure to a continuous average tax rate schedule (Longobardi et al. 2020).

6 Concluding remarks

Considering each fundamental component of a real-world personal income tax, that is statutory marginal tax rates and deductions as well as tax credits, the aim of this study is to reveal the importance of Axiom violations, as introduced by Kakwani and Lambert (1998).

To reach this goal, we propose two new decompositions compatible with this axiomatic approach. The contribution of our strategy is its mathematical simplicity, which makes it more understandable to researchers unfamiliar with these fundamental analyses of the personal income tax. More precisely, we divide the three original Axiom violations introduced by Kakwani and Lambert (1998) into three parts: the effects due to the rate schedule and deductions as well as tax credits. Our decompositions present some peculiarities not shown in previous attempts. We apply this methodology to the Italian personal income tax, showing the capillary analysis that is possible to infer using these instruments. They can be particularly useful for policymakers aiming for a tax reform to better choose the tax parameters in order to contain, as far as possible, violations of the horizontal and vertical equity principles, and, consequently, to increase its progressivity as well as to reduce the regressiveness implicitly existing in imperfect real-world taxes.

The ‘step by step’ approach immediately shows how deductions and, subsequently, tax credits, act on the statutory marginal tax rates, modifying their performance in respecting equity principles. Besides, the ‘ex post’ approach looks to Axiom violations according to a different perspective: it estimates Axiom violations due to each of the three tax intruments once the effects due to the other two instruments have been taken into account. Results by considering our ‘ex post’ approach can also be surprising. Although each tax instrument can introduce Axiom violations when it is applied, when the whole tax structure is considered the assessment of its impact can be different, not only in terms of relative incidence, but also for the sign of its effect.

Appendices

Appendix A1: Axiom 1 violations—‘step by step’

For what concerns Axiom violations, Axiom 1 can be decomposed as follows. Equation (8) becomes:

$$\begin{aligned} AV_1^{V}=\frac{\mu _V}{\mu _{X-V}}R_{V|X}=\frac{\mu _V}{\mu _{X-V}} \sum _{i=1}^I \frac{\mu _{V_i}}{\mu _V} \Big ( C_{V_i|V} - C_{V_i|X} \Big ). \end{aligned}$$

If only one deduction is considered, Eq. (10) is the following:

$$\begin{aligned} AV_1^{D}=AV_1^{S}-AV_1^{V}=\frac{\mu _S}{\mu _{X-S}} \sum _{i=1}^I \frac{\mu _{S_i}}{\mu _S} \Big ( C_{S_i|S} - C_{S_i|X} \Big ) - \frac{\mu _V}{\mu _{X-V}}R_{V|X}. \end{aligned}$$

On the contrary, when several deductions or allowances are considered, the previous Eq. can be decomposed into J Eqs., as follows:

$$\begin{aligned} \frac{\mu _S}{\mu _{X-S}} R_{S_j|X} - \frac{\mu _{S_{j-1}}}{\mu _{X-S_{j-1}}} R_{S_{(j-1)|X}}. \end{aligned}$$

Turning to tax credits, Eq. (11) becomes

$$\begin{aligned} AV_1^{C}=\frac{\mu _{T}}{\mu _Z} \sum _{h=1}^H \frac{\mu _{S-C_h}}{\mu _{S-C}} \Big ( C_{S-C_{h}|T}-C_{S-C_{h}|X} \Big ) - \frac{\mu _S}{\mu _{X-S}} R_{S|X}. \end{aligned}$$

Appendix A2: Axiom 2 violations—‘step by step’

Equation (13) becomes

$$\begin{aligned} AV_2^{V}=\frac{\mu _{V}}{\mu _{X-V}}R_{\tilde{V}|X}=\frac{\mu _{V}}{\mu _{X-V}} \sum _{i=1}^I \frac{\mu _{\tilde{V_i}}}{\mu _{\tilde{V}}} \Big ( C_{\tilde{V_i}|V} - C_{\tilde{V_i}|X} \Big ). \end{aligned}$$

If only one deduction is considered, Eq. (15) is

$$\begin{aligned} AV_2^{D}=AV_2^{S}-AV_2^{V}=\frac{\mu _S}{\mu _{X-S}}\sum _{i=1}^I \frac{\mu _{\tilde{S_i}}}{\mu _{\tilde{S}}} \Big ( C_{\tilde{S}_i|\tilde{S}} -C_{\tilde{S}_i|X} \Big )-\frac{\mu _V}{\mu _{X-V}}R_{\tilde{V}|X}. \end{aligned}$$

When several deductions or allowances are considered, the sum of J Eqs. have instead to be considered:

$$\begin{aligned} \frac{\mu _S}{\mu _{X-S}} R_{\tilde{S}_j|X} - \frac{\mu _{S_{j-1}}}{\mu _{X-S_{j-1}}} R_{\tilde{S}_{(j-1)|X}}. \end{aligned}$$

Turning to tax credits, Eq. (16) becomes

$$\begin{aligned} AV_2^{C}=\frac{\mu _{T}}{\mu _Z} \sum _{h=1}^H \frac{\mu _{\tilde{S}-\tilde{C}_h}}{\mu _{\tilde{S}-\tilde{C}}} \Big ( C_{\tilde{S}-\tilde{C}_{h}|T}-C_{\tilde{S}-\tilde{C}_{i}|X} \Big ) - \frac{\mu _S}{\mu _{X-S}} R_{\tilde{S}|X}. \end{aligned}$$

Appendix A3: Axiom 3 violations—‘step by step’

Equation (18) becomes

$$\begin{aligned} AV_3^{V}=G_{X-V}-C_{(X-V)|X}=\sum _{i=1}^I \frac{\mu _{X-V_i}}{\mu _{X-V}} \Big ( C_{(X-V_i)|(X-V)}-C_{(X-V_i)|X} \Big ) \end{aligned}$$

whilst, if only one deduction is considered, Eq. (20) becomes

$$\begin{aligned} AV_3^{D}=R_{(X-S)|X}-R_{(X-V)|X}=\sum _{i=1}^I \frac{\mu _{X-S_i}}{\mu _{X-S}} \Big ( C_{(X-S_i)|(X-S)}-C_{(X-S_i)|X} \Big ) - R_{(X-V)|X}. \end{aligned}$$

When several deductions or allowances are considered, the previous Eq. is the sum of J Eqs.:

$$\begin{aligned} R_{(X-S_j)|X}-R_{(X-(S_{j-1}))|X}. \end{aligned}$$

Turning to tax credits, Eq. (21) becomes

$$\begin{aligned} AV_3^{C}=R_{Z|X}-R_{(X-S)|X}=\sum _{h=1}^H \frac{\mu _{X-S+C_h}}{\mu _{X-S+C}} \Big ( C_{(X-S+C_h)|Z}-C_{(X-S+C_h)|X} \Big ) - R_{(X-S)|X}. \end{aligned}$$

Appendix B1: Axiom 1 violations—‘ex post’

For what concerns Axiom violations, Axiom 1 can be decomposed as follows (Eq. (25)):

$$\begin{aligned} AAV_1^{V}=\frac{\mu _V}{\mu _{Z}} \sum _{i=1}^I \frac{\mu _{V_i}}{\mu _V} \Big ( C_{V_i|T} - C_{V_i|X} \Big ). \end{aligned}$$

If only one deduction is considered, Eq. (27) becomes

$$\begin{aligned} AAV_1^{D}=-\frac{\mu _{V-S}}{\mu _{Z}} \sum _{i=1}^I \frac{\mu _{V_i-S_i}}{\mu _{V-S}} \Big ( C_{(V_i-S_i)|T} - C_{(V_i-S_i)|X} \Big ). \end{aligned}$$

On the contrary, when several deductions or allowances are considered, the previous Eq. is decomposed into the sum of J Eqs., as follows

$$\begin{aligned}{} & {} -\frac{\mu _{V-S_1}}{\mu _{Z}} \Big ( C_{(V-S_1)|T}-C_{(V-S_1)|X} \Big )\\{} & {} -\frac{\mu _{S_1-S_2}}{\mu _{Z}} \Big ( C_{(S_1-S_2)|T}-C_{(S_1-S_2)|X} \Big ) \end{aligned}$$

and so on, until the last Eq.:

$$\begin{aligned} -\frac{\mu _{S_{(J-1)}-S}}{\mu _{Z}} \Big ( C_{(S_{(J-1)}-S)|T}-C_{(S_{(J-1)}-S)|X} \Big ). \end{aligned}$$

Turning to tax credits, Eq. (28) becomes

$$\begin{aligned} AAV_1^{C}=\frac{\mu _{C}}{\mu _Z} \sum _{h=1}^H \frac{\mu _{C_h}}{\mu _{C}} \Big ( C_{C_{h}|T}-C_{C_{i}|X} \Big ). \end{aligned}$$

Appendix B2: Axiom 2 violations—‘ex post’

Equation (30) becomes

$$\begin{aligned} AAV_2^{V}=\frac{\mu _{T}}{\mu _{Z}} \frac{\mu _{\tilde{V}}}{\mu _{\tilde{T}}} \sum _{i=1}^I \frac{\mu _{\tilde{V_i}}}{\mu _{\tilde{V}}} \Big ( C_{\tilde{V_i}|\tilde{T}} - C_{\tilde{V_i}|X} \Big ). \end{aligned}$$

If only one deduction is considered, Eq. (32) is

$$\begin{aligned} AAV_2^{D}=\frac{\mu _T}{\mu _{Z}} \frac{\mu _{\tilde{V}-\tilde{S}}}{\mu _{\tilde{T}}} \Big ( C_{({\tilde{V}-\tilde{S})|\tilde{T}}} -C_{(\tilde{V}-\tilde{S})|X} \Big ). \end{aligned}$$

When several deductions or allowances are considered, the previous Eq. is decomposed into the sum of J Eqs., as follows:

$$\begin{aligned}{} & {} -\frac{\mu _T}{\mu _{Z}} \frac{\mu _{\tilde{V}-\tilde{S}_1}}{\mu _{\tilde{T}}} \Big ( C_{(\tilde{V}-\tilde{S}_1)|\tilde{T}}- C_{(\tilde{V}-\tilde{S}_1)|X} \Big ) \\{} & {} -\frac{\mu _T}{\mu _{Z}} \frac{\mu _{\tilde{S}_1-\tilde{S}_2}}{\mu _{\tilde{T}}} \Big ( C_{(\tilde{S}_1-\tilde{S}_2)|\tilde{T}}- C_{(\tilde{S}_1-\tilde{S}_2)|X} \Big ) \end{aligned}$$

until the J deduction is reached

$$\begin{aligned} -\frac{\mu _T}{\mu _{Z}} \frac{\mu _{\tilde{S}_{J-1}-\tilde{S}}}{\mu _{\tilde{T}}} \Big ( C_{(\tilde{S}_{J-1}-\tilde{S})|\tilde{T}}- C_{(\tilde{S}_{J-1}-\tilde{S})|X} \Big ). \end{aligned}$$

Turning to tax credits, Eq. (33) becomes

$$\begin{aligned} AAV_2^{C}=-\frac{\mu _{T}}{\mu _Z} \frac{\mu _{\tilde{C}}}{\mu _{\tilde{T}}} \sum _{h=1}^H \frac{\mu _{\tilde{C}_h}}{\mu _{\tilde{C}}} \Big ( C_{\tilde{C}_h|\tilde{T}}-C_{\tilde{C}_h|X} \Big ). \end{aligned}$$

Appendix B3: Axiom 3 violations—‘ex post’

Equation (37) becomes

$$\begin{aligned} AAV_3^{V}=\frac{\mu _{X-V}}{\mu _{Z}}\sum _{i=1}^I \frac{\mu _{X-V_i}}{\mu _{X-V}} \Big ( C_{(X-V_i)|Z}-C_{(X-V_i)|X} \Big ). \end{aligned}$$

When several deductions or allowances are considered, Eq. (39) has to be split into J Eqs.:

$$\begin{aligned}{} & {} \frac{\mu _{V-S_1}}{\mu _Z} \Big ( C_{(V-S_1)|Z}-C_{(V-S_1)|X} \Big ) \\{} & {} \frac{\mu _{S_1-S_2}}{\mu _Z} \Big ( C_{(S_1-S_2)|Z}-C_{(S_1-S_2)|X} \Big ) \end{aligned}$$

until the last one

$$\begin{aligned} \frac{\mu _{S_{J-1}-S}}{\mu _Z} \Big ( C_{(S_{J-1}-S)|Z}-C_{(S_{J-1}-S)|X} \Big ). \end{aligned}$$

Turning to tax credits, Eq. (40) becomes

$$\begin{aligned} AAV_3^{C}=\frac{\mu _C}{\mu _Z}\sum _{h=1}^H \frac{\mu _{C_h}}{\mu _{C}} \Big ( C_{C_h|Z}-C_{C_h|X} \Big ). \end{aligned}$$