1 Introduction

More than half a century ago, Buckingham [1, 2] proposed that measurements of the birefringence induced by a gradient of an external electric field on linearly polarized radiation passing through a sample of molecules could be used as a direct method to measure molecular quadrupole moments [3]. Today, this idea has evolved into an important technique for determining the molecular quadrupole moment, which is the leading electric multipole in nonpolar molecules and as such plays a major role in determining the structural and spectroscopic behavior of matter [4–7].

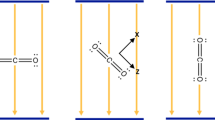
The electric-field-gradient induced (linear) birefringence, also known as the Buckingham effect or Buckingham birefringence, is measured by the optical retardation, proportional to the anisotropy n x  − n y , generated in the real part of the complex refractive index when linearly polarized radiation travels in the z direction through a fluid immersed in an electric field gradient with components in the xy plane. The phase difference is directly proportional to the optical path length and inversely proportional to the wave length of the impinging radiation. As a result of the interactions between the radiation field, the electric field gradient and the sample, the beam exiting the sample cell is elliptically polarized.

To lowest order in a perturbation expansion involving fields and molecular multipoles at constant pressure, Buckingham birefringence has two contributions. The first accounts for the thermal orientational effect of the electric field gradient on the freely rotating molecules and is inversely proportional to the temperature for a measurement carried out at constant pressure. This contribution depends on the electric quadrupole moment and the electric-dipole polarizability tensors; for polar molecules, the mixed electric-dipole–electric-quadrupole and electric-dipole–magnetic-dipole polarizabilities contribute in addition to the static molecular dipole moment [4]. The second, temperature-independent contribution to Buckingham birefringence arises from the response of the molecular electronic system to the external field and depends on higher-order mixed response properties. Although typically smaller than the temperature-dependent contribution, it is the only non-vanishing contribution to Buckingham birefringence in fluids consisting of atoms and spherically symmetric molecules.

In recent years, we have contributed to the study of Buckingham birefringence by developing and applying computational techniques for the ab initio prediction of the effect—see Refs. [8–11]. Since our first investigation of Buckingham birefringence in 1998 [12], which focused on the nonpolar molecules H2, N2, C2H2 and CH4 and led to a revised experimental quadrupole moment of N2 [13], we have extended our interest to larger and more complex systems, including polar [14, 15] as well as nonpolar [16–22] molecules, for which the effect of electron correlation and the choice of electronic-structure model have been analyzed in detail. In Ref. [23], the Buckingham birefringence of solvated molecules was investigated, thereby assessing the ability of our approach to simulate the effect of the environment. Our studies of carbon monoxide, nitrous oxide and carbonyl sulfide [14, 15] have resolved a controversy regarding the semiclassical theory of Buckingham birefringence [4, 24], supporting [25–27] the original derivation by Buckingham and Longuet-Higgins [4]. The dependence of Buckingham birefringence on the density for helium, neon and argon was analyzed in Ref. [28]. Most recently, we presented an approach to the study of Buckingham birefringence with perturbation-dependent basis sets that ensures invariance of the results to the origin of the multipole expansion [22].

An unexplored aspect of Buckingham birefringence is the influence of relativity. In this communication, we study relativistic effects on the Buckingham birefringence for the series of molecules CX2 (X = O, S, Se, Te). For CO2, CS2 and CSe2, we compare our results with previous nonrelativistic ab initio [17] data and with experiment [29–34]. The atomic-orbital-driven (AO-driven) scheme recently introduced by Bast et al. [35] for calculating time-dependent molecular properties with one-, two- and four-component relativistic methods is extended to first-order frequency-dependent magnetic perturbations with London atomic orbitals (LAOs) [36–40], thereby ensuring gauge-origin independence of the calculated results. The present work can also be considered an extension of our recent analytic implementation of Buckingham birefringence [22] to Kohn–Sham (KS) density-functional theory (DFT). Results are presented at the Hartree–Fock (HF) and KS levels of theory using nonrelativistic and four-component relativistic (reference) wave functions.

2 Theory

We here review the theory underlying the Buckingham-birefringence calculations presented in this paper. First, the important quantities of Buckingham birefringence are reviewed in Sect. 2.1. Following a discussion of our treatment of relativity in Sect. 2.2, we outline the response theory used to calculate Buckingham birefringence at the relativistic HF and KS levels of theory in Sect. 2.3.

2.1 Buckingham birefringence

For an ideal gas at constant pressure, the birefringence induced by applying an electric field gradient

$$ \nabla E =\nabla E_{XX} =-\nabla E_{YY}, \quad \nabla E_{ZZ}=0, $$
(1)

to a beam propagating in the z direction with circular frequency ω is given by the expression [3, 4, 6, 25, 41]

$$ \Updelta n = n_X - n_Y = \frac{3 \nabla E}{2 V_{\rm m}} {_{\rm m}Q}(\omega,T), $$
(2)

where V m is the molar volume and m Q(ω, T) is the Buckingham constant. For nonpolar systems, for which the molecular quadrupole moment does not depend on the choice of origin [1, 25, 41], the Buckingham constant (in the Einstein summation convention),

$$ {}_{\rm m}Q(\omega,T) = \frac{N_{\rm A}}{3 \varepsilon_0} \left[ b (\omega) + \frac{2}{15kT} \Uptheta_{\alpha\beta} \alpha_{\alpha\beta} (-\omega; \omega) \right], $$
(3)

consists of a temperature-independent term b(ω) and a temperature-dependent term bilinear in the quadrupole-moment components \(\Uptheta_{\alpha\beta}\) and in the frequency-dependent electric-dipole polarizability components, obtained from linear response theory as

$$ \alpha_{\alpha\beta}\left(-\omega;\omega\right) = -\langle\langle\hat{\mu}_\alpha;\hat{\mu}_\beta\rangle\rangle_\omega. $$
(4)

In Eq. 3 above, N A, k, and \(\varepsilon_0\) are the Avogadro, Boltzmann, and electric constants, respectively. The temperature-independent term b(ω) in Eq. 3 is given as

$$ b (\omega) = \frac{2}{15}B_{\alpha\beta,\alpha\beta} (-\omega; \omega, 0) - \frac{2}{15} {{\fancyscript{B}}}_{\alpha,\alpha\beta,\beta} (-\omega; \omega, 0) - \frac{2}{3\omega} \varepsilon_{\alpha\beta\gamma} J^{\prime}_{\alpha,\beta,\gamma} (-\omega; \omega, 0), $$
(5)

where \(\varepsilon_{\alpha\beta\gamma}\) is the Levi-Civita symbol and where we have introduced the mixed polarizabilities

$$ B_{\alpha\beta,\gamma\delta} (-\omega; \omega, 0) = B_{\alpha\beta,\gamma\delta} (\omega) = \langle\langle \hat{\mu}_\alpha; \hat{\mu}_\beta, \hat{\Uptheta}_{\gamma\delta} \rangle\rangle_{\omega, 0}, $$
(6)
$$ {{\fancyscript{B}}}_{\alpha,\beta\gamma,\delta} (-\omega; \omega, 0) = {{\fancyscript{B}}}_{\alpha,\beta\gamma,\delta} (\omega) = \langle\langle \hat{\mu}_\alpha; \hat{\Uptheta}_{\beta\gamma}, \hat{\mu}_\delta \rangle\rangle_{\omega, 0} = \langle\langle \hat{\mu}_\alpha; \hat{\mu}_\delta , \hat{\Uptheta}_{\beta\gamma} \rangle\rangle_{0, \omega}, $$
(7)
$$ J^{\prime}_{\alpha,\beta,\gamma} (-\omega; \omega, 0) = J^{\prime}_{\alpha,\beta,\gamma} (\omega) ={\rm i} \langle\langle \hat{\mu}_\alpha; \hat{m}_\beta, \hat{\mu}_\gamma \rangle\rangle_{\omega, 0}. $$
(8)

In the equations above, the electric-dipole \(\hat{\mu}_\alpha\), electric-quadrupole \(\hat{\Uptheta}_{\alpha\beta}\) and magnetic-dipole \(\hat{m}_\alpha\) operators are given by (atomic units)

$$ \hat{\mu}_\alpha = \sum_{K}Z_{K}R_{K,\alpha} - \sum_ir_{i,\alpha}, $$
(9)
$$ \begin{aligned} \hat{\Uptheta}_{\alpha\beta} & = \frac{1}{2}\sum_KZ_K \left(R_{K,\alpha} R_{K,\beta} -\frac{1}{3}\delta_{\alpha\beta} R_{K, \gamma}R_{K, \gamma} \right)\\ & \quad - \frac{1}{2}\sum_i \left(r_{i,\alpha} r_{i,\beta} -\frac{1}{3}\delta_{\alpha\beta} r_{i, \gamma}r_{i, \gamma} \right), \end{aligned} $$
(10)
$$ \hat{m}_\alpha = - \frac{c_0}{2} \sum_i ({\bf r}_i \times \varvec{\alpha})_\alpha, $$
(11)

where the summations are over nuclei K of charge Z K and positions R K and over electrons i of positions r i . In Eq. 8, c 0 is the speed of light in vacuum and \(\varvec{\alpha}\) is the four-by-four matrix

$$ \varvec{\alpha} = \left[\begin{array}{cc} 0_{2 \times 2} & \varvec{\sigma} \\ \varvec{\sigma} & 0_{2 \times 2} \\ \end{array}\right], $$
(12)

where \(\varvec{\sigma}\) is the vector of two-by-two Pauli spin matrices in the standard representation [42]. We have not included the nuclear part of the magnetic-dipole operator, which does not contribute to Buckingham birefringence in the Born–Oppenheimer approximation.

As shown by Buckingham and Longuet-Higgins [4], for polar fluids, where the quadrupole moment is origin dependent, Eq. 3 must be generalized to include a correction to the temperature-dependent part involving the molecular dipole moment and the mixed electric-dipole–magnetic-dipole and electric-dipole–electric-quadrupole polarizabilities. As we only study nonpolar molecules in this work, we do not consider this generalization here, referring the interested reader to Refs. [4, 14, 22] for more information.

2.2 Relativistic Hamiltonians and self-consistent-field wave functions

Relativistic effects are the differences between the results of a relativistic calculation with c 0 ≈ 137 a.u. at a given level of electronic-structure theory and the corresponding results obtained at the same level of theory with \(c_0 \rightarrow \infty\). They can be studied perturbatively by adding relativistic corrections to a nonrelativistic description [43, 44], or nonperturbatively, by starting from a relativistic Hamiltonian with spin–orbit coupling included variationally and selectively removing relativistic contributions [45, 46]. The latter approach is pursued in this work. Our point of reference is the four-component relativistic Hamiltonian which, in the Born–Oppenheimer approximation, may be written as a sum of one- and two-electron terms plus the classical nuclear electrostatic-repulsion term [47, 48]

$$ \hat{H} = \sum_i \hat{h}_{\rm D} (i) + \frac{1}{2} \sum_{i\neq j} \hat{g} (i, j) + V^{nn}. $$
(13)

The one-electron Dirac operator \(\hat{h}_{\rm D}\) is given by

$$ \hat{h}_{\rm D} = \left[\begin{array}{cc} V & c_0\varvec{\sigma}\cdot\varvec{\pi} \\ c_0\varvec{\sigma}\cdot\varvec{\pi} & -2c_0^2 + V \\ \end{array}\right], $$
(14)

where \(\varvec{\pi} = -{\hbox {i}} \varvec{\nabla} + e {\bf A}\) is the kinetic momentum of an electron in the vector potential A and V represents the scalar potential generated by the nuclei and the applied electromagnetic field. A closed Lorentz-invariant expression for the two-electron interaction does not exist and \(\hat{g}(i,j)\) is to zeroth order represented by the instantaneous Coulomb interaction

$$ \hat{g}^{\hbox {C}}(i,j) = \left(I_{4\times 4} \otimes I_{4\times 4}\right)r_{ij}^{-1} . $$
(15)

In the resulting Dirac–Coulomb (DC) Hamiltonian, the Gaunt interaction [49] and higher-order relativistic electron–electron corrections are neglected [50–52]. In terms of lowest-order perturbation theory, this means that the mass-velocity, Darwin and spin–orbit effects are accounted for, while the spin-other-orbit, orbit–orbit and spin–spin effects are neglected.

The many-electron wave function is a linear combination of antisymmetrized products of complex four-component spinors

$$ \psi = \left[\begin{array}{c} \psi^{{\rm L}\alpha} \\ \psi^{{\rm L}\beta} \\ \psi^{{\rm S}\alpha} \\ \psi^{{\rm S}\beta} \end{array}\right], $$
(16)

consisting of large (L) and small (S) component bispinors. Relativistic effects can now be removed by increasing the speed of light in vacuum c 0 in the DC operator. For numerical reasons, it is preferable first to perform the following nonunitary transformation of the large- and small-component bispinors

$$ \left[\begin{array}{c} \psi^{\rm L} \\ \psi^{\rm S} \\ \end{array}\right] = \left[\begin{array}{cc} I_{2 \times 2} & 0_{2 \times 2} \\ 0_{2 \times 2} & \frac{1}{c_0} I_{2 \times 2} \\ \end{array}\right] \left[\begin{array}{c} \psi^{\rm L} \\ \phi^{\rm S} \\ \end{array}\right], $$
(17)

which, in the nonrelativistic limit \((c_0 \rightarrow \infty)\), yields the following four-component nonrelativistic Hamiltonian for an electron in the external potential \(V:\)

$$ \hat{h}_{\rm LL} = \left[\begin{array}{cc} \hat{V} & \varvec{\sigma} \cdot \varvec{\pi} \\ \varvec{\sigma} \cdot \varvec{\pi} & -2 m\\ \end{array}\right], $$
(18)

proposed by Lévy-Leblond [53]. At the same time, the two-electron DC equation factorizes into four equations, one of which is equivalent to the two-electron Schrödinger equation [53–56].

The large- and small-component bispinors in Eq. 16 are typically expanded in separate AO basis sets. However, to obtain the correct nonrelativistic limit, the basis set spanning the small-component bispinors must be related to the large-component basis by the kinetic-balance condition [57]

$$ \{ \chi_\mu^{\rm S} \} \subseteq \{ (\varvec{\sigma} \cdot \varvec{\pi}) \chi_\mu^{\rm L} \}, $$
(19)

where χμ is a Cartesian or solid-harmonic Gaussian basis function. If this mapping is one-to-one, the basis set is of the restricted-kinetic-balance (RKB) type. By introducing external vector potentials A representing magnetic fields through the minimal electromagnetic coupling, this mapping is modified since \(\varvec{\pi}\) depends linearly on the vector potential. The use of RKB can therefore lead to significant errors for magnetic properties. This problem can be solved by invoking magnetic balance [58, 59] or the unrestricted-kinetic-balance (UKB) scheme. In the latter approach, the Cartesian components of the linear momentum p are treated separately, providing a more flexible basis set to ensure the correct nonrelativistic limit. The difference between the UKB and RKB treatments decreases with increasing quality of the AO basis.

2.3 Atomic-orbital-basis Kohn–Sham density-functional response theory

The framework used here to calculate the response functions contributing to Buckingham birefringence has been presented in Refs. [60, 61], which describe a self-consistent-field AO-based response theory for time- and perturbation-dependent basis sets. Being formulated in the AO basis, the approach is transparent to the explicit form of the molecular Hamiltonian and to the parametrization of the self-consistent-field wave function. We have previously utilized this feature to extend the approach to two- and four-component relativistic wave functions for the calculation of higher-order molecular properties involving one-electron operators [35]. Here, this approach is extended further to include KS exchange–correlation (XC) contributions using perturbation-dependent basis sets to first order.

We use as our reference system four-component relativistic and nonrelativistic (Lévy-Leblond) KS wave functions, respectively, obtained by solving the KS equation for the corresponding Hamiltonians, as described in the previous section. To this system, we apply a frequency-dependent electromagnetic field represented as

$$ \begin{aligned} \hat V (t) &= \left[ f \exp(\hbox{i} \omega t) + f^* \exp(-\hbox{i} \omega t) + e \right] \cdot \left( -\hat{\mu} \right) \\ &\quad + \left[ q \exp(-\hbox{i} \omega t) + q^{*} \exp(\hbox{i} \omega t) + g \right] \cdot \left( -\hat{\Uptheta} \right) \\ &\quad + \left[ -\hbox{i} b \exp(-\hbox{i} \omega t) +\hbox{i} b^* \exp(\hbox{i} \omega t) \right] \cdot \left( -\hat{m} \right). \end{aligned} $$
(20)

In this expression, f = (f x , f y , f z )T is the complex electric field, or Jones vector, defining the intensity, polarization and phase of the radiation; q is the complex electric-field-gradient tensor arising from the radiation; e, g and −ib are the static electric field, electric field gradient and magnetic field, respectively. The factor −i has been introduced to ensure real-valued derivative integrals. In our formalism, the complex magnetic field strength −ib enters the time-dependent LAOs defined as [36, 62]

$$ \xi_\mu ({\bf x}) = \exp \left\{ - \frac{\hbox{i}}{2} \left[ \left( -\hbox{i} b \exp(-\hbox{i} \omega t) +\hbox{i} b^{*} \exp(\hbox{i} \omega t) \right) \times {\bf R}_\mu \right] \cdot {\bf r} \right\} \chi_\mu ({\bf x}). $$
(21)

To obtain the working equations for the response functions, we define a quasi-energy gradient of our (non)relativistic wave function with respect to any of the perturbation strengths w in Eq. 20 as [60]

$$ Q^w = \frac{\hbox{d}Q}{\hbox{d}w} \mathop{=}\limits^{Tr_t} \frac{\partial}{\partial w} \left[E ({\bf D}) - {\bf S}^w {\bf W}\right], $$
(22)

where the notation “\(\stackrel{\mathrm{Tr}_{t}}{=}\)” indicates that we consider the trace of all matrix expressions and a time average over one period of the applied time-periodic perturbation. In this equation, we have generalized the molecular energy determined by the Hamiltonian in Eq. 13 (or Eq. 18 in the case of the nonrelativistic energy) to read

$$ E ({\bf D}) = \left( {\bf h} + {\bf V} (t) - \frac{\hbox{i}}{2} {\bf T} \right) {\bf D} + \frac{1}{2} {\bf G}^\gamma ({\bf D}) {\bf D} + E_{\rm xc} (n ({\bf D})). $$
(23)

Here h and \({\bf V}\left(t\right)\) are the one-electron AO matrices of the free-particle Dirac operator and the interaction operator in Eq. 20, respectively, whereas the AO matrix elements of the two-electron interaction operator are given as

$$ {\bf G}_{\mu\nu}^\gamma\left({\bf M}\right) = \sum_{\kappa\lambda}M_{\lambda\kappa}\left(g_{\mu\nu\kappa\lambda}-\gamma g_{\mu\lambda\kappa\nu}\right), $$
(24)

where 0 ≤ γ ≤ 1 determines the amount of exact exchange in the calculation (zero in pure KS theory, fractional in hybrid theories, and one in HF theory); for ease of notation, the superscript indicating this scaling is omitted in the following. In Eqs. 22 and 23 we have also introduced

$$ T_{\mu\nu} = \langle \dot \xi_\mu \vert \xi_\nu \rangle - \langle \xi_\mu \vert \dot \xi_\nu \rangle, $$
(25)
$$ S_{\mu\nu}^w = \frac{\hbox{d}}{\hbox{d}w} \langle \xi_\mu \vert \xi_\nu \rangle, $$
(26)
$$ {\bf W} = {\bf DFD} + \frac{\hbox{i}}{2} {\dot{\bf D}}{\bf SD} - \frac{\hbox{i}}{2} {\bf DS}\dot{\bf D}, $$
(27)

and the XC energy E xc (n (D)) calculated from the density n(D). In Eq. 27, the generalized Fock matrix F is defined as the partial derivative of the energy functional in Eq. 23 with respect to the transposed density matrix

$$ {\bf F} = \frac{\partial E ({\bf D}) }{\partial {\bf D}^{\rm T}} = {\bf h} + {\bf V} (t) - \frac{\hbox{i}}{2} {\bf T} + {\bf G} ({\bf D}) + {\bf F}_{\rm xc}, $$
(28)

where the XC contribution to the Fock matrix is given as

$$ F_{\hbox{xc},\mu\nu} = \int \!\!\hbox{d} {\bf r} \; \Upomega_{\mu\nu}({\bf r}) v_{\rm xc} ({\bf r}) = \int \!\!\hbox{d} {\bf r} \; \Upomega_{\mu\nu}({\bf r}) \frac{\delta E_{\rm xc}}{\delta n ({\bf r})}. $$
(29)

Here, the XC potential v xc(r) is the functional derivative of the XC energy with respect to the density, \(\Upomega_{\mu\nu}({\bf r})\) is the overlap distribution of two AOs, ξμ(r) and ξν(r), and integration is over all space. We have assumed the adiabatic approximation, taking the XC kernel to be time independent [63, 64].

To derive the working equations for Buckingham birefringence, we use the quasi-energy Lagrangian in Eq. 22 defined in terms of the magnetic-field perturbation b, differentiating this twice with respect to an electric-field perturbation, yielding [22]

$$ \begin{aligned} Q^{bfe}_{\omega,-\omega,0} &\stackrel{\mathrm{Tr}_{t}}{=} \left[{\bf V}^{bf}_{\omega,-\omega} + {\bf F}^{bf}_{{\rm xc};\omega,-\omega} \right] {\bf D}^e_0 + \left[ {\bf V}^{be}_{\omega,0} + {\bf F}^{be}_{{\rm xc};\omega,0} \right] {\bf D}^f_{-\omega} \\ &\quad + \left[ {\bf h}^b_{\omega} + {\bf V}^b_{\omega} - {\frac{\hbox{i}}{2}} {\bf T}^b_{\omega} + {\bf F}^b_{{\rm xc};\omega} \right] {\bf D}^{fe}_{-\omega, 0} \\ &\quad + \left[ {\bf G}^b_{\omega} ({\bf D}) + E_{{\rm xc}; \omega}^{2,b}({\bf D}) \right] {\bf D}^{fe}_{-\omega, 0}\\ &\quad + \left[ {\bf G}^b_{\omega} ({\bf D}^e_0) + E_{{\rm xc}; \omega}^{2,b}({\bf D}^e_0) \right] {\bf D}^f_{ -\omega} - {\bf S}^b_{\omega} {\bf W}^{fe}_{-\omega,0}, \end{aligned} $$
(30)
$$ Q^{b_\beta f_\alpha e_\gamma}_{\omega,-\omega,0} = J^{\prime}_{\alpha,\beta,\gamma}(\omega) =\hbox{i} \langle\langle \hat{\mu}_\alpha; \hat{m}_\beta, \hat{\mu}_\gamma \rangle\rangle_{\omega,0}. $$
(31)

We have here introduced the notation

$$ \left(E_{\mathrm{xc}}^{2,b}\right)_{\mu\nu}\left({\bf M}\right) = \sum_{\kappa\lambda}\left(E_{\mathrm{xc}}^{2,b}\right)_{\mu\nu\kappa\lambda}M_{\kappa\lambda}, $$
(32)
$$ \left(E_{\mathrm{xc};-\omega}^{2,b}\right)_{\mu\nu\kappa\lambda} = \frac{\partial^2E_{\mathrm{xc}}^{b}}{\partial D^T_{\mu\nu}\partial D^T_{\kappa\lambda}} = \int\!\!\hbox{d}{\bf r}\; \Upomega^b_{\mu\nu}\left({\bf r}\right) \left[\int\!\!\hbox{d}{\bf r}^{\prime} \Upomega_{\kappa\lambda}\left({\bf r}^{\prime}\right)\frac{\partial v_{\mathrm{xc}}\left({\bf r}\right)}{\partial n\left({\bf r}^{\prime}\right)}\right], $$
(33)

where we have used the fact that f only enters V, and only linearly. We have also exploited the fact that, when we take the trace of the resulting matrices, the following equality holds:

$$ \mathrm{Tr} \left[ {\bf G} ({\bf D}^{\rm A}) {\bf D}^{\rm B} \right] = \mathrm{Tr} \left[ {\bf G} ({\bf D}^{\rm B}) {\bf D}^{\rm A} \right]. $$
(34)

The quantity \({\bf W}^{fe}_{-\omega,0}\) in Eq. 30 is given by the expression [22]

$$ \begin{aligned} {\bf W}^{fe}_{-\omega,0} &= {\bf D}^{e}_0 {\bf F}^{f}_{-\omega} {\bf D} + {\bf D}^{e}_0 {\bf F} {\bf D}^{f}_{-\omega} + {\bf D} {\bf F}^{e}_0 {\bf D}^{f}_{-\omega} - {\frac{\omega}{2}} {\bf D}^{e}_0 {\bf S} {\bf D}^{f}_{-\omega} \\ & \quad + {\bf D} {\bf F}^{f}_{-\omega} {\bf D}^{e}_0 + {\bf D}^{f}_{-\omega} {\bf F} {\bf D}^{e}_0 + {\bf D}^{f}_{-\omega} {\bf F}^{e}_0 {\bf D} + {\textstyle \frac{\omega}{2}} {\bf D}^{f}_{-\omega} {\bf S} {\bf D}^{e}_0\\ & \quad + {\bf D}^{fe}_{-\omega,0} {\bf F} {\bf D} + {\bf D} {\bf F}^{fe}_{-\omega,0} {\bf D} + {\bf D} {\bf F} {\bf D}^{fe}_{-\omega,0} + {\frac{\omega}{2}} {\bf D}^{fe}_{-\omega,0} {\bf S} {\bf D} - {\frac{\omega}{2}} {\bf D} {\bf S} {\bf D}^{fe}_{-\omega,0} \end{aligned} $$
(35)

since the AOs do not depend on the electric-field perturbations e and f. In Eq. 35, both \({\bf D}^{f}_{-\omega}\) and \({\bf D}^{fe}_{-\omega,0}\) carry a phase factor \(\exp(\hbox{i}\omega t)\) and hence \(\hbox{i}\dot{\bf D}^{f}\!=\!-\omega{\bf D}^{f}_{-\omega}\), whereas \({\bf D}^{e}_0\) is static and hence \(\dot{\bf D}^{e} = {\bf 0}\). Although all matrices in Eq. 30 carry a time-dependent exponential phase factor, these cancel, making time averaging redundant. The integrals in \({\bf V}^{bf}_{\omega,-\omega}\) are given as [22, 65, 66]

$$ V_{\mu\nu}^{b_\beta f_\alpha} = Q_{MN} \langle \chi_\mu \vert r_\beta r_\alpha \vert \chi_\nu \rangle, $$
(36)

where r β refers to the β component of the electron position operator in the phase of the LAO, whereas r α is the α component of the position operator in the electric-dipole moment operator. The Q MN are the elements of an antisymmetric matrix containing the differences between the centers of the AOs χμ and χν:

$$ Q_{MN}=\frac{1}{2} \left[\begin{array}{ccc} 0 & -Z_{MN} & Y_{MN}\\ Z_{MN} & 0 & -X_{MN}\\ -Y_{MN} & X_{MN} & 0 \end{array} \right]. $$
(37)

If we do not employ LAOs, all contributions that involve derivatives of integrals with respect to the external magnetic field vanish in Eq. 30, yielding a simpler expression for the quadratic response function:

$$ Q^{bfe,{\rm NoLAO}}_{\omega,-\omega,0} \mathop{=}\limits^{Tr_t} \left({\bf V}^{b}_{\omega}+{\bf F}_{\mathrm{xc};\omega}^{b}\right){\bf D}^{fe}_{-\omega,0}. $$
(38)

Since the AOs do not have an explicit dependence on any of the perturbing operators in \({{\fancyscript{B}}_{\alpha,\beta\gamma,\delta}}\), this tensor is easily obtained from Eq. 38 by replacing b with q:

$$ Q^{qfe}_{\omega,-\omega,0} \mathop{=}\limits^{Tr_t} \left({\bf V}^{q}_{\omega}+{\bf F}_{\mathrm{xc};\omega}^{q}\right){\bf D}^{fe}_{-\omega,0}, $$
(39)
$$ Q^{q_{\beta\gamma}f_{\alpha}e_{\delta}}_{\omega,-\omega,0} ={\fancyscript{B}}_{\alpha,\beta\gamma,\delta}(\omega) =\langle\langle\hat{\mu}_{\alpha};\hat{\Uptheta}_{\beta\gamma},\hat{\mu}_{\delta}\rangle\rangle_{\omega,0}. $$
(40)

In the same manner, B αβ,γδ is obtained by replacing b with g, and e with \(f^*\), respectively:

$$ Q^{gff^*}_{0,-\omega,\omega} \mathop{=}\limits^{Tr_t} \left({\bf V}^{g}_0+{\bf F}_{\mathrm{xc};0}^{g}\right){\bf D}^{ff^*}_{-\omega,\omega}, $$
(41)
$$ Q^{g_{\gamma\delta}f_{\alpha}f_{\beta}^*}_{0,-\omega,\omega} =B_{\alpha\beta,\gamma\delta}(\omega) = \langle\langle\hat{\mu}_{\alpha};\hat{\mu}_{\beta},\hat{\Uptheta}_{\gamma\delta}\rangle\rangle_{\omega,0}. $$
(42)

For completeness, we note that the polarizability tensor given in Eq. 4 can be evaluated as

$$ Q^{f^* f}_{-\omega;\omega} \mathop{=}\limits^{Tr_t} {\bf V}^{f^*}_{-\omega}{\bf D}^f_{\omega}. $$
(43)

The expressions given here are almost the same as those recently presented in Ref. [22], with the exception of the additional XC contributions. However, the current equations have been derived on the basis of a four-component relativistic DC Hamiltonian. It is the generic nature of the AO-based response theory that allows us to calculate molecular properties in a manner that is transparent to the underlying reference wave function.

The evaluation of the XC functional derivatives has previously been described in the context of perturbation-independent basis sets by Sałek et al. [67] and, in the specific case of magnetic-field perturbed densities, by Krykunov et al. [68] and by Kjærgaard et al. [69]. A general strategy for the evaluation of higher-order perturbed XC energies and functionals is given in Ref. [60]. Extensions to include spin-density contributions have furthermore been described [70], also in conjunction with the use of perturbation-dependent basis sets [61].

To develop an implementation suitable for higher-order mixed-field XC response contributions, we have found it convenient to evaluate the XC terms in Eq. 30 in two steps. First, we calculate the magnetic-field derivatives n eb, ∇ n eb, and \((\nabla n \cdot \nabla n^e)^b\) of the perturbed density variables n e, ∇n e, and \((\nabla n \cdot \nabla n^e)\), where e represents an electric-field perturbation. Next, this set of magnetic-field derivatives is used as input variables to conventional (possibly LAO-unaware) response modules that form the required matrix elements without the need for additional programming. The functional derivatives needed for constructing the XC matrix elements are obtained using automatic differentiation [71].

We finally outline the procedure for determining the first- and second-order perturbed density matrices D b and D fe that appear in the equations above. The perturbed density matrix of order n with respect to a set of perturbations w can be separated into particular and homogeneous components

$$ {\bf D}^{w_n} = {\bf D}^{w_n}_{\mathrm{P}} + {\bf D}^{w_n}_{\mathrm{H}}. $$
(44)

The particular component \({\bf D}^{w_n}_{\mathrm{P}}\) can be calculated from a knowledge of the lower-order (perturbed) density matrices through the equation [60]

$$ {\bf D}^{w_n}_{\mathrm{P}}{\bf S}^\dagger{\bf D}^\dagger-\left({\bf 1}-{\bf DS}\right){\bf D}^{w_n}_{\mathrm{P}} = -\left({\bf DSD}\right)^{w_n}_{n-1}, $$
(45)

where the subscript n − 1 on the right-hand side indicates that only perturbed density matrices of order n − 1 are included in the total derivative. The particular component can thus be calculated from known quantities.

The homogeneous component of the perturbed density matrix \({\bf D}^{w_n}_{\mathrm{H}}\) in Eq. 44 is determined iteratively from the equation

$$ {\bf D}^{w_n}_{\mathrm{H}}{\bf S}^\dagger{\bf D}^\dagger-\left({\bf 1}-{\bf DS}\right){\bf D}^{w_n}_{\mathrm{H}}={\bf 0}. $$
(46)

As in our previous study of nonlinear electric responses in the relativistic domain [35], we determine the homogeneous component of the perturbed densities by solving a linear set of equations of the general form [60]

$$ \left({\bf E}^{\left[2\right]} -\omega{\bf S}^{\left[2\right]}\right){\bf X}^{w_n} = {\bf M}_{n-1}^{\left[w_1 w_2 \cdots \right]}. $$
(47)

In this equation, we have introduced the generalized electronic Hessian \({\bf E}^{\left[2\right]}\) and metric \({\bf S}^{\left[2\right]}\) matrices, as well as a response matrix \({\bf X}^{w_n}, \) which determines the unique elements of the homogeneous component of the perturbed density matrix through

$$ {\bf D}^{w_n}_{\mathrm{H}} = {\bf DSX}^{w_n} - {\bf X}^{w_n}{\bf S}^\dagger{\bf D}^\dagger. $$
(48)

In Eq. 47, \({\bf M}_{n-1}^{\left[w_1 w_2 \cdots \right]}\) is a general right-hand-side vector that involves a collection of perturbation and (lower-order) perturbed density matrices; for details, see Ref. [60].

Whereas our expression for the quasi-energy derivatives has been formulated in the AO basis, we do not solve the linear sets of equations in this basis. Instead, we transform the right-hand side of Eq. 47 to the four-component spinor basis and use the linear response solver of Saue and Jensen [72]. In this manner, we ensure that the electronic Hessian remains diagonally dominant, thereby improving convergence. The transformation to the spinor basis also allows us to utilize quaternion algebra in the solution of the linear equations in Eq. 47, as described by Saue and Jensen [72, 73]. The response vectors obtained are subsequently transformed back to the AO basis and converted into the homogeneous components of the perturbed density matrix, which is used in the calculation of the response functions that determine the Buckingham birefringence. For details, we refer to the literature describing the various aspects of the procedure [35, 60, 72].

3 Computational details

All Buckingham-birefringence results have been obtained using a development version of the DIRAC program package [74]. The relativistic calculations have been carried out employing the four-component DC Hamiltonian; for the nonrelativistic reference values, we have used the Lévy-Leblond Hamiltonian [53].

In addition to the HF method, we have employed the KS method with the LDA (SVWN5) [75, 76], BLYP [77–79], B3LYP [80, 81], PBE [82], and PBE0 [83] XC functionals. These are nonrelativistic functionals which, with the DC Hamiltonian, have been evaluated using relativistic densities and density gradients. We have employed the full derivatives of the functionals provided by the XCFun library [71, 84]. Spin-density contributions [70] to XC matrix elements have been ignored.

Experimental equilibrium geometries from the compilation in Ref. [85] were used for CO2 (R CO = 2.19169a 0) and CS2 (R CS = 2.93391a 0). For CSe2, we have used the experimental bond length of R CSe = 3.19993a 0 reported in Ref. [86]. For CTe2, we have calculated the bond length of R CTe = 3.60056a 0 using the Gaussian 09 package [87] with the Ahlrichs def2-TZVPP basis [88] for C and Te in combination with the Stuttgart/Dresden 28-electron effective core pseudopotential [89] and the B3LYP XC functional.

We have used the u-aug-cc-pVDZ and u-aug-cc-pVTZ (“u-” meaning uncontracted) basis sets of Dunning [90, 91] for C, O and S, and the augmented all-electron u-DZ and u-TZ basis sets of Dyall [92, 93] for Se and Te. The small-component basis for the DC calculations has been generated using UKB, with RKB imposed in the canonical orthonormalization step [45]. In the self-consistent-field and response calculations, the small-component two-electron Coulomb integrals (SS|SS) have been approximated using a point-charge model [94]. A Gaussian charge distribution has been chosen as the nuclear model in the relativistic and nonrelativistic calculations, using the recommended values in Ref. [95].

4 Results

4.1 The temperature-independent part of the Buckingham birefringence

We have collected our results for b(ω), the temperature-independent contribution to Buckingham birefringence, for the series CX2 (X = O, S, Se, Te) using the DC Hamiltonian for a variety of different XC functionals and basis sets in Table 1. To assess the effect of field dependence in the basis functions, we report results using conventional as well as London AOs. In this table, the coupled-cluster results for CO2 and CS2 in the large field-independent d-aug-cc-pVQZ basis [17] are listed, together with the available experimentally derived data, taken from Refs. [29, 30] for CO2 and from Ref. [30] for CS2. In Ref. [34], the authors performed a single-temperature (T = 298 K) measurement of the Buckingham birefringence of CSe2, obtaining an estimate for the infinite-dilution Buckingham constant m Q by assuming b(ω) to be negligible, vide infra.

Table 1 The value of b(ω) calculated using the DC Hamiltonian (atomic units)
Full size table

The first thing to note from Table 1 is the importance of introducing field dependence in the AOs—in particular, in the smallest u-aug-DZ basis. For CO2 and CS2, the LAO field dependence induces changes as large as 25–30%, largely independent of the choice of XC functional. Going down in the CX2 series, the effect of field dependence decreases, being only 10–15% for CTe2 in the u-aug-DZ basis. In the larger u-aug-TZ basis, the effect of LAOs is smaller, being on average 5–10% for the entire series, the largest effect being once again observed for the lightest members of the series. The changes observed in the LAO results when the basis is increased from u-aug-DZ to u-aug-TZ are small, about 5% for CO2 and less than 1–2% for CS2, CSe2 and CTe2. The observed importance of using LAOs for rapid basis-set convergence corroborates the findings of our recent nonrelativistic study [22]. Please note that the b(ω) term for the studied series CX2 (X = O, S, Se, Te) is gauge-origin independent by symmetry.

Given that the relativistic correction to the temperature-independent part of Buckingham birefringence is negligible for CO2 (vide infra), we can compare our CO2 results directly with the high-level coupled-cluster singles-and-doubles (CCSD) results of Ref. [17]. As this study employed the large d-aug-cc-pVQZ basis, these CCSD results are expected to be reasonably close to the basis-set limit. Prior to this work, there have been three Buckingham-birefringence studies using KS theory, but only with field-independent basis sets [18–20]. Having established the importance of LAOs in Ref. [22] and in Table 1, the qualities of different XC functionals can now be more reliably assessed.

As seen from Table 1, the effect of electron correlation on b(ω) is significant, with changes from HF theory to CCSD theory of about 13% for CO2 and 15% for CS2. Interestingly, whereas electron correlation increases the magnitude of the temperature-independent contribution to the Buckingham birefringence of CO2, the opposite happens for CS2. Moreover, the CO2 and CS2 results obtained with different XC functionals do not lead to any clear conclusions regarding their ability to capture the effect of electron correlation on b(ω). For CO2, all functionals overestimate the effect of correlation; the hybrid functionals B3LYP and PBE0 perform best, the PBE0 value being very close to the CCSD value. For CS2, all XC functionals perform poorly, typically recovering less than one third of the total correlation effect as calculated using CCSD theory, the PBE0 functional again providing the best KS result.

Because of the very large experimental error bars, comparison with experimental results does not provide a stringent test on the different computational methods—all calculated values fall comfortably within three standard deviations from the center of the experimental distribution for CO2 and CS2. As discussed for linear birefringences elsewhere [8–11], this difficulty arises from the extreme sensitivity of the infinite-temperature extrapolation performed on the experimental data to estimate b(ω). From comparison with experimentally derived data, it is therefore not possible to draw definite conclusions regarding the reliability of the various XC functionals for the mixed hyperpolarizabilities that determine the temperature-independent contribution to Buckingham birefringence. Note, however, that the b(ω) contribution for CSe2 is computed to be of the order of –700 a.u. We shall later return to the consequences that this computed value has for the estimate of the quadrupole moment of CSe2 made by Brereton and co-workers in Ref. [34].

In Table 2, we have collected the individual temperature-independent contributions to the Buckingham birefringence (see Eq. 3) calculated in the u-aug-TZ basis with LAOs, using both the relativistic DC Hamiltonian and the nonrelativistic Lévy-Leblond Hamiltonian. The dominant (negative) temperature-independent contribution to Buckingham birefringence is the electric-dipole–electric-dipole–magnetic-dipole hyperpolarizability \(J^{\prime}_{\alpha,\beta,\gamma}\) term, which according to Eq. 5 enters b(ω) multiplied by −2/3ω. The B αβ,αβ and \({\fancyscript{B}_{\alpha,\alpha\beta,\beta}}\) terms are much larger but nearly cancel, entering b(ω) as \({(2/15)(B_{\alpha\beta,\alpha\beta} - \fancyscript{B}_{\alpha,\alpha\beta,\beta})}\) and typically contributing about 10% to b(ω).

Table 2 The temperature-independent contribution to Buckingham-birefringence, b(ω), and its individual components (isotropic averages, Einstein implicit summation implied) calculated using the u-aug-cc-pVTZ LAO basis and the DC Hamiltonian
Full size table

As expected, the relativistic correction to b(ω) is dominated by the correction to \(J^{\prime}_{\alpha,\beta,\gamma}. \) The correction is fairly small, however, even for CSe2, thus leaving the resulting temperature-independent Buckingham birefringence virtually unaffected by relativity for the three lightest members of the CX2 series. Further studies are needed to establish whether this is a general feature of b(ω), valid also for polar systems, for example, or whether this insensitivity to relativity is unique to the CX2 series. The relativistic corrections to B αβ,αβ and \({\fancyscript{B}_{\alpha,\alpha\beta,\beta}}\) are small in relative terms, being only about 2% for CSe2. By cancellation, the total relativistic correction from \({B_{\alpha\beta,\alpha\beta}-\fancyscript{B}_{\alpha,\alpha\beta,\beta}}\) is even smaller, being less than one percent for all XC functionals.

The relativistic effects vary significantly with the choice of XC functional—in particular, for the heavier elements. It is noteworthy that the use of exact exchange (in HF and hybrid theories) gives a negative relativistic correction for CTe2 at λ = 632.8 nm, whereas pure KS theory gives a positive and much larger relativistic correction. For CTe2, we note from Table 2 that the relativistic correction becomes substantial for b(ω) at λ = 632.8 nm, amounting to 30% for the BLYP functional.

The reason for the much larger relativistic corrections in CTe2 is the presence of a low-lying \(^3\Upsigma_u^+\) state (scalar relativistic notation), rather close in energy to the frequency of the applied field. Whereas the transition to this state is dipole forbidden in the nonrelativistic case, it is allowed in the four-component relativistic case due to spin–orbit coupling. The four-component relativistic calculations are thus much more dependent on the predicted excitation energy for this state than are the nonrelativistic calculations, as the approaching electronic resonance may affect the different XC functionals differently depending on how close the energy of the relevant \(^3\Upsigma_u^+\) state is to the applied laser frequency.

At λ = 694.3 nm, the relativistic correction in CTe2 is positive for all employed XC functionals, again with the hybrid functionals standing out and yielding very similar relativistic corrections.

4.2 The temperature-dependent contribution to the Buckingham birefringence

In Tables 3 and 4, we have collected the values for the tensors that contribute to the temperature-dependent part of Buckingham birefringence in CO2 to CSe2 and CTe2, respectively, calculated in the u-aug-TZ basis. The contributing tensors are the polarizability (both the isotropic and anisotropic components) and the quadrupole moment along the molecular axis (which is the only unique component of the quadrupole-moment tensor for the linear molecules studied here). The relativistic corrections follow the trend observed for the temperature-independent part but their magnitude is larger, amounting to 5–7% of the nonrelativistic value for the polarizability anisotropy and quadrupole moment of CSe2.

Table 3 Results for \(\Updelta \alpha(-\omega;\omega), \alpha_{\rm ave}(-\omega;\omega), \Uptheta, b(\omega)\) and the temperature-dependent contribution to the Buckingham birefringence, F(ω) / T, for CO2,CS2, and CSe2, with \(F(\omega)=\frac{2\Uptheta \Updelta \alpha(-\omega;\omega)}{15kT}, T = 298.15\,\hbox{K}\), and using the DC Hamiltonian
Full size table
Table 4 Results for \(\Updelta \alpha(-\omega;\omega), \alpha_{\rm ave}(-\omega;\omega), \Uptheta, b(\omega)\) and the temperature-dependent contribution to the Buckingham birefringence, F(ω)/T, for CTe2, with \(F(\omega)=\frac{2\Uptheta \Updelta \alpha(-\omega;\omega)}{15kT}, T = 298.15\,{\rm K}\), and using the DC Hamiltonian
Full size table

Interestingly, the relativistic correction to the polarizability of CTe2 is largest in HF theory (Table 4) and positive for both frequencies, whereas the relativistic corrections to the polarizability are negligible for all studied XC functionals and negative except for the hybrid PBE0 and B3LYP functionals at λ = 632.8 nm.

By contrast, the relativistic corrections to the quadrupole moment are substantial, indicating that relativity leads to a significant restructuring of the electron density, increasing the quadrupole moment by 10–15%. Indeed, spin–free calculations confirm that the relativistic increase in the quadrupole moment is almost entirely a scalar relativistic effect. To understand this effect we have compared nonrelativistic and scalar relativistic orbital contributions to the quadrupole moment (data not shown). This analysis shows that the change in the quadrupole moment when including scalar relativity is due to a relativistic contraction of the valence σ orbitals related to a contraction of the participating s- and p-orbitals. This decreases the electronic contribution to the quadrupole moment and increases the total (electronic + nuclear) quadrupole moment. Clearly, despite being a property that largely probes the outer part of the electron density, the quadrupole moment is strongly dependent on a proper relativistic treatment.

Whereas the isotropic polarizability is fairly insensitive to electron correlation, with the notable exception of CTe2, electron correlation is moderately important for the polarizability anisotropy and quadrupole moment of the lighter members of the series (CO2, CS2 and CSe2), contributing 3–5%. Also in this case, CTe2 displays much larger dependence on correlation at λ = 632.8 nm (by almost 15% with the BLYP functional), possibly because of the low-lying \(^3\Upsigma_u^+\) state. Interestingly, the relativistic corrections show only a weak dependence on electron correlation. We also note that the polarizability anisotropy exhibits large correlation effects at λ = 632.8 nm, more than 25% at the relativistic four-component level of theory for the LDA functional.

In Table 3, we report the available experimental reference data for most of the observables involved in Buckingham birefringence of the series of studied molecules. Whereas the electric-dipole polarizability anisotropy of CO2 is reasonably well reproduced (albeit all XC functionals yield values below the center of the experimental distribution, with the PBE, LDA and BLYP functionals performing better than the hybrid PBE0 and B3LYP functionals), the disagreement with experiment is notable for CS2 (where we underestimate \(\Updelta \alpha\)) and CSe2 (where we overestimate \(\Updelta \alpha\)). For CO2 and CS2, our DFT results lie on the opposite side of experiment relative to the highly accurate ab initio values of Coriani and co-workers in Ref. [17]. Note also the neglect of vibrational corrections, whose magnitude for the heavier members of the series may heavily affect the comparison.

With regard to the quadrupole moment, comparisons can again be made with experiment, with the CCSD(T) results obtained by Benkova and Sadlej [97] employing the HyPolX and HyPolX_dk basis sets and including scalar relativistic effects within the two–component Douglas–Kroll approximation, and, for CO2 and CS2, with the results of Ref. [17]. Among the XC functionals, the BLYP and PBE0 functionals get closest to the nicely agreeing experimental and ab initio values (both aiming at a value of 3.18 a.u.) for the quadrupole moment of CO2. Again, the reader should consult Ref. [17] for further details—for instance, on the effect of molecular vibrations.

There are several experimental values for the quadrupole moment of CS2. Both ab initio and this relativistic DFT study are closer to the values in the range of 2.6–2.7 a.u. in Refs. [29, 30]. The experimental value for CSe2 was obtained by Brereton and co-workers [34] by infinite-dilution extrapolation of data at 298 K for Buckingham birefringence observed in carbon tetrachloride, with a laser source at λ = 632.8 nm. In deriving the value of \(\Uptheta = 3.43 \pm 0.60\;\hbox{a.u.}\), the authors assumed a negligible b(ω) term, which we compute instead at a value of about −700 a.u. When, as done in other cases [8–11], this value is inserted in the expression for m Q(ω, T) and the quadrupole moment is re-evaluated, assuming for the electric-dipole polarizability anisotropy and the reaction-field-correction factor the same values as in Ref. [34], the experimental estimate of \(\Uptheta\) for CSe2 is revised to 3.47 ± 0.60 a.u., with a small but not negligible shift towards the calculated results.

4.3 Assessment of the calculated Buckingham birefringences

The last four columns of Tables 3 and 4 allow us to comment on the magnitude of the Buckingham birefringence in the CX2 (X = O, S, Se, Te) series. First, we observe that the temperature-independent contribution to m Q(ω, T) in CO2 never exceeds 1% of the temperature-dependent contribution in column F(ω)/T of Tables 3 and 4. This percentage rises to 2% for CSe2 and CTe2 and to 3% for CS2. Still, the neglect of the temperature-independent contribution to the Buckingham birefringence in the CX2 (X = O, S, Se, Te) series is a good approximation—for example, we have seen that, when b(ω) is taken into account in the derivation of the quadrupole moment of CSe2, the quadrupole moment changes by only 1.2%.

Because of the large experimental error bars, our calculated value for the Buckingham constant m Q(ω, T) at 632.8 nm and 298.15 K is in good agreement with the experimental value (at 298 K) for CSe2, in spite of our overestimation of the quadrupole moment \(\Uptheta\) and the electric-dipole polarizability anisotropy \(\Updelta \alpha (-\omega;\omega)\).

The discrepancies between theory and experiment for these quantities are also responsible for our underestimation of the magnitude of m Q(ω, T) for CO2 and CS2, the HF values being closer to experiment than the DFT values. A reasonable value for the static electric field gradient in measurements of Buckingham birefringence is ∇E ≈ 1 × 109 Vm−2. With this value, we predict for the higher member of our series an anisotropy \(\Updelta n \approx 3\times 10^{-13}\), which, for an optical path length of 1 m, yields a retardation of 3 × 10−6 rad at 632.8 nm, well above the current limits of detection.

5 Concluding remarks

We have presented the first four-component relativistic study of Buckingham birefringence, using LAOs to ensure gauge-origin independence of the calculated results and, more importantly for the small systems considered here, to improve basis-set convergence. With the use of LAOs, the results obtained at the u-aug-DZ level of theory are within 5% of the estimated basis-set limit for the Buckingham birefringence of the molecules studied in this work. Electron correlation has been described using KS theory.

We have investigated the importance of relativity and electron correlation for the description of the Buckingham birefringence of the four nonpolar molecules CO2, CS2, CSe2, and CTe2. Electron correlation is significant, leading to changes of 10–15% relative to the DC HF results. However, the ability of the standard XC functionals LDA, BLYP, B3LYP, PBE and PBE0 investigated here to recover the correlation contribution to Buckingham birefringence is doubtful, noting that the agreement with earlier CCSD values is good for CO2 but poor for CS2, for which only one third of the electron-correlation effects are recovered. Further studies on the adequacy of modern XC functionals in describing electron-correlation contributions to Buckingham birefringence appear necessary.

In contrast to electron correlation, the effects of relativity on the temperature-independent contribution to Buckingham birefringence are negligible. The only exception to this observation in the series is CTe2, where the relativistic corrections amount to 20–30%. The importance of relativity for this molecule is due to low-lying, resonant states becoming dipole allowed when spin-orbit interactions are included in the calculations.

Relativistic effects have been found to be more important for the temperature-dependent contribution to Buckingham birefringence, where the quadrupole moments display fairly large relativistic corrections considering that the property probes the outer regions of the electron density. These relativistic corrections are almost exclusively scalar in nature, and are found to be due to the relativistic contraction of σ valence orbitals. It would therefore be of interest to also investigate relativistic effects on polar molecules, as additional contributions to the temperature-dependent part enters the expression for the Buckingham birefringence in this case.