Estate division: equal sharing, exchange motives, and Cinderella effects

Abstract

This study contributes to the empirical literature testing bequest motives by using a population-wide administrative dataset, covering data on inherited amounts for complete families matched with an extensive set of economic and demographic variables, to estimate the influence of child characteristics on differences in inherited amounts among siblings. Our main findings are, first, children who are more likely to have provided services to the parent receive more than their siblings, as predicted by the exchange model. Second, daughters with children receive more than sons with children. This is consistent with the prediction of the evolutionary model that larger investments should go to offspring who are certain to be genetically related. There are also Cinderella effects—that is, adopted stepchildren receive less than siblings who are biological or children who are adopted by both parents. Third, we do not find support for the prediction of the altruism model that bequests are compensatory.

1 Introduction

This paper is about the determinants of parents’ decisions regarding the allocation of bequests between their children. The objective is to test the relevance of both conventional and more unconventional explanations for parents’ bequest decisions. We do this by studying the determinants of differences in inherited amounts among siblings.

We use a population-wide dataset from Sweden covering data on bequests and inheritances for complete families (deceased parents and all their children) during the 2002–2004 period, matched with an extensive set of individual economic and demographic variables from other administrative registers. By exploiting the within-family variation in the data, we estimate the influence of child characteristics on inherited amounts using models with family-fixed effects that effectively account for unobserved heterogeneity in preferences across families.

The questions we analyze include:

Do children who are worse off financially than their siblings receive larger bequests? This is the hypothesis of the altruism model of bequests, which assumes that parents use bequests to equalize consumption possibilities within the family (Barro 1974; Becker 1974). In macroeconomics, for example, the Ricardian equivalence predictions about fiscal policy inefficiency are based on the assumption of dynastic altruistic behavior.

Do children who have provided more services to the parent inherit larger amounts than their siblings do? This is the hypothesis of the exchange model of bequests (Bernheim et al. 1985; Cox 1987). To the extent that services refer to informal care of the parent, unequal sharing on the basis of quid pro quo will work as a private insurance, compensating for the income losses from caregiving.

Do children who continue the family bloodline receive more than their siblings who do not? To the extent that this form of evolutionary motive (Cox 2003; Hamilton et al. 2007) is important, it would manifest itself in larger bequests to genetic children than to non-biological children. Moreover, children who produce offspring (grandchildren of the deceased) should receive more than the siblings who do not produce offspring, and especially daughters since their offspring are more certain to be genetic descendants.

The above explanations are all based on the idea that parents (with more than one child) make unequal bequests. But, as the vast majority of parents divide, or intend to divide, their estates equally between their children, these explanations are commonly rejected in the literature (Arrondel and Masson 2006). It should be noted already at this point that equal sharing is the default rule in the Swedish inheritance law. This is similar to the inheritance laws in most other European countries as well as in the USA (Angelini 2007; Pestieau 2003). It is apparent that equal sharing also is the common practice. In our data, 86% of the parents who pass away with a positive estate, more than one child and a will (which is needed to divide unequally) divide their estates equally among their children, even though they had the option to choose a different distribution.¹

It is important to learn about the degree at which bequests are typically divided unequally for an analysis of the evolution of wealth distribution.² But this is only a first step. It is, on a more general level, crucial to understand what determines the allocation decision in order to assess the normative implications of wealth inequality and consider potential policy interventions (Cremer and Pestieau 2006).

We begin our analysis by studying the factors influencing the parent’s decision to divide the bequest unequally. Our results show that the propensity to divide unequally does not appear to be random but rather that it is associated with within-family differences in child attributes and behaviors, as predicted by the transfers theories. We find, for instance, that a higher dispersion in economic resources (income and wealth) among the children increases the likelihood of unequal sharing, as predicted by the altruism model. Moreover, parents are more likely to divide unequally if they have a mix of children living and not living close to them, which could be seen as support for the exchange model. And, our finding that parents with a mix of biological and adopted children increases it the likelihood of unequal sharing is consistent with the evolutionary model.

One issue with the above findings is that they only provide indirect support for the transfer theories. For example, the finding that a greater income dispersion among the children is positively associated with unequal division is only consistent with the altruism model if the less well-off children receive a larger inheritance than their more affluent siblings. Similarly, the finding that a mix of biological and adopted children increases the likelihood of unequal sharing is only consistent with the evolutionary model if the biological children (who can carry on the family genes) receive disproportionally more.

To provide more direct tests of the transfer theories, we exploit the uniqueness of our data, that is the information on inherited amounts for complete families, and estimate how differences in inherited amounts across siblings are related to differences in their characteristics and behaviors. As far as we know, we are the first to use population-wide administrative data covering precise information on realized inherited amounts for complete families to apply this empirical strategy to test several bequest theories.

The identifying variation in these estimations comes from families with unequally divided bequests. This is unlike most previous studies that use the incidence of transfers as outcome, and thereby rely solely on variation induced by the small and particular subset of families in which the parent has disinherited at least one child (e.g., Dunn and Phillips 1997; McGarry 1999).

The results from our analysis are the following:

We find no evidence that the inherited amount is correlated with the child’s permanent income. This finding is inconsistent with the altruism model of bequest.³ To our knowledge, only Wilhelm (1996) provides tests of the compensatory nature of bequests using a similar approach. Based on estate tax return data on wealthy parents in the USA, he, similarly to us, finds no evidence of compensatory division of bequests within families. We also take Wilhelm’s work further and test for whether bequests are compensatory with respect to wealth and education but the relationships with the inherited amount are also in these cases statistically insignificant. Another improvement in relation to Wilhelm is that we show that these findings remain also when controlling for an extensive set of other children characteristics and behaviors that parents may use as a basis for discrimination.

We find that children who are more likely to have provided services and attention to the parent (e.g., because they lived close to the parent) benefit disproportionately from bequests. This is consistent with the predictions of the exchange model. There are no previous studies using our approach to test the predictions of the exchange model with respect to bequests.⁴ In their seminal paper, Bernheim et al. (1985) find that parents’ bequeathable wealth has a significant positive effect on attention (measured as number of visits, or phone calls) supplied by the children. Light and McGarry (2004) report that among mothers who plan to leave unequal bequests, one fourth intends to exchange bequests for services provided by the children. Finally, Brown (2006) finds that children who provide informal care to the parent, as compared to those who do not, are more likely to be included in the set of potential bequest recipients.

In order to investigate the relevance of the evolutionary model, we test for differences in inherited amounts between biological and adopted children within the same families. This strategy, as opposed to comparing transfers to biological children and non-adopted, non-biological children (e.g., stepchildren or foster children), is advantageous as it minimizes the influence of unobservable confounding factors, such as preferences, upbringing, etc.⁵ It also limits the possibility that smaller transfers to non-biological children are the result of the parent expecting the child’s biological parents to provide for him or her. Our results show that, among families with both biological and adopted children, adopted children receive less than half of what the siblings who are the parent’s biological children do.

A closer look at the relationship, however, indicates that it is largely driven by disfavored adopted stepchildren of the deceased. Adopted children with two adoptive parents, on the other hand, do not receive less than siblings who are the biological children of the deceased. The finding that stepparents invest less in their (step) children than biological parents do is the reason for why we use the term Cinderella effect.⁶ The crucial factor leading to a lower bequest for an adopted child, in other words, is whether the previously deceased parent was the child’s biological parent.

Our finding agrees with previous studies that report that mothers with both biological and non-biological children (adopted or step) are more likely to plan unequal bequests (Light and McGarry 2004), and that stepchildren are less likely to be included in the stepparent’s will before the death (Francesconi et al. 2015).

Moreover, we find that daughters with children of their own receive more than sons with children. This is also in line with the prediction of the evolutionary model that larger investments should be directed to offspring who are certain to be genetically related.

The paper is structured as follows: In Section 2, we discuss the hypotheses and some empirical issues. Section 3 presents the data and the construction of the analysis sample. In Section 4, we report the results from an analysis of the determinants of unequal division as well as from the main analysis; that is, the determinants of differences in inherited amounts among siblings. Finally, in Section 5, we conclude. Two appendices provide additional descriptive statistics and estimation results.

2 Hypotheses and empirical issues

2.1 Transfer motives

Different motives for intentional transfers from parents to children have been proposed in the theoretical literature. We will here discuss altruism, exchange, and evolutionary motives.

The altruism model of bequests is based on the idea that the parent obtains utility from own consumption as well as from each of her children’s utility levels (which depend on their lifetime consumption possibilities) (Barro 1974; Becker 1974). This implies that the higher the lifetime resources of the parent, the larger the transfer to all children. Another key prediction of the model is that bequeathed amounts from the parent are negatively correlated with child income. This is because the marginal utility of a transfer depends on the child’s lifetime income. For parents with more than one child, this, so-called derivative condition, implies that the parent will make larger transfers to children with low income relative to the siblings (Cox 1987). The compensating transfers will reduce the difference in lifetime consumption possibilities between low- and high-income siblings.

We test for the relevance of the altruism model by estimating the impact of child income on the inherited amount. The hypothesis is that children with lower incomes, relative to their siblings, should receive disproportionally larger inheritances. As noted above, the predictions regarding the connection between inheritance and income are based on permanent income. We will use the average of taxable labor income over the three years preceding the parent’s demise as a proxy for the child’s permanent income. We do not include the child’s income in the year when the parent passes away, as it is unclear whether this is observable to all parents. One concern is that the three-year average of income of persons who are, on average, in their early 50s may be a poor proxy for permanent income.⁷ As alternative measures of lifetime consumption possibilities, we use the child’s wealth (average of net wealth over the three years preceding the demise) and level of education.

The exchange model assumes that the parent values services provided by the children, and more so than similar services provided in the market (Bernheim et al. 1985; Cox 1987). Services may be attention paid to the parent, care, or assistance. The parent is assumed to pay for the services with transfers. Parents with higher resources will purchase more services and, consequently, make more and larger transfers. The price that the parent has to pay depends on the value of the child’s time (i.e., the child’s opportunity cost). This leads to the prediction that the parent is more likely to purchase services from children for whom the cost of time is low.⁸ Transaction costs—in the form of travel or travel time costs—will also affect the purchase of services. Children, for whom these transaction costs are relatively low because they, for example, live closer to the parent, are more likely to be service providers and, consequently, more likely to be rewarded with larger transfers (see Hochguertel and Ohlsson 2009, for a discussion).⁹

The prediction of the exchange model, that children who have provided relatively more services to the parent receive larger inheritances, is tested by comparing differences in inherited amounts between children who lived close to the parent prior to the demise and children who lived further away. The argument here is that services are more easily delivered when parents and children live geographically close (Cox and Rank 1992; Hochguertel and Ohlsson 2009). As a measure of geographic proximity, we use an indicator for whether the child and the parent resided in the same parish during the three years before the demise. The parish is the most disaggregated geographic identifier available in Swedish registers and ascertains that parents and children live no more than 20 km from each other.¹⁰ Another proxy for service provision that we consider is the sex of the child. Studies consistently report that daughters are disproportionally more involved in the provision of parental care than sons (Coward and Dwyer 1990; Stoller et al. 1992), due to the lower opportunity cost of their time (see, e.g., Ettner 1996).¹¹ Finding that daughters receive more than sons could, therefore, be explained by daughters being compensated for their relative more extensive service provision (Cox 1987). Moreover, it is possible that care giving and attention are correlated with the child’s marital status since single children are likely to have a lower opportunity cost of time than married or partnered children (Brown 2006). Finding that married children receive less than their unmarried siblings could, thus, be seen as support for the exchange model.

Another proxy for provision of services and attention that we consider is the child’s relative birth order. According to the model in Konrad et al. (2002), older children exploit their first mover advantage by moving away from their parents, inducing their younger siblings to locate closer to the parent and thus, bear a disproportionately larger share of long-term care responsibilities. There are also studies in sociology and psychology showing that parents have a closer adult relationship with their later-born children, and in particular the last-born child (e.g., Whiteman et al. 2003; Suitor and Pillemer 2007), suggesting that later-born children are more likely to provide attention to their parents than are earlier-born children.¹² Finding that later-born siblings, and in particular the youngest child, receive more than their earlier-born siblings could, thus, be seen as support for the exchange model.

A more recent theory of parental transfers is based on reproductive biology and evolutionary psychology, and argues that transfers arise from an inherent desire of the parent to support the survival of his or her genes (Cox 2003; Hamilton et al. 2007). Accordingly, parents will leave more and larger transfers to their biological children, who can pass on the genes, than to their non-biological children (i.e., adopted children or stepchildren). We study the relevance of this prediction by testing for differences in inherited amounts between biological and adopted siblings. In Sweden, adopted children enjoy the same legal status in the bequest division as biological children. Finding that adopted children receive smaller inheritances than biological children would thus imply that parents act in accordance with the evolutionary model.

The evolutionary model further suggests that parents care about the long-term continuation of the family blood line and will thus favor children who produce descendants (i.e., grandchildren). This prediction, however, is somewhat less straightforward than the previous one since, on the one hand, parents may give larger amounts to children who have already produced children, and, on the other hand, parents give relatively large amounts to childless children to assist with the eventual cost of raising a child or simply to “motivate” them to produce grandchildren (Cox and Stark 2005). To get closer to the theoretical prediction, we therefore extend the analyses to not only test for the impact of having children per se but also for the interaction effect of having children and being a woman. This follows from the reasoning and empirical observation in Cox (2003) that grandchildren by daughters are preferred over grandchildren by sons, as they are more certain to be genetic descendants.

2.2 Empirical issues

A joint prediction of the transfer theories discussed above is that parents with more than one child will divide the bequest unequally between the children, if the children differ in characteristics and behaviors. Studying how child-level variables affect the parent’s decision to divide equally or unequally would, however, only inform us about how the distribution of traits among the children correlates with the parent’s allocation decision, and not on what grounds the parent favor or disfavor particular children. Finding, for instance, that a greater income dispersion among the children is positively associated with the likelihood that the parent divides unequally could either imply that the parent gives more generously to children with low income (consistent with altruism) or more generously to the children with higher income (for example, to reward them for their past achievements).

We will instead provide more direct tests of the transfer theories by focusing on the distribution of bequests from the perspective of the child. More specifically, we test for how the inherited amount received by the child is affected by his or her economic and demographic traits.

Relating the inheritance of the child to his or her characteristics is not unproblematic. A simple cross-sectional regression is likely to produce biased estimates since the outcome is the result of preferences of the parent, which are unobservable and, presumably, correlated with the explanatory variables. For example, parents who desire a high level of consumption for their children may not only leave more generous bequests but may also have invested heavily in the children’s education. Since education is positively correlated with income, the coefficients estimate on child income is likely to be biased towards zero (McGarry 1999). Controlling for observable parent characteristics would only partly mitigate this bias. Moreover, since an inheritance by definition is only received at one point in time (as opposed to gifts, which could be received at several occasions), panel data methods cannot be employed to account for (time invariant) unobserved heterogeneity at the individual level.

We will instead exploit variation in inherited amounts and characteristics across children within the same families and estimate models with family-fixed effects.¹³ The family-fixed effect will effectively control for time-invariant observed and unobserved factors that are common for all children within the same family, but differ across families, such as parent inequity aversion. Using within-family variation rather than between-family variation is also appealing, as it is consistent with the predictions of the transfer theories. The coefficient estimates for the child-level variables from family-fixed effects models represent deviations from the within-family mean and could, hence, be interpreted as the impact of the characteristic relative to the siblings without the characteristic.¹⁴

We use the actual inherited amount as outcome variable. The estimation strategy thus requires that the inherited amounts vary across children within the same family. If parents give equally to all children, there would be no correlation between the explanatory variable and the inheritance; any deviation would be random (McGarry and Schoeni 1997). Consequently, we will rely on variation across families with unequally divided bequests.

In this respect, we differ from studies using survey data on bequest intentions to estimate the impact characteristics of the child will have on the probability that the child is (or will be) included in the parent’s will (Dunn and Phillips 1997; McGarry 1999; Light and McGarry 2004). These studies instead rely on variation from the particular sub-sample of families in which at least one child is not included in the set of bequest recipients (Menchik 1980; Brown 2006).¹⁵ To the best of our knowledge, Wilhelm (1996) is the only study exploiting within-family variation in inherited amounts to test bequest motives. While Wilhelm reports convincing results that inheritances provide negligible compensation to children with low earnings, it is difficult to generalize the findings to other settings, as they are based on a sample drawn from the uppermost tail of the wealth distribution. The mean amount of inheritance in the sample is almost USD 250,000 (in 1982 dollars) which is more than 20 times larger the mean inheritance in our sample. The study is also limited in that it lacks variables capturing the elements of the exchange and evolutionary models.

3 Data and study population

This section briefly details the data used for the analyses. It also describes how we proceed to obtain the relevant analysis sample, which contains children of parents who divide their estates unequally among the children.

3.1 The data

For the empirical analyses, we use the Belinda database, which covers information from the estates reports for all Swedes who passed away over the period 2002–2004 (around 90,000 observations per year). Elinder et al. (2014) describe the Belinda database more comprehensively. The database contains information on the deceased person’s identity number, date of death, marital status, whether there is a will, and the value of the estate at the time of death, as well as the bequest that is distributed between the heirs (including zeros).¹⁶

Of significance for our purpose, the database also contains the person identity numbers for all of the deceased’s legal heirs and beneficiaries of wills and their relationship with the deceased, as well as information about the inheritances they receive from the deceased (including zeros). The data on bequests and inheritances come from the Swedish Tax Agency’s Inheritance Tax Register, implying that errors from recall biases, underreporting, and non-response, which commonly couple other sources of data on intergenerational transfers, are of minor concern. The inheritance taxation integrated taxable gifts from the deceased to the heir during the previous ten years, and these gifts are therefore included in the database. Moreover, the database contains information on taxable insurances paid by the deceased with the heir as beneficiary. While the database does not cover all transfers in the form of gifts and insurance payments, we still believe that the data are valuable and we will use them in a sensitivity analysis.¹⁷

Relevant demographic characteristics for the heirs and the deceased parents that do not appear in the estate reports are retrieved from Swedish administrative registers: Birth Register (for date of birth and sex), Integrated Database for Labor Market Research (for place of residence, marital status, and level of education), and Income and Wealth Registers (personal income, net wealth) and are linked to the individuals using their person identity numbers.

The Belinda database does not contain information about the offspring of the deceased’s children. We therefore use the Multi-Generation Register, which contains information on all parent-child relations in Sweden, to link the children with their offspring (i.e., the deceased’s grandchildren). This data source also provides information on whether the child is a biological or adopted child of the deceased.

3.2 The analysis sample

We start out from the population of children heirs and their deceased parents in Sweden during the years 2002–2004, 455,544 and 201,581 individuals respectively. We hereafter use the term family to denote the parent-children entity. For our analysis, it is necessary to restrict the population in some dimensions. We impose six exclusion criteria. The first three naturally follow from our research questions, whereas the last three are needed in order to carry out the econometric analysis. The exclusions are made at the family level to assure that we keep all siblings within the family. The effects of the exclusion criteria on the sample size are summarized in Table 10 in Appendix A.

First, we exclude families with married or partnered decedents. This is because there is no, or only a partial, estate division and transfer to children when a married person passes away. There are similarly separate rules when a person leaves behind a cohabiting partner. Thus, we only include families for which a conventional estate division has taken place.

Second, we exclude families in which the parent passed away with no bequeathable wealth. This is because there are then no bequests to be transferred to the children.

Third, we exclude families with only one child, since there is then no estate division between children. Each family in our sample therefore contains two or more children and one parent.

Fourth, we exclude families for which we lack information about the inheritance amount for one or more children. Without this information, we cannot calculate the degree of unequal sharing within the family or identify within-family variation in inheritances.

Fifth, we exclude families in which a Swedish person identity number is missing for one or more children. Without a person identity number, we cannot add data on covariates to the child.

Sixth, we exclude families for which register data on some economic and demographic variables are missing in the registers for one or more children. This is because the coefficients with respect to the covariates in our econometric specifications are identified only for families in which there is variation in the variable.

Taken together, these adjustments leave us with a study population consisting of 60,430 families with 167,429 children.

As described in the previous section, our empirical analysis of sibling differences in inheritance amounts requires that there is variation in the inherited amounts within families implying that we should restrict the focus to families with unequally divided estates.

There are several different ways to define unequal division using our dataset. A first, fundamental, issue is, however, how one should think about decedents who have not written wills. Equal sharing of the estate between children is the legal default in Sweden if there is no written will. This is similar to the rules in other European countries and in the USA. The Swedish civil law, moreover, stipulates that half of the estate should be equally shared between the children even if there is a will. The other half of the estate can be freely bequeathed. A will is, therefore, a necessary, but not sufficient, criterion for unequal division of an estate.¹⁸ Among the families in the study population, 8156 (13.5%) have a will and 53,945 (86.5%) do not have one.¹⁹ One approach is to view the parent’s decision not to write a will as a desire to divide the bequests equally between the children. We should then calculate the frequency of unequal division using all families, including those without a will. However, since our empirical strategy, to test for the impact of child characteristics on relative inheritance amounts among siblings, requires that the estate is unequally divided, we consider only families with written wills, implicitly assuming that they are the only ones who have made conscious decisions whether or not the divide the bequests equally.²⁰

Regarding the classification of unequally divided estates, the most straightforward way would be to classify all deviations from exact equal division as unequal division. However, the issue with such an “exact” definition is that it classifies all differences in inheritance amounts among the children, also those resulting from rounding of amounts and cases in which it has been practically difficult to divide the assets so that the children receive equal amounts, as unequal division. Therefore, we consider a less restrictive definition, used in Wilhelm (1996), which classifies the estate as unequally divided if any child receives an inheritance that deviates more than ± 2% from mean inheritance calculated across all children within the family.²¹ A 2% deviation from the within-family mean in our sample corresponds to, on average, 3256 SEK (493 USD or 362 EUR).²²

Table 1 reports the incidence of unequal division according to the two definitions of unequal sharing. If we consider the “exact” definition (column 1) the incidence of unequal division is around 15.9%. The “± 2%” definition (column 2) yields an incidence that is slightly lower, 14.3%.

Table 1 Incidence of unequal division of estates among children

What could explain the discrepancy in incidences produced by the two definitions? A closer look at the families that divide unequally according to the exact definition but equally according to the “± 2%” definition can tell us something. First, we note that, for one third of the cases, rounding of amounts seems to be responsible for the discrepancy: the difference between the min and max inheritances within these families is less than 2 SEK. Moreover, the mean (median) of the difference between the min and max inheritance is 1500 SEK (500 SEK), which corresponds to less than 1% of the total bequest to the children. This suggests that the discrepancy in incidence (for cases where it is not rounding) is due to practical difficulties of distributing amounts equally rather the parents favoring/disfavoring one child over the other(s). We, therefore, consider the “± 2%” definition as the most preferable one. Consequently, restricting the study population to families with wills stipulating unequal division yields an analysis sample consisting of 3220 children heirs of 1166 families.

For a matter of completeness, we report (in parentheses) the incidences of unequal division also for all families, including those without wills (of whom some may have an explicit preference for equal division). The incidence of unequal sharing is, naturally, lower in this group: 3.3% and 2.4% according to the “exact” and “± 2%” definitions, respectively.

How well does the incidence of unequal division in our data correspond with the incidence reported in other studies? The incidence in data on actual bequest distributions from the USA (Menchik 1980, 1988; Judge and Hrdy 1992; Wilhelm 1996; Behrman and Rosenzweig 2004; Norton and Taylor Jr. 2005)²³ and France (Arrondel et al. 1997) ranges between 8 and 30%. Moreover, the incidence in survey data on parents intended division of bequests from the USA (Dunn and Phillips 1997; McGarry and Schoeni 1997; McGarry 1999; Light and McGarry 2004) and Japan (Horioka 2009) ranges between 8 and 22%.²⁴

The incidence of unequal division in our population falls somewhere in-between the ones reported in previous studies, with studies from the USA typically reporting higher incidences. A priori, one may expect the incidence of unequal division to be lower in Sweden than in the USA because parents in Sweden (as well as in most other European countries) are not allowed to completely disinherit their children. The children are always allowed to their statutory share which is half of what they would have received in the absence of a will, or put differently, the parent has testamentary freedom over half of the property.²⁵ In addition to differences in legal circumstances, differences in culture and social norms across countries and over time (Horioka 2016) as well as changes in family structure (Francesconi et al. 2015) may also explain why estimates differ across studies. The general finding in the literature is, however, that the lion’s share of parents divides their estates equally between the children.

3.3 Summary statistics for key variables

To get a sense of the magnitudes of the empirical estimates, we report, in Table 2, descriptive statistics (means and, for continuous variables, standard deviations, reported in parentheses) for parent (decedents) and children characteristics.

Table 2 Sample characteristics of parents and children of families with unequally and equally divided estates

For each child characteristic, we, moreover, report the incidence of variation at the family level. This is to show for what fraction of families we identify the coefficients on the explanatory variables. Continuous variables, besides the incidence of variation, are accompanied by the coefficient of variation (reported in brackets).²⁶

The first two columns report the descriptive statistics for the main sample: families with unequally divided estates.

The parent characteristics are intended to capture the parent’s taste or ability to divide unequally and are the same that commonly appear in previous studies: the estate value, income, age, gender, marital status, level of education, and number of children.

We see that the average estate amounts to slightly more than SEK 447,000 and the mean income is SEK 155,900. Moreover, the average parent is 83.4 years old at death and women and widows/widowers are in majority (57.3% and 77.5%, respectively). Less than 10% have university education and the average parent leaves behind 2.8 children.

Regarding the children characteristics, we see that the mean inheritance (before transfers taxes were paid) amounts to slightly more than SEK 137,500. The incidence of within-family variation in the variable is 100%. This follows from the fact that the sample contains only those families with unequally divided bequests. Moreover, the coefficient of variation indicates that a great deal of inequality results from unequal inheritances to children within the same family.

The mean permanent income is around SEK 245,000 per year. Moreover, we see that the mean wealth is slightly more than one million SEK, which is more than seven times larger than the mean inheritance. In all families, there are differences between the children in income and in wealth (within-family variation is 100%) implying that all families will contribute to the identification of the coefficients on the variables. Moreover, we see that almost one third of the children have university education and that the within-family variation is around 41%.

Regarding the variables relating to the exchange model, we note, first, that there are somewhat fewer women than men among the children. The within-family variation, however, indicates that a majority of the families contain both women (daughters) and men (sons). We also see that about one fourth of the children resided in the same parish as the parent prior to the demise and that 11% are daughters living in the same parish as the parent. At the family level, the incidence of variation with respect to these two variables is above 45 and 26%, respectively. Moreover, a slight majority (53%) of the children are married and for 60% of the families, there is a mix of married and unmarried children. Finally, the incidence of the interaction between living in the same parish as the parent and being married is almost 13% (with a within-family variation of 28%).

The variables related to the evolutionary model are reported in the bottom panel of the table. We see that slightly more than 2.7% of the children are adopted and the identifying variation comes from the 4.5% of the families that have a mix of adopted and biological children.²⁷

Finally, we note that 82% of the children have at least one child of their own (within-family variation is 36%) and that almost 42% of the sample consists of women with children, together producing an average within-family variation of almost 65%.

In the two rightmost columns, we report descriptive statistics for families with equally divided bequests. We see that parents who divide their bequests equally are remarkably similar to parents who divide unequally. The only notable differences are that the incidences of women and widows/widowers are slightly higher in the sample of equal dividers than in the sample of unequal dividers. We note, however, that the differences between the two groups are starker in terms of children characteristics and, especially in within-family differences. In fact, for all children characteristics, the variation in characteristics among siblings is higher in families with unequally divided bequests than in families with equally divided bequests. This is consistent with the transfer theories, which predict that the likelihood of unequal division is higher if siblings differ greatly in their characteristics. In Section 4.1, we test for differences in parent and children characteristics between families that divide unequally and equally using regression analysis.

4 Empirical analysis

In this section, we report the results from the empirical analyses. The first subsection provides estimates of the factors influencing the parent’s decision to divide the bequest unequally. The second subsection details the results from an analysis of the determinants of variation in inherited amounts among siblings. The third reports estimates from a generalized tobit model. And, finally, the fourth subsection reports some additional results.

4.1 Are children more different in families that divide unequally?

In this section, we present estimates of the factors influencing the parent’s decision to divide the bequest unequally. The motivation for this is twofold. First, it allows one to evaluate how families with unequally divided bequest compares with families with equally divided bequests. Second, it allows one to evaluate to what extent our sample is comparable to the samples used in previous studies.

Practically, we estimate a linear probability model with the dependent variable being an indicator variable for whether the bequest is unequally divided, as defined by any child receiving outside ± 2% of the within-family mean.²⁸ The estimation sample consists of 8156 families with unequally divided estates (1166) and equally divided estates (6990).²⁹

The explanatory variables entering the estimations are the parent and children characteristics discussed in Section 3.3. The coefficients on these child-level variables should indicate whether the parent’s decision to divide equally or unequally is in line with the transfer theories (altruism, exchange, evolutionary). However, it should be noted that the coefficients only are informative about how the distribution of traits among the children correlates with the distribution decision, and not on what grounds the parent favor or disfavor particular children (which is the most direct test of the theories and the focus of the analysis in the next section).

The regression results are reported in Table 3 and may be summarized as follows: the likelihood of unequal sharing of bequest between children is unrelated to the size of the estate and the parent’s income. The finding corresponds with that in Light and McGarry (2004). Moreover, we find that older parents are more likely to divide unequally than younger parents. Women, compared to men, are less likely to divide unequally. This is consistent with the results in Wilhelm (1996). Widows/widowers are less likely to divide unequally than divorced decedents and decedents who have never married. The distribution decision is unaffected by the deceased’s level of education. Moreover, the decision to divide unequally is positively associated with the number of children, though at a decreasing rate.

Table 3 The determinants of unequal division of estates

Regarding the child-level variables, we see that a higher inter-sibling dispersion in permanent income and in wealth, measured by the coefficient of variation (cv),³⁰ is associated with a higher likelihood of unequal division. This is consistent with the prediction of the altruism model (assuming that more inheritance is transferred to the less affluent child) and in accordance with the results in McGarry (1999) and Light and McGarry (2004). Having at least one child with university education reduces the likelihood of unequal division among families with wills whereas having a mix of children with and without university education increases it. The latter finding could be considered in line with the results for income and wealth.

Having one or more daughters is negatively associated with unequal division whereas the indicator for having both daughters and sons (as opposed to having only sons or daughters) is positive, indicating that parents may have preferences for one sex over the other. Moreover, having a mix of children living and not living in the same parish is positively associated with the outcome. Assuming that daughters and children living close to the parents receive disproportionally more these findings could be seen as support for the exchange model.

Moreover, in line with previous tests of the evolutionary model (Light and McGarry 2004; Francesconi et al. 2015), we find that having a mix of biological and adopted children increases the likelihood of the outcome. Having grandchildren reduces the likelihood of unequal division, but having a mix of children with and without children of their own increases it. This result is also line with Light and McGarry (2004).

It is obvious from the analysis reported above that the decision to divide unequally does not appear random; instead, it depends on attributes of the children and, in particular, within-family differences in characteristics and behaviors. While the patterns are consistent with the three transfer theories (altruism, exchange, and evolutionary), they should only be considered suggestive evidence. For example, that a mix of biological and adopted children increases the likelihood of unequal sharing is only consistent with the evolutionary model given that the adopted children receive less than the biological ones, which is not found in the analysis. In the next section, we thus investigate how differences in inheritance amounts among siblings in families with unequally divided bequests are affected by differences in siblings’ characteristics and behaviors.

4.2 The determinants of within-family differences in inherited amounts

This section presents an analysis of the determinants of variation in inherited amounts among siblings. The analysis is based on children of families with unequally divided bequests, in total 3220 children of 1166 families.³¹

We exploit variation across siblings and estimate models with family-fixed effects to test for the impact of child attributes on inherited amounts. The basic specification is of the following form:

$$ {y}_{i,f}=\alpha +\boldsymbol{\delta} {\boldsymbol{X}}_{\boldsymbol{i}}+{\lambda}_f+{\varepsilon}_{i,f}, $$

where y_{i, f} is the inherited amount, in SEK 100,000, received by child i of family f.³²X_i is a vector of the child characteristics displayed in Table 2, and λ_f is a family-fixed effect that varies across families, but is common to all children within the same family. The fixed effect does not only control for unobserved heterogeneity at the family level but also for observable parent characteristics. The parameter of interest is δ and it measures how the transfer received by child i is related to her characteristics, relative to the within-family average. In addition to the variables associated with the transfer theories, we augment the model with indicator variables for the children’s age, in years.³³ To account for the possibility that the parent’s bequest behavior is correlated with family size, we weight the observations by the inverse of the number of children in the family.³⁴

The results are reported in the following way: each bequest theory is first tested individually using separate regressions for each child characteristic(s) that is (are) related to the theory, and then, finally, we test the theory in a regression including all children characteristics (those related to the specific theory and those related to the other theories). This joint test should be considered the most reliable one since it accounts for the largest set of observable (and potentially unobservable) factors affecting the inheritance amount.

Starting with the altruism model, Table 4 column 1, we see that the coefficient estimate on the permanent income variable is negative, but not statistically different from zero at conventional levels. This corresponds with the results in Wilhelm (1996) and could be seen as proof against the altruism model’s prediction regarding perfect equalization, which requires a statistically significant negative one-to-one relationship between income and inheritance amount. One possible explanation for the absence of a link is that the three-year average of (current) income is a poor proxy for permanent income (McGarry 1999). We therefore considers the child’s wealth as an additional proxy for her lifetime consumption possibilities. Assuming that wealth is a valid proxy, the altruism model predicts that parents will transfer more to children who are relatively less well off in terms of wealth, implying that we would expect a negative coefficient if the theory holds up. The coefficient estimate (column 2) is, however, similarly to that on income, statistically insignificant at conventional levels. One may think that education is a better proxy for permanent income than a three-year average of (current) income and thus, that siblings with relatively high education should receive less than those with comparably low education, if bequests are compensatory. However, we find that education is, if anything, positively related with the inherited amount, a finding that also speaks against the altruism model (see column 3). This relationship remains when we control for income, wealth, and education simultaneously, as well as for other characteristics that are likely to determine the relative inherited amount (see table note), as do the (insignificant) coefficients on income and wealth, see column 4. Taken together, the results in Table 4 are inconsistent with the prediction of the altruism model that bequests are compensatory.³⁵

Table 4 Test of the altruism model

The results with respect to the exchange model are reported in Table 5. First, we see that the coefficient estimate on the indicator for being daughter (column 1) is positive and statistically significant, implying that daughters receive more than sons. While this result is in line with the hypothesis that daughters are more engaged in service provision and compensated accordingly, it is also consistent with the predictions of Wedgewood (1928) and Blinder (1973) that parents have preferences for daughters over sons. Moreover, we see that children living in the same parish as the parent receive more than their siblings living further away (column 2).³⁶ This is consistent with the prediction of the exchange model that parents purchase more services (with bequests) from children for whom the cost of provision is relatively low. Relating the point estimate to the mean inheritance yields that a child living in the same parish as the parent receives 14% more than the sibling(s). In column 3, we report the results from a specification with controls for being daughter and living in the same parish as the parent as well as interaction between the two characteristics. The coefficient estimate on the latter variable is statistically insignificant, implying that daughters living in the same parish as the parent do not receive larger inheritances than sons living in the same parish as the parent. This suggests that parents do not discriminate with respect to sex but rather that they compensate for service provision, as indicated by the positive and statistically coefficient on Same parish. The coefficient on the latter variable is statistically significant and positive in the most extensive specification (column 8) as well, and a comparison of the estimate to the mean inheritance implies that children living in the same parish as the parent receive 35% larger inheritances than their siblings living elsewhere.

Table 5 Test of the exchange model

Moreover, we do not find any evidence that married children (who are less likely to be service providers because of their relatively higher time cost) receive less than their never married or divorced siblings (column 4). However, we do find that the interaction between being married and living in the same parish as the parent is negative and statistically significant (column 5), a finding that appears to be robust to the inclusion of all controls (see column 8). This could be viewed as further support for prediction of the exchange model that larger inheritances should flow to children for whom the opportunity cost of time is relatively low. The coefficient estimate implies that being married and living in the same parish as the parent is associated with a 26% lower inheritance.

In columns 6 and 7, we report estimates of the impact of the child’s relative birth order on the inherited amount. Looking first at column 6, we see that relative to the firstborn child, there is a steady increase in inheritance amount by birth order. This finding is in line with the conjecture that parents compensate the later-born children for their disproportionally higher provision of services and attention. The finding that later-born children receive more than their earlier-born siblings remains also in the more extensive specification, see column 8. The independent, positive effects of birth order and the same parish indicator indicate that the latter variable does not just pick up the fact that younger children are more likely to reside close to the parent.³⁷ Moreover, the fact that the birth order effect is robust to the control for education and income indicates that it does not capture parents’ use of inheritances to compensate their later-born children for potentially lower investments in these children during their childhood.³⁸ While the pattern of the birth order effect indicates that younger children receive more than their earlier-born siblings, it is not really informative as to whether the youngest child receives relatively more, because the number of children varies across families (see Appendix Table 11). In column 7, we test for this more specifically by adding an indicator for whether the child is the youngest sibling, and the coefficient estimate on this variable shows that it is indeed the case that the youngest child receives the most. This is consistent with the conjecture that last-born children have closer relationships with their parents (e.g., Whiteman et al. 2003; Suitor and Pillemer 2007) and are compensated for this with larger bequests. The result holds also in the extensive specification (column 8) and the estimate implies a 12% larger inheritance to last-born children.³⁹ In sum, we interpret the findings with respect to birth order as further support for the exchange model but acknowledge the possibility that parents favor their later-born children because these children have a relatively longer life expectancy; hence, that money may be more valuable to them.

We now turn to the tests of the evolutionary model of bequests, in Table 6. We find no evidence that children who have children of their own receive more or less than their siblings without children of their own (columns 1, 2, and 5). This suggests that parents neither use bequest to encourage childless children to reproduce or to reward children that have already produced grandchildren. We see, however, that the interaction between being daughter and having children is positive and statistically significant (p < 0.05), implying that daughters with children receive more than sons with children (see columns 2 and 5). This corresponds with the evolutionary model, predicting that parents care about the continuation of the bloodline and favor the offspring of daughters, as these are certain to be genetic descendants (Cox 2003). The implied percentage difference in inheritance amount relative to that of brothers with children is 31% (from column 5).⁴⁰

Table 6 Test of the evolutionary model

Further support for parents’ bequest behavior being governed by evolutionary motives is found in column 3, displaying that adopted children receive substantially less than siblings who are the parent’s biological children. A comparison of the point estimate and the average inheritance implies a 58% difference in amounts. However, the indicator in column 3 makes no distinction between adopted children with one or two adoptive parents, it only conditions on the child being adopted by the current deceased parent, from whom the bequest is received. As noted in Section 4, 2.7% of the children in our sample are adopted. However, 2% of the children (71% of the adopted children) are adopted only by the current deceased parent.⁴¹ While it is possible that some of these children have been adopted by single parents, it is more likely that they are stepchildren who have been adopted by a stepparent. To study more carefully whether the adopted effect is driven by adopted stepchildren being disfavored (relative to their siblings) by their adoptive stepparents, we substitute the previous adopted indicator with two new variables for adopted status: one indicator indicating whether the child is adopted by both parents and one indicator indicating whether the child is adopted only by the parent from whom the bequest is received. The results from this specification are reported in column 4. It can be seen that adopted children with two adoptive parents do not receive differently from their siblings who are the parent’s biological children: the coefficient estimate is, though negative, statistically insignificant. However, the indicator for being adopted only by the giving parent is negative and statistically significant at the 5% level suggesting that adopted stepchildren receive less than the siblings. This finding holds also in the specification with all additional controls (column 5) and suggests that the adopted effect is largely driven by families in which adopted stepparents disfavor their adopted stepchildren. The coefficient estimate implies that adopted stepchildren receives 63% less than their siblings do. There is, in other words, a Cinderella effect. This finding is in line with findings in Light and McGarry (2004) and Francesconi et al. (2015). However, Light and McGarry (2004) report evidence suggesting that the mothers in their sample may disfavor both their adopted children and their stepchildren. In the next section, we provide a discussion about why our results appear to be different from theirs.

Taken together, the results presented above provide little support for the altruism model but some support for the exchange model and the evolutionary model. The support for the latter two theories exists jointly (as indicated by the significant coefficient estimates in the rightmost columns in Tables 5 and 6) and is in line with the general conclusion in the literature that bequest decisions are governed by a mix of motives, rather than a single one (e.g., Kopczuk 2013). While we cannot test for whether mixed motives are present for a given person at the same time, we can test for heterogeneity in preferences in the study population. In the following section, we do so as well as assess the robustness of our main findings.

4.3 Generalized tobit estimates

The results from the analysis in Section 4.1 show that families with unequally divided bequests differ from families with equally divided bequests, particularly with respect to within-family variation in child characteristics. In this section, we asses to what extent our main estimates are influenced, or biased, by the fact that they are based on families where the parents have consciously decided to divide unequally. To test for this, we follow Wilhelm (1996) and estimate a generalized tobit model (GTM). The GTM models the decision whether or not to divide unequally (the participation decision) and the decision regarding the extent of unequal division, conditional on unequal division (the quantity decision) jointly, allowing the two decisions to be correlated.⁴² Wilhelm (1996) assumes that the decision to divide unequally is associated with a psychic cost resulting from, for example, inter-sibling jealousy and family conflict.⁴³ The conjecture is that parents for whom the differences in child characteristics are large relative to the psychic cost are more likely to divide unequally. In the estimations of the GTM model, we consequently use heirs from families with both unequally and equally divided estates. The unit of observation in the estimations is the child and the interpretation of the coefficients in the participation equation is thus, how belonging to a family with the certain characteristic (say decedent with university education or a high level of inter-sibling dispersion in income) affects the likelihood of belonging to a family with a parent dividing unequally. The coefficients in the quantity equation (i.e., the impact of the child’s characteristics on the relative inherited amount) should be interpreted as deviations from the within-family means, as previously. The estimates with respect to the child characteristics are reported in Table 7 while reports the estimates from the participation equation; in other words, the determinants of the decision to divide unequally or, in the terminology of Wilhelm (1996), the psychic costs. We see that the estimates for the child characteristics are akin to the main estimates. The few notable differences are, first, that the estimate of the interaction between living in same parish as the parent and being married is marginally statistically insignificant (p = 0.108), rather than statistically significant (although, still negative), second, that the indicator for having children is now statistically significant and negative (implying that children with children on their own receive less than their childless siblings) and, finally, that the birth order effects are statistically insignificant. Moreover, going from the main family-fixed effects model to the GTM decreases the magnitudes of the estimates only slightly. In sum, these findings suggest that the selectivity bias from using a sample of children from families with unequally divided estates is negligible and that accounting for it does not alter our main conclusions. This is consistent with the findings in Wilhelm (1996).⁴⁴ Regarding the estimates for the characteristics in the participation equation (Appendix Table 14), we see that they largely resemble the estimates reported in Table 3 in terms of sign and statistical significance.⁴⁵

Table 7 Generalized tobit model of within-family inheritance, children’s characteristics (quantity equation)

4.4 Additional results

In this section, we present results from some sensitivity analyses with respect to the estimates in Section 4.2.

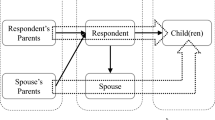
Children of widowed descents (77% in our sample) typically receive two inheritances: one from the currently deceased (widowed) parent and one from the previously deceased parent. This is because, when a married person in Sweden passes away, the estate is transferred to the surviving spouse and, if the spouses have common children, the children receive the inheritance from the first deceased parent when the second parent passes away. The focus of the main analysis in the previous section is on the decisions of the currently deceased parent. However, it is possible that the children who are disfavored (favored) by the currently deceased parent have been favored (disfavored) by the previously deceased parent. For example, a disfavored stepchild may receive a disproportionally larger inheritance from the previously deceased (biological) parent and hence that the child receives similarly to the siblings if we consider the total inheritance amount instead. In Table 8, column 1, we report estimates from a regression with the total inheritance amount as dependent variable. It can be noted that these estimates are akin to the main estimates in terms of sign and statistical significance suggesting that it is not the case that disfavored (favored) children receive disproportionally more (less) from the previously deceased parent.⁴⁶

Table 8 Sensitivity analyses

In Table 8, columns 2 and 3, we redo the analysis separately on a sample excluding families with deceased who have given gifts (to at least one child) during the ten years before death (column 2), and a sample excluding families with deceased who have transferred wealth through insurance to at least one child (column 3). This is to test whether the main results are driven by decedents who, potentially, already have achieved their desired compensation during life, through gifts, or at death, through insurances (rather than through bequests).⁴⁷ Neither of these sensitivity checks alters the estimates substantially, with the exception that in the no-insurance sample, the coefficient on the married indicator is statistically significant at the 10% level and the having children indicator is statistically insignificant. The latter coefficient is, however, of the same order of magnitude and has the same sign as the corresponding main estimate.

In Table 8, column 4, we consider a different model specification in the tradition of Mundlak (1978). The model includes the same child characteristics as previously, but rather than explicitly controlling for family-fixed effects, we include as additional regressors the parent-level variables that entered the probability models for unequal division, as well as child characteristics that are averaged over all children in the respective family (and hence do not vary across children within the same family). By conditioning on parent characteristics and child means, we capture within-variation at the family level and could thus interpret the coefficients on the child characteristics as in the family-fixed effects regressions. The results with respect to the child-level variables display a similar pattern as the main estimates, suggesting that the main estimates are robust to this change in model specification.⁴⁸

One concern with the interpretation of the positive impact of living in the same parish as the parent as compensation for services is that the location choice of the child may be due to the child’s own needs of services from the parent, rather than by the needs of the parent. To investigate this more carefully, we test for whether characteristics of the child that indicate needs of assistance influence the inherited amount. The first characteristic we consider is the child’s health status. The conjecture is that children in poor health receive larger inheritance than the siblings. The child is assumed to be in poor health if he or she has been hospitalized for any cause and/or has had any (insured) sick leave during the three years prior to the demise.⁴⁹ The second characteristic is unemployment. We define unemployment based on whether child has received any unemployment benefits during the three years prior to the demise and the conjecture is that unemployment is associated with financial strain, which is compensated for with larger inheritance (Cox 1990).⁵⁰ The third characteristic we consider is whether the child has children and it should be seen as a proxy for the child’s need of childcare. In Table 8, column 5, we report results from an estimation including each characteristic separately as well as interactions between the three characteristics and the indicator for living in the same parish as the parent. The indicator on unemployment is statistically insignificant at conventional levels. The indicator for the presence of children is statistically insignificant as in the main specification (Table 6, column 5), suggesting that the, potential, need of childcare does not translate into larger inheritance. However, the indicator for poor health is statistically significant and negative, suggesting that parents, if anything, give less to children in poor health. Regarding the interactions between the needs indicators and same parish, neither of them turns out statistically significant and, reassuringly, the indicator for same parish remains statistically significant and positive. Taken together, these results strengthen our conclusion that the relationship between location choice of the child and the inheritance amount is due to exchange motives.

As an additional way to evaluate the robustness of the impact of location on the inheritance amount as support for the exchange motive, we test for whether the relationship is stronger if the parent has been in need informal care. We use three proxies for informal care needs, constructed using data from the Swedish National Patient Register and the Cause of Death Register, to test this hypothesis. The first proxy is an indicator variable for whether the parent has suffered from dementia (which is often referred to as the disease that requires most informal care, e.g., Wimo et al. 2007) during the three years prior to the demise.⁵¹ The second one is an indicator variable for whether the parent has suffered from a stroke (which is also informal care intensive disease, e.g., Albrecht et al. 2016) at any time during the three years prior to the demise.⁵² Finally, the third proxy, is an indicator variable for whether the parent has had any surgery (which is commonly associated with need of informal care), for any cause, during the three years prior to the demise.⁵³ The empirical test is conducted by regressing the empirical specification used in Section 4.2 augmented with interaction terms between the informal care need indicators and the indicator for living in the same parish as the parent (the informal care need indicators are captured by the family-fixed effects). A positive and statistically significant coefficient on the interaction terms implies that the difference in inheritance amounts between siblings living and not living in the same parish is larger if the parent has had larger health-related care needs and should be seen as support for the hypothesis. The results are reported in Table 8, column 6, and we can see that the interaction between parent having had dementia and the same parish is statistically significant (p < 0.10). The interactions with respect to stroke and surgery are positive as well, though statistically insignificant. Taken together, these findings provide some additional evidence that informal care provided by the children is compensated for with larger inheritance, in line with the findings in Brown (2006).

Our results differ from the results in Light and McGarry (2004) in that they find suggestive evidence that both adopted children and stepchildren are disfavored in the bequest division, whereas our results (see Table 6) imply that only adopted stepchildren are disfavored. One potential reason for the discrepancy in findings is that the sample used in Light and McGarry (2004) consists of mothers only, while our sample contains both mothers and fathers (albeit with an predominance of mothers). However, when we restrict the sample to mothers and redo the analysis, we find that both the indicator for being adopted by two parents and the indicator for being adopted by the giving parent only are negative and statistically significant, see Table 9, column 1. This suggests that the mothers in our data behave similarly to the mothers in Light and McGarry (2004) in that they disfavor both (adopted) stepchildren and adopted children. We note, however, that the interaction between being married and living in same parish as the parent is statistically insignificant. This is the case also for the birth order indicators (except for the youngest child indicator). How about if we restrict the sample to fathers? The estimate for the adopted stepchild indicator is negative as previously, while the estimate for the indicator for being adopted by both parents is positive, suggesting that fathers give disproportionally more to these children, see Table 9, column 2. However, both estimates are statistically insignificant and should thus be interpreted with caution. In fact, the estimates with respect to all child characteristics, except for the birth order indicators (less the indicator for youngest child), are statistically insignificant, although they have the similar sign and are of similar magnitudes as the ones obtained for the main sample. The imprecise estimates may stem either from fathers not discriminating with respect to these characteristics or from insufficient statistical power resulting from the relatively small sample size.

Table 9 Heterogeneity analyses with respect to parent’s sex and within-family dispersion in income and wealth

Moreover, in Table 9, columns 3–6, we test for how the results with respect to income and wealth change if we restrict the sample to families with a low or high dispersion in these variables (based on the within-family coefficient of variation being below or above the median in the sample), the conjecture being that parents are more (less) likely to make compensatory transfers if dispersion is relatively high (low). The estimates with respect to income and wealth obtained from these sub-samples, however, are similar to the main estimates in that the coefficients on income and wealth are statistically insignificant in general. One exception is that the coefficient for wealth is statistically significant (p < 0.10) in the sample with high wealth dispersion. However, the estimate is positive, suggesting that, if anything, wealthier children receive more than their less wealthy siblings! One speculative explanation for this unintuitive finding is that parents use bequests to reward wealthier children for their economic success. However, as for the heterogeneity analysis with respect to the parent’s sex, one should interpret the estimates with caution as they are based on relatively small samples.

5 Concluding discussion

A large literature provides empirical tests of the theoretical models of bequest motives. However, the previous studies rely either on survey data on parents’ bequest intentions, focusing on the particular subset of families in which the parent will disinherit at least one child, or on data from tax records that commonly only cover bequests from the very wealthy and lack control variables to facilitate empirical tests of several bequest theories.

Our contribution to this literature is that we use a population-wide dataset covering individual-level data on realized inherited amounts for complete families (deceased parents and all their children), matched with an extensive set of economic and demographic variables from administrative registers. This data allows us to test three bequest models: altruism, exchange, and evolutionary, by estimating the influence of child characteristics on differences in inherited amounts among siblings. To the best of our knowledge, we are the first to test the importance of more than one bequest motive by exploiting within-family variation in inherited amounts.

We do not find any support for the altruism model: there is no correlation between the inherited amount and child’s economic circumstances, measured either as permanent income, wealth, or education. This is in line with the general finding in the earlier literature that bequests do not tend to be compensatory (e.g., Wilhelm 1996; Dunn and Phillips 1997). These findings suggest that that government efforts to reach intergenerational redistribution are unlikely to be counteracted by bequests (as opposed to the prediction of the Ricardian equivalence proposition).

We do find, however, that in families with unequally distributed estates, children who are more likely to have provided services to the parent (e.g., because they lived close to the parent) receive larger bequests than their siblings. This could be interpreted as if, at least for some parents, transfers are motivated by exchange.

A large share of the population in most Western countries is involved in caregiving for an older parent, experiencing lost labor income, pensions, and other work-related benefits. Because of increasing longevity and life expectancy, the number of elderly persons with chronic health conditions who are in need of caregiving by their adult children is expected to increase even further, and one may expect to see exchange-motivated bequests to grow in importance, with potential consequences for economic policy (Yakita 2018).

We also find some support for the evolutionary model in the data. First, daughters with children receive more than sons with children, implying that parents have preferences for descendants that are more certain to be genetic. Second, adopted stepchildren receive less than siblings who are the biological children of both parents or adopted by both parents. This is consistent with the predictions of models from evolutionary psychology. The fact that the effect is largely driven by disfavored adopted stepchildren indicates that bequest decisions are influenced by so-called Cinderella effects.

An increase in incidence of divorce, remarriage, repartnering, and cohabitation has led to an evolvement of new, more heterogeneous family arrangements with both biological and non-biological children (Lundberg and Pollak 2007; Stevenson and Wolfers 2007). To the extent that these patterns will continue, one may expect unequal division of estates to become more common.

It should be noted that our focus is on the determinants of parents’ decisions regarding the allocation of bequests at death and not the allocation of gifts during life. That we do not find any support for the altruism model may be because some parents have already achieved their desired compensation during life, through inter vivos gifts, as indicated by, for example, McGarry and Schoeni (1995), Dunn and Phillips (1997), and Hochguertel and Ohlsson (2009). This is consistent with the model in Chang and Lou (2015), which builds on Cigno (2006) and predicts that concerns regarding distributional fairness lead altruistic parents to divide their estates equally while they divide inter vivos gifts unequally for strategic reasons (i.e., to induce the children to provide merit goods, such as companionship). Although our sensitivity tests indicate that such compensation has not been achieved with reported taxable gifts, we cannot assess the relative importance of unreported and non-taxable gifts. An ideal, complete account would, however, not only consider monetary gifts but also gifts in terms of time, social networks, and other parental resources, which seem to matter a lot for inequality in success across children (Björklund et al. 2012).

We should also emphasize that this analysis is based on families where the parent has decided to divide the estate unequally among the children. We cannot, therefore, say anything about the bequest motives for parents who divide their estates equally.

While there is no feasible strategy to test the bequest motives of these parents, there are at least three possible reasons for why equal division of their bequests reflects a deliberate choice rather than the bequests being “accidental.”

First, the estate allocation is public information and the children can directly see how their shares compare with their siblings’ and thereby might interpret this as if they are loved more or less than their siblings. If parents care about their reputation after death, equal treatment with respect to bequests may be considered the most rational and desirable decision (Lundholm and Ohlsson 2000; Bernheim and Severinov 2003).

Second, parents may choose equal treatment because the alternative, unequal division, could lead to jealousy and conflicts among the children, and ultimately, a breakdown of the family as a social entity (Menchik 1988 and Wilhelm 1996).

Third, parents might distribute their estates equally because it is less costly and requires less effort and, therefore, may be more rational than other distributive principles (Elster 1989). The parent does not have to collect and compare information on the financial status of the children and the parent does not have to value the services provided by the children.

Appendices

Additional data description

Table 10 Exclusion criteria and study population

Table 11 Number of children in the family, by family

Table 12 The determinants of unequal division of estates, families with and without wills

Additional estimations, the determinants of unequal amounts

Table 13 Estimates of birth order effects on geographical proximity to parent and education

Table 14 Generalized tobit model of within- family inheritance, family’s characteristics (participation equation)

Table 15 Replication of Wilhelm (1996)