Curved channels with constant cross sections may support trapped surface waves

Abstract

Curved channels with constant cross sections are constructed which support a trapped surface wave. Since corresponding eigenvalues are embedded in the continuous spectrum of the water wave problem and therefore possess the natural instability, the construction procedure requires “fine-tuning” of several parameters in the (small) curvature of the channel as well as geometrical restrictions on the cross section. In particular, the mirror symmetry of the cross section with respect to the vertical axis disrupts the procedure, but examples of suitable non-symmetric cross sections are provided.

1 Introduction

1.1 The formulation of the problem

Let $\Omega ={\mathbb {R}}\times \omega$ be a straight cylinder in the Euclidean space ${\mathbb {R}}^3$ with a cross section $\omega \subset {\mathbb {R}}^2$ bounded by the line segment $\gamma =\{x'=(x_2,x_3):\ x_3=0,\ |x_2|\le 1\}$ and a piecewise smooth curve connecting the points $(\pm 1,0)$ inside the lower half plane ${\mathbb {R}}^2_{-}=\{x':\ x_3<0\}$, see Fig.  1. The rescaling has been performed in order to reduce the length of $\gamma$ to 2. Considering $\Omega$ as the water domain, the free surface is denoted by $\Gamma ={\mathbb {R}}\times \gamma$ and by $\Sigma =\partial \Omega {\setminus } {\overline{\Gamma }}$ the union of walls and bottom.

Let $\Upsilon ^\epsilon$ be a slightly deformed mid-line $\Upsilon$ of $\Gamma$, the abscissa axis, where $\epsilon \ll 1$ is a small positive parameter. In the neighbourhood ${\mathcal {U}}$ of $\Upsilon ^\epsilon$ on the plane $\{x:\ x_3=0\}$, we introduce the local coordinate system $(n,{\varsigma })$, where $n$ is the oriented distance to $\Upsilon ^\epsilon$ and ${\varsigma }$ is the arc length on $\Upsilon ^\epsilon$. We assume that the curvature $\kappa ^\epsilon ({\varsigma })$ of $\Upsilon ^\epsilon$ satisfies the conditions

$$\begin{aligned} \kappa ^\epsilon ({\varsigma })=\epsilon \kappa ^0({\varsigma }),\ \kappa ^0({\varsigma })\in C^\infty ({\mathbb {R}}),\ \kappa ^0({\varsigma })=0\ \text {for } |{\varsigma }|>l>0. \end{aligned}$$

(1.1)

The curved channel with a constant cross section $\omega$, in Fig. 2, is then

$$\begin{aligned} \Omega ^\epsilon =\{x: {\varsigma }\in {\mathbb {R}},\ (n,z)\in \omega \}, \end{aligned}$$

(1.2)

where $z=x_3$ is the vertical coordinate. According to (1.1), the channel (1.2) has the straight cylindrical outlets to infinity $\Omega ^\epsilon _{\pm }=\{x\in \Omega ^\epsilon :\ \pm {\varsigma }>l\}$ and the curved middle part $\Omega ^\epsilon _0=\{x\in \Omega ^\epsilon :\ |{\varsigma }|<l\}$, see Fig. 2.

In the channel, we consider the linearized water-wave problem, see, for example, [14], consisting of the Laplace equation

$$\begin{aligned} -\Delta u^\epsilon (x)=0,\ x\in \Omega ^\epsilon , \end{aligned}$$

(1.3)

together with the Neumann condition (no flow condition)

$$\begin{aligned} \partial _\nu u^\epsilon (x)=0,\ x\in \partial \Omega ^\epsilon \setminus \overline{\Gamma ^\epsilon }, \end{aligned}$$

(1.4)

and the (kinematic) spectral Steklov condition

$$\begin{aligned} \partial _z u^\epsilon (x)=\lambda ^\epsilon u^\epsilon (x),\ x\in \Gamma ^\epsilon , \end{aligned}$$

(1.5)

on the free surface $\Gamma ^\epsilon =\{x:\ z=0,\,|n|<1\}$. Here, $u^\epsilon$ is the velocity potential and $\lambda ^\epsilon =\varpi ^2_\epsilon g^{-1}$ is the spectral parameter, where $\varpi _\epsilon$ is the frequency of the time-harmonic oscillations and $g>0$ the acceleration due to gravity.

In the sequel, the problem (1.3)–(1.5) is referred to as problem ${\mathcal {P}}^\epsilon$, while at $\epsilon =0$ we obtain the problem ${\mathcal {P}}^0$ in the straight channel $\Omega ^0=\Omega$.

Fig. 1

A straight channel and the cross section

Fig. 2

a Curved channel, b The cross section

1.2 Spectra of the problems

The continuous spectrum $\sigma ^0_{co}$ of the problem is the closed real semi-axis $\overline{{\mathbb {R}}_+}=[0,+\infty )$ in the complex plane ${\mathbb {C}}$. The threshold values

$$\begin{aligned} 0=\Lambda _0<\Lambda _1\le \Lambda _2\le \dots \le \Lambda _j\le \dots \rightarrow +\infty \end{aligned}$$

(1.6)

divide $\sigma ^0_{co}$ into the intervals of constant multiplicity. In what follows, we consider the first interval $(0,\Lambda _1)$. The entries of the sequence (1.6) are the eigenvalues of the model problem on the cross section

$$\begin{aligned} -\Delta ' U(x')= & {} 0,\ x'\in \omega ,\nonumber \\ \partial _\nu U(x')= & {} 0,\ x'\in \partial \omega \setminus {\overline{\gamma }},\nonumber \\ \partial _z U(x')= & {} \Lambda U(x'),\ x'\in \gamma , \end{aligned}$$

(1.7)

where $\Delta '$ is the Laplacian in the coordinates $x'=(x_2,x_3)$.

The spectrum of the problem ${\mathcal {P}}^0$ is absolutely continuous but, for $\epsilon >0$, the spectrum $\sigma ^\epsilon =\overline{{\mathbb {R}}_{+}}$ of problem ${\mathcal {P}}^\epsilon$ may contain embedded eigenvalues forming the point spectrum $\sigma ^\epsilon _{p}$. Our main goal is to find out a domain $\omega$ and a curve $\Upsilon ^\epsilon$, that is, the curvature $\kappa ^\epsilon$ in (1.1), such that $\sigma ^\epsilon _{p}$ includes at least one eigenvalue

$$\begin{aligned} \lambda ^\epsilon =\Lambda _1-\epsilon ^ 2\mu ^2. \end{aligned}$$

(1.8)

Being embedded in the continuous spectrum, this eigenvalue possesses the intrinsic instability, i.e. a small “wrong” perturbation of the appropriate curvature removes the eigenvalue out of the spectrum and turns it into a point of complex resonance [1, 15]. In other words, our choice of the desired channel must be very precise and requires for “fine-tuning” of several parameters in the curvature (1.1), although many geometrical characteristics of $\omega$ and $\Upsilon ^\epsilon$ can be fixed rather arbitrarily so that we are able construct infinitely many channels with the desired property.

1.3 Description of the paper

In the literature, there are many examples of local perturbations of three-dimensional channels which support trapped surface water waves and the corresponding spectral parameter is an embedded eigenvalue, see, for example, [2, 4, 6, 9, 14,15,16, 18, 20, 22, 23, 33,34,35,36,37]. The original paper [6] introduces an elegant trick which requires the mirror symmetry about the mid-plane $\{x:\ x_2=0\}$ of a channel $\Xi \subset {\mathbb {R}}^3$. By imposing the Dirichlet condition on the surface $\{x\in \Xi :\ x_2=0\}$, one creates an artificially positive cut-off value $\lambda ^{+}_\dagger$. The obtained mixed spectral problem in the half-channel $\Xi ^{+}=\{x\in \Xi : x_2>0\}$ may have the discrete spectrum $\sigma ^{+}_{d}\subset (0,\lambda ^{+}_\dagger )$, while the odd extensions of the corresponding eigenfunctions in $x_2$-variable become an eigenfunction of the original problem in the original waveguide $\Xi$. Hence, $\sigma ^{+}_{d}$ is a part of the point spectrum in $\Xi$. However, under various assumptions on the geometry, variational and asymptotic methods have been applied to detect eigenvalues in $\sigma ^{+}_{d}$, see [2, 6, 23, 39] and others.

The above-mentioned approach does not apply here since the channel $\Omega ^\epsilon$, surely, does not possess the mirror symmetry. Instead, we employ an asymptotic method based on a sufficient condition for the existence of trapped modes involving an artificial object, the augmented scattering matrix $S^\epsilon$, see [11, 24, 26] and Theorem 1. The necessary definitions are provided in Sect. 2, while the asymptotics of $S^\epsilon$ is constructed in Sect. 5. In Sect. 4, we implement the fine-tuning procedure and introduce the parametrization

$$\begin{aligned} \kappa ^0({\varsigma })= & {} \kappa ^0_0({\varsigma })+\tau _{+}\kappa ^0_{+}({\varsigma })+ \tau _{-}\kappa ^0_{-}({\varsigma })\end{aligned}$$

(1.9)

$$\begin{aligned} \mu= & {} \mu _0+\tau _0 \end{aligned}$$

(1.10)

of the quantities in (1.1) and (1.8). Our asymptotic analysis allows us to reduce the sufficient condition to the abstract fixed point equation

$$\begin{aligned} \tau =T^\epsilon (\tau ). \end{aligned}$$

(1.11)

We will show that the operator $T^\epsilon$ is a contraction in the ball

$$\begin{aligned} {\textbf{B}}_\rho =\{\tau =(\tau _0,\tau _{+},\tau _{-})\in {\mathbb {R}}^3:\ |\tau |\le \rho \} \end{aligned}$$

for small positive $\epsilon$ and $\rho$. Thus, the Banach fixed point theorem delivers a unique solution for (1.11) which additionally satisfies the estimate

$$\begin{aligned} |\tau |\le c\epsilon . \end{aligned}$$

(1.12)

In this way, we obtain the curved channel with a constant cross section which has a trapped mode and the embedded eigenvalue

$$\begin{aligned} \lambda ^\epsilon (\tau )=\Lambda _1-\epsilon ^2(\mu _0+\tau _0)^2, \end{aligned}$$

cf. (1.8) and (1.10). This result will be formulated in Theorem 2.

It is worth to mention that the components $\kappa ^0_0$ and $\kappa ^0_\pm$ in (1.9) must satisfy the normalization and orthogonality conditions (3.18), (4.5), (4.7) and (4.8) under the appropriate choice of the cross section $\omega$, see (3.21), (3.19) and (3.14), (3.17), while afterwards the coefficients $\tau _{\pm }$ are defined uniquely from (1.11). These conditions can be verified with quite arbitrary ingredients in the definition of the channel $\Omega ^\epsilon$, so that infinitely many channels supporting trapped surface waves are constructed by our procedure.

1.4 Motivation

In the frequently quoted paper [5], it was proved that the Dirichlet problem

$$\begin{aligned} -\Delta u^1(x)= & {} \lambda ^ 1 u^1(x),\ x\in \Omega ^1,\\ u^1(x)= & {} 0,\ x\in \Omega ^1, \end{aligned}$$

which models the planar quantum waveguide of the unit width and a non-trivial curvature $\kappa ^1\in C^\infty _0({\mathbb {R}})$ of the mid-line, has an eigenvalue $\lambda ^1$ below the continuous spectrum $\sigma ^1_{c}=[\pi ^2,+\infty )$. Notice that here we put the superscript $\epsilon =1$ in order to point out that this result holds true without the smallness assumption (1.1) on the curvature. The variational approach, proposed in [5], has been applied in many works, cf. [22, 25, 31, 32], in particular, for three-dimensional waveguides which in the case of a non-trivial twisting of the waveguide axis may have an empty discrete spectrum; we refer to the monograph [7] for the detailed survey about spectra in quantum waveguides.

With the reasons mentioned above, the fine-tuning procedure, as in [24], becomes quite limited. Hence, to construct a trapped mode, we have to accept two restrictive conditions, see (3.21) and (3.17) in Sect. 3.3. The latter condition forbids a cross section which is symmetric about the $x_3$-axis. For this reason, we are not able to prove the existence of a trapped mode in a curved channel with a rectangular cross section, i.e. with vertical walls and horizontal bottom. Notice that in this case, by factoring out the dependence on $z=x_3$ variable, the problem reduces to the Neumann problem for the Helmholtz operator on a curved strip of width 2, that is, on the free surface $\Gamma ^\epsilon$. In this way, being an apparent modification of the quantum waveguide in [5], an example of a curved two-dimensional acoustic waveguide of constant width supporting a trapped mode is not known yet. In [28], several examples of curved acoustic waveguides in dimension $d\ge 3$ with constant cross section have been given that support a trapped mode.

We also point out that the criteria for the existence of trapped modes in [21] and [29] are adopted mainly for computational simulations. Finally, we emphasize that Sect. 5.2 gives examples of asymmetric cross sections $\omega$ such that both the introduced conditions (3.17) and (3.21) are satisfied.

2 Waves and scattering matrices

2.1 Asymptotic analysis of the model problem

In the sequel, we assume that the first positive eigenvalue in the sequence (1.6) is simple, that is

$$\begin{aligned} \Lambda _1<\Lambda _2. \end{aligned}$$

(2.1)

This assumption is supported by our examples in Sect. 5.2.

By the max–min principle, cf. [3, Thm. 10.2.2] or [40, Ch. 22], the non-positive part of the $M$-spectrum of the problem

$$\begin{aligned} -\Delta ' V(x')&=MV(x'),\,x'\in \omega ,\nonumber \\ \partial _\nu V(x')&=0,\,x'\in \partial \omega \setminus {\overline{\gamma }},\nonumber \\ \partial _z V(x')&=\Lambda _1V(x'),\,x'\in \gamma , \end{aligned}$$

(2.2)

consists of two eigenvalues $M_0<0$ and $M_1=0$. The corresponding eigenfunctions are denoted by $V_0$ and $V_1$ normalized in the Lebesgue space $L^2(\omega )$:

$$\begin{aligned} \Vert V_0;L^2(\omega )\Vert =\Vert V_1;L^2(\omega )\Vert =1. \end{aligned}$$

Notice that $V_1=U_1$.

Let us construct the asymptotics of the eigenpairs $\{M^\epsilon ,V^\epsilon \}$ of the perturbed problem with the parameter $\lambda ^\epsilon$ in (1.8)

$$\begin{aligned} -\Delta ' V^\epsilon (x')&=M^\epsilon V^\epsilon (x'),\,x'\in \omega ,\nonumber \\ \partial _\nu V^\epsilon (x')&=0,\,x'\in \partial \omega \setminus {\overline{\gamma }},\nonumber \\ \partial _z V^\epsilon (x')&=\lambda ^\epsilon V^\epsilon (x'),\,x'\in \gamma , \end{aligned}$$

(2.3)

The correction terms in the asymptotic ansätze with $q=0,1$

$$\begin{aligned} M^\epsilon _q&=M_q+\epsilon ^2 M^\sharp _q+{\widetilde{M}}^\epsilon _q,\nonumber \\ V^\epsilon _q&=V_q+\epsilon ^2 V^\sharp _q+{\widetilde{V}}^\epsilon _q \end{aligned}$$

(2.4)

must be deduced from the problem

$$\begin{aligned} -\Delta 'V^\sharp _q(x')-M_q V^\sharp _q(x')&=M^\sharp _q(x')V_q(x')=:F_q(x'),\ x'\in \omega ,\nonumber \\ \partial _\nu V^\sharp _q(x')&=0,\ x'\in \partial \omega \setminus \gamma ,\nonumber \\ \partial _z V^\sharp _q(x')-\Lambda _1 V^\sharp _q(x')&=-\mu ^2 V_q(x')=:G_q(x'),\ x'\in \gamma . \end{aligned}$$

(2.5)

Notice that this problem is obtained directly by inserting (2.4) into (2.3) and extracting terms of order $\epsilon ^2$.

Since both eigenvalues $M_0$ and $M_1$ are simple, the only compatibility condition in the problem (2.5) reads as

$$\begin{aligned} (F_q,V_q)_\omega +(G_q,V_q)_\gamma =0, \end{aligned}$$

where $(\cdot ,\cdot )_\omega$ is the natural scalar product in the Lebesgue space $L^2(\omega )$. Thus, in view of the normalization of the eigenfunctions $V_q$, we obtain

$$\begin{aligned} M^\sharp _q=\mu ^ 2\Vert V_q;L^2(\gamma )\Vert ^2>0. \end{aligned}$$

(2.6)

Now, the problem (2.5) has a solution which becomes unique under the orthogonality condition

$$\begin{aligned} (V^\sharp _q,V_q)_\omega =0. \end{aligned}$$

Since (2.3) is a spectral problem with a regular perturbation of coefficients in differential operators, the error estimates

$$\begin{aligned} |{\widetilde{M}}^\epsilon _q|+\Vert {\widetilde{V}}^\epsilon _q;H^1(\omega )\Vert \le c_q \epsilon ^4\ \text {for } \epsilon \in (0,\epsilon _q],\ q=0,1, \end{aligned}$$

are supported by the general results of the perturbation theory of linear operators, see, for example, [12, Ch. 8]. Here, $c_q$ and $\epsilon _q$ are some positive numbers and $H^1(\omega )$ is the Sobolev space.

2.2 Waves

In the straight channel, there are two oscillatory and two exponential waves

$$\begin{aligned} w^\epsilon _{0\pm }(x)&=a^\epsilon _0 e^{\pm i m^\epsilon _0x_1} V^\epsilon _0(x'), \end{aligned}$$

(2.7)

$$\begin{aligned} v^\epsilon _{1\pm }(x)&=a^\epsilon _1 e^{\pm m^\epsilon _1x_1} V^\epsilon _1(x'), \end{aligned}$$

(2.8)

where

$$\begin{aligned} \begin{array}{ll} m^\epsilon _0=\sqrt{-M^\epsilon _0}=m_0+O(\epsilon ^2),&{}\ m_0=\sqrt{-M_0}>0,\\ m^\epsilon _1=\sqrt{M^\epsilon _1}=\epsilon (m_1+O(\epsilon ^2)),&{}\ m_1=\mu \Vert U_1;L^2(\gamma )\Vert >0 \end{array} \end{aligned}$$

(2.9)

and $\{M^\epsilon _q,V^\epsilon _q\}$ is the eigenpair of the problem (2.3) satisfying (2.4) and (2.6). Furthermore, $a^\epsilon _q$ is a normalization factor:

$$\begin{aligned} a^\epsilon _q&=(2m^\epsilon _q)^{-\frac{1}{2}} \Vert V^\epsilon _q;L^2(\omega )\Vert ^{-1},\ q=0,1, \end{aligned}$$

(2.10)

$$\begin{aligned} a^\epsilon _0&=a^0_0+O(\epsilon ^2),\ a^0_0=(2\sqrt{-M_0})^{-\frac{1}{2}}, \end{aligned}$$

(2.11)

$$\begin{aligned} a^\epsilon _1&=\epsilon ^{-\frac{1}{2}}a^0_1(1+O(\epsilon ^2)),\ a^0_1=2^{-\frac{1}{2}}\mu ^{-1} \Vert V_q;L^2(\gamma )\Vert ^{-1}. \end{aligned}$$

(2.12)

For the further use, we define the symplectic (sesquilinear and anti-Hermitian) form

$$\begin{aligned} Q(v,w)=\int \limits _{\omega } \Big (\overline{w(R,x')}\frac{\partial v}{\partial x_1}(R,x') - v(R,x')\overline{\frac{\partial w}{\partial x_1}(R,x')}\Big )\,dx'. \end{aligned}$$

The form comes from Green’s formula in problem ${\mathcal {P}}^0$. Therefore, it is independent on the parameter $R\in {\mathbb {R}}$ for the waves in (2.7), (2.8) and their linear combinations as well as for other solutions of the problem in the unit strip. A direct calculation demonstrates that, owing to the normalization coefficients (2.10)-(2.12), we have

$$\begin{aligned} \begin{array}{ll} Q(w^\epsilon _{0\pm },w^\epsilon _{0\pm })=\pm i,&{} Q(w^\epsilon _{0\pm },w^\epsilon _{0\mp })=0\\ Q(v^\epsilon _{1\pm },v^\epsilon _{1\pm })=0,&{} Q(v^\epsilon _{1\pm },v^\epsilon _{1\mp })=1. \end{array} \end{aligned}$$

(2.13)

Furthermore, since the exponents $im^\epsilon _0$ and $m^\epsilon _1$ in the waves (2.7) and (2.8) are different, we conclude by the independence property of $Q(\cdot ,\cdot )$ that

$$\begin{aligned} Q(w^\epsilon _{0\pm },v^\epsilon _{1\theta })= -\overline{Q(v^\epsilon _{1\theta },w^\epsilon _{0\pm })}=0,\ \theta =\pm . \end{aligned}$$

(2.14)

2.3 The scattering matrix

The oscillating waves (2.7) propagate along $\Omega ^0$ without any distortion but in the curved channel (1.1) they are scattered inside the middle part $\Omega ^\epsilon _0$, so that problem ${\mathcal {P}}^\epsilon$ with $\epsilon >0$ gets the following solutions:

$$\begin{aligned} \zeta ^\epsilon _{\pm }(x)= \chi _{\pm }({\varsigma })w^\epsilon _{0\mp }({\varsigma },n,z)+ \sum _{\theta =\pm } \chi _\theta ({\varsigma })s^\epsilon _{\theta \pm } w^\epsilon _{\theta }({\varsigma },n,z)+{\widetilde{\zeta }}^\epsilon _{\pm }(x). \end{aligned}$$

(2.15)

Here, the remainders ${\widetilde{\zeta }}^\epsilon _{\pm }(x)$ decay at rate $O(e^{-\mu ^\epsilon _1|{\varsigma }|})$ as $|{\varsigma }|\rightarrow \infty$ in $\Omega ^\epsilon _{\pm }$ and $\chi _{\pm }$ are smooth cut-off functions:

$$\begin{aligned} \chi _{\pm }({\varsigma })={\left\{ \begin{array}{ll} \begin{array}{l} 1\ \text {for } \pm {\varsigma }>2l,\\ 0\ \text {for }\pm {\varsigma }<l. \end{array} \end{array}\right. } \end{aligned}$$

The transmission and reflection coefficients $s^\epsilon _{\theta \,\vartheta }$ in (2.15) form the scattering matrix $s^\epsilon$. It is unitary ($(s^\epsilon )^*=(s^\epsilon )^{-1}$) and symmetric ($s^\epsilon _{+-}=s^\epsilon _{-+}$) due to the conditions (2.13) and the relation $w^\epsilon _{0-}=\overline{w^\epsilon _{0+}}$, see, for example, [24].

2.4 The augmented scattering matrix

Following [11], see also [24], we introduce the exponential wave packets

$$\begin{aligned} w^\epsilon _{1\pm }(x)=2^{-\frac{1}{2}}(v^\epsilon _{1+}(x)\mp i v^\epsilon _{1-}(x)) \end{aligned}$$

(2.16)

and readily observe that, according to (2.13) and (2.14), we have

$$\begin{aligned} Q(w^\epsilon _{j\pm },w^\epsilon _{k\pm })=\pm \delta _{j,k},\ Q(w^\epsilon _{j\pm },w^\epsilon _{k\mp })=0,\ j,k=0,1, \end{aligned}$$

(2.17)

where $\delta _{j,k}$ is the Kronecker symbol.

It is known, see, for example, [11, 24, 26], that the orthogonality and normalization conditions (2.17) assure the existence of the following solutions to the problem ${\mathcal {P}}^\epsilon$:

$$\begin{aligned} Z^\epsilon _{0\pm }(x)&=\chi _{\pm }({\varsigma })w^\epsilon _{0\mp }({\varsigma },n,z)+ \sum _{\theta =\pm } \chi _\theta ({\varsigma }) S^\epsilon _{\theta \pm }w^\epsilon _{0,\pm }({\varsigma },n,z)\nonumber \\&+ \chi _{+}({\varsigma })S^\epsilon _{1\pm } w^\epsilon _{1-}({\varsigma },n,z) +\chi _{-}({\varsigma })T^\epsilon _{1\pm } v^\epsilon _{1+}({\varsigma },n,z) +{\widetilde{Z}}^\epsilon _{0\pm }(x) \end{aligned}$$

(2.18)

$$\begin{aligned} Z^\epsilon _{1}(x)&=\chi _{+}({\varsigma })w^\epsilon _{1-}({\varsigma },n,z)+ \sum _{\theta =\pm } \chi _\theta ({\varsigma }) S^\epsilon _{\theta 1}w^\epsilon _{0,\pm }({\varsigma },n,z)\nonumber \\&+ \chi _{+}({\varsigma })S^\epsilon _{11} w^\epsilon _{1+}({\varsigma },n,z) +\chi _{-}({\varsigma })T^\epsilon _{11} v^\epsilon _{1+}({\varsigma },n,z) +{\widetilde{Z}}^\epsilon _{1}(x). \end{aligned}$$

(2.19)

Here, the remainders ${\widetilde{Z}}^\epsilon _{0\pm }(x)$ and ${\widetilde{Z}}^\epsilon _{1}(x)$ get the exponential decay $o(e^{-\delta |{\varsigma }|})$, while the exponents $\delta >0$ are defined by the eigenvalues (2.1) and can be fixed independently on $\epsilon \in (0,\epsilon _0],\ \epsilon _0>0$.

The coefficients in (2.18) and (2.19) form a $3\times 3$-matrix $S^ \epsilon$,

$$\begin{aligned} S^\epsilon =\begin{bmatrix}S^\epsilon _{\bullet \bullet }&{}S^\epsilon _{\bullet 1}\\ S^\epsilon _{1\bullet }&{}S^\epsilon _{11}\end{bmatrix},\ S^\epsilon _{\bullet \bullet }=\begin{bmatrix} S^\epsilon _{++}&{}S^\epsilon _{+-}\\ S^\epsilon _{-+}&{}S^\epsilon _{--} \end{bmatrix},\ S^\epsilon _{\bullet 1}= \begin{bmatrix} S^\epsilon _{+1}\\ S^\epsilon _{-1}, \end{bmatrix} \end{aligned}$$

which is called the augmented scattering matrix.

It should be mentioned that, following [26], we include the exponentially growing packets $w^\epsilon _{1\pm }$ in the outlet $\Omega ^\epsilon _{+}$ only, while the decompositions (2.18) and (2.19) contain the decaying wave $v^\epsilon _{1+}$, see (2.8) and (2.16). However, the proofs in [11, 24] allow us to derive that the matrix $S^\epsilon$ is unitary and symmetric due to the equalities (2.17) and $w^\epsilon _{1-}=\overline{w^\epsilon _{1+}}$.

2.5 The existence of a trapped mode

In contrast to the classical scattering matrix $s^\epsilon$ arising from the solutions (2.15) to the standard diffraction problem, the augmented scattering matrix $S^\epsilon$ is an artificial object, because (2.18) and (2.19) involve exponentially growing waves and lose physical sense. However, the very reason to introduce such a matrix can be explained by the following observation: in the case

$$\begin{aligned} S^\epsilon _{11}=-1 \end{aligned}$$

(2.20)

, the solution (2.19) takes the form

$$\begin{aligned} Z^\epsilon _1(x)=i\sqrt{2}\chi _{+}({\varsigma })v^\epsilon _{1-}({\varsigma },n,z)+ \chi _{-}({\varsigma }) T^\epsilon _{11} v^\epsilon _{1+}({\varsigma },n,z)+ {\widetilde{Z}}^\epsilon _{1}(x) \end{aligned}$$

(2.21)

and, therefore, becomes a trapped mode, since $v^\epsilon _{1\pm }$ decay in $\Omega ^\epsilon _{\mp }$, see (2.8). To derive (2.21), we have used the relations (2.16) and the evident formula

$$\begin{aligned} |S^\epsilon _{11}|=1\ \Leftrightarrow \ S^\epsilon _{\bullet 1}=0\in {\mathbb {C}}^2 \end{aligned}$$

(2.22)

supported by the unitary property of $S^\epsilon$.

In other words, (2.20) is a sufficient condition for the existence of a trapped mode. The next theorem assures that (2.20) also becomes necessary in our particular case and it will be proven at the end of Sect. 4.2.

Theorem 2.1

There exists a positive number ${\textbf{q}}$ such that, in the case

$$\begin{aligned} N(\kappa ^0)=\Vert \kappa ^0; C^2(-l,l)\Vert \le {\textbf{q}}, \end{aligned}$$

the problem ${\mathcal {P}}^\epsilon$ admits a trapped mode if and only if the relation (2.20) is satisfied.

3 Asymptotics of the augmented scattering matrix

3.1 Differential operators in curvilinear coordinates

Since the equation $\Psi (n,z)=0$ describing the surface $\partial \Omega ^\epsilon {\setminus }\Gamma ^\epsilon$ does not depend on the variable ${\varsigma }$ and the gradient operator takes the form

$$\begin{aligned} \nabla _{({\varsigma },n,z)}=(J^\epsilon ({\varsigma },n)^{-1}\partial _{{\varsigma }},\partial _n,\partial _z), \end{aligned}$$

the normal $\nu ({\varsigma }^0,n,z)$ to this surface coincides with the normal of the cross section $\{x\in \Omega ^ \epsilon :\ {\varsigma }={\varsigma }^0\}$. Here, $J^ \epsilon ({\varsigma },n)=1+n\kappa ^ \epsilon ({\varsigma })$ is the Jacobian. Furthermore, the Laplace operator in the curvilinear coordinates reads as

$$\begin{aligned} J^\epsilon ({\varsigma },n)^{-1}\partial _{{\varsigma }}J^\epsilon ({\varsigma },n)^{-1}\partial _{{\varsigma }}+ J^\epsilon ({\varsigma },n)^{-1}\partial _{n}J^\epsilon ({\varsigma },n)\partial _{n} + \partial ^ 2_z. \end{aligned}$$

(3.1)

Taking (1.1) into account and extracting from (3.1) the terms of order $\epsilon ^0$ and $\epsilon ^1$, we obtain

$$\begin{aligned} \Delta _x=\partial ^2_{{\varsigma }}+\partial ^ 2_n+\partial ^2_z+ \epsilon (\kappa ^0({\varsigma })\partial _n-n\kappa ^0({\varsigma })\partial ^2_{{\varsigma }}- n\partial _{{\varsigma }}\kappa ^0({\varsigma })\partial _{{\varsigma }})+\dots , \end{aligned}$$

(3.2)

where the ellipsis stands for higher-order terms, which are inessential in our formal asymptotic analysis.

3.2 Asymptotic ansätze

Let us examine the asymptotics of the solution (2.19) to problem ${\mathcal {P}}^\epsilon$ as $\epsilon \rightarrow \,+0$. The factor $\epsilon ^{-\frac{1}{2}}$ in the normalization coefficient $a^\epsilon _1$ and the relation $a^\epsilon _0=O(1)$, see (2.11) and (2.12), suggest the ansatz

$$\begin{aligned} Z^\epsilon _1(x)=\epsilon ^ {-\frac{1}{2}}Z^0_1(x)+\epsilon ^ {\frac{1}{2}}Z^\sharp _1({\varsigma },n,z)+ \dots \end{aligned}$$

(3.3)

for the solution and the ansätze

$$\begin{aligned} S^\epsilon _{11}&=S^0_{11}+\epsilon S^\sharp _{11}+\dots ,\ T^\epsilon _{11}=T^0_{11} + \epsilon T^\sharp _{11}+\dots , \end{aligned}$$

(3.4)

$$\begin{aligned} S^\epsilon _{\pm 1}&=\epsilon ^{\frac{1}{2}} S^0_{\pm 1} +\epsilon ^ {\frac{3}{2}}S^\sharp _{\pm 1}+\dots \end{aligned}$$

(3.5)

for its coefficients.

We insert formulae (1.8), (3.2) and (3.3) into the problem (1.3)-(1.5) and extract terms of order $\epsilon ^{-\frac{1}{2}}$ and $\epsilon ^\frac{1}{2}$. As a result, we obtain the following problems:

$$\begin{aligned} -\Delta _{({\varsigma },n,z)} Z^0_1({\varsigma },n,z)&=0,\ ({\varsigma },n,z)\in {\mathbb {R}}\times \omega ,\nonumber \\ \partial _{\nu ({\varsigma },n,z)} Z^0_1({\varsigma },n,z)&=0,\ ({\varsigma },n,z)\in {\mathbb {R}}\times (\partial \omega \setminus {\overline{\gamma }}),\nonumber \\ \partial _{z}Z^0_1({\varsigma },n,z)&=\Lambda _1 Z^0_1({\varsigma },n,z),\ ({\varsigma },n,z)\in {\mathbb {R}}\times \gamma , \end{aligned}$$

(3.6)

and

$$\begin{aligned} -\Delta _{({\varsigma },n,z)} Z^\sharp _1({\varsigma },n,z)&= \kappa ^0({\varsigma })\partial _n Z^0_1({\varsigma },n,z) + n\kappa ^0({\varsigma })\partial ^ 2_{{\varsigma }} Z^0_1({\varsigma },n,z) +\nonumber \\&+n\partial _{{\varsigma }}\kappa ^0({\varsigma })\partial _{{\varsigma }}Z^0_1({\varsigma },n,z),\ ({\varsigma },n,z)\in {\mathbb {R}}\times \omega ,\nonumber \\ \partial _{\nu (n,z)} Z^\sharp _1({\varsigma },n,z)&=0,\ ({\varsigma },n,z)\in {\mathbb {R}}\times (\partial \omega \setminus {\overline{\gamma }}),\nonumber \\ \partial _{z}Z^\sharp _1({\varsigma },n,z)&=\Lambda _1 Z^\sharp _1({\varsigma },n,z),\ ({\varsigma },n,z)\in {\mathbb {R}}\times \gamma . \end{aligned}$$

(3.7)

However, Eqs. (3.6) and (3.7) are not sufficient to determine the desired ingredients of the ansatz (3.3), and it is necessary to prescribe the behaviour of $Z^0_1$ and $Z^\sharp _1$ at infinity. To this end, we employ the method of matched asymptotic expansion, cf. [10, 38], in the interpretation of [24]. In this way, we regard (3.3) as the inner expansion, which is valid near the middle part $\Omega ^\epsilon _0$ of the channel. The outer expansions in its outlets $\Omega ^\epsilon _{\pm }$ are again obtained by inserting the ansätze (3.4), (3.5) into (2.18) and (2.19) but using the representations for the waves (2.7), (2.8)

$$\begin{aligned} w^\epsilon _{0\pm }({\varsigma },n,z)&= w^0_{0\pm }({\varsigma },n,z)+O(\epsilon ^2(1+|{\varsigma }|)),\\ v^\epsilon _{1\pm }({\varsigma },n,z)&= \epsilon ^{-\frac{1}{2}}a^0_1 \Big (U_1(n,z)\pm \epsilon m_1{\varsigma }U_1(n,z)\Big ) +O\Big (\epsilon ^\frac{3}{2} (1+{\varsigma }^2)\Big ) \end{aligned}$$

derived in Sect. 2 with $m_1$ given in (2.9). Thus, we have

$$\begin{aligned} Z^\epsilon _1(x)&= \frac{1}{\sqrt{\epsilon }}\frac{a^0_1}{\sqrt{2}} \Big (1+S^0_{11} +i(1-S^0_{11})\Big ) U_1(n,z) +\nonumber \\&\quad + \sqrt{\epsilon } \Big (\frac{a^0_1}{\sqrt{2}}(1+S^0_{11} -i(1-S^0_{11})) m_1{\varsigma }+ \frac{a^0_1}{\sqrt{2}} (1-i) S^\sharp _{11} \Big )U_1(n,z)\nonumber \\&\quad +\sqrt{\epsilon } S^0_{+1} w^0_1({\varsigma },n,z) +\dots ,\ {\varsigma }\rightarrow +\infty \text { in }\Omega ^\epsilon _{+}, \end{aligned}$$

(3.8)

$$\begin{aligned} Z^\epsilon _1(x)&= \frac{1}{\sqrt{\epsilon }}a^0_1 T^0_{11}U_1(n,z) + \sqrt{\epsilon } \Big (a^0_1 T^0_{11}m_1{\varsigma }+a^0_1 T^\sharp _{11} \Big )U_1(n,z)\nonumber \\&\quad +\sqrt{\epsilon } S^0_{-1} w^0_{0-}({\varsigma },n,z) +\dots ,\ {\varsigma }\rightarrow -\infty \text { in }\Omega ^\epsilon _{-}. \end{aligned}$$

(3.9)

3.3 Computation of the coefficients

Extracting from (3.8) and (3.9) the terms of order $\epsilon ^{-\frac{1}{2}}$ yields the conditions

$$\begin{aligned} Z^0_1({\varsigma },n,z)&=\frac{a^0_1}{\sqrt{2}} \Big (1+S^0_{11} +i(1-S^0_{11}) \Big )U_1(n,z)+\dots ,\ {\varsigma }\rightarrow +\infty \\ Z^0_1({\varsigma },n,z)&=a^0_1 T^0_{11} U_1(n,z),\ {\varsigma }\rightarrow -\infty \end{aligned}$$

in the problem (3.6). Hence, since $U_1(x')$ is the only bounded solution of problem ${\mathcal {P}}^0$ in $\Omega ^0$ with the threshold spectral parameter $\lambda ^0=\Lambda _1$, we conclude that

$$\begin{aligned} Z^0_1({\varsigma },n,z)&=\frac{a^0_1}{\sqrt{2}} \Big (1+S^0_{11}+i(1-S^0_{11}) \Big )U_1(n,z),\end{aligned}$$

(3.10)

$$\begin{aligned} \sqrt{2} T^0_{11}&= 1+S^0_{11} + i(1-S^0_{11}). \end{aligned}$$

(3.11)

Notice that the function (3.10) does not depend on ${\varsigma }$ and, therefore, two last terms on the right-hand side of the Poisson equation in (3.7) vanish.

Collecting the coefficients of $\sqrt{\epsilon }$ in (3.8) and (3.9) provides the following behaviour of the solution $Z^\sharp _1$ to the problem (3.7):

$$\begin{aligned} Z^\sharp _1&= \frac{a^0_1}{\sqrt{2}} \Big ((1+S^0_{11}-i(1-S^0_{11}))m_1{\varsigma }+ (1-i)S^\sharp _{11}\Big ) U_1(n,z)+\nonumber \\&\quad +S^0_{+1}w^0_{0+}({\varsigma },n,z)+\dots ,\quad {\varsigma }\rightarrow \, +\infty ,\nonumber \\ Z^\sharp _1&=a^0_1\Big (T^0_{11} m_1{\varsigma }+T^\sharp _{11}\Big ) U_1(n,z) + S^0_{-1} w^0_{0-}({\varsigma },n,z)+\dots ,\quad {\varsigma }\rightarrow \,-\infty . \end{aligned}$$

(3.12)

Since the linear growth of this solution is allowed in (3.12) when ${\varsigma }\rightarrow \pm \infty$, the problem (3.7), (3.12) with compactly supported right-hand side $F^\sharp _{0}({\varsigma },n,z)=\kappa ^0({\varsigma })\partial _n Z^0_1(n,z)$ always has a solution which is defined up to a function $(c_0+c_1{\varsigma })\times U_1(n,z)$ linear in ${\varsigma }$-variable. Hence, some of the coefficients in (3.12) cannot be determined. However, we can derive certain relations between them. To this end, we insert $Z^\sharp _1$ and $U_1$ into Green’s formula in the truncated straight cylinder $\Omega _R=(-R,R)\times \omega$ and obtain in the limit $R\rightarrow +\infty$

$$\begin{aligned} \int \limits _{\Omega }&U_1(n,z)\kappa ^0({\varsigma })\partial _nZ^0_1({\varsigma },n,z)\,d{\varsigma }dn dz\nonumber \\ {}&=-\lim _{R\rightarrow \infty } \int \limits _{\Omega _R} U_1(n,z) \Delta _{({\varsigma },n,z)}Z^0_1({\varsigma },n,z)\,d{\varsigma }dn dz\nonumber \\&=-\lim _{R\rightarrow \infty } \sum _{\pm }\pm \int \limits _{\omega } U_1(n,z) \partial _{{\varsigma }} Z^0_1(\pm R,n,z)\,dndz\nonumber \\&= \frac{a^0_1}{\sqrt{2}}(1+S^0_{11}+i(1-S^0_{11}))m_1 - a^0_1 T^0_{11} m_1. \end{aligned}$$

(3.13)

In the computation, we have used the formulae (2.7) and (3.12). Moreover, according to (3.10), the left-hand side of (3.13) is equal to

$$\begin{aligned} \frac{a^0_1}{\sqrt{2}} (1+S^0_{11}+i(1-S^0_{11}))K_1 J_1(\kappa ^0), \end{aligned}$$

where

$$\begin{aligned} J_1(\kappa ^0)=\int \limits _{-l}^{l}\kappa ^0({\varsigma })\,d{\varsigma },\quad K_1=\int \limits _{\omega } U_1(n,z)\partial _n U_1(n,z)\,dndz. \end{aligned}$$

(3.14)

Hence, applying (3.11), we conclude from (3.13) the relation

$$\begin{aligned} S^0_{11}=\frac{K_1J_1(\kappa ^0)+(1+i)m_1}{iK_1J_1(\kappa ^0)+(1+i)m_1}. \end{aligned}$$

(3.15)

Notice that the equality $S^0_{11}=-1$ occurs at the point

$$\begin{aligned} \mu _0=-\frac{1}{2} \Vert U_1;L^2(\gamma )\Vert ^{-1} K_1 J_1(\kappa ^0). \end{aligned}$$

(3.16)

To assure $\mu _0>0$, we must assume that

$$\begin{aligned} K_1\ne 0 \end{aligned}$$

(3.17)

and

$$\begin{aligned} K_1 J_1(\kappa ^0)<0. \end{aligned}$$

(3.18)

For the calculation of $S^0_{\pm 1}$, we apply Green’s formula to the functions $Z^\sharp _1$ and $w^0_{\pm }$ as follows:

$$\begin{aligned} \frac{a^0_1}{\sqrt{2}}(1+S^0_{11}&+i(1-S^0_{11}))K_0 J_{\pm }(\kappa ^0)\\&=-\lim _{R\rightarrow \infty } \int \limits _{\Omega _R} \overline{w^0_{0\pm }({\varsigma },n,z)} \Delta _{({\varsigma },n,z)} Z^\sharp _1({\varsigma },n,z)\, d{\varsigma }dndz\\&=-\lim _{R\rightarrow \infty } \Big ( Q(S^0_{+1} w^0_{0+},w^0_{0+}) -Q(S^0_{-1}w^0_{0-},w^0_{0\pm })\Big )=-i S^0_{\pm 1}, \end{aligned}$$

where

$$\begin{aligned} K_0&=\int \limits _{\omega }V_0(n,z)\partial _n U_1(n,z)\,dndz,\end{aligned}$$

(3.19)

$$\begin{aligned} J_{\pm }(\kappa ^0)&=a^0_0\int \limits _{-l}^{l} e^{\mp i m_0{\varsigma }}\kappa ^0({\varsigma })d{\varsigma }. \end{aligned}$$

(3.20)

In the rest of the paper, we will need the assumption

$$\begin{aligned} K_0\ne 0. \end{aligned}$$

(3.21)

Finally, based on (3.15), we conclude that

$$\begin{aligned} S^0_{\pm 1}= -(1-i)\sqrt{2}a^0_1 m_1 \frac{(1-i)m_1-i K_1J_1(\kappa ^0)}{m_1^2+(K_1J_1(\kappa ^0)+m_1)^2}K_0J_{\pm }(\kappa ^0). \end{aligned}$$

(3.22)

In Appendix A, we will verify the following estimates for the remainders in the asymptotic expansions (3.4) and (3.5):

$$\begin{aligned} |S^\epsilon _{11}-S^0_{11}|&\le c_0\epsilon ,\quad |T^\epsilon _{11}-T^0_{11}|\le c_0\epsilon \nonumber \\ |S^\epsilon _{\pm 1}-\sqrt{\epsilon }S^0_{\pm 1}|&\le c_0 \epsilon ^{\frac{3}{2}}\ \text { for } \epsilon \in (0,\epsilon _0], \end{aligned}$$

(3.23)

where $c_0$ and $\epsilon _0$ are positive constants depending on the cross section $\omega$ and $C^2$-norm of the rescaled curvature:

$$\begin{aligned} N(\kappa ^0)=\Vert \kappa ^0; C^2(-l,l)\Vert . \end{aligned}$$

Analysing the solutions (2.18) in a similar manner yields the asymptotic formulae

$$\begin{aligned}&S^\epsilon _{\bullet \bullet }={\mathbb {J}}+ {\widetilde{S}}^\epsilon _{\bullet \bullet },\quad {\mathbb {J}}=\begin{bmatrix} 0&{}1\\ 1&{}0 \end{bmatrix}\end{aligned}$$

(3.24)

$$\begin{aligned}&\Vert {\widetilde{S}}^\epsilon _{\bullet \bullet };{\mathbb {C}}^{2\times 2}\Vert \le c_0\epsilon \end{aligned}$$

(3.25)

for the upper left corner of the augmented scattering matrix. Notice that the appearance of the involution ${\mathbb {J}}$ in (3.24) has an evident reason: the waves $w^0_{0\pm }$ pass the straight channel without any distortion, so that the reflection and transition coefficients are equal to $0$ and $1$, respectively.

We postpone the derivation of the estimates (3.23) and (3.25) to Appendix. The procedure is quite standard and straightforward based on the technique of weighted spaces with detached asymptotics.

4 Identifying a trapped mode

4.1 Preparing for the fine-tuning

We accept the representation (1.9) for the rescaled curvature $\kappa ^0$ in (1.1) and (1.10) for the quantity $\mu$ in (1.8). The small parameters $\tau \in {\textbf{B}}_\rho$ will be fixed, cf. (1.12), and the functions $\kappa ^0_0,\ \kappa ^0_{\pm }$ are assumed to be smooth and supported in the closed interval $[-l,l]$. Thus, the asymptotic formulae (2.21), (3.5) and (3.24) remain valid as well as the estimates (3.25). In the sequel, we refer to these formulae when indicating the dependence on $\tau$ in $S^\epsilon (\tau )$ and $\kappa ^0({\varsigma };\tau ),\ J(\kappa ^0;\tau )$.

Using (1.10), (2.9) and (3.15) gives

$$\begin{aligned} m_1(\tau )&=-\frac{1}{2} K_1J_1(\kappa ^0;\tau )+\Vert U_1;L^2(\gamma )\Vert ^2\tau _0,\end{aligned}$$

(4.1)

$$\begin{aligned} S^0_{11}(\tau )&=\frac{K_1J_1(\kappa ^0;\tau )+2i \Vert U_1;L^2(\gamma )\Vert \tau _0}{-K_1J_1(\kappa ^0;\tau )+2i \Vert U_1;L^2(\gamma )\Vert \tau _0}\nonumber \\&= -1 - 4i\tau _0 \frac{\Vert U_1;L^2(\gamma )\Vert }{K_1J_1(\kappa ^0;\tau )} +O(\tau ^2_0) =: -1-4 i \tau _0 b_1(\tau )+O(\tau ^2_0). \end{aligned}$$

(4.2)

Observing that

$$\begin{aligned} \text {Re}\ S^0_{11}(\tau )=-1+O(\tau ^2_0),\quad \text {Im}\ S^0_{11}(\tau )=-4 i \tau _0 b_1(\tau )+O(\tau ^2_0), \end{aligned}$$

we see that our one-term asymptotic formula (4.2) is not sufficient to obtain $\text {Re}\ S^\epsilon _{11}(\tau )=-1$. For this reason, it is not possible to apply the sufficient condition (2.20) directly.

4.2 Reformulation of the sufficient condition

In view of (2.22), it follows from (2.20) that

$$\begin{aligned} \text {Im }S^\epsilon _{11}(\tau )&=0 \end{aligned}$$

(4.3)

$$\begin{aligned} \text {Re }(e^{i\psi }S^\epsilon _{\pm 1}(\tau ))&=0 \end{aligned}$$

(4.4)

with any phase $\psi \in [0,2\pi )$. To derive (2.20) from (4.3) and (4.4), we use the asymptotic formulae. Indeed, recalling that $S^\epsilon (\tau )$ is unitary and symmetric, we have

$$\begin{aligned} 0&=S^\epsilon _{\bullet \bullet }(\tau ) \Big (e^{i\psi } S^\epsilon _{1\bullet }(\tau ) \Big )^*+S^\epsilon _{\bullet 1}(\tau ) (\overline{e^{i\psi }S^\epsilon _{11}(\tau )})\\&=S^\epsilon _{\bullet \bullet }(\tau )(\overline{e^{i\psi }S^\epsilon _{\bullet 1}(\tau )}) +e^{-i\psi }\overline{S^\epsilon _{11}(\tau )} S^\epsilon _{\bullet 1}(\tau )\\&=-e^{i\psi }(S^\epsilon _{\bullet \bullet }(\tau ) S^\epsilon _{\bullet 1}(\tau ) +e^{-i\psi } \overline{S^\epsilon _{11}(\tau )} S^\epsilon _{\bullet 1}(\tau ) =-e^{i\psi } T^\epsilon _{\bullet \bullet }(\tau ) S^\epsilon _{\bullet 1}(\tau ), \end{aligned}$$

where

$$\begin{aligned} T^\epsilon _{\bullet \bullet }(\tau )= S^\epsilon _{\bullet \bullet }(\tau ) -e^{-2i\psi }{\mathbb {I}}_2 \overline{S^\epsilon _{11}(\tau )} ={\mathbb {J}}+e^{-2i\psi }{\mathbb {I}}_2+O(\epsilon +|\tau |) \end{aligned}$$

and ${\mathbb {I}}_2$ is the $2\times 2$ unit matrix. If $-e^{-2i\psi }$ is not an eigenvalue of the matrix ${\mathbb {J}}$, i.e. $\psi \ne 0,\pi$, the matrix $T^\epsilon _{\bullet \bullet }(\tau )$ is non-singular for small $\epsilon$ and $\tau$. Hence, one obtains

$$\begin{aligned} S^\epsilon _{\bullet 1}(\tau )=0,\ |S^\epsilon _{11}(\tau )|=1. \end{aligned}$$

Then, the formula (4.3) implies that $S^\epsilon _{11}(\tau )=\pm 1$. Finally, the condition (2.20) is met, since $S^1_{11}(\tau )=1$ is impossible due to (4.2) for sufficiently small $\epsilon$ and $\tau$. In the sequel, we choose $\psi =\frac{\pi }{4}$, that is, $e^{i\psi }=2^{-1/2}(1+i)$.

For simplicity, we assume, in addition to (3.18), that

$$\begin{aligned} J_1(\kappa ^0_{\pm })=0, \end{aligned}$$

(4.5)

i.e. the functions $\kappa ^0_{\pm }$ are of mean value zero. Hence, $J_1(\kappa ^ 0;\tau )=J_1(\kappa ^0_0)$ is independent of $\tau$. Setting

$$\begin{aligned} {\widetilde{S}}^\epsilon _{11}(\tau )= S^\epsilon _{11}(\tau )-S^0_{11}(\tau ),\ |{\widetilde{S}}^\epsilon _{11}(\tau )|\le c_0\epsilon \end{aligned}$$

and using (4.2), we may rewrite (4.3) as a transcendental equation

$$\begin{aligned} \tau _0=-\frac{K_1^2 J_1(\kappa ^0_0)^2+\Vert U_1;L^2(\gamma )\Vert ^4\tau _0^2}{4 \Vert U_1;L^2(\gamma )\Vert ^2 K_1 J_1(\kappa ^0_0)} \text { Im }{\widetilde{S}}^\epsilon _{11}(\tau ). \end{aligned}$$

(4.6)

Notice that the denominator does not vanish due to (3.18), (1.9) and (4.5).

Now, we fix $\kappa ^0_0$ and $\kappa ^0_\pm$ such that

$$\begin{aligned} \text {Re}\ \Big ((1+i)J_\pm (\kappa ^0_0)\Big )&=0, \end{aligned}$$

(4.7)

$$\begin{aligned} \text {Re}\ \Big ((1+i)J_\pm (\kappa ^0_\theta )\Big )&=\delta _{\pm , \theta },\ \theta =\pm , \end{aligned}$$

(4.8)

where the functionals $J_\pm$ are given by (3.20). Notice that kernels of the integral operators in (4.8) are

$$\begin{aligned} \cos (m_0{\varsigma })\pm i\sin (m_0{\varsigma }). \end{aligned}$$

They are linearly independent, and hence, the requirements of (4.8) can be satisfied.

Similarly, we set

$$\begin{aligned} {\widetilde{S}}^\epsilon _{\pm 1}(\tau )&= \epsilon ^{-\frac{1}{2}} S^\epsilon _{\pm 1}(\tau ) -S^0_{\pm 1}(\tau ),\ |{\widetilde{S}}^\epsilon _{\pm 1}(\tau )|\le c_0\epsilon . \end{aligned}$$

Now using (4.1) and (3.22), we rewrite the condition (4.4) as follows:

$$\begin{aligned} 0&=\text {Re}\Big ((1+i){\widetilde{S}}^\epsilon _{\pm 1}(\tau ) \Big ) \nonumber \\&+ \sqrt{2} a^0_1 K_1J(\kappa ^0_0) \frac{K_1J(\kappa ^0_0)-2 \Vert U_1;L^2\Vert ^2\tau _0}{ K_1^2J(\kappa ^0_0)^2 +4 \Vert U_1;L^2\Vert ^4\tau _0^2} K_0\text {Re}\Big ((1+i)J_\pm (\kappa ^0;\tau )\Big ) \nonumber \\&- 2(1-i)\tau _0 \frac{\Vert U_1;L^2(\gamma )\Vert ^2}{ K_1J_1(\kappa ^0_0)}J_\pm (\kappa ^0;\tau ). \end{aligned}$$

(4.9)

According to (4.7) and (4.8) $\text {Re}\Big ((1+i)J_\pm (\kappa ^0;\tau ) \Big )=\tau _\pm$. Then, we can complete the system (1.11) by augmenting (4.6) with two scalar equations derived from (4.9):

$$\begin{aligned} \tau _\pm&= 2\tau _0 \frac{\Vert U_1;L^2(\gamma )\Vert ^2}{ K_1J_1(\kappa ^0_0)} \text {Re}\Big ((1-i)(\tau _{+}J_\pm (\kappa ^0_{+}) +\tau _{-}J_{\pm }(\kappa ^0_{-}))\Big )\nonumber \\&- \frac{K_1^2J(\kappa ^0_0)^2 +4 \Vert U_1;L^2\Vert ^4\tau _0^2}{ K_1J(\kappa ^0_0) -2 \Vert U_1;L^2\Vert \tau _0} \frac{\text {Re}((1+i){\widetilde{S}}^\epsilon _{\pm 1}(\tau ))}{\sqrt{2}a^0_1K_0K_1 J_1(\kappa ^0)}. \end{aligned}$$

(4.10)

Since the coordinate change $x\mapsto ({\varsigma },n,z)$ brings small perturbations into differential operators of the water wave problem in $\Omega ^0$, which smoothly depend on the parameters $\tau =(\tau _o,\tau _{+},\tau _{-})$, the solution $Z^\epsilon _1$ as well as coefficients in its representation (3.8) has a similar dependence on $\tau$. (For our purpose, Lipschitz continuity would be enough.) Observing also that the first term on the right-hand side of (4.10) involves the factors $\tau _0,\ \tau _\pm$, we conclude that the operator ${\mathcal {T}}^\epsilon$ in the equation (1.11), which is a short form of (4.6) and (4.10), satisfies the inequalities

$$\begin{aligned}&|{\mathcal {T}}^\epsilon (\tau )|\le c(\epsilon +|\tau |^2),\\&|{\mathcal {T}}^\epsilon (\tau )-{\mathcal {T}}^\epsilon (\tau ')|\le c (\epsilon +|\tau |)|\tau -\tau '|\ \forall \ \tau ,\tau '\in {\textbf{B}}_{\rho }. \end{aligned}$$

Hence, it is a contraction in the ball ${\textbf{B}}_\rho$ provided the radius $\rho >0$ is small enough. Thus, the Banach fixed point theorem guarantees that there are positive $\epsilon$ and $\rho$, $\rho _0$ such that the equation (1.11) has a unique solution $\tau$ in the ball ${\textbf{B}}_{\epsilon \rho _0}$ and the estimate (1.12) is valid.

Recall that the equation (1.11) is equivalent to the relations (4.3) and (4.4) (and also to the sufficient condition (2.20) for the existence of a trapped mode). Therefore, formulae (1.1), (1.8), (1.9) and (1.10) determine the curved channel which supports a trapped surface wave and the eigenvalue of the problem (1.3)-(1.5) embedded in its continuous spectrum.

4.3 The main result

Theorem 4.1

Let the assumptions (2.1), (3.17), (3.18) and (3.21) be satisfied, and let the curvature $\kappa ^\epsilon$ of the mid-curve $\Upsilon ^\epsilon$ of the channel (1.2) with the constant cross-section $\omega$ take the form $\kappa ^0({\varsigma })=\kappa ^0_0({\varsigma })+\tau _{+}\kappa ^0_{+}({\varsigma })+ \tau _{-}\kappa ^0_{-}({\varsigma })$, where the functions $\kappa ^0_{\theta },\ \theta \in \{\pm ,0\},$ fulfil the condition (1.1) and the orthogonality and normalization conditions (4.7) and (4.8).

Then, there exists $\epsilon _0$ such that for $\epsilon \in (0,\epsilon _0]$ the problem (1.3)-(1.5) in $\Omega ^\epsilon$ has an embedded eigenvalue (1.13), where $\Lambda _1$ is the first positive threshold value in (1.6) and $\mu _0$ is given in (3.16). The parameters $\tau _0$ in (1.13) and $\tau _{\pm }$ above solve the fixed point equation (1.11) and meet the estimate (1.12).

Since Theorem 4.1 makes the relation (2.20) a criterion for the existence of trapped modes, for $\epsilon \in (0,\epsilon _0)$, the interval

$$\begin{aligned} (\Lambda _1-c_0\epsilon ^2,\Lambda _1) \end{aligned}$$

(4.11)

may contain at most one eigenvalue of problem ${\mathcal {P}}^\epsilon$, where $\epsilon _0$ and $c_0$ are some positive numbers. The asymptotic technique applied in this paper does not allow us to control the point spectrum outside the interval (4.11).

5 Final remarks

5.1 Straight walls and bottom

Let the cross section be a rectangle

$$\begin{aligned} \omega =\{x':\ |x_2|<1,\ x_3\in (-d,0)\},\ d>0. \end{aligned}$$

In this case, the eigenvalues for the Steklov problem (1.7) can be computed explicitly by the separation of variables:

$$\begin{aligned} \Lambda _k&=\frac{\pi k}{2}\tanh (\frac{\pi k d}{2}),\ k=0,1,2,\dots \nonumber \\ U_k(x_2,x_3)&= \cos (\pi k(x_2+1)/2)\cosh (\pi k(x_3+d)/2). \end{aligned}$$

(5.1)

Notice that all the eigenvalues in (5.1) are simple, fulfilling our assumption (2.1). Particularly, $\Lambda _0=0$ and

$$\begin{aligned} \Lambda _1=\frac{\pi }{2} \tanh (\frac{\pi d}{2}) \end{aligned}$$

(5.2)

with the eigenfunctions $U_0(x')=1$ and

$$\begin{aligned} V_1(x')=U_1(x')= A_1\cosh \big (\frac{\pi (x_3+d)}{2}\big ) \cos \big (\frac{\pi ( x_2+1)}{2}\big ), \end{aligned}$$

(5.3)

where $A_1$ is the normalization constant. The negative eigenvalue $M_0=-m_0^2$ of the problem (2.2) is defined by the root $m_0\in (0,\frac{\pi }{2d})$ of the transcendental equation

$$\begin{aligned} \Lambda _1=m_0\tan (m_0 d) \end{aligned}$$

, and the corresponding eigenfunction is

$$\begin{aligned} V_0(x')=\cos (\mu _0(x_3+d)). \end{aligned}$$

Then, by direct integration, we have

$$\begin{aligned} \int \limits _{\omega }V_0(x_2,x_3)\partial _{x_2} V_1(x_2,x_3)dx_2dx_3\ne 0. \end{aligned}$$

(5.4)

However,

$$\begin{aligned} \int \limits _{\omega }V_1(x_2,x_3)\partial _{x_2} V_1(x_2,x_3)dx_2dx_3=0 \end{aligned}$$

(5.5)

violating the condition (3.17).

The same phenomenon appears also in any cross section $\omega$ with the mirror symmetry about the $x_3$-axis, because all the modes of oscillations are either symmetric or anti-symmetric about the ordinate axis $x_2=0$, see [8, 19]. In particular, $V_0$ is symmetric and $V_1$ anti-symmetric, and hence, the relations (5.4) and (5.5) are valid, too. In view of (5.5), the result obtained in the previous section on the existence of a trapped mode does not apply. Nevertheless, it could be possible that constructing higher-order terms in the asymptotic expansion in Sect. 3 may help to satisfy the criterion (2.20). The authors do not know whether this would lead to a success.

Fig. 3

Local perturbations of the wall

Another way to fulfil both conditions (3.21) and (3.17) simultaneously is to give a small perturbation to the walls, see Fig. 3 and apply the asymptotic methods, as in [10, 17], to compute $J_1(\kappa ^0),\ K_0$ and $K_1$, cf. (3.14) and (3.19). The relation $K_0\not =0$ remains valid because the perturbation is small. Moreover, according to the general results in [12, Ch. 7] $K_1$ is a real analytic function with respect to the perturbation parameter. Hence, either $K_1$ is zero only at the isolated parameter values or vanishes identically. To prove that the last case is not possible, we have to solve explicitly at least two boundary value problems in the original domain, to find out the change of $K_1$ under a small regular or singular perturbation of the boundary. This calculation is quite long and cumbersome. Instead, in the next section, we consider the depth d as a small parameter and employ the traditional analysis of elliptic problems in thin domains (see [17, Ch. 15, 16] and [4, 27]) for which one needs to solve a system of ordinary differential equations and to compute an integral of only one solution in a semi-infinite strip.

5.2 An example of a feasible cross section

Fig. 4

Cross section with a skewed wall

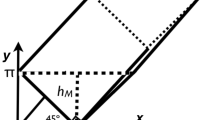
Let us assume that the cross section has the shape of a thin trapezoid, in Fig. 4

$$\begin{aligned} \begin{array}{c}{\displaystyle } \omega ^d_\theta =\{(x,y): \,y\in (-d,0),\ \cot (\theta )y<x<1\} \end{array} \end{aligned}$$

(5.6)

where the depth $d>0$ is a small parameter and $\theta \in (0,\pi /2)$ is the sharp angle of $\omega ^d_\theta$. The skewed part of the boundary is denoted by

$$\begin{aligned} \Sigma ^d_\theta =\{(x,y): \,x=\cot (\theta )y,\ y\in (-d,0)\} \end{aligned}$$

By [4, 27], we know that the eigenvalues (1.6) of the problem (1.7) in $\omega ^d_\theta$ are small, of order d, and satisfy the estimate

$$\begin{aligned} \begin{array}{c}{\displaystyle } \left| \Lambda ^d_k-\pi ^2k^2d\right| \le c_p(\theta )d^2\,\,\text{ for }\,\,d\in (0,d_k(\theta )] \end{array}\end{aligned}$$

(5.7)

with some positive numbers $c_p(\theta )$ and $d_p(\theta )$ depending on the angle $\theta$ and the index $k\in {{\mathbb {N}}}$. Clearly, as before $\Lambda ^d_0=0$ and the eigenfunction is a constant: $U^d_0=B,\ B\in {\mathbb {R}}$. Notice that the normalization of eigenfunctions is not needed in this section.

Following the general asymptotic analysis, the solution of the problem (1.7) has the following asymptotic representation

$$\begin{aligned} U^d_1(x,y)&=\cos (\pi x)+d^2 Z(x,\eta )+d^2W^1(\xi ,\eta )+\dots , \end{aligned}$$

(5.8)

$$\begin{aligned} \Lambda ^d_1&=\pi ^2 d+\mu d^2+\dots . \end{aligned}$$

(5.9)

Here, the fast variables are denoted by $(\xi ,\eta )=d^{-1}(x,y)$. The function $Y(x)=\cos (\pi x)$ is the solution of the limit spectral problem, when $d\rightarrow 0^+$,

$$\begin{aligned} Y''(x)&=- L Y(x),\ 0<x<1,\\ Y'(0)&=Y'(1)=0 \end{aligned}$$

and $L=\pi ^2$ is the corresponding eigenvalue. The function

$$\begin{aligned} Z(x,\eta )=\frac{\pi ^2}{2}\cos (\pi x)(\eta +1)^2 \end{aligned}$$

is the solution of the problem

$$\begin{aligned} \partial _\eta ^2 Z(x,\eta )&=\pi ^2\cos (\pi x),\ 0<x<1\\ \partial _\eta Z(x,0)&=\pi ^2\cos (\pi x),\ \partial _\eta Z(x,-1)=0. \end{aligned}$$

Inserting (5.8) and (5.9) into the problem (1.7), we observe that the correction term $W^1$ satisfies on the semi-infinite pointed strip

$$\begin{aligned} {\textbf{P}}_\theta =\{(\xi ,\eta ): -1<\eta<0,\ \cot (\theta )\eta <\xi \} \end{aligned}$$

the boundary value problem

$$\begin{aligned} \Delta W^1(\xi ,\eta )&=0,\ (\xi ,\eta )\in {\textbf{P}}_\theta ,\nonumber \\ \partial _\eta W^1(\xi ,\eta )&=0,\ (\xi ,\eta )\in \partial {\textbf{P}}_\theta \setminus \Sigma _\theta ,\nonumber \\ \partial _\nu W^1(\xi ,\eta )&=-\pi ^2\Big (\sin (\theta )\xi +\cos (\theta )(\eta +1)\Big )=:G(\xi ,\eta ). \end{aligned}$$

(5.10)

Here, we have denoted by $\Sigma _\theta$ the sharp end of the strip ${\textbf{P}}_\theta$:

$$\begin{aligned} \Sigma _\theta =\{(\xi ,\eta ): \xi =\cot (\theta )\eta ,\ -1<\eta <0\}. \end{aligned}$$

Since the integral $\int \limits _{\Sigma _\theta } G(\xi ,\eta )\text {d}s=0$, the problem (5.10) has a solution that decays at the rate $O(\text {e}^{-\pi \xi })$ as $\xi \rightarrow +\infty$, cf. [30, Ch. 2].

Since for small $d$, we have $x=d\xi , y=d\eta$, the asymptotic representation of the function $U^d_1(x,y)$ on $\Sigma _\theta$ takes the form

$$\begin{aligned} U^d_1(x,y)&=\cos (\pi x)+d^2Z(x,\eta )+d^2 W^1(\xi ,\eta )+{\tilde{U}}^d_1(x,y)\\&= 1-\frac{\pi ^2}{2}d^2\xi ^2+\frac{\pi ^2}{2}d^2(\eta +1)^2-\frac{\pi ^2}{4}d^4\xi ^2(\eta +1)^2\\&+d^2 W^1(\xi ,\eta )+{\tilde{U}}^d_1(d\xi ,d\eta ). \end{aligned}$$

According to the asymptotic analysis, the remainder satisfies the estimate

$$\begin{aligned} \Vert {\tilde{U}}^d_1\Vert _{H^1(\omega ^d_\theta )}\le C_\theta d^{\frac{7}{2}},\ d\in (0,D_\theta ) \end{aligned}$$

(5.11)

for some positive constants $C_\theta$ and $D_\theta$.

As was mentioned in Sect. 5.1, the requirement (3.21) is met for a small $d>0$ because the main term $\cos (\pi x)$ in (5.8) is anti-symmetric with respect to the “middle line” $\{(x,y):\, y\in (-d,0), x=1/2\}$ of the trapezoid $\omega ^d$. It remains to evaluate the integral $K^d_1$ in (3.14).

In view of the definition of the cross section (5.6), we have, using the Green formula,

$$\begin{aligned} K^d_1=\frac{1}{2}\int \limits _{\Sigma ^d_1}\left| U^d_1(1,y)\right| ^2\text {d}y- \frac{1}{2}\sin (\theta )\int \limits _{\Sigma ^d_\theta }\left| U^d_1(x,y)\right| ^2\text {d}s, \end{aligned}$$

(5.12)

where $\Sigma ^d_\theta$ is above and $\Sigma ^d_1=\{(x,y):\ x=1,\ y\in (-d,0)\}$. Using the asymptotic expansion (5.8), the exponential decay of the boundary layer term together with (5.11) yields

$$\begin{aligned} \begin{array}{c}{\displaystyle } \int \limits _{\Sigma ^d_1}|U^d_1(1,z)|^2dz=d+\frac{\pi ^2}{3}d^3+ O(d^{7/2}). \end{array} \end{aligned}$$

(5.13)

The boundary integral

$$\begin{aligned} \sin (\theta )\int \limits _{\Sigma ^d_\theta } |U^d_1(x,y)|^2\text {d}s, \end{aligned}$$

takes the form

$$\begin{aligned} \sin (\theta )\int \limits _{\Sigma ^d_\theta } |U^d_1(x,y)|^2\text {d}s&=\sin (\theta )\int \limits _{\Sigma ^d_\theta } \Big (\cos (\pi x)+d^2Z(x,\eta )\\&+d^2W^{1}(\xi ,\eta )+{\tilde{U}}^d_1(x,y)\Big )^2\text {d}s\\&=I_1+I_2+O(d^4), \end{aligned}$$

where

$$\begin{aligned} I_1&=\int \limits _{-d}^{0} \Big (\cos (\pi \cot (\theta )y)+\frac{\pi ^2d^2}{2}\cos (\pi \cot (\theta )y)\Big (\frac{y}{d}+1\Big )^2\Big )^2\text {d}y\\ \end{aligned}$$

and

$$\begin{aligned} I_2=2d^2\sin (\theta )\int \limits _{\Sigma ^d_\theta }W^1(\xi ,\eta )ds+O(d^4). \end{aligned}$$

The first integral has the asymptotic representation

$$\begin{aligned} I_1=d+\frac{\pi ^2}{3}d^3-\frac{2\pi ^2}{3}\cot (\theta )^2d^3+O(d^4). \end{aligned}$$

Since the normal derivative on $\Sigma ^d_\theta$ is

$$\begin{aligned} \partial _\nu =-sin(\theta )\partial _\xi +\cos (\theta )\partial _\eta , \end{aligned}$$

the integral $I_2$ takes the form

$$\begin{aligned} I_2&=2d^2\int \limits _{\Sigma ^d_\theta }\sin (\theta ) W^1(\xi ,\eta )ds= -2d^2\int \limits _{\Sigma ^d_\theta } W^1(\xi ,\eta )\partial _\nu \xi \,ds\\&=-2d^3\int \limits _{\Sigma ^d_\theta } \xi \partial _\nu W^1(\xi ,\eta )\,ds =d^3\pi ^2 \int \limits _{-1}^{0} \cot (\theta )^2 \eta (2\eta +1)\,d\eta \\&=\frac{d^3\pi ^2}{3}\cot (\theta )^2. \end{aligned}$$

Adding the previous results together, we finally obtain that

$$\begin{aligned} K^d_1=-\frac{\pi ^2}{6}\cot (\theta )^2)d^3+O(d^{7/2}). \end{aligned}$$

In other words, at least for small values of the depth of the channel the coefficient $K^2_1<0$ and the condition (3.17) are valid. Therefore, with a suitable local distortion of the straight channel we can create a trapped mode.

It is worth to repeat the fact that according to general results in [12, Ch.7] the function $(0,+\infty )\ni d\mapsto K^d_1$ is real analytic and therefore it can vanish only for isolated sequence of values of the parameter d.

5.3 On multiple eigenvalues

In the previous sections, we have found the eigenvalue (1.8) near the first positive threshold $\Lambda _1$. A similar procedure can be applied to higher thresholds. However, searching for an eigenvalue $\lambda ^\epsilon$ near the threshold $\Lambda _n$ in (1.6), our procedure requires more assumptions about the spectral problem (2.2) with the new Robin condition

$$\begin{aligned} \partial _z V'(x')=\Lambda _n V'(x'),\ x'\in \gamma . \end{aligned}$$

Namely, first the non-positive eigenvalues

$$\begin{aligned} M_0,\ M_1,\ \dots ,\ M_n=0 \end{aligned}$$

(5.14)

must be simple and, secondly, the corresponding eigenfunctions must satisfy

$$\begin{aligned} K_k:=\int \limits _{\omega }V_k(n,z)\partial _n V_k(n,z)\,dndz\ne 0,\ k=0,\dots ,n. \end{aligned}$$

(5.15)

Indeed, the conditions (5.15) are absolutely necessary because the equations similar to (4.6) and (4.10) involve the multipliers $K^{-1}_k$. Furthermore, if there is a multiple eigenvalue among (5.14), then it is not possible to fulfil requirements of type (4.8), because several integral operators of type (3.20) have the same kernels.

It may happen that our scheme works in the case of the eigenvalue $M_n=0$ of multiplicity $J>1$. However, in this case the analysis becomes incomparably more complicated, since the sufficient condition [11] deals with the right bottom $J\times J$-block of the augmented scattering matrix and requires that this block has the eigenvalue $-1$. Notice that also some results in Appendix A about the justification hold true only for a simple eigenvalue $\Lambda _1$.

Finally, we mention that the question of the existence of a curved waveguide supporting two different trapped modes is fully open yet.

Appendix A

The Kondratiev space $W^1_\beta (\Omega ^\epsilon )$, see [13] or [30, Ch. 5], is introduced by completing $C^\infty _0(\overline{\Omega ^\epsilon })$ with respect to the weighted Sobolev norm

$$\begin{aligned} \Vert u; W^ 1_\beta (\Omega ^\epsilon )\Vert = \Vert e^{\beta |{\varsigma }|} u; H^1(\Omega ^\epsilon )\Vert , \end{aligned}$$

(A.1)

where $\beta \in {\mathbb {R}}$ is a weight index. Clearly, $W^1_0(\Omega ^\epsilon )=H^1(\Omega ^\epsilon )$ but, for $\beta >0$, functions in $W^1_\beta (\Omega ^\epsilon )$ possess exponential decay at infinity. The weak formulation of the inhomogeneous water wave problem in the weighted space reads: find $u^\epsilon \in W^1_\beta (\Omega ^\epsilon )$ such that

$$\begin{aligned} (\nabla u^\epsilon ,\nabla v)_{\Omega ^\epsilon } -\lambda ^\epsilon (u^\epsilon ,v)_{\gamma ^\epsilon }=f(v)\quad \forall v\in W^1_{-\beta }(\Omega ^\epsilon ), \end{aligned}$$

(A.2)

where $f\in W^1_{-\beta }(\Omega ^\epsilon )^*$ is (anti)-linear functional acting in $W^1_{-\beta }(\Omega ^\epsilon )$.

The integral identity (A.2) defines a continuous mapping

$$\begin{aligned} {\mathcal {A}}^\epsilon _\beta (\lambda ^\epsilon ):\quad W^1_\beta (\Omega ^\epsilon )\rightarrow W^1_{-\beta } (\Omega ^\epsilon )^*:\ {\mathcal {A}}^\epsilon _{\beta }(\lambda ^\epsilon ) u=f. \end{aligned}$$

The Kondratiev theory [13], see also [30, Ch. 3 and 5], provides for this mapping ancillary “nice” properties under certain restrictions on the weight index. Particularly, by fixing $\beta >0$ such that the interval $[0,\beta ]$ contains only the eigenvalue $M=0$ of the problem (2.2), the operator ${\mathcal {A}}^0_{\beta }(\Lambda _1)$ becomes a monomorphism. In other words, the straight channel $\Omega ^0$ cannot support a trapped mode, in particular, at a threshold frequency. A standard perturbation argument gives us positive $\epsilon _0$ and $\delta _0$ such that, for any $\epsilon \in (0,\epsilon _0]$ and $\delta \in [-\delta _0,\delta _0]$, the operator ${\mathcal {A}}^\epsilon _\beta (\Lambda _1+\delta )$ is also a monomorphism. Since ${\mathcal {A}}^1_{-\beta }(\Lambda _1+\delta )$ is the adjoint operator for ${\mathcal {A}}^1_\beta (\Lambda _1+\delta )$, it is an epimorphism, i.e. the problem (A.2), with changing $\beta$ to $-\beta$ and $\lambda ^\epsilon$ to $\Lambda _1+\delta$, has a solution $u^\epsilon \in W^1_{-\beta }(\Omega ^\epsilon )$ for any $f\in W^1_\beta (\Omega ^\epsilon )^*$. Finally, we mention that, by the index increment theorem (see [30, Thm. 3.3.3] and our calculations in Sect. 2.1), we have

$$\begin{aligned} \text {dim ker}\,{\mathcal {A}}^\epsilon _{-\beta }(\Lambda _1+\delta )=2\times 2=4. \end{aligned}$$

(A.3)

Here, we want to mention that the dimension (=4) is the product of the number of the outlets to infinity (=2) and the number of linear solutions (=2) in the $x_1$-variable to problem ${\mathcal {P}}^0$ at the threshold frequency. The linear solutions $V_1(x')$ and $x_1V_1(x')$ are given in section 2.1. Hence, the homogeneous problem ($f=0$) in the space $W^1_{-\beta }(\Omega ^\epsilon )$ has just four linearly independent solutions.

Let us return to the spectral parameter $\lambda ^\epsilon =\Lambda _1-\epsilon ^2 \mu ^2$ introduced in (1.8). Since $W^1_{-\beta }(\Omega ^\epsilon )^*\subset W^1_{\beta }(\Omega ^\epsilon )$, the problem (A.2) with the replacement $\beta \mapsto -\beta$ has a solution $u^\epsilon \in W^1_{-\beta }(\Omega ^\epsilon )$ which may have an exponential growth at infinity. Then, according to Kondratiev’s theorem on asymptotics [13], see also [30, Thm. 3.4.1], the solution $u^\epsilon$ of (A.2) admits the representation

$$\begin{aligned} u^\epsilon (x)=\sum _{\pm }\chi _\pm (x)\sum _{p=\pm } \Big (c^{\epsilon p}_{\pm } w^{\epsilon }_{0\pm }(x) + b^{\epsilon p}_{\pm } v^\epsilon _{1\pm }(x)\Big ) +{\widetilde{u}}^\epsilon (x), \end{aligned}$$

(A.4)

where the coefficients $c^{\epsilon p}_{\pm },\,b^{\epsilon p}_{\pm }\in {\mathbb {C}}$ and the remainder ${\widetilde{u}}^\epsilon$ satisfy the estimate

$$\begin{aligned} \sum _{\pm }\sum _{p=\pm }\Big (|c^{\epsilon p}_{\pm }|+ \sqrt{\epsilon } |b^{\epsilon p}_{\pm }| \Big ) +&\Vert {\widetilde{u}}^\epsilon ;W^1_\beta (\Omega ^\epsilon )\Vert \\&\le c\ \Big ( \Vert f;W^1_{-\beta }(\Omega ^\epsilon )^*\Vert +\Vert {u}^\epsilon ;W^1_{-\beta }(\Omega ^\epsilon )\Vert \Big ). \end{aligned}$$

Notice that the factor $\sqrt{\epsilon }$ compensates the normalization factor $a^\epsilon _1=O(\epsilon ^{-\frac{1}{2}})$ in (2.8) and (2.11), which was absolutely necessary to yield the unitary property for the augmented scattering matrix $S^\epsilon$.

The space ${\textbf{W}}^1_{-\beta }(\Omega ^\epsilon ;\lambda ^\epsilon )$ with detached asymptotics is composed of functions in the form (A.4). It is a Banach space when equipped with the norm

$$\begin{aligned} \Vert u^\epsilon ;{\textbf{W}}^1_{-\beta }(\Omega ^\epsilon ;\lambda ^\epsilon )\Vert = \sum _{\pm }\sum _{p=\pm }\Big (|c^{\epsilon p}_{\pm }|+ \sqrt{\epsilon } |b^{\epsilon p}_{\pm }| \Big ) + \Vert {\widetilde{u}}^\epsilon ;W^1_\beta (\Omega ^\epsilon )\Vert , \end{aligned}$$

and it is a subspace of $W^1_{-\beta }(\Omega ^\epsilon )$. The restriction of ${\mathcal {A}}^\epsilon _{-\beta }(\lambda ^\epsilon )$ onto ${\textbf{W}}^1_{-\beta }(\Omega ^\epsilon ;\lambda ^\epsilon )$ is denoted by

$$\begin{aligned} {\textbf{A}}^\epsilon _{-\beta }(\lambda ^\epsilon ):\, {\textbf{W}}^1_{-\beta }(\Omega ^\epsilon ;\lambda ^\epsilon ) \rightarrow W^1_{-\beta }(\Omega ^\epsilon )^*\end{aligned}$$

and, according to Kondratiev’s theorem on asymptotics, inherits all properties of the operator ${\mathcal {A}}^\epsilon _{-\beta }(\lambda ^\epsilon )$. Hence, ${\textbf{A}}^\epsilon _{-\beta }(\lambda ^\epsilon )$ is a Fredholm epimorphism with a 4-dimensional kernel. Next, we introduce the restrictions ${\textbf{A}}^\epsilon _{-\beta ,out}(\lambda ^\epsilon )$ and ${\textbf{A}}^\epsilon _{-\beta ,art}(\lambda ^\epsilon )$ of the operator ${\textbf{A}}^\epsilon _{-\beta }(\lambda ^\epsilon )$ onto the subspaces

$$\begin{aligned} {\textbf{W}}^1_{-\beta ,out}(\Omega ^\epsilon ;\lambda ^\epsilon )&= \{u^\epsilon \in {\textbf{W}}^1_{-\beta }(\Omega ^\epsilon ;\lambda ^\epsilon ):\ c^{\epsilon \pm }_{\mp }=b^{\epsilon \pm }_{\pm }=0\}\quad \text {and} \end{aligned}$$

(A.5)

$$\begin{aligned} {\textbf{W}}^1_{-\beta ,art}(\Omega ^\epsilon ;\lambda ^\epsilon )&= \{u^\epsilon \in {\textbf{W}}^1_{-\beta }(\Omega ^\epsilon ;\lambda ^\epsilon ):\ c^{\epsilon \pm }_{\mp }=b^{\epsilon -}_{-}=0,\,b^{\epsilon -}_{+}=-i b^{\epsilon +}_{+}\}, \end{aligned}$$

(A.6)

respectively. Both of these subspace have co-dimension 4 and, therefore, ${\textbf{A}}^\epsilon _{-\beta ,out}(\lambda ^\epsilon )$ and ${\textbf{A}}^\epsilon _{-\beta ,art}(\lambda ^\epsilon )$ are Fredholm operators of index zero.

Since the conditions in (A.5) eliminate two incoming waves and two exponentially growing waves in the representation of the solutions in (A.4), ${\textbf{A}}^\epsilon _{-\beta ,out}(\lambda ^\epsilon )$ must be considered as the operator for the diffraction problem in the channel $\Omega ^\epsilon$ with the Sommerfeld radiation conditions at infinity. This operator gains all necessary properties, see the monograph [30, Ch. 5] for details.

The incoming waves $\chi _{\pm } w^\epsilon _{0\mp }$ do not belong to the subspace (A.6). The wave $\chi _{-}w^\epsilon _{1-}$ with the exponential growth as $x_1\rightarrow -\infty$ is also absent in ${\textbf{W}}^1_{-\beta , art}(\Omega ^\epsilon ;\lambda ^\epsilon )$ but the last relation in (A.6) allows for the outgoing wave packet $\sqrt{2} b^\epsilon _{+}\chi _{+}w^\epsilon _{1-}$, see (2.16). Hence, the operator ${\textbf{A}}^\epsilon _{-\beta ,art}(\lambda ^\epsilon )$ realizes the artificial radiation conditions which we used to introduce the solutions (2.18) and (2.19) initiated by the incoming waves $\chi _{\pm }w^\epsilon _{0\mp }$ and the exponential packet $\chi _{+}w^\epsilon _{1-}$.

The kernel of the operator ${\textbf{A}}^\epsilon _{-\beta ,out}(\lambda ^\epsilon )$ can be non-trivial, and we have constructed a channel supporting a trapped mode

$$\begin{aligned} u^\epsilon \in \text {ker }({\textbf{A}}^\epsilon _{-\beta ,out}(\lambda ^\epsilon )). \end{aligned}$$

At the same time, the operator ${\textbf{A}}^\epsilon _{-\beta ,art}(\lambda ^\epsilon )$ is an isomorphism. This property gives an implicit assistance in constructing the asymptotics of the solution $Z^\epsilon _1$ as $\epsilon \rightarrow +0$.

Since ${\textbf{A}}^\epsilon _{-\beta ,art}(\lambda ^\epsilon )$ is a Fredholm operator of index zero, it becomes an isomorphism provided

$$\begin{aligned} \text {dim ker}\, {\textbf{A}}^\epsilon _{-\beta ,art}(\lambda ^\epsilon )=0. \end{aligned}$$

(A.7)

Let then

$$\begin{aligned} u^\epsilon = \sum _{\pm }\chi _{\pm }c^\epsilon _{0\pm } w^\epsilon _{0\pm } + \chi _{+} c^\epsilon _{1+} w^\epsilon _{1+} +\chi _{+} c^\epsilon _{1-} v^\epsilon _{1+} + {\widetilde{u}}^\epsilon \end{aligned}$$

be a solution of the problem (1.3)–(1.5). Inserting it into Green’s formula at both positions yields

$$\begin{aligned} 0=\lim _{R\rightarrow \infty } \Big ( Q_{R}(u^\epsilon ,u^\epsilon ) - Q_{-R}(u^\epsilon ,u^\epsilon ) \Big ) = i|c^\epsilon _{0+}|^2+i|c^\epsilon _{0-}|^2 +i |c^\epsilon _{1+}|^2 \end{aligned}$$

according to the relations (2.13) and (2.17). Thus,

$$\begin{aligned} u^\epsilon = \chi _{-} c^\epsilon _{1-} v^\epsilon _{1+} + {\widetilde{u}}^\epsilon ,\ {\widetilde{u}}^\epsilon \in W^1_\beta (\Omega ^\epsilon ), \end{aligned}$$

(A.8)

is a trapped mode with the slow decay rate as ${\varsigma }\rightarrow -\infty$ and the fast decay rate as ${\varsigma }\rightarrow +\infty$. To conclude that the solution (A.8) is trivial, we again employ the Kondratiev theory and introduce the weighted Sobolev space $V^1_{\beta }(\Omega ^\epsilon )$ as the completion of $C^\infty _{0}(\overline{\Omega ^\epsilon })$ with respect to the norm

$$\begin{aligned} \Vert u^\epsilon ; V^1_\beta (\Omega ^\epsilon )\Vert = \Vert e^{\beta {\varsigma }} u^\epsilon ; H^1(\Omega ^\epsilon )\Vert . \end{aligned}$$

(A.9)

We emphasize here the difference between the exponents in the norms (A.9) and (A.1), so that for positive $\beta$ the functions in $V^1_\beta (\Omega ^\epsilon )$ decay as ${\varsigma }\rightarrow \infty$ but may grow as ${\varsigma }\rightarrow -\infty$. In particular, we have the inclusion $W^1_\beta (\Omega ^\epsilon )\subset V^1_\beta (\Omega ^\epsilon )$. Therefore, the trapped mode (A.8) belongs to $V^1_\beta (\Omega ^\epsilon )$. According to [13], see also [30, Thm. 3.1.1], the mapping

$$\begin{aligned} {\textbf{A}}^0(\Lambda _1): V^1_\beta (\Omega ^0)\rightarrow V^1_{-\beta }(\Omega ^0)^*\end{aligned}$$

generated by the variational problem ${\mathcal {P}}^0$ at the threshold $\lambda =\Lambda _1$ is an isomorphism. By a perturbation argument, the solution operator from $V^1_\beta (\Omega ^\epsilon )$ onto $V^1_{-\beta }(\Omega ^\epsilon )^*$ of the problem ${\mathcal {P}}^\epsilon$ with the parameter (1.8) keeps the same property. Thus, we conclude that $u^\epsilon =0$.

Calculations in [11] and [24] for general elliptic problems and the Dirichlet problem for the Helmholtz operator, respectively, turn into formulas (2.21) and (2.22) to prove that (2.20) is necessary and sufficient condition for the existence of a trapped mode $u^\epsilon \in H^1(\Omega ^\epsilon )$ with a slow decay rate as $\varsigma \rightarrow +\infty$. That is, the coefficient $b^\epsilon _{+}$ does not vanish in the representation

$$\begin{aligned} u^\epsilon (x)=\sum _{\pm }\chi _{\pm }(\varsigma )b^\epsilon _{\pm }\ v^\epsilon _{\mp }(\varsigma ,n,z)+{\tilde{u}}^\epsilon (x), \end{aligned}$$

(A.10)

where the remainder ${\tilde{u}}^\epsilon \in W^1_\beta (\Omega ^\epsilon )$. If $b^\epsilon _{+}=0$, the trapped mode (A.10) falls into the space (A.6) and becomes zero due to the formula (A.7). This concludes the proof of Theorem 2.1.