Parabolicity on Graphs

Abstract

Large scale properties of Riemannian manifolds, in particular, those properties preserved by quasi-isometries, can be studied using discrete structures which approximate the manifolds. In a sequence of papers, M. Kanai proved that, under mild conditions, many properties are preserved by a certain (quasi-isometric) graph approximation of a manifold. One of these properties is p-parabolicity. A manifold M (respectively, a graph G) is said to be p-parabolic if all positive p-superharmonic functions on M (resp. G) are constant. This is equivalent to not having p-Green’s function (i.e. a positive fundamental solution of the p-Laplace-Beltrami operator). Herein we study directly the p-parabolicity on graphs. We obtain some characterizations in terms of graph decompositions. Also, we give necessary and sufficient conditions for a uniform hyperbolic graph to be p-parabolic in terms of its boundary at infinity. Finally, we prove that if a uniform hyperbolic graph satisfies the (Cheeger) isoperimetric inequality, then it is non-p-parabolic for every $1<p<\infty$.

1 Introduction

Using discrete structures approximating Riemannian manifolds has proven to be a useful tool in the study of large scale properties. This is done by M. Kanai defining a graph, the $\varepsilon$-net of the manifold, so that the manifold and its $\varepsilon$-net are quasi-isometric. There are many works following Kanai’s ideas or proving the relation between the large scale behavior of a manifold and some other associated graph (see, e.g., [3, 14, 23, 25, 27, 28, 36,37,38, 43,44,45,46, 52, 54]).

This large-scale structure of the manifold or the corresponding graph is usually preserved by quasi-isometries, which at the same time, allow an important distortion of the local geometry. One of the main large-scale properties preserved by quasi-isometries is the Gromov hyperbolicity (see, e.g., [24, 26]).

In [31,32,33], M. Kanai studied several geometric properties (such as isoperimetric inequalities, Poincaré-Sobolev inequalities, parabolicity, growth rate of the volume of balls, and Liouville type theorems) for a large class of Riemannian manifolds with some conditions on their local geometry. Kanai proved that these properties are preserved under quasi-isometries. Also, quasi-isometries preserve the parabolic Harnack inequality (see [17]) and several estimates on transition probabilities of random walks, such as heat kernel estimates. Moreover, Holopainen and Soardi, among other authors (see [27, 28, 52]), proved that the existence of non-trivial solutions of a wide class of partial differential equations is also preserved under quasi-isometries.

A manifold M (respectively, a graph G) is said to be p-parabolic if all positive p-superharmonic functions on M (resp. G) are constant. This is equivalent to not having p-Green’s function (i.e. a positive fundamental solution of the p-Laplace-Beltrami operator). In [39] we studied the stability of p-parabolicity (with $1<p<\infty$) by quasi-isometries between Riemannian manifolds weakening Kanai’s assumptions. Also, we obtained some results on the p-parabolicity of graphs and trees; in particular, we characterized p-parabolicity for a large class of trees.

Our focus herein is to study p-parabolicity on (uniform) graphs. Sects. 2 and 3 provide the necessary background: in Sect. 2 we include basic definitions and main tools in the study of p-parabolicity; in Sect. 3 we recall the definition of boundary at infinity of a geodesic space, some basic results about the boundary at infinity of a hyperbolic space and a useful construction named hyperbolic approximation (see [13]). The hyperbolic approximation of a given metric space is a hyperbolic graph whose boundary at infinity is the given metric space.

In Sect. 4 we study the behavior of p-parabolicity in a uniform graph through certain operations as decompositions or vertex identifications.

Let X be a metric space. Fix a base point $o\in X$ and for $x,x'\in X$ let

$$\begin{aligned} (x|x')_o=\frac{1}{2}\big (d(x,o)+d(x',o)-d(x,x')\big ). \end{aligned}$$

The number $(x|x')_o$ is non-negative and it is called the Gromov product of $x,x'$ with respect to o.

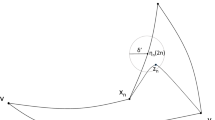
A metric space X is (Gromov) hyperbolic if it satisfies the $\delta$-inequality

$$\begin{aligned}(x|y)_o\ge \min \{(x|z)_o,(z|y)_o\}-\delta \end{aligned}$$

for some $\delta \ge 0$, for every base point $o\in X$ and all $x,y,z \in X$.

We denote by $\delta (X)$ the sharp hyperbolicity constant of X:

$$\begin{aligned} \delta (X)= \sup \Big \{ \min \{(x|z)_o,(z|y)_o\} - (x|y)_o \,\big | \;\, x,y,z,o \in X \Big \}. \end{aligned}$$

(1)

Hence, X is hyperbolic if and only if $\delta (X)<\infty$.

The theory of Gromov hyperbolic spaces was introduced by M. Gromov for the study of finitely generated groups (see [26]). The concept of Gromov hyperbolicity grasps the essence of negatively curved spaces like the classical hyperbolic space, Riemannian manifolds of negative sectional curvature bounded away from 0, and of discrete spaces like trees and the Cayley graphs of many finitely generated groups (see [1, 24, 26]). This theory has been developed from a geometric point of view to the extent of making hyperbolic spaces an important class of metric spaces to be studied on their own (see, e.g., [10, 11, 13, 24, 55]). In the last years, Gromov hyperbolicity has been intensely studied in graphs (see, e.g., [5, 6, 8, 29, 30, 34, 35, 47, 49,50,51, 56] and the references therein).

In Sect. 5 we give necessary and sufficient conditions for a uniform hyperbolic graph to be p-parabolic in terms of the boundary at infinity of the graph.

In the last section, we prove the relation between p-parabolicity and Cheeger isoperimetric inequality for uniform hyperbolic graphs. Isoperimetric inequalities are of interest in pure and applied mathematics (see, e.g., [15, 42]). The Cheeger isoperimetric inequality is related with many conformal invariants in graphs and Riemannian manifolds, namely the bottom of the spectrum of the Laplace-Beltrami operator, Poincaré-Sobolev inequalities, the exponent of convergence, and the Hausdorff dimensions of the sets of both escaping and bounded geodesics in negatively curved surfaces (see [2, 7, 12, p.228], [16, 18,19,20,21,22, 40, 41, 53, p.333]).

There is a natural connection between isoperimetric inequality and hyperbolicity. Recall that one of the definitions of Gromov hyperbolicity involves some kind of isoperimetric inequality (see [1, 26]). In [36, 38] we studied the relationship between the hyperbolicity and the Cheeger isoperimetric inequality in the context of graphs and Riemannian manifolds with bounded local geometry. In those works we obtained a characterization of graphs and Riemannian manifolds (with bounded local geometry) satisfying the (Cheeger) isoperimetric inequality, in terms of their Gromov boundary.

Here, in Sect. 6, we prove that if a uniform hyperbolic graph satisfies the Cheeger isoperimetric inequality, then it is non-p-parabolic for every $1<p<\infty$.

2 Definitions and Background

A function between two metric spaces $f:X\rightarrow Y$ is said to be an (a, b)-quasi-isometric embedding with constants $a\ge 1,\,b\ge 0$, if

$$\begin{aligned} \frac{1}{a}\,d_{X}(x_1,x_2)-b\le d_{Y}(f(x_1),f(x_2)) \le a \, d_{X}(x_1,x_2)+b, \qquad \text {for every } x_1,x_2\in X. \end{aligned}$$

Such a quasi-isometric embedding f is a quasi-isometry if there exists a constant $c\ge 0$ such that f is c-full, i.e., if for every $y\in Y$ there exists $x\in X$ with $d_Y(y,f(x))\le c$.

Two metric spaces X and Y are quasi-isometric if there exists a quasi-isometry between them. It is well-known that to be quasi-isometric is an equivalence relation (see, e.g., [31]).

Given a graph G let us denote as V(G) its vertex set and E(G)) its edge set. Given a function $u:V(G)\rightarrow \mathbb {R}$, define the p-modulus of its discrete gradient $|\nabla _{G} u|_p$ and its discrete p-Dirichlet integral $D_{p,G} (u)$, respectively, by

$$\begin{aligned} |\nabla _{G} u|_p(x):= & {} \Big ( \sum _{y\in N(x)}\big |u(y)-u(x)\big |^p \Big )^{1/p}, \\ D_{p,G} (u):= & {} \sum _{x\in V(G)}|\nabla _{G} u|_p^p(x) = 2 \!\!\!\! \sum _{vw\in E(G)} \big | u(v)-u(w) \big |^p, \end{aligned}$$

where the edges are considered unoriented.

For a finite subset S of V(G), the p-capacity of S is defined by

$$\begin{aligned} {{\,\mathrm{{\text {cap}}}\,}}_p S= & {} {{\,\mathrm{{\text {cap}}}\,}}_p (S,G) \\= & {} \inf \Big \{D_{p,G} (u): u \text { function on } V(G) \text { with finite support}, \; u|_{S} =1 \Big \}. \end{aligned}$$

A graph G is said to be $\mu$-uniform if each vertex p of V(G) has at most $\mu$ neighbors, i.e.,

$$\begin{aligned}\sup \big \{|N(p)| \,: \,\, p\in V(G)\big \}\le \mu . \end{aligned}$$

If a graph G is $\mu$-uniform for some constant $\mu$ we say that G is uniform

Theorem 1

Given $1<p<\infty$, a uniform graph G is p-parabolic if and only if ${{\,\mathrm{{\text {cap}}}\,}}_p S =0$ for some (and then for every) non-empty finite subset of $S \subset V(G)$.

For a proof of Theorem 1, see [33, Proposition 6] and [27, Final remark 5.16]. Note that the definition of discrete p-Dirichlet integral in [27] is slightly different, but both are equivalent.

Also, Corollary 7 in [32] can be trivially extended to the general case (being p-parabolic for an arbitrary $p>1$ instead of $p=2$) to obtain the following:

Proposition 2

If two uniform graphs P and Q are quasi-isometric, then P is p-parabolic if so is Q.

The Cantor tree $(T_C,v_0)$ is a rooted tree such that the root, $v_0$, has degree two and any other vertex has degree three.

Proposition 3

[39, Corollary 5]. The Cantor tree $(T_{C},v_0)$ is non-p-parabolic for every $1<p<\infty$.

Proposition 4

[39, Proposition 6]. If a uniform graph $\Gamma$ contains a non-p-parabolic subgraph $\Gamma '$ for some $1<p<\infty$, then $\Gamma$ is non-p-parabolic.

3 Boundary at Infinity and Hyperbolic Approximation

Let us recall that a geodesic space is a metric space such that for every couple of points there exists a geodesic joining them. A geodesic ray in a metric space X is the image of an isometric embedding $F: [0,\infty ) \rightarrow X$. In this case, we say that the geodesic ray emanates from F(0). A geodesic space X has a pole in a point v if there exists $M > 0$ such that each point of X lies in an M-neighborhood of some geodesic ray emanating from v.

Let us recall the concepts of geodesic and sequential boundary of a hyperbolic space and some basic properties. For further information and proofs we refer the reader to [10, 13, 24, 26]. Let X be a hyperbolic space and $o\in X$ a base point.

The relative geodesic boundary of X with respect to the base-point o is

$$\begin{aligned} \partial _o^g X:= \{ [\gamma ] \, | \, \gamma : [0,\infty ) \rightarrow X \text { is a geodesic ray with } \gamma (0) = o\}, \end{aligned}$$

where $\gamma _1 \sim \gamma _2$ if there exists some $K>0$ such that $d(\gamma _1(t),\gamma _2(t))<K$, for every $t\ge 0.$

In fact, in the definition above the equivalence classes of the geodesic rays do not depend on the election of the base point. Therefore, the set of classes of geodesic rays is called geodesic boundary of X, $\partial ^gX$. Herein, we do not distinguish between the geodesic ray and its image.

A sequence of points $\{x_i\}\subset X$ converges to infinity if

$$\begin{aligned} \lim _{i,j\rightarrow \infty } (x_i|x_j)_o=\infty . \end{aligned}$$

This property is independent of the choice of o since

$$\begin{aligned} |(x|x')_o-(x|x')_{o'}|\le d(o,o') \end{aligned}$$

for any $x,x',o,o' \in X$.

Two sequences $\{x_i\},\{x'_i\}$ that converge to infinity are equivalent if

$$\begin{aligned}\lim _{i\rightarrow \infty } (x_i|x'_i)_o=\infty . \end{aligned}$$

Using the $\delta$-inequality (1), we easily see that this defines an equivalence relation for sequences in X converging to infinity. The sequential boundary at infinity $\partial _\infty X$ of X is defined to be the set of equivalence classes of sequences converging to infinity.

Note that given a geodesic ray $\gamma$, the sequence $\{\gamma (n)\}$ converges to infinity and two equivalent rays induce equivalent sequences. Thus, in general, $\partial ^g X\subseteq \partial _\infty X$.

We say that a metric space is proper if every closed ball is compact. Every uniform graph is a proper geodesic space.

Proposition 5

[10, Chapter III.H, Proposition 3.1]. If X is a proper hyperbolic geodesic space, then the natural map from $\partial ^g X$ to $\partial _\infty X$ is a bijection.

For every $\xi , \xi ' \in \partial _\infty X$, its Gromov product with respect to the base point $o\in X$ is defined as

$$\begin{aligned} (\xi |\xi ')_o = \inf \ \liminf _{i\rightarrow \infty } (x_i|x'_i)_o, \end{aligned}$$

where the infimum is taken over all sequences $\{x_i\} \in \xi$, $\{x'_i\} \in \xi '$.

Remark 1

[13, Lemma 2.2.2] If X is a $\delta$-hyperbolic geodesic space, then for every pair of geodesic rays $\sigma , \sigma '$ with $\sigma (0)=x_0=\sigma '(0)$ and such that $\{\sigma (n)\}\in \xi$ and $\{\sigma '(n)\} \in \xi '$,

$$\begin{aligned} (\xi |\xi ')_{x_0}\le \liminf _{t\rightarrow \infty } (\sigma (t)|\sigma '(t))_{x_0}\le \limsup _{t\rightarrow \infty } (\sigma (t)|\sigma '(t))_{x_0}\le (\xi |\xi ')_{x_0}+2\delta . \end{aligned}$$

The Gromov product

$$\begin{aligned}(x|\xi )_o = \inf \ \liminf _{i\rightarrow \infty } (x|x_i)_o \end{aligned}$$

is defined for any $x \in X$, $\xi \in \partial _\infty X$, where the infimum is taken over all sequences $\{x_i\} \in \xi$.

A hyperbolic space X is said to be visual, if for some base point $o\in X$ there is some constant $D>0$ such that for every $x\in X$ there is $\xi \in \partial _\infty X$ with $d(o,x) \le (x|\xi )_o + D$. Moreover, this property is independent of the choice of o.

Proposition 6

[36, Proposition 4.4]. A proper hyperbolic geodesic space has a pole if and only if it is visual.

A metric d on the sequential boundary at infinity $\partial _\infty X$ of X is said to be visual, if there are $o\in X$, $a > 1$ and positive constants $c_1$, $c_2$, such that

$$\begin{aligned}c_1a^{-(\xi |\xi ')_o} \le d(\xi , \xi ') \le c_2a^{-(\xi |\xi ')_o} \end{aligned}$$

for all $\xi , \xi ' \in \partial _\infty X$. In this case, we say that d is a visual metric with respect to the base point o and the parameter a.

Theorem 7

[13, Theorem 2.2.7]. Let X be a hyperbolic space. Then for any $o \in X$, there is $a_0 > 1$ such that for every $a \in (1, a_0]$ there exists a metric d on $\partial _\infty X$, which is visual with respect to o and a.

Remark 2

Notice that for any visual metric, $\partial _\infty X$ is bounded and complete.

Proposition 8

[24, Proposition 7.9]. If X is a proper, geodesic, hyperbolic space, then $\partial _\infty X$ is compact.

Let us recall the following construction from [13].

A subset A in a metric space (X, d) is called r-separated, $r>0$, if $d(a,a')\ge r$ for any distinct $a,a'\in A$. Note that if A is maximal with this property, then the union $\cup _{a\in A} B_r(a)$ covers X. A maximal r-separated set A in a metric space X is called an r-approximation of X.

A hyperbolic approximation of a metric space X, ${\mathcal {H}}(X)$, is a graph defined as follows. Fix a positive $s\le \frac{1}{6}$ which is called the parameter of ${\mathcal {H}}(X)$. For every $k\in \mathbb {Z}$, let $A_k\in X$ be an $s^k$-approximation of X. For every $a\in A_k$, consider the ball $B(a,2s^k)\subset X$. Let us define, for every k, the set $V^*_k:=\{B(a,2s^k)\,|\;a\in A_k\}$ and a set $V_k$ which has a vertex corresponding to each ball in $V^*_k$. Then let $V=\cup _{k\in \mathbb {Z}}V_k$ be the set of vertices of the graph ${\mathcal {H}}(X)$. Thus, every vertex $v \in V$ corresponds to some ball $B(a, 2s^k)$ with $a \in A_k$ for some k. Let us denote the corresponding ball to $v\in V$ simply by B(v).

There is a natural level function $l:V \rightarrow \mathbb {Z}$ defined by $l(v)=k$ for $v\in V_k$.

Vertices $v,v'$ are connected by an edge if and only if they either belong to the same level, $V_k$, and the closed balls ${\bar{B}}(v),{\bar{B}}(v')$ intersect, ${\bar{B}}(v)\cap {\bar{B}}(v')\ne \emptyset$, or they lie on neighboring levels $V_k,V_{k+1}$ and the ball of the upper level, $V_{k+1}$, is contained in the ball of the lower level, $V_k$.

Since $A_k$ is an $s^k$-approximation of X for any $k\in \mathbb {Z}$ and $s\le \frac{1}{6}$, every vertex in $V_k$ has a neighbor in $V_{k+1}$.

An edge $vv'\subset {\mathcal {H}}(X)$ is called horizontal if its vertices belong to the same level, $v,v'\in V_k$ for some $k\in \mathbb {Z}$. Other edges are called radial. Consider the path metric on X for which every edge has length 1.

Note that any (finite or infinite) sequence $\{v_k\}\in V$ such that $v_kv_{k+1}$ is a radial edge for every k and such that the level function l is monotone along $\{v_k\}$, is the vertex sequence of a geodesic in ${\mathcal {H}}(X)$. Such a geodesic is called radial.

Proposition 9

[13, Proposition 6.2.10]. For any metric space (X, d), ${\mathcal {H}}(X)$ is a geodesic 3-hyperbolic space.

Assume now that X is bounded and non-trivial. Then, since $s<1$, there is a maximal integer k with ${{\,\textrm{diam}\,}}X<s^k$ and it is denoted by $k_0=k_0({{\,\textrm{diam}\,}}X,s)$. Then, for every $k\le k_0$ the vertex set $V_k$ consists of one point, and therefore contains no essential information about X. Thus, the graph ${\mathcal {H}}(X)$ can be modified making $V_k=\emptyset$ for every $k<k_0$. This modified graph is called the truncated hyperbolic approximation of X, ${\mathcal {H}}^t(X)$. The level function l restricted to ${\mathcal {H}}^t(X)$ has a unique minimum, v, with $l(v)=k_0$. This point v can be considered as the natural base point of the truncated hyperbolic approximation.

Theorem 10

[13, Proposition 6.4.1]. Let $\Gamma$ be a truncated hyperbolic approximation of a complete bounded metric space (X, d). Then there is a canonical identification $\partial _\infty \Gamma = X$ under which the metric d of X is a visual metric on $\partial _\infty \Gamma$ with respect to the natural base point v of $\Gamma$ and the parameter $a = \frac{1}{s}$.

The following definition appeared in [36] and was inspired by the definition of a metric space having bounded geometry in [9].

Definition 1

Given a metric space (X, d), we say that X has strongly bounded geometry if for every $K>0$ there exists $M>0$ such that the following condition is satisfied: for any $\varepsilon >0$, any $\varepsilon$-approximation of X, $A_\varepsilon$, and any $x\in X$, $|A_\varepsilon \cap B(x,K\varepsilon )|<M$.

Remark 3

Note that if $\varepsilon > {{\,\textrm{diam}\,}}X$, then $|A_\varepsilon |=1$ and $|A_\varepsilon \cap B(x,K\varepsilon )| \le |A_\varepsilon |=1$. Hence, in order to check whether X has strongly bounded geometry or not, it suffices to consider $0 < \varepsilon \le {{\,\textrm{diam}\,}}X$.

Theorem 11

[13, Corollary 7.1.6]. Visual hyperbolic geodesic spaces $X,X'$ with bilipschitz equivalent boundaries at infinity are roughly similar to each other. In particular, every visual hyperbolic space (i.e. every hyperbolic space with a pole) is roughly similar (and therefore, quasi-isometric) to any (truncated) hyperbolic approximation of its boundary at infinity; and any two hyperbolic approximations of a complete bounded metric space Z are roughly similar (and therefore, quasi-isometric) to each other.

Proposition 12

[36, Proposition 4.14]. If G is a hyperbolic uniform graph with a pole, then $\partial _\infty G$ with any visual metric has strongly bounded geometry.

Proposition 13

[36, Proposition 4.11]. If the metric space (X, d) has strongly bounded geometry, then ${\mathcal {H}}(X)$ is uniform.

4 Parabolicity and Graph Decomposition

The following results are useful, as Proposition 4, in order to determine if a graph is p-parabolic or not.

Proposition 14

Let $G_1,G_2$ be two uniform graphs such that $V_0:=\big (V(G_1) \cup V(G_2)\big ) {\setminus } \big (V(G_1) \cap V(G_2)\big )$ is a finite set and such that the subgraphs of $G_1$ and $G_2$ induced by $V(G_1) \cap V(G_2)$ are the same. Then $G_1$ is p-parabolic if and only if $G_2$ is p-parabolic.

Proof

Let us define for each $i=1,2,$

$$\begin{aligned} R:= \cup _{v \in V_0} N(v), \qquad S_i:= \big (V_0 \cup R\big ) \cap V(G_i). \end{aligned}$$

If $u_1: V(G_1) \rightarrow \mathbb {R}$ has finite support and $u_1|_{S_1}=1$, then the function $u_2: V(G_2) \rightarrow \mathbb {R}$ defined by $u_2|_{S_2}=1$ and $u_2|_{V(G_2)\setminus S_2}=u_1$ has finite support and $D_{p,G_2} (u_2) = D_{p,G_1} (u_1)$. Hence, ${{\,\mathrm{{\text {cap}}}\,}}_p (S_2,G_2) \le {{\,\mathrm{{\text {cap}}}\,}}_p (S_1,G_1)$. By symmetry, we have the converse inequality and Theorem 1 gives that $G_1$ is p-parabolic if and only if $G_2$ is p-parabolic. $\square$

Proposition 15

Let G be a uniform graph and consider a finite or countable set $\{e_n\} \subseteq E(G)$ and $1<p<\infty$. Let us consider the graph $G'$ obtained from G by replacing each edge $e_n$ by a path of length $\ell _n$ for each n.

(1) If G is p-parabolic, then $G'$ is p-parabolic.

(2) If $\sup _n \ell _n < \infty$, then G is p-parabolic if and only if $G'$ is p-parabolic.

Proof

Let us fix $S \subset V(G) \subset V(G')$ and denote by $v_n$ and $w_n$ the incident vertices to $e_n$.

Consider $u: V(G)\longrightarrow \mathbb {R}$ with finite support and $u|_{S} =1$. Define the function $u': V(G')\longrightarrow \mathbb {R}$ defined as $u'|_{V(G)}:=u$ and if $z \in V(G')\setminus V(G)$, then z is contained in the path of length $\ell _n$ for some n and

$$\begin{aligned} u'(z):= u(v_n) + d_{G'}(z,v_n)\,\frac{u(w_n)-u(v_n)}{\ell _n}. \end{aligned}$$

It is clear that $u'$ has finite support and $u'|_{S} =1$. Since $p>1$, we have

$$\begin{aligned} \big | u(w_n)-u(v_n)\big |^p \ge \ell _n \Big | \, \frac{u(w_n)-u(v_n)}{\ell _n}\, \Big |^p \end{aligned}$$

and so, $D_{p,G'} (u') \le D_{p,G} (u)$. Thus, ${{\,\mathrm{{\text {cap}}}\,}}_p (S,G') \le {{\,\mathrm{{\text {cap}}}\,}}_p (S,G)$ and Theorem 1 gives that $G'$ is p-parabolic if G is p-parabolic.

Assume now that $\sup _n \ell _n = \ell < \infty$. Consider $U: V(G')\longrightarrow \mathbb {R}$ with finite support and $U|_{S} =1$. Define the function $U_0: V(G)\longrightarrow \mathbb {R}$ defined as $U_0:=U|_{V(G)}$. It is clear that $U_0$ has finite support and $U_0|_{S} =1$.

Fix n and denote by $\{z_j\}_{j=0}^{\ell _n}$ the vertices contained in the path of length $\ell _n$ with $d_{G'}(z_j,v_n)=j$ (thus, $z_0=v_n$ and $z_{\ell _n}=w_n$). If $x_j:= \big | U(z_{j})-U(z_{j-1})\big |$ for $1 \le j \le \ell _n$, then

$$\begin{aligned} \big | U_0(w_n)-U_0(v_n)\big |^p \le \left( \sum _{j=1}^{\ell _n} x_j \right) ^p \le \ell _n^{p-1}\sum _{j=1}^{\ell _n} x_j^p \le \ell ^{p-1}\sum _{j=1}^{\ell _n} x_j^p. \end{aligned}$$

and so, $D_{p,G} (U_0) \le \ell ^{p-1} D_{p,G'} (U)$.

Thus, ${{\,\mathrm{{\text {cap}}}\,}}_p (S,G) \le \ell ^{p-1} {{\,\mathrm{{\text {cap}}}\,}}_p (S,G')$ and Theorem 1 gives that G is p-parabolic if $G'$ is p-parabolic. $\square$

Proposition 16

Let G be a uniform graph and $1<p<\infty$. Consider some constant $K>1$ and a finite or countable set $\cup _n \{v_n^j\}_{j=1}^{k_n} \subseteq V(G)$ with $\{v_n^j\}_{j=1}^{k_n}\cap \{v_m^j\}_{j=1}^{k_m}=\emptyset$ for $n\ne m$ and $1< k_n \le K$ for every n. Let us consider the graph $G'$ obtained from G by identifying the vertices $\{v_n^j\}_{j=1}^{k_n}$ at a single vertex $v_n^*$ for each n, with $N(v_n^*) = \cup _{j=1}^{k_n} N(v_n^j) {\setminus } \{v_n^j\}_{j=1}^{k_n}$ for each n. If $G'$ is p-parabolic, then G is p-parabolic. Furthermore, if $\cup _n \{v_n^j\}_{j=1}^{k_n}$ is a finite set, then $G'$ is p-parabolic if and only if G is p-parabolic.

Proof

Proposition 14 gives the second statement. Let us prove the first one.

Suppose G is $\mu$-uniform. Therefore, $G'$ is $(K\cdot \mu )$-uniform.

Consider $S'=\{v_1^*\}$ and a function $u'$ on $V(G')$ with finite support and $u'|_{S'}=1$. Let us define $S= \{v_1^j\}_{j=1}^{k_1}$ and a function u on V(G) as follows: $u(v_n^j)=u'(v_n^*)$ for each n and j, and $u(v)=u'(v)$ if $v \ne v_n^j$ for every n and j. Thus, u has finite support and $u|_{S}=1$.

Notice that, since G is $\mu$-uniform, $|N(w)\cap \{v_n^j\}_{j=1}^{k_n}|\le \mu$ for any $w\in V(G)$. Therefore, $D_{p,G} (u) \le \mu \cdot D_{p,G'} (u')$. Consequently,

$$\begin{aligned} {{\,\mathrm{{\text {cap}}}\,}}_p (S,G) \le \mu \cdot {{\,\mathrm{{\text {cap}}}\,}}_p (S',G') \end{aligned}$$

and Theorem 1 gives that G is p-parabolic, since $G'$ is uniform and p-parabolic.$\square$

Let us denote ${{\,\textrm{diam}\,}}(A):= \sup _{x,y\in A} d(x,y)$.

Proposition 17

Let G be a uniform graph and $1<p<\infty$. Consider some constant $D>1$ and a finite or countable set $\cup _n \{v_n^j\}_{j=1}^{k_n} \subseteq V(G)$ with ${{\,\textrm{diam}\,}}(\{v_n^j\}_{j=1}^{k_n})< D$ for every n. Let us consider the graph $G'$ obtained from G by identifying the vertices $\{v_n^j\}_{j=1}^{k_n}$ at a single vertex $v_n^*$ for each n, with $N(v_n^*) = \cup _{j=1}^{k_n} N(v_n^j) {\setminus } \cup _{j=1}^{k_n} \{v_n^j\}$ for each n. Then G is p-parabolic if and only if $G'$ is p-parabolic.

Proof

Since G is uniform, if ${{\,\textrm{diam}\,}}(\{v_n^j\}_{j=1}^{k_n})<D$, then there exists some constant K such that $k_n \le K$ for every n. Thus, by Proposition 16, if $G'$ is p-parabolic, then G is p-parabolic.

Suppose G is p-parabolic and let $v_0\in V(G){\setminus } \cup _n \{v_n^j\}_{j=1}^{k_n}$. Then, by Theorem 1, for every $i\in \mathbb {N}$ there is a function $u_i:V(G)\rightarrow \mathbb {R}$ with finite support and $u_i(v_0)=1$ such that $D_{p,G}(u_i)<\frac{1}{i}$. Let us define $u^*_i:V(G')\rightarrow \mathbb {R}$ so that $u^*_i(w)=u_i(w)$ for every $w\in V(G){\setminus } \cup _n \{v_n^j\}_{j=1}^{k_n}$ and $u^*_i(v_n^*):=u_i(v_n^1)$ for every $n\in \mathbb {N}$. Then, $u^*_i(v_0)=1$ and $u^*_i$ has finite support.

For each $n\in \mathbb {N}$, let

$$\begin{aligned} \begin{aligned} {\mathcal {A}}_n&= \big \{ vw \in E(G): \, v\in B(v_n^1,D), \, w\in N(v) \big \}, \\ d_n&=\max \big \{ |u_i(v)-u_i(w)|: \, vw \in {\mathcal {A}}_n \big \}. \end{aligned} \end{aligned}$$

If G is $\mu$-uniform, in ${\overline{B}}(v,2D)$ there are at most

$$\begin{aligned} 1+\mu +\mu ^2+\cdots +\mu ^{2D} = \frac{\mu ^{2D+1}-1}{\mu -1} \end{aligned}$$

vertices, for every $v \in V(G)$. Thus,

$$\begin{aligned}{} & {} \big | \big \{ k: \, {\overline{B}}(v_n^1,D) \cap {\overline{B}}(v_k^1,D) \ne \emptyset \big \} \big |\\{} & {} \quad \le \big | \,{\overline{B}}(v_n^1,2D) \setminus \{v_n^1\} \big | \\{} & {} \quad \le \frac{\mu ^{2D+1}-1}{\mu -1} -1 = \frac{\mu ^{2D+1}-\mu }{\mu -1} , \end{aligned}$$

and so, an edge in E(G) belongs at most to $\frac{\mu ^{2D+1}-\mu }{\mu -1}$ different sets ${\mathcal {A}}_n$.

Notice that, since ${{\,\textrm{diam}\,}}(\{v_n^j\}_{j=1}^{k_n})< D$, for every vertex $w\in V(G')$ adjacent to $v_n^*$,

$$\begin{aligned} |u^*_i(v_n^*)-u^*_i(w)|\le D\cdot d_n. \end{aligned}$$

Also, since G is uniform and ${{\,\textrm{diam}\,}}(\{v_n^j\}_{j=1}^{k_n})<D$, there is a constant $\mu '$ such that $G'$ is $\mu '$-uniform. Therefore,

$$\begin{aligned} D_{p,G'}(u^*_i)\le \frac{\mu ^{2D+1}-\mu }{\mu -1} \,\mu '\cdot D^p\cdot D_{p,G}(u_i) <\frac{\mu ^{2D+1}-\mu }{\mu -1}\,\frac{\mu ' D^p}{i}. \end{aligned}$$

Hence, $G'$ is p-parabolic by Theorem 1. $\square$

In general, a graph obtained by attaching a countable set of p-parabolic graphs through arbitrary subsets of vertices is not necessarily a p-parabolic graph. However, the following result provides sufficient conditions for G to be p-parabolic.

Theorem 18

Consider $1<p<\infty$ and a finite or countable set of graphs $\{G_n\}_{n=0}^N$ $(1\le N \le \infty )$. Let G be the graph obtained from $\{G_n\}_{n=0}^N$ as follows: given some $D\in \mathbb {N}$, a finite or countable set of vertices $\{v_{n,j}\}_{j=1}^{N_n} \subseteq V(G_n)$ with $1\le N_n < \infty$ and ${{\,\textrm{diam}\,}}(\{v_{n,j}\}_{j=1}^{N_n})<D$ for each $n\ge 1$, and a set of vertices $\cup _{n=1}^N \{v_{n,j}^0\}_{j=1}^{N_n} \subseteq V(G_0)$, G is the graph obtained by identifying $v_{n,j}$ with $v_{n,j}^0$ for each $n,j\ge 1$. If G is a uniform graph, then G is p-parabolic if and only if $G_n$ is p-parabolic for every $n \ge 0$.

Proof

Note that $G_n$ is uniform for every $n \ge 0$ since G is uniform.

If G is p-parabolic, then Proposition 4 gives that $G_n$ is p-parabolic for every $n \ge 0$.

Assume now that $G_n$ is p-parabolic for every $n \ge 0$.

By Proposition 17 it suffices to prove that the graph $G'$ obtained from G by identifying the vertices $\{v_{n,j}=v_{n,j}^0\}_{j=1}^{N_n}$ at a single vertex $v^*_n$ for each $n\ge 1$ is p-parabolic. Also, by Proposition 17, if $G'_n$ is the corresponding subgraph of $G'$ obtained by the identification of these vertices in $G_n$, then $G'_n$ is p-parabolic for each $n \ge 0$. Therefore, it suffices to prove that G is p-parabolic assuming that $N_n=1$ for every $n \ge 1$.

Since $G_0$ is a p-parabolic graph, given $\varepsilon >0$ and a non-empty finite subset $S_0 \subset V(G_0)$, by Theorem 1 there exists a function $u_0$ on $V(G_0)$ with finite support, $u_0|_{S_0}=1$ and $D_{p,G_0} (u_0)< \varepsilon /2$. Since $u_0$ has finite support and G is a uniform graph, there is K such that $u_0(v_{n,1}^0)=0$ for every $n>K$. Since $G_1,\dots ,G_K$ are p-parabolic graphs, if we choose the finite sets $S_1=\{v_{1,1}\},\dots ,S_K=\{v_{K,1}\},$ then Theorem 1 gives that there exist functions $u_n$ on $V(G_n)$ with finite support, $u_n(v_{n,1})=u_0(v_{n,1}^0)$ and $D_{p,G_n} (u_n)< \varepsilon /2^{n+1}$ for each $1 \le n \le K$.

Define a function u on V(G) as follows: $u|_{V(G_0)}=u_0$, $u|_{V(G_n)}=u_n$ for each $1 \le n \le K$ and $u|_{V(G_n)}=0$ for each $n > K$. It is clear that u has finite support, $u|_{S_0}=1$ and

$$\begin{aligned} D_{p,G} (u) = D_{p,G_0} (u_0) + \sum _{n=1}^K D_{p,G_n} (u_n)< \frac{\varepsilon }{2} + \sum _{n=1}^K \frac{\varepsilon }{2^{n+1}} < \varepsilon . \end{aligned}$$

Since G is a uniform graph, Theorem 1 gives that G is p-parabolic. $\square$

5 Parabolicity of Hyperbolic Graphs

Given a subset A in metric space (X, d) let us denote ${{\,\textrm{diam}\,}}(A):= \sup _{x,y\in A} d(x,y)$.

Definition 2

A metric space (X, d) is locally perfect at the point $x\in X$ if there exist $\varepsilon _0 >0$ and $\lambda >1$ such that ${{\,\textrm{diam}\,}}(B(z,\varepsilon ))>\frac{\varepsilon }{\lambda }$ for every $z\in B(x,\varepsilon _0)$ and every $0<\varepsilon <\varepsilon _0$.

Theorem 19

If G is a hyperbolic uniform graph with a pole such that $\partial _\infty G$ is locally perfect at some point for some visual metric, then G is non-p-parabolic for every $1<p<\infty$.

Proof

Suppose that $\partial _\infty G$ is locally perfect at the point x for some visual metric $d_\infty$ with parameters $\varepsilon _0$ and $\lambda$. Let us assume, with no loss of generality, that $\varepsilon _0<{{\,\textrm{diam}\,}}(\partial _\infty G)$. Consider some parameter $s<\min \{\frac{1}{6},\frac{1}{2\lambda }\}$ and let $\Gamma$ be the truncated hyperbolic approximation of $\partial _\infty G$ with parameter s.

Now, let $m\in \mathbb {N}$ be such that $2s^m<\varepsilon _0$ and let $a\in B(x,\varepsilon _0)\cap A_m$. Let v be the vertex in $\Gamma$ such that $B(v)=B(a,2s^m)$. Since $\partial _\infty G$ is locally perfect, ${{\,\textrm{diam}\,}}(B(a,s^m))>\frac{s^m}{\lambda }>2s^{m+1}$. Hence, there are two points $z_1,z_2\in B(a,s^m)$ with $d_\infty (z_1,z_2)>2s^{m+1}$, and since $A_{m+1}$ is a maximal $s^{m+1}$-separated set, there are two points $a_1\in B(z_1,s^{m+1})\cap A_{m+1}$, $a_2\in B(z_2,s^{m+1})\cap A_{m+1}$ so that $B(a_1,2s^{m+1})\ne B(a_2,2s^{m+1})$. Hence, there are two different vertices $w_1,w_2$ in $\Gamma$ with $B(w_i)=B(a_i,2\,s^{m+1})$ for $i=1,2$ and such that $vw_1$ and $vw_2$ are radial edges in $\Gamma$.

Since every vertex v as above has two adjacent vertices in the next level of $\Gamma$ it follows that $\Gamma$ contains a Cantor tree as a subgraph. Thus, by propositions 3 and 4, $\Gamma$ is non-parabolic for every $1<p<\infty$.

Therefore, by Theorem 11 and propositions 12 and 13, G and $\Gamma$ are quasi-isometric uniform graphs and by Proposition 2, G is non-p-parabolic for every $1<p<\infty$. $\square$

Given two sequences of positive integers $L=\{\ell _n\}_{n=1}^\infty$ and $R=\{r_n\}_{n=1}^\infty$, with $2 \le r_n \le N$ for every $n \ge 1$ and some constant N, the Cantor tree $(T_{L,R},v_0)$ is a rooted tree such that the root, $v_0$, has degree $r_1$, the vertices at distance $\ell _1 + \dots + \ell _{n-1}$ have degree $r_n+1$, and any other vertex has degree two. In [36] we gave the following characterization of p-parabolicity for Cantor trees.

Theorem 20

[36, Theorem 7] Given $1<p<\infty$ and sequences $L=\{\ell _n\}_{n=1}^\infty$ and $R=\{r_n\}_{n=1}^\infty$, the Cantor tree $(T_{L,R},v_0)$ is p-parabolic if and only if

$$\begin{aligned} \sum _{k=1}^{\infty } \frac{\ell _k}{(r_1\cdots r_k)^{1/(p-1)}} = \infty . \end{aligned}$$

Using the same ideas, we can obtain a sufficient condition for a hyperbolic uniform graph to be p-parabolic. Moreover, Theorem 22 bellow, when $p=2$ is a consequence of Corollary 2.6 in [48]. Let us recall the following Lemma:

Lemma 21

[39, Lemma 11]. Let $1< p < \infty$, $a_1,\dots ,a_n > 0$, $f: [0,\infty )^n \rightarrow \mathbb {R}$ given by

$$\begin{aligned} f(y_1,\dots , y_n) = a_1y_1^p+\dots + a_ny_n^p, \end{aligned}$$

and

$$\begin{aligned} D = \{y=(y_1,\dots , y_n) \in \mathbb {R}^n: \, y_k \ge 0 \text { for } 1 \le k \le n \text { and }\, y_1+\dots + y_n = 1\}. \end{aligned}$$

Then the minimum of f on D is attained at the point $y^0$ with

$$\begin{aligned} y_k^0 = a_k^{-1/(p-1)} \left( \sum _{j=1}^{n} a_j^{-1/(p-1)} \right) ^{-1} \end{aligned}$$

for $1 \le k \le n$, and

$$\begin{aligned} \min _{y \in D} f(y) = f(y^0) = \left( \sum _{k=1}^{n} a_k^{-1/(p-1)} \right) ^{-(p-1)}. \end{aligned}$$

Theorem 22

Let G be a hyperbolic uniform graph with a pole and some visual metric d on $\partial _\infty G$ and let $0<s\le \frac{1}{6}$ and $1<p<\infty$. If there exist two sequences of positive integers $\{\ell _n\}_{n=1}^\infty$ and $\{r_n\}_{n=1}^\infty$, with $r_n \ge 2$ for every $n \ge 1$,

$$\begin{aligned} \sum _{k=1}^{\infty } \frac{\ell _k}{(r_1\cdots r_k)^{2/(p-1)}} = \infty , \end{aligned}$$

and for every point $x\in \partial _\infty G$, the ball $B(x,2s^{L_{k-1}})$ contains at most $r_k$ points which are $s^{L_{k}}$-separated (where $L_0=0$ and $L_k=\sum _{j=1}^k\ell _j$), then G is p-parabolic.

Proof

Let $\Gamma$ be the truncated hyperbolic approximation of $(\partial _\infty G,d)$ with parameter s and let us denote by $d_\Gamma$ the usual minimal path distance in the graph $\Gamma$. Let us assume that the maximal integer k with ${{\,\textrm{diam}\,}}X<s^k$, $k_0$, satisfies $k_0\le 0$. Otherwise, it suffices to extend the truncated hyperbolic approximation considering $V_k$ to be a single point for every $0\le k <k_0$.

Let $S=\cup _{k\le 0} V_k$. By Proposition 8, $\partial _\infty G$ is compact. Therefore, S is finite.

For each $m\in \mathbb {N}$ let us consider a decreasing sequence $\{u_k\}_{k=0}^m$ with $1 =u_0$ and $u_m=0$ and define a function $u_m:V(\Gamma )\rightarrow \mathbb {R}$ such that:

• $u_m(w)=u_0=1$ for every $w\in V_k$ with $k\le 0$,

• $u_m(w)= u_{k}$ for every $w\in V_{L_k}$ (that is, for every $w\in \Gamma _v$ such that $d_\Gamma (v,w)=L_k=\sum _{j=1}^k\ell _j$),

• $u_m(w)=u_{k-1}-\frac{u_{k-1}-u_k}{\ell _k}i$ for every w such that $d_\Gamma (v,w)=L_{k-1}+i$ for every $1\le k\le m$ and every $1<i<\ell _k$,

• $u_m(w)=u_m=0$ for every w such that $d_\Gamma (v,w)\ge L_m=\sum _{j=1}^m \ell _j$.

As we saw above, $V_0$ is finite. For each fixed $v\in V_0$, let $\Gamma _v$ be the subgraph of $\Gamma$ induced by v and all the vertices in $\cup _{k>0}V_k$ which are connected to v by radial edges. Let $V^v_k:=V_k\cap \Gamma _v$.

Claim: ${{\,\mathrm{{\text {cap}}}\,}}_p (v,\Gamma _v)=0$.

Since each ball $B(x,2s^{L_{k-1}})$ contains at most $r_k$ points which are $s^{L_{k}}$-separated, then if $L_{k-1}<i\le L_k$, for each vertex w in $V_{i-1}$ there are at most $r_k$ vertices in $V_i$ connected to w by radial edges. Therefore, the set $V^v_i$ has at most $r_1\cdots r_k$ vertices and there are at most $(r_1\cdots r_k)^2$ radial edges between $V^v_{i-1}$ and $V^v_i$ (notice that the balls associated to the vertices in $V_{i-1}$ may all intersect and a vertex from $V_i$ can be joined by a radial edge to every vertex in $V_{i-1}$). Thus,

$$\begin{aligned}{} & {} \frac{1}{2} D_{p,\Gamma _v}(u_m)\le \frac{|u_0-u_1|^p}{\ell _1^p}r_1^2\ell _1+\cdots +\frac{|u_{m-1}-u_m|^p}{\ell _m^p}(r_1 \cdots r_m)^2\ell _m\\{} & {} \quad =\sum _{k=1}^m\frac{(r_1 \cdots r_k)^2|u_{k-1}-u_k|^p}{\ell _k^{p-1}}. \end{aligned}$$

By Lemma 21, with $a_k=(r_1 \cdots r_k)^2\ell _k^{-(p-1)}$, the minimum possible value of $\frac{1}{2} D_{p,\Gamma _v}(u_m)$ is

$$\begin{aligned} \left( \sum _{k=1}^{m} \left( (r_1 \cdots r_k)^2\ell _k^{-(p-1)}\right) ^{-1/(p-1)} \right) ^{-(p-1)}= \left( \sum _{k=1}^{m} \frac{\ell _k}{(r_1 \cdots r_k)^{2/(p-1)}} \right) ^{-(p-1)}. \end{aligned}$$

Therefore, if $\sum _{k=1}^{\infty } \frac{\ell _k}{(r_1 \cdots r_k)^{2/(p-1)}}=\infty$, it follows that ${{\,\mathrm{{\text {cap}}}\,}}_p(v,\Gamma _v)=0$.

Since $V_0$ is finite and, by the definition of $u_m$, the horizontal edges do not contribute to $D_{p,\Gamma }(u_m)$, it is immediate to see that ${{\,\mathrm{{\text {cap}}}\,}}_p(v,\Gamma )=0$. By propositions 12 and 13, $\Gamma$ is uniform. Hence, by Theorem 1, $\Gamma$ is p-parabolic.

Thus, by Theorem 11, G and $\Gamma$ are quasi-isometric uniform graphs, and by Proposition 2, G is p-parabolic. $\square$

Corollary 23

Let G be a hyperbolic uniform graph with a pole and some visual metric d on $\partial _\infty G$ and let $0<s\le \frac{1}{6}$ and $1<p<\infty$. If there exist a sequence of positive integers $\{\ell _n\}_{n=1}^\infty$ and a constant N such that

$$\begin{aligned} \lim _{k\rightarrow \infty }\frac{\ell _{k+1}}{\ell _k}>N^{\frac{2}{p-1}}, \end{aligned}$$

and for every point $x\in \partial _\infty G$, the ball $B(x,2s^{L_{k-1}})$ contains at most N points which are $s^{L_{k}}$-separated (where $L_0=0$ and $L_k=\sum _{j=1}^k\ell _j$), then G is p-parabolic.

Proof

Notice that

$$\begin{aligned} \lim _{k\rightarrow \infty }\frac{\ell _{k+1}}{\ell _k}>N^{\frac{2}{p-1}} \qquad \Leftrightarrow \qquad \lim _{k\rightarrow \infty }\frac{\frac{\ell _{k+1}}{N^{(2k+2)/(p-1)}}}{\frac{\ell _k}{N^{2k/(p-1)}}}>1, \end{aligned}$$

and by the D’Alembert criterion it follows that

$$\begin{aligned} \sum _{k=1}^{\infty } \frac{\ell _k}{N^{2k/(p-1)}} = \infty . \end{aligned}$$

$\square$

6 Parabolicity and Isoperimetric Inequality

From Theorem 19 and Theorem 24 bellow we can immediately prove Theorem 25. This could also be obtained from Theorem 1.1 in [4]

Definition 3

The combinatorial Cheeger isoperimetric constant of a graph G is defined to be

$$\begin{aligned} h(G) = \inf _U \frac{|\partial U|}{|U|} , \end{aligned}$$

where U ranges over all non-empty finite subsets of vertices in G, $\partial U = \{v \in G \, | \, d_{\,G}(v,U) = 1\}$ and |U| denotes the cardinality of the set U.

A graph G satisfies the (Cheeger) isoperimetric inequality if $h(G)>0$, since this means that

$$\begin{aligned} |U| \le h(G)^{-1} |\partial U| \end{aligned}$$

for every finite set of vertices U.

Let us recall the following from [38].

Definition 4

Given a metric space (X, d) and a constant $A>1$, we say that (X, d) is A-uniformly perfect if there exists some $\varepsilon _0>0$ such that for every $0<\varepsilon \le \varepsilon _0$ and every $x\in X$ there exist a point $y\in X$ such that $\varepsilon /A <d(x,y) \le \varepsilon$. We say that (X, d) is uniformly perfect if there exists some A such that (X, d) is A-uniformly perfect.

Theorem 24

[38, Theorem 5]. Given a uniform hyperbolic graph G, then $h(G)>0$ if and only if G is an infinite graph with a pole and $\partial _\infty G$ is uniformly perfect for some visual metric.

Theorem 25

Given a uniform hyperbolic graph G, if $h(G)>0$, then G is non-p-parabolic for every $1<p<\infty$.

Proof

Since $h(G)>0$, by Theorem 24, G has a pole. Also, since $\partial _\infty G$ is uniformly perfect for some visual metric, there exist $A>0$ and $\varepsilon _0>0$ such that ${{\,\textrm{diam}\,}}(B(z,\varepsilon ))>\frac{\varepsilon }{A}$ for any $z\in \partial _\infty G$ and any $0< \varepsilon <\varepsilon _0$. This means that $\partial _\infty G$ with that visual metric is locally perfect and, by Theorem 19, G is non-p-parabolic for every $1<p<\infty$. $\square$