The Most Beautiful Experiment in Physics

In May 1974 the Italian physicists Pier Giorgio Merli, Gian Franco Missiroli, and Giulio Pozzi (figure 1) submitted an article to the American Journal of Physics entitled “On the statistical aspect of electron interference phenomena,” which was published in March 1976.1 They obtained an interference pattern with an electron microscope that was fitted with a special interferometer, an electron biprism, that consisted basically of a very thin wire oriented perpendicularly to the electron beam and positioned symmetrically between two plates at ground potential, so that when a positive or negative potential was applied to the wire the electron beam was split into two deflected components. Their use of this electron biprism was the first important technical and conceptual feature of their experiment; the second was its ability to observe the continuous arrival of the electrons, one at a time, on a television monitor. Together with Lucio Morettini and Dario Nobili, the trio also produced a 16-millimeter movie entitled Interferenza di elettroni (Interference of Electrons) that was awarded first prize in the Physics Section of the VII Scientific and Technical Cinema Festival in Brussels in 1976.Footnote 1

Fig. 1
figure 1

Giulio Pozzi (b. 1945) and Gian Franco Missiroli (b. 1933), professors in the Department of Physics of the University of Bologna, Italy, and Pier Giorgio Merli (1943–2008), experts on electron microscopy as they appeared in the newspaper Sole 24 ore on September 6, 2003. Pozzi has done pioneering research in interferometry. Missiroli has been deeply engaged in educational research, which prompted him to initiate the work that led to the Merli–Missiroli–Pozzi experiment. Merli was President of the Italian Society of Electron Microscopy from 1984 to 1987 and Director of the LAMEL-CNR Institute in Bologna from 1992 to 1998; he died on February 24, 2008. Credit: Photograph by Pino Guidolotti

Full size image

Twenty-six years later, in September 2002, Physics World published the results of a survey in which readers were asked to name the most beautiful experiment in physics of all time. They voted the following experiments as the top ten: (1) Young’s double-slit experiment applied to the interference of single electrons; (2) Galileo’s experiment on falling bodies (1600s); (3) Millikan’s oil-drop experiment (1910s); (4) Newton’s decomposition of sunlight with a prism (1665–1666); (5) Young’s light-interference experiment (1801); (6) Cavendish’s torsion-bar experiment (1798); (7) Eratosthenes’s measurement of the Earth’s circumference (3rd century BC); (8) Galileo’s experiments with rolling balls down inclined planes (1600s); (9) Rutherford’s discovery of the nucleus (1911); and (10) Foucault’s pendulum (1851).2 Historian-philosopher Robert P. Crease, who proposed the survey, commented that:

The double-slit experiment with electrons possesses all of the aspects of beauty most frequently mentioned by readers…. It is transformative, being able to convince even the most die-hard sceptics of the truth of quantum mechanics…. It is economical: the equipment is readily obtained and the concepts are readily understandable, despite its revolutionary result. It is also deep play: the experiment stages a performance that does not occur in nature, but unfolds only in a special situation set up by human beings. In doing so, it dramatically reveals–before our very eyes–something more than was put into it.3

In sketching the historical background to this beautiful experiment, Peter Rodgers, Editor of Physics World, asked:

[Who] actually carried out the experiment? Standard reference books offer no answer to this question but a search through the literature does reveal several unsung experimental heroes.4

Rodgers’s list of unsung heroes began with Geoffrey Ingram Taylor, who in 1909 obtained interference fringes using a light source that was so weak that only very few “indivisible units” (later, photons) struck a photographic plate.5 Nearly a half-century later, in 1955, Gottfried Möllenstedt and Heinrich Düker used their invention of the electron biprism to obtain interference fringes with an electron microscope,6 and six years after that Claus Jönsson carried out electron-interference experiments with up to five slits of width 3 × 10−7 meter.7 Rodgers failed to mention Merli, Missiroli, and Pozzi’s 1974 experiment, but he did call special attention to the

milestone … experiment in which there was just one electron in the apparatus at any one time [which was carried out] by Akira Tonomura and co-workers at Hitachi in 1979 [sic, 1989] when they observed the build-up of the fringe pattern with a very weak electron source and an electron biprism.8

Tonomura and his coworkers carried out their experiment at the Advanced Research Laboratory in Hitachi, Tokyo, and published their 1989 paper in the American Journal of Physics. 9 In it they gave the impression that they were the first to demonstrate the formation of interference fringes by single electrons.

Rodgers’s account was challenged eight months later, in May 2003, by John Steeds, at that time Head of the Department of Physics at the University of Bristol, who had seen a preliminary version of Merli, Missiroli, and Pozzi’s 1976 movie. As Steeds wrote in a Letter to the Editor of Physics World:

I believe that the first double-slit experiment with single electrons was performed by Pier Giorgio Merli, Gian Franco Missiroli and Giulio Pozzi in Bologna in 1974–some 15 years before the Hitachi experiment. Moreover, the Bologna experiment was performed under very difficult experimental conditions: the intrinsic coherence of the thermionic electron source used by the Bologna group was much lower than that of the field-emission source in the Hitachi experiment.10

Merli, Missiroli, and Pozzi themselves then pointed out in a Letter to the Editor of Physics World that Tonomura and his coworkers did not cite their 1976 paper in the American Journal of Physics,11 as Greyson Gilson had already noted after the publication of the Hitachi group’s paper in 1989.12 The Italian trio further pointed out that Tonomura and his coworkers included only an incorrect reference to their 1976 movie, and did not even mention that it shows the arrival of single electrons, one after the another, on their television monitor. The referees of the Hitachi group’s 1989 paper were evidently unaware that Merli, Missiroli, and Pozzi’s paper also had been published in the American Journal of Physics thirteen years earlier.

The Hitachi version of the experiment was indeed excellent,13 but in 2003 Akira Tonomura was still reluctant to grant Merli, Missiroli, and Pozzi priority, as indicated in his reply to Steeds’s Letter to the Editor of Physics World:

We believe that we carried out the first experiment in which the build-up process of an interference pattern from single electron events could be seen in real time as in Feynman’s famous double-slit Gedanken experiment. This was under the condition, we emphasize, that there was no chance of finding two or more electrons in the apparatus.14

American physicist Mark P. Silverman, who was personally involved in the Hitachi experiment, based his discussion of electron interference exclusively on that experiment in his 1993 and 1995 books,15 making no reference to Merli, Missiroli, and Pozzi’s much earlier experiment, although he acknowledged that:

The Hitachi experiment is not the first of its kind (although it was the first I had personally witnessed), but rather one of the last and most conclusive in a line of analogous experiments dating back to just a few years after Einstein proposed the existence of photons.16

There can be no doubt, however, that Merli, Missiroli, and Pozzi carried out the first conclusive double-slit single-electron interference experiment.

I have dwelled on this question of priority not for parochial reasons, but to emphasize the vital role of experiment in the history of science. Philosopher Ian Hacking, for example, criticized philosophers who “[by] legend and perhaps by nature … are more accustomed to the armchair than the workbench,”17 and hence reflect “the standard preference for hearing about theory rather than experiment.”18 According to Hacking:

History of the natural sciences is now almost always written as a history of theory. Philosophy of science has so much become philosophy of theory that the very existence of pre-theoretical observations or experiments has been denied.19

My aim is to help rectify this alleged imbalance.

The Merli–Missiroli–Pozzi Experiment

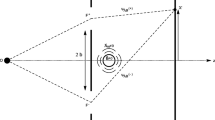
Merli, Missiroli, and Pozzi have provided a complete description of their experimental apparatus,20 as shown in figure 2. S is the effective electron source, in other words, not the real source of the electrons, which are emitted thermionically by a hot filament about 36 centimeters above S, and by means of a system of condenser lenses are focused on an area whose diameter can be reduced to approximately 6 millimeters, thus effectively making S a monochromatic point source of electrons. The biprism wire F (radius r = 2 × 10−7 meter) is at a distance a = 10 centimeters below S and is 2 millimeters away from each of two opposing plates at ground potential. When a voltage V is applied to the biprism wire, an electric field is produced that is equivalent to one produced by a cylindrical condenser of external radius R (slightly smaller than the distance between the two opposing plates) and internal radius r. Merli, Missiroli, and Pozzi showed that when an electron of charge e, mass m, and speed v 0 passes the biprism wire F at a distance x away from it, it will be deflected through an angle α given by:21

Fig. 2
figure 2

Schematic diagram (not to scale) of Merli, Missiroli, and Pozzi’s electron-biprism experimental apparatus. Electrons emerge as if from the effective source S (or from the virtual sources S 1 and S 2), are diffracted by the biprism wire F when at a potential V, and interfere inside the region W on the observation plane OP or strike it as particles outside of it. The system of lenses represented by the Ls magnify the image on the observation plane OP onto the viewing plane VP. Source: Adapted from Merli, Missiroli, and Pozzi, “Diffrazione e interferenza” (ref. 23), p. 87, Fig. 3

Full size image
$$ \alpha = \frac{2eV}{{mv_{0}^{2} \ln \left( {2/R} \right)}}\tan^{ - 1} \frac{{\left( {R^{2} - x^{2} } \right)^{1/2} }}{x}.$$

If the voltage V is positive (converging biprism), the electron will be deflected toward the wire; if V is negative (diverging biprism), it will be deflected away from the wire.

In the overlapping (hatched) region, Merli, Missiroli, and Pozzi state that “a non-localized interference pattern will be produced,”22 non-localized because the interference pattern spans the entire overlapping region, but to see it a fluorescent screen, for example, must be placed in the observation plane OP at a distance b below the biprism wire, where fringes are formed in the interference field of width W given by:

$$ W = 2\left| {\frac{a + b}{a}} \right|\,\left( {\alpha \frac{ab}{a + b} - r} \right). $$

Inserting numbers, with α = 5 × 10−5 radian, r = 2 × 10−7 meter, a = 10 centimeters, and b = 24 centimeters,23 it follows that W = 23 × 10−6 meter. To make the fringes on the observation plane OP visible, however, a system of lenses represented by the Ls is required to enlarge them (240 times), which enables them to be seen on the viewing plane VP with the naked eye or on a television monitor or to be recorded on a photographic plate.24

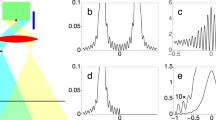
As noted above, Möllenstedt and Düker reported experiments in which they obtained interference fringes using an electron microscope;25 Merli, Missiroli, and Pozzi replaced their photographic plate with an image intensifier, which converts an electronic image into an optical image that is 200 times brighter than the image that would be seen on a fluorescent screen in the observation plane OP. The optical image is then transmitted by optic fibers to the photocathode of a SEC (Secondary Electron Conduction) tube that is connected through a video amplifier and control unit to a television monitor. The SEC tube can retain electrostatic charges for a relatively long period of time even after the electron beam has been switched off, which permits the observation of extremely low intensities (one electron at a time) for as long as it takes for the image to be formed. The shortest storage time that Merli, Missiroli, and Pozzi achieved with their image intensifier was 0.04 second,Footnote 2 which enabled them to operate with such low electron-current densities that only one electron, or very few electrons, were seen as one or more tiny white dots on their television monitor. Then, by increasing the storage time to on the order of minutes, electrons striking certain areas of the viewing plane VP could be seen arriving one at a time, so that fringes began to appear after the arrival of thousands of electrons. In this way, Merli, Missiroli, and Pozzi saw, for the first time, the formation of electron-interference fringes with increasing electron-current densities, as shown in figure 3.26 Every electron hits the television monitor at a precise spot, like a particle, as revealed by the dot of light it produces, but the cumulative behavior of many electrons (even when they are transferred one by one from the emitting filament to the television monitor) shows a wave-like pattern.

Fig. 3
figure 3

The formation of electron-interference fringes inside the interference region W and particle dots outside it with increasing electron-current densities as seen on a television monitor in the viewing plane VP. Source: Merli, Missiroli, and Pozzi, “On the statistical aspect” (ref. 1), p. 306, Fig. 1

Full size image

As indicated above, if we know the angle of deflection α of an electron emitted from the effective source S and then passes the biprism wire F, we can calculate its point of arrival on the observation plane OP. However, the computed distribution of many such points is not identical to the distribution of the electrons that we actually observe; in other words, the computed distribution does not reproduce the fringes in the interference field W on the observation plane OP. To reproduce these fringes, we have to introduce the electron’s wave behavior; we have to introduce de Broglie waves. To do so, note that the system illustrated in figure 2 is equivalent to a Fresnel optical biprism: It is as if the electrons were emitted from two virtual point sources, S 1 and S 2, positioned symmetrically on each side of, and in the same plane as the effective source S. The separation of the two virtual sources is d = 2αa, so that by introducing the de Broglie wavelength λ, we find that the fringes in the interference field W have a periodicity l = λ(a + b)/d. This optical analogy is useful for understanding the parameters in the Merli–Missiroli–Pozzi experiment, but I should note that other models also have been proposed, some more complex than others, in which quantum–mechanical equations are used directly to explain the observed phenomena.27

Three Comments

I first note that Merli, Missiroli, and Pozzi, when discussing the technical specifications and operation of their image intensifier in their 1976 article,28 cite a paper that K.H. Hermann and his coworkers presented at the International School of Electron Microscopy in Erice, Sicily, in April 1970,29 which Merli and Pozzi also attended. In it Hermann and his coworkers illustrated a number of experiments using a Siemens image intensifier that showed the formation of Fresnel interference fringes when an electron-current density of 10−15 amperes per square centimeter passed through a tiny hole in a carbon film,30 so that with a storage time of 0.04 second “only the signals of individual electrons are visible.”31 Then, by increasing the storage time up to 120 seconds, they observed directly how the fringes took shape.32 Their experiments were designed mainly to show the technical potential of the Siemens image intensifier, and as such were of interest only to electron microscopists; the broader scientific community failed to grasp their fundamental physical importance. Nonetheless, they were of substantial influence on Merli, Missiroli, and Pozzi’s later single-electron experiments, as they themselves pointed out.33

I note, secondly, that the electron-biprism experiment differs in important respects from a traditional double-slit experiment. In the former, there are no real slits, and both the wave and the particle natures of the electron are observed in the same experiment. The statement that “the electron passed through slit 1 (or 2)” is replaced by the statement that “the electron passed to the left (or right) of the wire” or, in the optical analogy, that “the electron was emitted by the virtual source S 1 (or S 2).” Interference fringes form only in the overlapping region W of the observation plane OP, which contains electrons that passed on both sides of the wire. The equation for the angle of deflection α does not envision the formation of interference fringes on the observation plane OP inside the interference field W; it predicts the point of arrival of one electron outside of the interference field W. More precisely, the observation plane OP contains a region A within which the electrons deflected by the biprism’s wire arrive; within region A is the region W in which the interference pattern forms. Electrons continue to arrive outside W, and their angles of deflection and hence trajectories can be calculated. The broader region A can be enlarged onto the viewing plane VP, and using the image intensifier it can be observed on the television monitor. Note, in fact, that Merli, Missiroli, and Pozzi’s photographic images clearly show, as seen in figure 3, a number of white dots produced by electrons that have been deflected outside of the region W in which the interference fringes are formed. Today, the width W of the interference region is routinely set, thus leaving a region outside it in which one can think in terms of classical particle trajectories. In the single-electron experiment, if an electron arrives at a point x = P 1 – ε (where ε is the experimental limit of resolution), we may say that it passed to the left of the biprism wire, that is, its trajectory is perfectly specified; if, however, it arrives at a point x = P 1 + ε, then its trajectory (if it now even makes sense to use this term) cannot be specified. This highlights the point that, in the same experiment, a transition takes place continuously, as it were, from its description in classical terms to its description in quantum terms.

I note, finally, that concerning the option of observing the electron either within or outside the interference region W after it has interacted with the biprism wire, when we establish its potential V, the width of region W depends on the distance b, which we can choose after the electron has passed the biprism wire. Thus, we can choose the width of the interference region W in which the electron reveals its wave-like nature after it has interacted with the biprism wire. This experimental variation, although yet to be tested, is reminiscent of the “delayed choice” that John Archibald Wheeler proposed in 1977 in a Gedanken experiment.34

A Crucial Experiment

Merli, Missiroli, and Pozzi’s experiment was a crucial experiment because it demonstrated empirically that electrons are not (only) waves, and not (only) particles. It also is a paradigmatic exemplar of the frequentist interpretation of probability. Thus, from an operational point of view, to determine the probability of an electron reaching a given point x on a screen means counting the number of electrons within a radius of, say, dx around x, relative to the total number of electrons that reached the screen. This count is performed, for example, by using a microdensitometer to measure the blackening of a photographic film in a direction perpendicular to the interference fringes to obtain an intensity curve like the one shown in figure 4.35 According to Merli, Missiroli, and Pozzi:

Fig. 4
figure 4

The intensity curve of the electron-interference fringes as measured by a microdensitometer to record the blackening of a photographic plate in the viewing plane VP. Source: Merli, Missiroli, and Pozzi, “Diffrazione e interferenza” (ref. 23), p. 97, Fig. 10

Full size image

This curve, which is familiar to us from the study of the intensity resulting from the interference of two wave-like perturbations, in this case indicates the number n of electrons that have hit the various regions of the photographic plate. Thus, if N is the total number of incident electrons, the curve enables us to derive the fraction of them that is distributed in the various different positions. If this curve refers to a single electron, then it will show the probability the electron has of arriving at one point rather than at another.36

Merli, Missiroli, and Pozzi thus clearly support a frequentist interpretation of probability.

That the single-electron experiment demonstrates that the interference pattern results from the accumulation of single events, as for example in the case of a Gaussian distribution, seems to lend support to philosopher Karl R. Popper’s claim that:

[What] I call the great quantum muddle consists in taking a distribution function, i.e. a statistical measure function characterizing some sample space (or perhaps some “population” of events), and treating it as a physical property of the elements of the population. It is a muddle: the sample space has hardly anything to do with the elements.37

Popper’s muddle thus consists in mistaking the physical properties of the elements in a statistical distribution for its distributive properties. Thus, in the single-electron double-slit experiment, the muddle is that because the observed distribution is the same as that of light in optical-interference experiments, this reflects the nature of the electrons producing the distribution. This, in turn, means admitting the existence of a real wave (or wave packet) of a known physical entity, that is, an electromagnetic wave, which in some way is linked to the electron. The formation of fringes thus could be explained if we hypothesize that the electron reveals: (a) its particle nature during emission; (b) its wave nature in the experimental apparatus; and (c) its particle nature again at the screen. This hypothesis cannot apply to the single-electron double-slit experiment, however. As Merli, Missiroli, and Pozzi wrote:

The fringes of interference (and of diffraction) are not due to the fact that the electron is spatially distributed in a continuous manner and becomes a wave (in fact, if this had been the case we would have had fringes of decreasing intensity as the current decreased).38

Instead, as the intensity of the electron-beam current was reduced, the number of electrons reaching the screen in a given interval of time also fell.

In the Merli–Missiroli–Pozzi experiment, the events are independent of each other because only one electron at a time passes through the biprism: On average, the electrons are separated from each other by 10 meters,39 which means that a given electron hits the screen after the preceding electron had been absorbed in it. I emphasize that this aspect of their experiment, which they achieved for the first time, is of crucial importance because, first and foremost, it excludes the possibility that the fringes were in some way produced by an interaction of the electrons inside the biprism apparatus. It also excludes the possibility that such an interaction occurred in the photographic plate or other detector.

By contrast, Patrick Suppes and Jose Acacio de Barros explained the interference and diffraction of photons on the basis of the following hypotheses:

(i) Photons are emitted by harmonically oscillating sources. (ii) They have definite trajectories. (iii) They have a probability of being scattered at a slit. (iv) Detectors, like sources, are periodic. (v) Photons have positive and negative states which locally interfere, i.e., annihilate each other, when being absorbed.40

The Merli–Missiroli–Pozzi experiment proves that, for electrons, there cannot be any kind of destructive interference involving the detector, because they never interact on their journey to, or arrival at the detector. Further, Suppes and Acacio de Barros assumed “that the absorber, or photodetector, itself behaves periodically with a frequency ω,”41 but in the Merli–Missiroli–Pozzi experiment the absorber is a well-defined macroscopic device, a photographic plate or an image intensifier, which is totally devoid of periodic oscillations. Moreover, the source of electrons in it is an image of very small diameter produced by a lens system that collects the electrons after they have been emitted thermionically by an incandescent point filament—which involves no periodicity whatsoever. In any case, since the probability of two or more electrons being present simultaneously between the source and detector is negligible, they experience no significant interaction at any time in the entire apparatus.

Suppes and Acacio de Barros, of course, focused on photons, not electrons. Indeed, the Berlin experimentalists Gerhard Simonsohn and Ernst Weihreter pointed out that in double-slit experiments the similarity between photons and electrons, although frequently noted, is valid “only in a restricted sense.”42 Nevertheless, Merli, Missiroli, and Pozzi’s experiment disproved empirically that Suppes and Acacio de Barros’s hypotheses cannot apply to electrons. The Italian trio developed and described all of its technical details in such a way as to leave no room for ambiguity or for any ad hoc hypotheses that cannot be tested experimentally. Therefore, their experiment, which is a real experiment, should be borne in mind when new hypotheses are advanced on the basis of Gedanken experiments involving either electrons or photons.

Philosophical Implications

The two-slit experiment is central to interpretations of quantum mechanics. Albert Einstein and Niels Bohr often focused on it in their long debate over the completeness of quantum mechanics beginning in 1927.43 Much later, in the 1990s, the question of whether Werner Heisenberg’s uncertainty relations derive from Bohr’s principle of complementarity, or vice versa, arose,44 and philosophers entered the debate: Suppes and Acacio de Barros, as we have seen, derived the phenomena of photon interference and diffraction on the basis of certain hypotheses on their emission, absorption, and interaction;45 Arthur Fine argued that the two-slit experiment, when analyzed correctly, confirms the validity of the “classical” theory of probability even in the microworld;46 Karl R. Popper argued that it leads to a new interpretation of probability that is connected ontologically to the introduction of a new physical property, propensity;47 and Peter Milne argued that it provides proof of the inadequacy of such proposals.48 In general, the Merli–Missiroli–Pozzi experiment, which today can be carried out with microscopic objects (electrons, photons, neutrons, and atoms) and with mesoscopic systems such as fullerene molecules,49 did not prompt any fundamental rethinking of the interpretation of quantum mechanics, but I shall argue that it should have engendered philosophical reflection and debate.

In 1970 Leslie E. Ballantine published a classical article on the statistical interpretation of quantum mechanics in which he treated the wave function not as a physical entity, but simply as a mathematical device for calculating probability; the wave-like pictures are epiphenomena produced by the impacts of particles.50 Merli, Missiroli, and Pozzi’s single-electron experiment would seem to support Ballantine’s view, at least at first glance: The observed image that gradually appears on the television monitor is produced by single electrons, and after a sufficient number of them appear their probability distribution is the same as that of the intensity of light in a corresponding optical experiment. Still required, however, was a physical explanation for the behavior of the particles that give rise to these images, for which supporters of the statistical interpretation leaned on the Duane-Landé theory of interference and diffraction.51

Thus, in 1923 William Duane attempted to explain the diffraction of X rays in crystals by introducing a third quantum rule, one for linear momentum, according to which a crystal with a periodicity l in a certain direction can change its momentum p in this direction by an amount Δp = h/l, where h is Planck’s constant. Four decades later, in 1965, Alfred Landé, by taking the law of conservation of momentum into account, used Duane’s rule to derive the Bragg law of X-ray diffraction. He argued that:

The incident particles do not have to spread like waves…; they stay particles all the time. It is the crystal with its periodic lattice planes which is already spread out in space and as such reacts under the third quantum rule.52

Landé extended this reasoning to an ideal double-slit experiment, concluding that the slit screen reacts to electrons incident on it as a mechanical unit, a “whole solid body,” in such a way that it transfers quantized momentum to the electrons, the collective action of which results in their interference behavior.53 The interference of the electrons therefore is not due to a quality inherent in them, but to the quantum-mechanical activity of the diffractor, such as a crystal or a screen with two slits in it.

The Duane-Landé theory, however, is not capable of explaining the results of the Merli–Missiroli–Pozzi experiment, because the interference image in it is obtained with no mechanical transfer of momentum to or from the biprism apparatus. In fact, its “slits” are only virtual slits, and there is nothing mechanical about the formation of the interference fringes. As members of the Bologna group, in describing an electron-biprism interference experiment (this time not with single electrons), wrote in 1973:

In interference experiments it is not necessary to introduce the concepts of interaction between electrons and atoms, regular distribution of atoms in crystalline lattice[s], their dimensions, etc., as for diffraction experiments, but the splitting and superposition of the electron beam is achieved by macroscopic fields without any interaction of the electron with the material. 54

The Merli–Missiroli–Pozzi experiment demonstrates, in fact, that although at first sight it seems to support the statistical interpretation of quantum mechanics, its detailed experimental arrangement proves that the opposite is true, since to explain wave-particle dualism the statistical interpretation invariably has to resort to a model based on a transfer of mechanical momentum.

In 1999 Ballantine, explicitly referring to the single-electron experiment (the one conducted by the Hitachi group), advanced two explanations for the wave-like behavior of electrons, one based on the wave-particle duality, the other on the “quantized momentum transfer to and from” a periodic object like a crystal lattice.55 As in his 1970 article, he considered the latter explanation to be simpler because it does not appeal to any hypotheses about the wave-like nature of the electron, and he therefore employed Occam’s razor to prefer it. Regarding Popper’s propensity interpretation of probability,56 the problem basically comes down to the necessity to resolve the connection between the meaning of the probability of a single event and the relative frequency of its probability.

Despite the absence of any explicit reference to these philosophical problems, Merli, Missiroli, and Pozzi clearly revealed the tension between the necessity of assigning to an individual electron the probability it has of reaching a given point on a photographic plate, and the necessity of acknowledging interference fringes as a statistical distribution of relative frequencies.57 Moreover, they emphasized that interference must be perceived as resulting from the interaction of a single electron within the experimental apparatus, that is, of the “generating conditions” underlying the intensity distribution:

[The] electron is a particle that reaches a clearly identifiable point on the screen, exposing a single grain of the photographic emulsion, and the interference pattern is the statistical result of a large number of electrons….

Thus we may conclude that the phenomenon of interference is exclusively the consequence of the interaction of the individual electron within the experimental apparatus. 58

In short, in the Merli–Missiroli–Pozzi experiment the observed system is a single electron, and its result is the product of single events. Probability thus has to be assigned to a single event.

I stress, finally, that the crucially important feature of the Merli–Missiroli–Pozzi experiment consists essentially in showing the empirical meaning of the probability of a single event within the experimental context of quantum mechanics. In microphysical experiments, we check, for example, whether or not a statistical distribution conforms to theoretical expectations, so frequencies themselves are seen as the sole constituents of probability. In the single-electron experiment, this is turned on its head. The focus now is on the individual particle, in that there are empirical grounds for enquiring about the probability that a single electron will reach a certain point on a screen after the arrival of the preceding electron, even after the apparatus has been switched off. The Merli–Missiroli–Pozzi experiment excludes the possibilities that the interference fringes are due to (i) a real (electromagnetic) wave (or wave packet) that is in some way associated with the electron, (ii) the interaction between one electron and another electron, (iii) any specific characteristics of the electron source, and (iv) to a transfer of momentum from the slit screen to the electron. The only remaining explanation is to regard probability as a physical property that is revealed in the single-electron case. In sum, the Merli–Missiroli–Pozzi experiment may be particularly significant philosophically in regard to the role of probability in quantum mechanics.

Postscript

Pier Giorgio Merli, Gian Franco Missiroli, and Giulio Pozzi never received any official award from the University of Bologna, from the Italian Research Council (Consiglio Nazionale delle Ricerche, CNR), or from any Italian civic or scientific institution, although they brought great credit to all of these institutions.Footnote 3 However, after Merli’s death in February 2008, some of his friends established the website <http://l-esperimento-piu-bello-della-fisica.bo.imm.cnr.it/english/index.html>, where anyone can learn how the Merli–Missiroli–Pozzi experiment was constructed and performed, and that it “also aims at clarifying the scientific and personal motivations and conditions which allowed the team of Italian physicists to perform the experiment successfully, giving a brilliant contribution to fundamental research in the field of physics.” One also can hear Giulio Pozzi explain how the thin biprism wire was prepared. Giorgio Lulli (lulli@bo.imm.cnr.it) supervises the website and is prepared to answer questions about the experiment. He also organized a project to produce a remastered version of the original film, Interferenza di elettroni, on a DVD as well as a documentary film (directed by Dario Zanasi and Diego L. Gonzalez) on the Merli–Missiroli–Pozzi experiment that shows the scientific, historical, and human factors involved in its realization. Giorgio Matteucci has described and reproduced subsequent electron experiments performed by the Bologna group,59 including ones analogous to the optical experiments performed in 1818 that showed the existence of Fresnel zones and the Poisson spot.