1 Introduction

Advanced LIGO (aLIGO) [61, 9] and Advanced Virgo (AdV) [24, 23, 25] are kilometer-scale gravitational-wave (GW) detectors that are expected to yield direct observations of GWs. In this article we describe the currently projected schedule, sensitivity, and sky-localization accuracy for the GW-detector network. We discuss the proposed sequence of observing runs (designated O1, O2, O3, etc.) and the prospects for multi-messenger astronomy.

The purpose of this article is to provide information to the astronomy community to assist in the formulation of plans for the upcoming era of GW observations. In particular, we intend this article to provide the information required for assessing the features of programs for joint observation of GW events using electromagnetic, neutrino, or other facilities.

The full science of aLIGO and AdV is broad [8], and is not covered in this article. We concentrate solely on candidate GW transient signals. We place particular emphasis on the coalescence of binary neutron-star (BNS) systems, which are the GW source for which electromagnetic follow-up seems most promising. For more general introductory articles on GW generation, detection and astrophysics, we point readers to [33, 87, 94].

Although our collaborations have amassed a great deal of experience with GW detectors and analysis, it is still difficult to make predictions for both improvements in search methods and for the rate of progress for detectors which are not yet fully installed or operational. The scenarios of LIGO and Virgo detector sensitivity evolution and observing times given here represent our best estimates as of January 2016. They should not be considered as fixed or firm commitments.

As the detectors’ construction and commissioning progress, we intend to release updated versions of this article. This is the second version of the article, written to coincide with the first observing run (O1) of the advanced-detector era. Changes with respect to the first version [4] are given in Appendix A. Progress has been made in the commissioning of the detectors, and the plausible observing scenarios are largely the same; the predicted sky-localization accuracies have been updated following improvements in parameter estimation.

2 Commissioning and Observing Phases

We divide the development of the aLIGO and AdV observatories into three components:

  • Construction includes the installation and testing of the detectors. This phase ends with acceptance of the detectors. Acceptance means that the interferometers can lock for periods of hours: light is resonant in the arms of the interferometer with no guaranteed GW sensitivity. Construction incorporates several short engineering runs with no astrophysical output as the detectors progress towards acceptance. The aLIGO construction project ended (on time and on budget) in March 2015. The acceptance of AdV is expected in the first part of 2016.

  • Commissioning takes the detectors from their configuration at acceptance through progressively better sensitivity to the design advanced-generation detector sensitivity. Engineering runs in the commissioning phase allow us to understand our detectors and analyses in an observational mode; these are not intended to produce astrophysical results, but that does not preclude the possibility of this happening. Rather than proceeding directly to design sensitivity before making astrophysical observations, commissioning is interleaved with observing runs of progressively better sensitivity.

  • Observing runs begin when the detectors have reached (and can stably maintain) a significantly improved sensitivity compared with previous operation. It is expected that observing runs will produce astrophysical results, including upper limits on the rate of sources and possibly the first detections of GWs. During this phase, exchange of GW candidates with partners outside the LIGO Scientific Collaboration (LSC) and the Virgo Collaboration will be governed by memoranda of understanding (MOUs) [17, 2]. After the first four detections, we expect free exchange of GW event candidates with the astronomical community and the maturation of GW astronomy.

The progress in sensitivity as a function of time will affect the duration of the runs that we plan at any stage, as we strive to minimize the time to successful GW observations. Commissioning is a complex process which involves both scheduled improvements to the detectors and tackling unexpected new problems. While our experience makes us cautiously optimistic regarding the schedule for the advanced detectors, we are targeting an order of magnitude improvement in sensitivity relative to the previous generation of detectors over a wider frequency band. Consequently, it is not possible to make concrete predictions for sensitivity or duty cycle as a function of time. We can, however, use our experience as a guide to plausible scenarios for the detector operational states that will allow us to reach the desired sensitivity. Unexpected problems could slow down the commissioning, but there is also the possibility that progress may happen faster than predicted here. As the detectors begin to be commissioned, information on the cost in time and benefit in sensitivity will become more apparent and drive the schedule of runs. More information on event rates, including the first detection, could also change the schedule and duration of runs.

In Section 2.1 we present the commissioning plans for the aLIGO and AdV detectors. A summary of expected observing runs is in Section 2.2.

2.1 Commissioning and observing roadmap

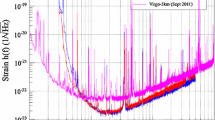
The anticipated strain sensitivity evolution for aLIGO and AdV is shown in Figure 1. A standard figure of merit for the sensitivity of an interferometer is the BNS range RBNS: the volume- and orientation-averaged distance at which a compact binary coalescence consisting of two 1.4 M⊙ neutron stars gives a matched filter signal-to-noise ratio (SNR) of 8 in a sing le detector [58].Footnote 1 The BNS ranges for the various stages of aLIGO and AdV expected evolution are also provided in Figure 1.

Figure 1
figure 1

aLIGO (left) and AdV (right) target strain sensitivity as a function of frequency. The binary neutron-star (BNS) range, the average distance to which these signals could be detected, is given in megaparsec. Current notions of the progression of sensitivity are given for early, mid and late commissioning phases, as well as the final design sensitivity target and the BNS-optimized sensitivity. While both dates and sensitivity curves are subject to change, the overall progression represents our best current estimates.

Full size image

The commissioning of aLIGO is well under way. The original plan called for three identical 4-km interferometers, two at Hanford (H1 and H2) and one at Livingston (L1). In 2011, the LIGO Lab and IndIGO consortium in India proposed installing one of the aLIGO Hanford detectors (H2) at a new observatory in India (LIGO-India) [64]. As of early 2015, LIGO Laboratory has placed the H2 interferometer in long-term storage for possible use in India. Funding for the Indian portion of LIGO-India is in the final stages of consideration by the Indian government.

Advanced LIGO detectors began taking sensitive data in August 2015 in preparation for the first observing run. O1 formally began 18 September 2015 and ended 12 January 2016. It involved the H1 and L1 detectors; the detectors were not at full design sensitivity. We aimed for a BNS range of 40–80 Mpc for both instruments (see Figure 1), and both instruments were running with a 60–80 Mpc range. Subsequent observing runs will have increasing duration and sensitivity. We aim for a BNS range of 80–170 Mpc over 2016–2018, with observing runs of several months. Assuming that no unexpected obstacles are encountered, the aLIGO detectors are expected to achieve a 200 Mpc BNS range circa 2019. After the first observing runs, circa 2020, it might be desirable to optimize the detector sensitivity for a specific class of astrophysical signals, such as BNSs. The BNS range may then become 215 Mpc. The sensitivity for each of these stages is shown in Figure 1.

As a consequence of the planning for the installation of one of the LIGO detectors in India, the installation of the H2 detector has been deferred. This detector will be reconfigured to be identical to H1 and L1 and will be installed in India once the LIGO-India Observatory is complete. The final schedule will be adopted once final funding approvals are granted. If project approval comes soon, site development could start in 2016, with installation of the detector beginning in 2020. Following this scenario, the first observing runs could come circa 2022, and design sensitivity at the same level as the H1 and L1 detectors is anticipated for no earlier than 2024.

The time-line for the AdV interferometer (V1) [23] is still being defined, but it is anticipated that in 2016 AdV will join the aLIGO detectors in their second observing run (O2). Following an early step with sensitivity corresponding to a BNS range of 20–60 Mpc, commissioning is expected to bring AdV to a 60–85 Mpc in 2017–2018. A configuration upgrade at this point will allow the range to increase to approximately 65–115 Mpc in 2018–2020. The final design sensitivity, with a BNS range of 130 Mpc, is anticipated circa 2021. The corresponding BNS-optimized range would be 145 Mpc. The sensitivity curves for the various AdV configurations are shown in Figure 1.

The GEO 600 [76] detector will likely be operational in the early to middle phase of the AdV and aLIGO observing runs, i.e. 2015–2017. The sensitivity that potentially can be achieved by GEO in this time-frame is similar to the AdV sensitivity of the early and mid scenarios at frequencies around 1 kHz and above. GEO could therefore contribute to the detection and localization of high-frequency transients in this period. However, in the ∼ 100 Hz region most important for BNS signals, GEO will be at least 10 times less sensitive than the early AdV and aLIGO detectors, and will not contribute significantly.

Japan has begun the construction of an advanced detector, KAGRA [100, 28]. KAGRA is designed to have a BNS range comparable to AdV at final sensitivity. We do not consider KAGRA in this article, but the addition of KAGRA to the worldwide GW-detector network will improve both sky coverage and localization capabilities beyond those envisioned here [96].

Finally, further upgrades to the LIGO and Virgo detectors, within their existing facilities (e.g., [63, 78, 11]) as well as future underground detectors (for example, the Einstein Telescope [93]) are envisioned in the future. These affect both the rates of observed signals as well as the localizations of these events, but this lies beyond the scope of this paper.

2.2 Envisioned observing schedule

Keeping in mind the important caveats about commissioning affecting the scheduling and length of observing runs, the following is a plausible scenario for the operation of the LIGO-Virgo network over the next decade:

  • 2015–2016 (O1) A four-month run (beginning 18 September 2015 and ending 12 January 2016) with the two-detector H1L1 network at early aLIGO sensitivity (40–80 Mpc BNS range).

  • 2016–2017 (O2) A six-month run with H1L1 at 80–120 Mpc and V1 at 20–60 Mpc.

  • 2017–2018 (O3) A nine-month run with H1L1 at 120–170 Mpc and V1 at 60–85 Mpc.

  • 2019+ Three-detector network with H1L1 at full sensitivity of 200 Mpc and V1 at 65–115 Mpc.

  • 2022+ H1L1V1 network at full sensitivity (aLIGO at 200 Mpc, AdV at 130 Mpc), with other detectors potentially joining the network. Including a fourth detector improves sky localization [72, 109, 79, 91], so as an illustration we consider adding LIGO-India to the network. 2022 is the earliest time we imagine LIGO-India could be operational, and it would take several more years for it to achieve full sensitivity.

This time-line is summarized in Figure 2. The observational implications of this scenario are discussed in Section 4.

Figure 2
figure 2

The planned sensitivity evolution and observing runs of the aLIGO and AdV detectors over the coming years. The colored bars show the observing runs, with the expected sensitivities given by the data in Figure 1. There is significant uncertainty in the start and end times of the observing runs, especially for those further in the future, and these could move forward or backwards by a few months relative to what is shown above. The plan is summarised in Section 2.2.

Full size image

3 Searches for Gravitational-Wave Transients

Data from GW detectors are searched for many types of possible signals [8]. Here we focus on signals from compact binary coalescences (CBCs), including BNS systems, and on generic transient or burst signals. See [19, 18, 14] for observational results from LIGO and Virgo for such systems.

The rate of BNS coalescences is uncertain [54]. For this work we adopt the estimates of [13], which predicts the rate to lie between 10−8–10−5 Mpc−3 yr−1, with a most plausible value of 10−6 Mpc−3 yr−1; this corresponds to 0.4–400 signals above an SNR of 8 per year of observation for a single aLIGO detector at final sensitivity, and a best estimate of 40 BNS signals per year [13]. Rate estimation remains an active area of research (e.g., [69, 50, 49, 47]), and will be informed by the number of detections (or lack thereof) in observing runs.

While the intrinsic rates of neutron star-black hole (NS-BH) and binary black hole (BBH) mergers are expected to be a factor of tens or hundreds lower than the BNS rate, the distance to which they can be observed is a factor of two to five larger. Consequently, the predicted observable rates are similar [13, 92]. Expected rates for other transient sources are lower and/or less well constrained.

The gravitational waveform from a BNS coalescence is well modeled and matched filtering can be used to search for signals and measure the system parameters [74, 35, 34, 90]. For systems containing black holes, or in which the component spin is significant, uncertainties in the waveform model can reduce the sensitivity of the search [81, 62, 45, 103, 85, 95, 68]. Searches for bursts make few assumptions on the signal morphology, using time-frequency decompositions to identify statistically significant excess-power transients in the data. Burst searches generally perform best for short-duration signals (≲ 1 s), although search development remains an area of active research (e.g., [71, 102, 40, 106, 26, 104, 43, 105, 66]); their astrophysical targets include core-collapse supernovae, magnetar flares, BBH coalescences, cosmic string cusps, and, possibly, as-yet-unknown systems.

In the era of advanced detectors, the LSC and Virgo will search in near real-time for CBC and burst signals for the purpose of rapidly identifying event candidates. A prompt notice of a potential GW transient by LIGO-Virgo might enable follow-up observations in the electromagnetic spectrum. A first follow-up program including low-latency analysis, event candidate selection, position reconstruction and the sending of alerts to several observing partners (optical, X-ray, and radio) was implemented and exercised during the 2009–2010 LIGO-Virgo science run [16, 15, 53]. Latencies of less than 1 hour were achieved and we expect to improve this in the advanced-detector era. Increased detection confidence, improved sky localization, and identification of host galaxy and redshift are just some of the benefits of joint GW-electromagnetic observations. With this in mind, we focus on two points of particular relevance for follow-up of GW events: the source localization afforded by a GW network as well as the relationship between signal significance, or false alarm rate (FAR), and source localization.

3.1 Detection and false alarm rates

The rate of false alarm triggers above a given SNR depends critically upon the data quality of the advanced detectors; non-stationary transients or glitches [1, 10] produce an elevated background of loud triggers. For low-mass binary coalescence searches, the waveforms are well modeled, and signal consistency tests reduce the background significantly [27, 38, 107]. For burst sources which are not well modeled, or which spend only a short time in the detectors’ sensitive band, it is more difficult to distinguish between the signal and a glitch, and so a reduction of the FAR comes at a higher cost in terms of reduced detection efficiency.

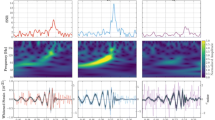
Figure 3 shows the noise background as a function of detection statistic for the low-mass binary coalescence and burst searches with the 2009–2010 LIGO-Virgo data [19, 14].Footnote 2 For binary mergers, the background rate decreases by a factor of ∼ 100 for every unit increase in combined SNR ρ c . Here ρ c is a combined, re-weighted SNR [29, 19]. The re-weighting is designed to reduce the SNR of glitches while leaving signals largely unaffected. Consequently, for a signal, ρ c is essentially the root-sum-square of the SNRs in the individual detectors. For bursts, we use the coherent network amplitude η, which measures the degree of correlation between the detectors [22, 86]. Glitches have little correlated energy and so give low values of η. Both ρ c and η give an indication of the amplitude of the signal and can be used to rank events.

Figure 3
figure 3

False alarm rate versus detection statistic for compact binary coalescence (CBC) and burst searches on 2009–2010 LIGO-Virgo data. Left: Cumulative rate of background events for a subset of the CBC search parameter space, as a function of the threshold ranking statistic ρ c [19]. Different methods were used to estimate the background for rate for high and low ρ c , which is why there is an apparent gap in the data points. The background for the full search was approximately a factor of six higher. Right: Cumulative rate of background events for the burst search, as a function of the coherent network amplitude η [14]. For ease of comparison, we have also plotted the approximate equivalent ρ c for the burst search (an exact identification is not possible as the search methods differ). The burst events are divided into two sets based on their central frequency.

Full size image

For CBC signals, we conservatively estimate that a ρ c threshold of 12 is required for a FAR below ∼ 10−2 yr−1 in aLIGO-AdV. To arrive at this estimate, we begin with Figure 3 which indicates that an SNR of around 10.5 corresponds to a FAR of 10−2 yr−1. However, this corresponds to only a subspace of the CBC parameter space and the background of the full search is a factor of six higher. Additionally, due to the improved low frequency sensitivity of the advanced detectors and the inclusion of templates for binaries with (aligned) component spins, at least ten times as many waveform templates are required to perform the search [84, 34, 88, 46]. The background increases approximately linearly with the number of templates required. Consequently, we expect a background around a factor of 100 higher than indicated by Figure 3, which leads us to quote a threshold of 12 for a FAR of 10−2 yr−1 [31]. A combined SNR of 12 corresponds to a single-detector SNR of 8.5 in each of two detectors, or 7 in each of three detectors.

Instrumental disturbances in the data can have a greater effect on the burst search. At frequencies above 200 Hz, the rate of background events falls off steeply as a function of amplitude. At lower frequencies, however, the data often exhibit a significant tail of loud background events that are not simply removed by multi-detector consistency tests. Although the advanced detectors are designed with many technical improvements, we anticipate that similar features will persist, particularly at low frequencies. Improvements to detection pipelines, which better distinguish between glitches and potential waveforms, can help eliminate these tails (e.g., [66]). For a given FAR, the detection threshold may need to be tuned for different frequency ranges; for the initial detectors, a threshold of η ≳ 4.5–6 (approximately equivalent to ρ c ≳ 12 from Figure 3) was needed for a FAR of 1/8 yr−1 [14]. The unambiguous observation of an electromagnetic counterpart could increase the detection confidence.

3.2 Sky localization

Following the detection by the aLIGO-AdV network of a GW transient, determining the source’s location is a question for parameter estimation. Typically, posterior probability distributions for the sky position are constructed following a Bayesian framework [110, 43, 98]. Information comes from the time of arrival, plus the phase and amplitude of the GW.

An intuitive understanding of localization can be gained by considering triangulation using the observed time delays between sites [55, 56]. The effective single-site timing accuracy is approximately

$${\sigma _t} = {1 \over {2\pi \,\rho {\sigma _f}}},$$
(1)

where ρ is the SNR in the given detector and σ f is the effective bandwidth of the signal in the detector, typically of order 100 Hz. Thus a typical timing accuracy is on the order of 10−4 s (about 1/100 of the light travel time between sites). This sets the localization scale. The simple model of equation (1) ignores many other relevant issues such as information from the signal amplitudes across the detector network, uncertainty in the emitted gravitational waveform, instrumental calibration accuracies, and correlation of sky location with other binary parameters [55, 112,