Asymptotic behaviour and boundedness of solutions for third-order stochastic differential equation with multi-delay

Abstract

In the present paper, we study stochastic stability and stochastic boundedness for the stochastic differential equation (SDE) with multi-delay of third order. The derived results extend and improve some earlier results in the relevant literature, which are related to the qualitative properties of solutions to third-order delay differential equations (DDEs) and SDEs with multi-delay. Two examples are given to illustrate the results.

1 Introduction

During the past several years, the DDEs and the differential equations (DEs) with multiple delays have received more attention because of their widely applied backgrounds, such as population ecology, heat exchanges, mechanics, and economics. Here, we can mention the books by Burton [15], Hale [17], Hale and Verduyn Lunel [18], Iannelli [19] and numerous researchers activities such that, Abdel-Razek et al. [1], Abou-El-Ela et al. [2], Ademola and Arawomo [10], Ademola et al [11, 13], Mahmoud and Bakhit [24] Omeike [35, 36] Remili and Beldjerd [37], Remili and Oudjedi [38–40], Remili [41], Tunç [43–48], and the references therein.

Moreover, another kind of the DEs is the stochastic delay differential equations (SDDEs), where relevant parameters are modeled as suitable stochastic processes; see the book by Gikhman and Skorokhod [16]. The SDDE is a DE whose coefficients are random numbers or random functions of the independent variable (or variables). It is the appropriate tool for describing systems with external noise. The models of SDDEs play an important role in a range of application areas, including biology, chemistry, epidemiology, mechanics, microelectronics, economics, and finance. For example, in biology, we see that recently, Fathalla A. Rihan [42] studied the SDDEs for the spread of Coronavirus Infection COVID-19.

Furthermore, SDDEs are crucial in ecology, epidemiology, and many other fields; see, for example, Arnold [14], Mao [29–33], Øksendal [34], and references therein.

In the last few decades, the theory of SDDEs has attracted much attention, and numerous papers have been published. Here, we can mention the works by Abou-El-Ela et al. [3–7], Ademola [8], Ademola et al. [9, 12], Liu [21], Liu and Raffoul [22], Luo [23], Mahmoud and Tunç [26–28], Tunç [49], Zhi and Liping [20], and the references therein. Recently, Mahmoud and Bakhit [25] established the properties of solutions for nonautonomous third-order stochastic differential equation with a constant delay

$$\begin{aligned} \begin{aligned} &x'''(t)+a(t)f \bigl(x(t), x'(t)\bigr)x''(t)+b(t)\phi \bigl(x(t)\bigr)x'(t)+c(t)\psi \bigl(x(t-r)\bigr) \\ &\quad {}+g(t,x)w'(t)=p\bigl(t,x(t),x'(t),x''(t) \bigr). \end{aligned} \end{aligned}$$

The main purpose of this note is to establish new criteria for the uniformly stochastic asymptotical stability (USAS) and uniformly stochastic boundedness (USB) for solutions of the following more general third-order SDE with multi-delay as the form

$$\begin{aligned} \begin{aligned} &\bigl[h\bigl(x'(t)\bigr) x''(t) \bigr]'+\bigl(\psi _{1} \bigl(x,x'\bigr)x'\bigr)'+\sum _{i=1}^{n}{Q_{i}\bigl(x \bigl(t-r_{i}(t)\bigr),x'\bigl(t-r_{i}(t)\bigr) \bigr)} \\ &\quad {} +\sum_{i=1}^{n}{f_{i}\bigl(x \bigl(t-r_{i}(t)\bigr)\bigr)}+\alpha x\bigl(t-l(t)\bigr)w'(t)= \varepsilon \bigl(x,x',x''\bigr), \end{aligned} \end{aligned}$$

(1.1)

where $r_{i}(t)$ is continuously differentiable functions with $0 \leq r_{i}(t) \leq \gamma _{i}$, ($i=1,2,\ldots,n$), $\gamma _{i} > 0$ are constants, $\psi _{1}$, $Q_{i}$, $f_{i}$ and ε are continuous functions in their respective arguments, with $Q_{i}(x,0)=Q(0,y)=0$ and $f_{i}(0)=0$. In addition, $l(t)$ is a continuous function and defined from $[0,\infty )$ to $[0,l_{1}]$. $w(t) \in \mathbb{R}^{n}$ is a standard Brownian motion.

Consider the following notations

$$\begin{aligned}& \Phi _{1}(t)=\frac{\partial \psi _{1}}{\partial x}\frac{dx}{dt}+ \frac{\partial \psi _{1}}{\partial y} \frac{dy}{dt}, \qquad h\bigl(x'(t)\bigr)= H(t), \\& \Phi _{2}(t)= \frac{H'(t)}{H^{2}(t)} \quad \text{and} \quad \Phi _{3}(t)= \biggl(\frac{\psi _{1}(x,y)}{H(t)} \biggr)'. \end{aligned}$$

Therefore, equivalent system of (1.1) can be written as

$$\begin{aligned} \begin{aligned} &x '=y, \\ &y '=\frac{z}{H(t)}, \\ & z'=-\psi _{1}(x,y)\frac{z}{H(t)}-y \Phi _{1}(t) -\sum_{i=1}^{n}{Q_{i}(x,y)} \\ & \hphantom{z'=}{}+ \sum_{i=1}^{n} \biggl\{ \int _{t-r_{i}(t)}^{t}{ \frac{\partial Q_{i}(x(s),y(s))}{\partial x} y(s)\,ds }+ \int _{t-r_{i}(t)}^{t}{ \frac{\partial Q_{i}(x(s),y(s))}{\partial y} \frac{z(s)}{H(s)}\,ds } \biggr\} \\ & \hphantom{z'=}{}-\sum_{i=1}^{n}{f_{i}(x)} + \sum_{i=1}^{n}{ \int _{t-r_{i}(t)}^{t}{f'_{i} \bigl(x(s)\bigr)y(s)}}\,ds-\alpha x\bigl(t-l(t)\bigr)w'(t) + \varepsilon \biggl(x,y,\frac{z}{H(t)} \biggr). \end{aligned} \end{aligned}$$

(1.2)

Remarks

• (1) Whenever $\alpha x(t-l(t))w'(t)=0$, and we consider the case that $i=1$, then equation (1.1) reduces to a DDE of third order discussed in [39].

• (2) Suppose that $\alpha =0$, $h(x'(t))=g(x''(t))$, $\psi _{1}(x,x') =h(x'(t))$, and with $i=1$ if we let $Q(x(t-r(t)),x'(t-r(t)))=(\varphi (x(t))x(t))'$, then (1.1) can be reduced to the equation studied in [41].

• (3) In the case $i=1$, $\alpha =0$ and if $h(x'(t))=1$, $(\psi _{1}(x,x') x')'= f(x,x')x''$, then equation (1.1) specialises to that considered in [2]. Our results generalize all the previous results.

• (4) Whenever, $h(x'(t))=1$, $(\psi _{1}(x,x')x')'= a(t)f(x(t),x'(t))x''(t)$, and when $i=1$, $Q_{i}(x(t-r_{i}(t)),x'(t-r_{i}(t)))= b(t)\phi (x(t))x'(t)$, $f_{i}(x(t-r_{i}(t)))=c(t)\psi (x(t-r))$, and $\alpha x(t-l(t))=g(t,x)$, then (1.1) reduces to the studied equation in [25]. Thus, equation (1.1) generalizes the results obtained in [25]. Hence, our results include and extend all the previous results.

2 Stability results

Let $B(t) = (B_{1}(t),\ldots ,B_{m}(t))$ be an m-dimensional Brownian motion defined on the probability space. Consider an n-dimensional SDDE

$$ dx(t) = N_{1}(t,x_{t})\,dt +N_{2}(t,x_{t})\,dB(t),\quad x_{t}(\theta )=x(t+ \theta ) -r \leq \theta \leq 0, t\geq t_{0},$$

(2.1)

with initial value $x(0) = x_{0}\in \mathcal{C}([-r,0];\mathbb{R}^{n} )$. Suppose that $N_{1}: \mathbb{R}^{+}\times \mathbb{R}^{n}\rightarrow \mathbb{R}^{n}$ and $N_{2} :\mathbb{R}^{+}\times \mathbb{R}^{n}\rightarrow \mathbb{R}^{n\times m}$ satisfy the local Lipschitz and the linear growth conditions. Hence, for any given initial value $x(0) = x_{0}\in \mathbb{R}^{n}$, it is known that equation (2.1) has a unique continuous solution on $t\geq 0$, which is known as $x(t; x_{0})$ in this section. Suppose that $N_{1}(t,0) = 0\text{ and }N_{2}(t,0) = 0$, for all $t\geq 0$. Hence, the SDDE admits the zero solution $x(t;0)\equiv 0$.

Consider a functional $W(t, \varphi )$ that can be represented in the form $W(t, \varphi )=W(t, \varphi (0), \varphi (s))$, $s<0$, for $\varphi =x_{t}$, put

$$\begin{aligned} W_{\varphi}(t,\varphi )= W(t,\varphi )=W(t, x_{t})=W\bigl(t, x, x(t+s)\bigr), \quad x=\varphi (0)=x(t), s< 0, \end{aligned}$$

and suppose that the function $W_{\varphi}(t, x)$ has a continuous derivative with respect to t and two continuous derivatives with respect to x.

Let $C^{1,2}(\mathbb{R}^{+} \times \mathbb{R}^{n};\mathbb{R}^{+})$ denote the family of nonnegative functionals $W(t,x_{t})$ defined on $\mathbb{R}^{+}\times \mathbb{R}^{n}$, which are once continuously differentiable in t and twice continuously differentiable in x.

By the Itô formula, we have

$$ dW(t,x_{t}) =\mathcal{L}W(t,x_{t})\,dt+W_{x}(t,x_{t})N_{2}(t,x_{t})\,dB(t),$$

where

$$ \begin{aligned} L W(t,x_{t}) ={}& W_{t}(t,x_{t})+ W_{x}(t,x_{t})N_{1}(t,x_{t}) \\ & {}+\frac{1}{2}\operatorname{trace}\bigl[N_{2}^{T}(t,x_{t})W_{xx}(t,x_{t}) N_{2}(t,x_{t})\bigr], \end{aligned} $$

(2.2)

such that

$$\begin{aligned}& W_{x}=(W_{x_{1}},\ldots ,W_{x_{n}}), \qquad W_{t}(t,x_{t})= \frac{\partial W(t, x_{t})}{\partial t}, \\& W_{x}(t,x_{t})= \biggl( \frac{\partial W(t, x_{t})}{\partial x_{1}} , \ldots, \frac{\partial W(t, x_{t})}{\partial x_{n}} \biggr). \end{aligned}$$

Furthermore,

$$\begin{aligned} W_{xx}=(W_{x_{i}x_{j}})_{n\times n}= \biggl( \frac{\partial ^{2} W(t, x_{t})}{\partial x_{i} x_{j}} \biggr)_{n \times n},\quad i, j = 1,2,3, \ldots, n. \end{aligned}$$

Now, we will give some definitions

Definition 2.1

[32] The zero solution of (2.1) is said to be stochastically stable or stable in probability if for every pair of $\varepsilon \in (0,1)$ and $r > 0$, there exists a $\delta = \delta (\varepsilon ,r ) > 0$ such that

$$ P\bigl\{ \bigl\vert x(t; x_{0}) \bigr\vert < r\text{ for all }t \geq 0\bigr\} \geq 1-\varepsilon ,$$

whenever $|x_{0}| <\delta$. Otherwise, it is said to be stochastically unstable.

Definition 2.2

[32] The zero solution of (2.1) is said to be stochastically asymptotically stable if it is stochastically stable, and, moreover, for every $\varepsilon \in (0,1)$, there exists a $\delta _{0} = \delta _{0}(\varepsilon ) > 0$, such that

$$ P\Bigl\{ \lim_{t\rightarrow \infty}x(t; x_{0})=0\Bigr\} \geq 1- \varepsilon ,$$

whenever $|x_{0}| <\delta _{0}$.

Definition 2.3

[22] (Stochastic boundedness) A solution $x(t; t_{0}, x_{0})$ of (2.1) is said to be stochastically bounded, or bounded in probability, if it satisfies

$$ E^{x_{0}} \bigl\Vert x(t; t_{0}, x_{0}) \bigr\Vert \leq C\bigl( \Vert x_{0} \Vert , t_{0}\bigr),\quad \text{for all } t \geq t_{0}, $$

where $E^{x_{0}}$ denotes the expectation operator with respect to the probability law associated with $x_{0}$, and $C : \mathbb{R}^{+} \times \mathbb{R}^{+} \rightarrow \mathbb{R}^{+}$ is a constant depending on $t_{0}$ and $x_{0}$. We say that solutions of (2.1) are uniformly stochastically bounded if C is independent of $t_{0}$.

Hypotheses

Suppose that there exist positive constants $a_{0}$, a, μ, D, C, $b_{i}$, $c_{i}$, $d_{i}$, $L_{i}$, $M_{i}$, $N_{i}$, $A_{i}$, $B_{i}$, $C_{i}$, $D_{i}$, $\gamma _{i}$, $H_{1}$, $H_{2}$ and $l_{1}$, such that

($h_{1}$):

$1 < a \leq \psi _{1}(x,y)\leq a_{0}$, $y \frac{\partial \psi _{1}}{\partial x}\leq 0$ for all $x,y \in \mathbb{R}$.

($h_{2}$):

$\frac{Q_{i}(x,y)}{y}\geq b_{i}>0$, $y\neq 0$; $f_{i}(x)\geq d_{i} x$ with $\sup \{f'_{i}(x)\} =\frac{c_{i}}{2}$, $f_{i}(x) \operatorname{sgn} x>0$ for $x\neq 0$ and $|f'_{i}(x)| \leq L_{i}$.

($h_{3}$):

$H_{1} \leq H(t) \leq H_{2} \leq 1$, $H_{1}(a-1)\geq 2\mu$.

($h_{4}$):

$|\frac{\partial Q_{i}}{\partial x} | \leq M_{i}$, $| \frac{\partial Q_{i}}{\partial y}| \leq N_{i}$ and $r_{i}(t) \leq \gamma _{i}$, $r'_{i}(t)\leq \beta _{i}$, $0<\beta _{i} \leq 1$.

($h_{5}$):

$ab_{i}-c_{i}> 2 M_{i}+2b_{i}+6$.

($h_{6}$):

$0< l(t) \leq l_{1}$, $|l'(t)|\leq \frac{1}{2}$.

($h_{7}$):

$2\alpha ^{2} \leq 2H_{1} d_{i}-H_{1}(a+b_{i}+2)$.

($h_{8}$):

$\int _{-\infty}^{\infty}{ | \frac{\partial \psi _{1}(u,v)}{\partial u}|\,du}+\int _{-\infty}^{ \infty}{ |\frac{\partial \psi _{1}(u,v)}{\partial v}|\,dv}\leq D< \infty$, $\int _{-\infty}^{\infty}{|h'(u)|\,du}\leq C <\infty$.

Theorem 2.1

Assuming that the hypotheses $(h_{1})$–$(h_{8})$ hold true provided that

$$\begin{aligned} \begin{aligned} \gamma _{i}\leq {}&\min \biggl[ \biggl\{ \frac{2H_{1}d_{i}-H_{1}(b_{i}+a+1)-2\alpha ^{2}}{2A_{i}} \biggr\} , \biggl\{ \frac{B_{i}(ab_{i}-c_{i}-2M_{i}-2b_{i}-6)}{4(\mu B_{i} N_{i} +D_{i})} \biggr\} , \\ &{} \biggl\{ \frac{B_{i}H_{1}(aH_{1}+2H_{1}\mu +H_{1})}{2(B_{i}A_{i} +C_{i})} \biggr\} \biggr], \end{aligned} \end{aligned}$$

where

$$\begin{aligned} \begin{aligned} &A_{i}=H_{1}(M_{i}+L_{i})+N_{i}, \\ & B_{i}=1-\beta _{i}, \\ & C_{i}=N_{i}\bigl((\mu +1)H_{1}+1\bigr), \\ & D_{i}=(M_{i}+L_{i}) \bigl\{ H_{1}( \mu +1)+\mu H_{1}(1-\beta _{i})+1 \bigr\} , \end{aligned} \end{aligned}$$

(2.3)

with

$$\begin{aligned} \mu =\sum_{i=1}^{n}{\frac{ab_{i}+c_{i}}{4b_{i}}}. \end{aligned}$$

Then, the zero solution of (1.1) is USAS.

Proof

The main tool of the stability results is the continuously differentiable functional $W_{1}=W_{1}(x_{t},y_{t},z_{t})$, defined as

$$\begin{aligned} W_{1}=\exp \biggl(-\frac{\omega (t)}{\mu _{1}}\biggr)U_{1}, \end{aligned}$$

where

$$\begin{aligned} \omega (t)= \int _{0}^{t}{\eta _{1}(s)\,ds },\quad \text{such that } \eta _{1}(t)= \bigl\vert \Phi _{1}(t) \bigr\vert + \bigl\vert \Phi _{2}(t) \bigr\vert . \end{aligned}$$

Considering $\varepsilon \equiv 0$, we can observe that the Lyapunov functional $U_{1}=U_{1}(x_{t},y_{t},z_{t})$, where $x_{t}= x(t+s)$, $s \leq 0$, can be written as follows

$$\begin{aligned} U_{1}={}&\mu \sum _{i=1}^{n}{ \int _{0}^{x}{f_{i}(\xi )\,d\xi}} + y \sum_{i=1}^{n}{f_{i}(x)} + \mu \int _{0}^{y}{\psi _{1}(x, \eta )\eta d \eta} \\ \begin{aligned}&{}+\sum_{i=0}^{n}{ \int _{0}^{y}{Q_{i}(x, \eta )\,d\eta }} +\mu y z + \frac{1}{2 H(t)}z^{2} + x^{2} + x z \\ &{} + \frac{\alpha ^{2}}{H_{1}} \int _{t-l(t)}^{t}{x^{2}(s)\,ds } + \sum _{i=1}^{n}{ \lambda _{i} \int ^{0}_{-r_{i}(t)}{ \int ^{t}_{t+s}{y^{2}(\theta )\,d\theta \,ds }}}\end{aligned} \\ &{}+\sum_{i=1}^{n}{\delta _{i} \int ^{0}_{-r_{i}(t)}{ \int ^{t}_{t+s}{z^{2}( \theta )\,d\theta \,ds }}}. \end{aligned}$$

(2.4)

Since the integrals $\int _{t-l(t)}^{t}{x^{2}(s)\,ds}$, $\int ^{0}_{-r_{i}(t)}{ \int ^{t}_{t+s}{y^{2}(\theta )\,d\theta \,ds}}$ and $\int ^{0}_{-r_{i}(t)}{\int ^{t}_{t+s}{z^{2}(\theta )\,d\theta \,ds }}$ are positive, from the conditions $(h_{1})$–$(h_{3})$, we conclude

$$\begin{aligned} \begin{aligned} U_{1}\geq {}&\mu \sum _{i=1}^{n}{ \int _{0}^{x}{f_{i}(\xi )\,d\xi}} + y \sum_{i=1}^{n}{f_{i}(x)} + \frac{1}{2}\mu a y^{2}+ \frac{1}{2}y^{2} \sum _{i=1}^{n}{b_{i}} \\ &{}+\mu y z + \frac{1}{2}z^{2} + x^{2} + x z. \end{aligned} \end{aligned}$$

Therefore, we get

$$\begin{aligned} \begin{aligned} U_{1}\geq{} & \sum _{i=1}^{n}{\frac{1}{2b_{i}} \bigl(b_{i}y+f_{i}(x) \bigr)^{2}} + \biggl(\mu y+\frac{z}{2} \biggr)^{2}+ \biggl(x+ \frac{z}{2} \biggr)^{2}+\frac{1}{2}\mu (a-2 \mu ) y^{2} \\ &{} + \sum_{i=1}^{n}{\frac{1}{2b_{i} y^{2}} \biggl[ 4 \int _{0}^{x} f_{i}( \xi ) \biggl\{ \int _{0}^{y}\bigl(\mu b_{i}-f'_{i}( \xi )\bigr)\eta \,d\eta \biggr\} \,d\xi \biggr]}. \end{aligned} \end{aligned}$$

Since $\mu =\sum_{i=1}^{n}{\frac{ab_{i}+c_{i}}{4b_{i}}}$ and $\sup \{f'(x)\}=\frac{c_{i}}{2}$, it follows that

$$\begin{aligned} a-2 \mu =\sum_{i=1}^{n}{ \frac{ab_{i}-c_{i}}{2b_{i}}}>0, \end{aligned}$$

and

$$\begin{aligned} \sum_{i=1}^{n}{ \bigl(\mu b_{i}- f'_{i}(x)\bigr)} \geq \sum_{i=1}^{n}{ \frac{ab_{i}-c_{i}}{4}}>0. \end{aligned}$$

Then, we get

$$\begin{aligned} \sum_{i=1}^{n}{\frac{1}{2b_{i} y^{2}} \biggl[ 4 \int _{0}^{x} f_{i}( \xi ) \biggl\{ \int _{0}^{y}\bigl(\mu b_{i}-f'_{i}( \xi )\bigr)\eta \,d\eta \biggr\} \,d\xi \biggr]}\geq \sum _{i=1}^{n}{ \frac{ab_{i}-c_{i}}{4b_{i}} \int _{0}^{x} f_{i}(\xi )\,d\xi}, \end{aligned}$$

which tends to the following

$$\begin{aligned} \begin{aligned} U_{1}\geq{} & \sum _{i=1}^{n}{\frac{1}{2b_{i}} \bigl(b_{i}y+f_{i}(x) \bigr)^{2}} + \biggl(\mu y+\frac{z}{2} \biggr)^{2}+ \biggl(x+ \frac{z}{2} \biggr)^{2} \\ &{}+\frac{1}{2}\mu \sum_{i=1}^{n}{} \biggl({ \frac{ab_{i}-c_{i}}{2b_{i}}} \biggr)y^{2} + \sum _{i=1}^{n}{ \frac{ab_{i}-c_{i}}{4b_{i}} \int _{0}^{x} f_{i}(\xi )\,d\xi}. \end{aligned} \end{aligned}$$

(2.5)

Hence, there exists a positive constant $E_{1}$, such that

$$\begin{aligned} U_{1}\geq E_{1}\bigl(x^{2}+y^{2}+z^{2} \bigr). \end{aligned}$$

(2.6)

In view of the hypotheses $(h_{1})$–$(h_{4})$ and the following inequalities

$$\begin{aligned}& \int ^{0}_{-r_{i}(t)}{ \int ^{t}_{t+s}{y^{2}(\theta )\,d\theta \,ds }} \leq \Vert y \Vert ^{2} \int _{t-r_{i}(t)}^{t}{\bigl(\theta -t+r_{i}(t) \bigr)\,d\theta},\\& \int ^{0}_{-r_{i}(t)}{ \int ^{t}_{t+s}{z^{2}(\theta )\,d\theta \,ds }} \leq \Vert z \Vert ^{2} \int _{t-r_{i}(t)}^{t}{\bigl(\theta -t+r_{i}(t) \bigr)\,d\theta}, \end{aligned}$$

and

$$\begin{aligned} \int ^{t}_{t-l(t)}{x^{2}(s)\,ds } \leq l(t) \Vert x \Vert ^{2}. \end{aligned}$$

Therefore, we can write (2.4) as

$$\begin{aligned} \begin{aligned} U_{1}\leq {}&\frac{1}{2}\mu x^{2} \sum_{i=1}^{n}L_{i} +x y \sum_{i=1}^{n}{L_{i}} + \frac{1}{2}\mu a_{0} y^{2} +\frac{1}{2}y^{2} \sum_{i=0}^{n}{M_{i} } +\mu y z + \frac{1}{2 H_{1}}z^{2} \\ &{}+ x^{2} + x z+\frac{\alpha ^{2}}{H_{1}}l(t) \Vert x \Vert ^{2}+ \sum_{i=1}^{n}{ \bigl( \lambda _{i} \Vert y \Vert ^{2}+\delta _{i} \Vert z \Vert ^{2} \bigr) \int _{t-r_{i}(t)}^{t}{\bigl( \theta -t+r_{i}(t) \bigr)}\,d\theta}. \end{aligned} \end{aligned}$$

Since $r_{i}(t)\leq \gamma _{i}$ and $l(t) \leq l_{1}$, with applying the estimate $2pq\leq (p^{2}+q^{2})$, we find

$$\begin{aligned} \begin{aligned} U_{1}\leq {}& \sum _{i=1}^{n}{} \biggl\{ \frac{L_{i}(\mu +1)+3}{2}+ \frac{\alpha ^{2}}{H_{1}}l_{1} \biggr\} \Vert x \Vert ^{2} \\ &{}+\sum_{i=1}^{n}{} \biggl\{ \frac{\mu (a+a_{0}+1)+(L_{i}+M_{i})+\gamma _{i}^{2}\lambda _{i}}{2} \biggr\} \Vert y \Vert ^{2} \\ &{}+ \sum_{i=1}^{n}{} \biggl\{ \frac{1+H_{1}(\mu +1+\gamma _{i}^{2}\delta _{i})}{2H_{1}} \biggr\} \Vert z \Vert ^{2}. \end{aligned} \end{aligned}$$

(2.7)

Then, there exists a positive constant $E_{2}$, such that

$$\begin{aligned} U_{1}\leq E_{2}\bigl(x^{2}+y^{2}+z^{2} \bigr). \end{aligned}$$

(2.8)

Now, using the equivalent system (1.2) with $\varepsilon =0$ and the Itô formula (2.2), the derivative of the Lyapunov functional $U_{1}$ is given by

$$\begin{aligned} L U_{1}={}& y^{2}\sum _{i=1}^{n}{f'_{i}(x)+\mu \int _{0}^{y}} {y \frac{\partial \psi _{1}(x,\eta )}{\partial x}\eta d \eta} + \sum_{i=1}^{n}{ \int _{0}^{y}} {\frac{\partial Q_{i}(x,\eta )}{\partial x}y d \eta }+ \frac{\mu}{H(t)}z^{2} \\ &{}- \biggl(\mu y^{2}+ \frac{1}{H(t)}yz+xy \biggr) \Phi _{1}(t) -\mu y \sum_{i=1}^{n}{Q_{i}(x,y)}- \frac{\psi _{1}(x,y)}{(H(t))^{2}}z^{2} \\ &{}- \frac{\psi _{1}(x,y)}{H(t)}xz-x \sum_{i=1}^{n}{Q_{i}(x,y)} -x \sum_{i=1}^{n}{f_{i}(x)}+2xy+yz \\ & {}- \frac{H'(t)}{2(H(t))^{2}}z^{2} +\frac{\alpha ^{2}}{H_{1}}x^{2}(t)- \frac{\alpha ^{2}}{H_{1}}x^{2}\bigl(t-l(t)\bigr) \bigl(1-l'(t) \bigr) \\ &{}+\frac{\alpha ^{2}}{2H_{1}}x^{2}\bigl(t-l(t)\bigr) +y^{2}\sum _{i=1}^{n}{ \lambda _{i} \gamma _{i}}+z^{2}\sum_{i=1}^{n}{ \delta _{i} \gamma _{i}} \\ &{}- \sum_{i=1}^{n}{\lambda _{i}(1-\beta _{i}) \int _{t-r_{i}(t)}^{t}{y^{2}( \theta )\,d\theta }}- \sum_{i=1}^{n}{\delta _{i}(1-\beta _{i}) \int _{t-r_{i}(t)}^{t}{z^{2}( \theta )\,d\theta }} \\ &{}+ \biggl(x+\mu y+ \frac{1}{H(t)}z \biggr) \Biggl[ \sum _{i=1}^{n}{ \int _{t-r_{i}(t)}^{t} \biggl\{ { \frac{\partial Q_{i}(x(s),y(s))}{\partial x}y(s)}} \\ &{} +\frac{\partial Q_{i}(x(s),y(s))}{\partial y} \frac{z(s)}{H(s)}+ f'\bigl(x(s)\bigr)y(s)\,ds \biggr\} \Biggr]. \end{aligned}$$

Therefore, using the definition of $\Phi _{2}(t)$ and considering the conditions $(h_{1})$–$(h_{4})$ of Theorem 2.1, we have

$$\begin{aligned} \begin{aligned} L U_{1}\leq{} & \frac{1}{2} y^{2}\sum_{i=1}^{n}{c_{i}}+ \frac{\mu}{H_{1}}z^{2}- \biggl(\mu y^{2}+ \frac{1}{H(t)}yz+xy \biggr) \Phi _{1}(t)+\frac{y^{2}}{2}\sum _{i=1}^{n}{M_{i}}- \frac{1}{2} \Phi _{2}(t) z^{2} \\ &{}- az^{2}- a xz-x y\sum_{i=1}^{n}{b_{i}} -x^{2} \sum_{i=1}^{n}{d_{i}}+2xy+yz +\frac{\alpha ^{2}}{H_{1}}x^{2}(t) +y^{2}\sum _{i=1}^{n}{\lambda _{i} \gamma _{i}} \\ &{}+z^{2}\sum_{i=1}^{n}{\delta _{i} \gamma _{i}}- \sum_{i=1}^{n}{ \lambda _{i}(1-\beta _{i}) \int _{t-r_{i}(t)}^{t}{y^{2}(\theta )\,d\theta }} \\ &{}- \sum_{i=1}^{n}{\delta _{i}(1-\beta _{i}) \int _{t-r_{i}(t)}^{t}{z^{2}( \theta )\,d\theta }} \\ &{}-\mu y^{2} \sum_{i=1}^{n}{b_{i}}+ \biggl(x+\mu y+ \frac{1}{H_{1}}z \biggr) \Biggl[ \sum _{i=1}^{n}{ \int _{t-r_{i}(t)}^{t} \biggl\{ { \biggl\vert \frac{\partial Q_{i}(x(s),y(s))}{\partial x} \biggr\vert y(s)}} \\ & {}+ \biggl\vert \frac{\partial Q_{i}(x(s),y(s))}{\partial y} \biggr\vert \frac{z(s)}{H(s)}+ f'\bigl(x(s)\bigr)y(s)\,ds \biggr\} \Biggr]. \end{aligned} \end{aligned}$$

(2.9)

Suppose that

$$\begin{aligned} \begin{aligned} \Phi (t)=- \biggl(\mu y^{2}+ \frac{1}{H(t)}yz+xy \biggr) \Phi _{1}(t)- \frac{1}{2} \Phi _{2}(t) z^{2}. \end{aligned} \end{aligned}$$

Using the Schwarz inequality $|pq|\leq \frac{1}{2}(p^{2}+q^{2})$ and $(h_{3})$, we can write the above equation as

$$\begin{aligned} \begin{aligned} \Phi (t) \leq \biggl(\mu y^{2}+ \frac{1}{2H_{1}}y^{2}+ \frac{1}{2H_{1}}z^{2}+ \frac{1}{2}\bigl(x^{2}+y^{2}\bigr) \biggr) \bigl\vert \Phi _{1}(t) \bigr\vert + \frac{1}{2} z^{2} \bigl\vert \Phi _{2}(t) \bigr\vert . \end{aligned} \end{aligned}$$

Therefore, we get

$$\begin{aligned} \begin{aligned} \Phi (t) & \leq \biggl\{ \biggl(\mu + \frac{1}{H_{1}}+ 1 \biggr) \bigl\vert \Phi _{1}(t) \bigr\vert + \frac{1}{2} \bigl\vert \Phi _{2}(t) \bigr\vert \biggr\} \bigl(x^{2}+y^{2}+z^{2}\bigr) \\ & \leq \biggl\{ \biggl(\mu + \frac{1}{H_{1}}+ 1 \biggr)+\frac{1}{2} \biggr\} \bigl( \bigl\vert \Phi _{1}(t) \bigr\vert + \bigl\vert \Phi _{2}(t) \bigr\vert \bigr) \bigl(x^{2}+y^{2}+z^{2} \bigr). \end{aligned} \end{aligned}$$

For the positive constant $E_{3}$, the last inequality becomes

$$\begin{aligned} \begin{aligned} \Phi (t) \leq E_{3} \eta _{1}(t) \bigl(x^{2}+y^{2}+z^{2}\bigr), \end{aligned} \end{aligned}$$

where

$$\begin{aligned} \begin{aligned} E_{3} =\max \biggl\{ \frac{1}{2}, \mu +\frac{1}{H_{1}}+1 \biggr\} . \end{aligned} \end{aligned}$$

It follows from (2.6) that

$$\begin{aligned} \begin{aligned} \Phi (t) \leq \frac{ E_{3}}{E_{1}} \eta _{1}(t) U_{1}. \end{aligned} \end{aligned}$$

(2.10)

Thus, by (2.9), (2.10) and the fact that $2pq\leq ( p^{2}+q^{2})$, we obtain the following estimate

$$\begin{aligned} \begin{aligned} L U_{1}\leq {}& - \frac{1}{2H_{1}} \sum_{i=1}^{n}{} \bigl\{ 2H_{1}d_{i}-H_{1}(a+b_{i}+2)-2 \alpha ^{2}- \bigl(H_{1}(M_{i}+L_{i})+N_{i} \bigr)\gamma _{i} \bigr\} x^{2} \\ &{}-\sum_{i=1}^{n}{} \biggl\{ \mu b_{i}-\frac{c_{i}}{2}-\frac{M_{i}}{2}- \frac{b_{i}}{2}- \frac{3}{2}- \frac{\mu (H_{1}(M_{i}+L_{i})+N_{i})}{2H_{1}}\gamma _{i} -\lambda _{i} \gamma _{i} \biggr\} y^{2} \\ &{}-\sum_{i=1}^{n}{} \biggl\{ \frac{H_{1}(a-1)-2\mu}{2H_{1}}- \frac{\mu (H_{1}(M_{i}+L_{i})+N_{i})}{2H_{1}^{2}}\gamma _{i} -\delta _{i} \gamma _{i} \biggr\} z^{2}+\frac{ E_{3}}{E_{1}} \eta _{1}(t) U_{1} \\ &{}+\sum_{i=1}^{n}{} \biggl\{ \frac{1}{2H_{1}} (M_{i}+L_{i}) \bigl(H_{1}(\mu +1)+1\bigr)-(1- \beta _{i}) \lambda _{i} \biggr\} \int _{t-r_{i}(t)}^{t}{y^{2}(\theta )\,d\theta} \\ &{}+\sum_{i=1}^{n}{} \biggl\{ \frac{N_{i}}{2H_{1}^{2}} \bigl(H_{1}(\mu +1)+1\bigr)-(1- \beta _{i}) \delta _{i} \biggr\} \int _{t-r_{i}(t)}^{t}{z^{2}(\theta )\,d\theta}. \end{aligned} \end{aligned}$$

(2.11)

If we let

$$\begin{aligned} \begin{aligned} \lambda _{i}= \frac{(M_{i}+L_{i})\{H_{1}(\mu +1)+1\}}{2H_{1}(1-\beta _{i})} , \end{aligned} \end{aligned}$$

and

$$\begin{aligned} \begin{aligned} \delta _{i}=\frac{N_{i}\{H_{1}(\mu +1)+1\}}{2H_{1}^{2}(1-\beta _{i})} . \end{aligned} \end{aligned}$$

We also have $\mu b_{i}-\frac{c_{i}}{2}=\frac{ab_{i}-c_{i}}{4}>0$ and $H_{1}(a-1)\geq 2\mu$; therefore, (2.11) becomes

$$\begin{aligned} \begin{aligned} L U_{1}\leq {}& -\frac{1}{2H_{1}} \sum _{i=1}^{n}{} \bigl\{ 2H_{1}d_{i}-H_{1}(a+b_{i}+2)-2 \alpha ^{2}- \bigl(H_{1}(M_{i}+L_{i})+N_{i} \bigr)\gamma _{i} \bigr\} x^{2} \\ & {}-\sum_{i=1}^{n}{} \biggl[ \frac{ab_{i}-c_{i}-2M_{i}-2b_{i}-6}{4} \\ &{}- \frac{ (M_{i}+L_{i} ) \{H_{1}(\mu +1)+\mu H_{1}(1-\beta _{i})+1 \}+\mu N_{i}(1-\beta _{i})}{2H_{1}(1-\beta _{i})} \gamma _{i} \biggr]y^{2} \\ &{}-\sum_{i=1}^{n}{} \biggl\{ \frac{H_{1}(a-1)-2\mu}{2H_{1}}- \frac{(1-\beta _{i})(H_{1}(M_{i}+L_{i})+N_{i}) +N_{i}((\mu +1)H_{1}+1)}{2(1-\beta _{i})H_{1}^{2}}\gamma _{i} \biggr\} z^{2} \\ &{}+\frac{ E_{3}}{E_{1}} \eta _{1}(t) U_{1}. \end{aligned} \end{aligned}$$

Now, in view of (2.3), the last inequality becomes

$$\begin{aligned} L U_{1}\leq {}& -\frac{1}{2H_{1}} \sum _{i=1}^{n}{} \bigl\{ 2H_{1}d_{i}-H_{1}(a+b_{i}+2)-2 \alpha ^{2}-A_{i}\gamma _{i} \bigr\} x^{2} \\ & {}-\sum_{i=1}^{n}{} \biggl\{ \frac{ab_{i}-c_{i}-2M_{i}-2b_{i}-6}{4}- \frac{D_{i}+\mu N_{i} A_{i} }{2H_{1}B_{i}}\gamma _{i} \biggr\} y^{2} \\ &{}-\sum_{i=1}^{n}{} \biggl\{ \frac{H_{1}(a-1)-2\mu}{2H_{1}}- \frac{B_{i}A_{i} +C_{i}}{2B_{i}H_{1}^{2}}\gamma _{i} \biggr\} z^{2} \\ &{}+\frac{ E_{3}}{E_{1}} \eta _{1}(t) U_{1}. \end{aligned}$$

Hence, for the positive constant $E_{4}>0$, we obtain

$$\begin{aligned} L U_{1}\leq - E_{4}\bigl(x^{2}+y^{2}+z^{2} \bigr)+\frac{ E_{3}}{E_{1}} \eta _{1}(t) U_{1}. \end{aligned}$$

Now, if we let

$$\begin{aligned} \begin{aligned} &\sigma _{1}(t)=\min \bigl\{ x'(0),x'(t)\bigr\} , \qquad \sigma _{2}(t)=\max \bigl\{ x'(0),x'(t) \bigr\} \\ & \sigma _{3}(t)=\min \bigl\{ x''(0),x''(t) \bigr\} ,\qquad \sigma _{4}(t)=\max \bigl\{ x''(0),x''(t) \bigr\} , \end{aligned} \end{aligned}$$

then we get

$$\begin{aligned} \begin{aligned} \omega (t)&= \int _{0}^{t}{\eta _{1}(s)\,ds } \\ &= \int _{0}^{t}{ \bigl\{ \bigl\vert \Phi _{1}(s) \bigr\vert + \bigl\vert \Phi _{2}(s) \bigr\vert \bigr\} }\,ds \\ &\leq \int _{\sigma _{1}(t)}^{\sigma _{2}(t)}{ \biggl\vert \frac{\partial \psi _{1}(u,v)}{\partial u} \biggr\vert \,du}+ \int _{\sigma _{3}(t)}^{ \sigma _{4}(t)}{ \biggl\vert \frac{\partial \psi _{1}(u,v)}{\partial v} \biggr\vert \,dv} + \int _{\sigma _{1}(t)}^{\sigma _{2}(t)}{ \biggl\vert \frac{H'(u)}{(H(u))^{2}} \biggr\vert \,du} \\ &\leq \int _{-\infty}^{\infty}{ \biggl\vert \frac{\partial \psi _{1}(u,v)}{\partial u} \biggr\vert \,du}+ \int _{-\infty}^{ \infty}{ \biggl\vert \frac{\partial \psi _{1}(u,v)}{\partial v} \biggr\vert \,dv} +\frac{1}{H_{1}^{2}} \int _{-\infty}^{\infty}{ \bigl\vert h'(u) \bigr\vert \,du}. \end{aligned} \end{aligned}$$

From the condition $(h_{6})$, it follows that

$$\begin{aligned} \omega (t)\leq D+ \frac{C}{H_{1}^{2}}< \infty . \end{aligned}$$

(2.12)

Because of

$$\begin{aligned} W_{1}=\exp \biggl(-\frac{\omega (t)}{\mu _{1}} \biggr)U_{1}, \qquad \mu _{1}=\frac{E_{1}}{E_{3}}. \end{aligned}$$

The stochastic derivative of the above equation is

$$\begin{aligned} L W_{1}\leq \exp \biggl(-\frac{\omega (t)}{\mu _{1}} \biggr) \biggl( L U_{1}-\frac{E_{3}}{E_{1}}\eta _{1}(t)U_{1} \biggr). \end{aligned}$$

Therefore, for the positive constant $D_{1}$, we conclude that

$$\begin{aligned} L W_{1}\leq - D_{1}\bigl(x^{2}+y^{2}+z^{2} \bigr). \end{aligned}$$

(2.13)

Hence, from the results (2.6), (2.8), and (2.13), all conditions of the Lemma of the stability in [8, 14] are satisfied. Therefore, the proof of Theorem 2.1 is now complete. □

3 Uniformly stochastically boundedness results

Theorem 3.1

Assume that the hypotheses $(h_{1})$–$(h_{8})$ hold true and suppose that there exist positive constants $F_{i}$, $K_{i}$, and m such that

$$\begin{aligned} F_{i} =a(a_{0}+1)+H_{1}( \mu +1)+1) (M_{i}+L_{i}) (a+1) +(a b_{i}-c_{i})L_{i}, \end{aligned}$$

(3.1)

and

$$\begin{aligned} K_{i}=H_{1}(\mu +1)+1+a(a_{0}+1)+H_{1}(ab_{i}-c_{i})N_{i}. \end{aligned}$$

(3.2)

Furthermore, we assume that

$$\begin{aligned} \alpha ^{2} < \sum_{i=1}^{n}{} \frac{H_{1} \{(ab_{i}-c_{i}+1)d_{i}-(a+b_{i}+2) \}}{a+1}, \end{aligned}$$

and

$$\begin{aligned} \bigl\vert \varepsilon \bigl(x,x',x'' \bigr) \bigr\vert \leq m. \end{aligned}$$

Provided that the positive constant $\gamma _{i}$ satisfies the following

$$\begin{aligned} \begin{aligned} \gamma _{i}\leq {}&\min \biggl[ \biggl\{ \frac{H_{1} ( H_{1}(ab_{i}-c_{i}+1)d_{i}-H_{1}(b_{i}+a+H_{1})-(a+1)\alpha ^{2} )}{(ab_{i}-c_{i}+H_{1})A_{i}} \biggr\} , \\ &{} \biggl\{ \frac{H_{1}^{2}B_{i}(ab_{i}-c_{i}+2ac_{i}-2M_{i}-2b_{i}-6)}{4(\mu B_{i} N_{i}(\mu H_{1}+aa_{0}) +F_{i}H_{1})} \biggr\} , \\ &{} \biggl\{ \frac{B_{i}H_{1}(H_{1}(a+1)-2\mu )}{2(B_{i}A_{i}(a_{0}+1) +K_{i})} \biggr\} \biggr]. \end{aligned} \end{aligned}$$

Then, all solutions of (1.1) are USB.

Proof

Here, consider $\varepsilon \neq 0$ and define the Lyapounov functional as follows

$$\begin{aligned} U(x_{t},y_{t},z_{t})=U_{1}(x_{t},y_{t},z_{t})+U_{2}(x_{t},y_{t},z_{t}), \end{aligned}$$

where $U_{1}$ is defined in (2.4), and we define $U_{2}$ as

$$\begin{aligned} \begin{aligned} U_{2}={}& a\frac{\psi _{1}(x,y)}{H(t)}\sum _{i=1}^{n}{ \int _{0}^{x}{f_{i}( \xi )\,d\xi}} +aa_{0} \int _{0}^{y}{\psi _{1}(x,\eta )\eta d \eta}+ a \sum_{i=1}^{n}{f_{i}(x)}y \\ &{}+ \frac{ 1}{2H_{1}}\sum_{i=1}^{n}{b_{i}(ab_{i}-c_{i})} x^{2} + \frac{1}{2}\sum_{i=1}^{n}{c_{i}}y^{2}+ \sum_{i=1}^{n}{(ab_{i}-c_{i})} \biggl(\frac{z}{H_{1}}+a_{0}y \biggr)x \\ &{}+\frac{a_{0}}{2H_{1}} z^{2} +\frac{aa_{0}}{H_{1}}yz+ \frac{a}{H_{1}} \alpha ^{2} \int _{t-l(t)}^{t}{x^{2}(s)\,ds.} \end{aligned} \end{aligned}$$

(3.3)

Since $\int _{t-l(t)}^{t}{x^{2}(s)\,ds}$ is nonnegative, recall the hypotheses $(h_{1})$–$(h_{4})$, and then $U_{2}$ becomes

$$\begin{aligned} U_{2}\geq{} & \frac{a^{2}}{H_{1}}\sum _{i=1}^{n}{ \int _{0}^{x}{f_{i}( \xi )\,d\xi}} + \frac{1}{2}a^{2}a_{0} y^{2}+ a \sum _{i=1}^{n}{f_{i}(x)}y +\frac{a_{0}}{2H_{1}} z^{2} +\frac{aa_{0}}{H_{1}}yz \\ &{}+ \frac{1}{2H_{1}}\sum_{i=1}^{n}{b_{i}(ab_{i}-c_{i})} x^{2} + \frac{1}{2}\sum_{i=1}^{n}{c_{i}}y^{2}+ \sum_{i=1}^{n}{(ab_{i}-c_{i})} \biggl(\frac{z}{H_{1}}+a_{0}y \biggr)x. \end{aligned}$$

Furthermore, we have $H_{2}\leq H(t)\leq H_{1} \leq 1$, $\frac{1}{H_{1}}\geq 1$, so the above inequality leads to the following

$$\begin{aligned} \begin{aligned} U_{2} \geq {}& \sum _{i=1}^{n}{\frac{a^{2}}{2c_{i}y^{2}} \biggl[ 4 \int _{0}^{x} {f_{i}(\xi )\,d\xi} \biggl\{ \int _{0}^{y}{ \bigl(c_{i}-f'_{i}( \xi ) \bigr) \biggr\} \eta \,d\eta} \biggr]}+\sum_{i=1}^{n}{ \frac{1}{2c_{i}} \bigl(c_{i}y+af_{i}(x) \bigr)^{2}} \\ &{}+ \sum_{i=1}^{n}{\frac{c_{i}}{2b_{i}}}(z+ay)^{2}+ \sum_{i=1}^{n}{ \frac{(ab_{i}-c_{i})}{2b_{i}} \bigl\{ b_{i}x+(z+ay) \bigr\} ^{2}}. \end{aligned} \end{aligned}$$

(3.4)

Therefore, from $(h_{2})$, we find

$$\begin{aligned} \begin{aligned} U_{2} \geq {}& \frac{a^{2}}{2}\sum _{i=1}^{n}{ \int _{0}^{x} {f_{i}(\xi )\,d\xi}}+\sum _{i=1}^{n}{\frac{1}{2c_{i}} \bigl(c_{i}y+af_{i}(x) \bigr)^{2}} \\ &{}+ \sum_{i=1}^{n}{\frac{c_{i}}{2b_{i}}}(z+ay)^{2}+ \sum_{i=1}^{n}{ \frac{(ab_{i}-c_{i})}{2b_{i}} \bigl\{ b_{i}x+(z+ay) \bigr\} ^{2}}. \end{aligned} \end{aligned}$$

We can find a positive constant $\varphi _{1}$ such that the last inequality gives

$$\begin{aligned} U_{2}\geq \varphi _{1}\bigl(x^{2}+y^{2}+z^{2} \bigr). \end{aligned}$$

(3.5)

Thus, from (2.5) and (3.4), we conclude

$$\begin{aligned} \begin{aligned} U \geq {}& \sum_{i=1}^{n}{ \biggl(\frac{a^{2}}{2}+ \frac{ab_{i}-c_{i}}{4b_{i}} \biggr) \int _{0}^{x} {f_{i}(\xi )\,d\xi}}+ \sum _{i=1}^{n}{\frac{1}{2c_{i}} \bigl(c_{i}y+af_{i}(x) \bigr)^{2}} \\ &{}+ \sum_{i=1}^{n}{\frac{c_{i}}{2b_{i}}}(z+ay)^{2}+ \sum_{i=1}^{n}{ \frac{(ab_{i}-c_{i})}{2b_{i}} \bigl\{ b_{i}x+(z+ay) \bigr\} ^{2}} \\ &{}+\sum_{i=1}^{n}{\frac{1}{2b_{i}} \bigl(b_{i}y+f_{i}(x)\bigr)^{2}} + \biggl( \mu y+ \frac{z}{2} \biggr)^{2}+ \biggl(x+\frac{z}{2} \biggr)^{2}+ \frac{1}{2}\mu \biggl({\frac{ab_{i}-c_{i}}{2b_{i}}} \biggr)y^{2}. \end{aligned} \end{aligned}$$

Hence, for the positive constant $\varphi _{2}$, we get

$$\begin{aligned} U(x_{t},y_{t},z_{t}) \geq \varphi _{2}\bigl(x^{2}+y^{2}+z^{2}\bigr). \end{aligned}$$

(3.6)

Since $|\frac{\partial Q_{i}}{\partial x}| \leq M_{i}$, $|f'_{i}(x)|\leq L_{i}$, $a \leq \psi _{1} \leq a_{0}$ and $H_{1}\leq H(t) \leq H_{2} \leq 1$, we can rewrite (3.3) in the following form

$$\begin{aligned} \begin{aligned} U_{2} \leq {}& \frac{a a_{0}}{ H_{1}} \sum _{i=1}^{n}{L_{i}} x^{2} + \frac{aa_{0}}{2}y^{2}+ \frac{a}{2}\sum _{i=1}^{n}{L_{i}}xy + \frac{ 1}{H_{1}} \sum_{i=1}^{n}{b_{i}(ab_{i}-c_{i})}x^{2} \\ & +\frac{1}{2}\sum_{i=1}^{n}{c_{i}}y^{2}+ \biggl(\frac{z}{H_{1}}+a_{0}y \biggr)x\sum _{i=1}^{n}{b_{i}(ab_{i}-c_{i})} \\ &{}+\frac{a_{0}}{2H_{1}} z^{2} +\frac{aa_{0}}{H_{1}}yz+ \frac{a}{H_{1}} \alpha ^{2} l(t) \Vert x \Vert ^{2}. \end{aligned} \end{aligned}$$

Applying the inequality $2pq\leq (p^{2}+q^{2})$ and using the condition $0< l(t)\leq l_{1}$, it tends to

$$\begin{aligned} \begin{aligned} U_{2} \leq {}& \frac{1}{ 2H_{1}} \sum_{i=1}^{n}{} \bigl\{ aL_{i}(1+a_{0})+(ab_{i}-c_{i}) (b_{i}+1+a_{0}H_{1})+2a \alpha ^{2} l_{1} \bigr\} \Vert x \Vert ^{2} \\ &{}+ \frac{1}{ 2H_{1}} \sum_{i=1}^{n}{} \bigl\{ aa_{0}(H_{1}+1)+H_{1} \bigl(aL_{i}+c_{i}+a_{0}(ab_{i}-c_{i}) \bigr) \bigr\} \Vert y \Vert ^{2} \\ & {}+\frac{1}{ 2H_{1}} \sum_{i=1}^{n}{} \bigl\{ a_{0}(a+1)+ab_{i}-c_{i} \bigr\} \Vert z \Vert ^{2}. \end{aligned} \end{aligned}$$

(3.7)

Then, with $\varphi _{3}>0$, we have

$$\begin{aligned} U_{2}(x_{t},y_{t},z_{t}) \leq \varphi _{3}\bigl(x^{2}+y^{2}+z^{2} \bigr). \end{aligned}$$

(3.8)

Combining the inequality (2.7) with (3.7), we conclude

$$\begin{aligned} \begin{aligned} U \leq {}& \frac{1}{ 2H_{1}} \sum _{i=1}^{n}{} \bigl\{ L_{i} \bigl(H_{1}( \mu +1)+a(1+a_{0}) \bigr)+(ab_{i}-c_{i}) (b_{i}+1+a_{0}H_{1}) \\ &{}+3H_{1}+\alpha ^{2} l_{1}(2a+1) \bigr\} \Vert x \Vert ^{2}+ \frac{1}{ 2H_{1}} \sum_{i=1}^{n}{} \bigl\{ \mu aa_{0}( H_{1}+1) \\ &{}+a(a_{0}+1)+(L_{i}+M_{i})+H_{1} \bigl(aL_{i}+c_{i}+a_{0}(ab_{i}-c_{i}) \bigr)+\gamma _{i}^{2} \lambda _{i} \bigr\} \Vert y \Vert ^{2} \\ &{}+ \frac{1}{ 2H_{1}} \sum_{i=1}^{n}{} \bigl\{ H_{1}(\mu +1)+1+a_{0}(a+1)+ab_{i}-c_{i}+ \gamma _{i}^{2} \delta _{i} \bigr\} \Vert z \Vert ^{2}. \end{aligned} \end{aligned}$$

Hence, for the positive constant $\varphi _{4}$, the last inequality gives

$$\begin{aligned} U(x_{t},y_{t},z_{t}) \leq \varphi _{4}\bigl(x^{2}+y^{2}+z^{2}\bigr). \end{aligned}$$

(3.9)

In view of the hypothesis of Theorem 3.1 and the Itô formula, the derivative of the Lyapunov functional (3.3) with respect to the system (1.2) becomes

$$\begin{aligned} \begin{aligned} L U_{2}\leq {}& a \biggl(\frac{\psi _{1}(x,y)}{H(t)} \biggr)'\sum_{i=1}^{n}{ \int _{0}^{x}{f_{i}(\xi )\,d\xi}} - \frac{1}{H_{1}}\Phi _{1}(t) \sum_{i=1}^{n}{(ab_{i}-c_{i})}x y -\frac{ayz}{H_{1}}\Phi _{1}(t) \\ &{}-\frac{a a_{0}}{H_{1}}\Phi _{1}(t)y^{2}+aa_{0} \int _{0}^{y}{y \frac{\partial \psi _{1}(x,\eta )}{\partial x}\eta d \eta} - \frac{1}{H_{1}}\sum_{i=1}^{n}{(ab_{i}-c_{i})f_{i}(x)}x \\ &{}-\frac{a}{2}\sum_{i=1}^{n}{c_{i}}y^{2} +\frac{a}{2H_{1}}\alpha ^{2}x^{2}\bigl(t-l(t)\bigr) - \frac{a}{H_{1}}\alpha ^{2}x^{2}\bigl(t-l(t)\bigr) \bigl(1-l'(t)\bigr) \\ &{}+\frac{a}{H_{1}}\alpha ^{2}x^{2}(t)+\frac{m}{H_{1}} \sum_{i=1}^{n}{(ab_{i}-c_{i})} \vert x \vert + \frac{a a_{0} m}{H_{1}} \vert y \vert +\frac{a_{0} m}{H_{1}} \vert z \vert \\ &{}+ \Biggl\{ \frac{1}{H_{1}}\sum_{i=1}^{n}{(ab_{i}-c_{i})}x+ \frac{a a_{0} }{H_{1}}y+\frac{a_{0} }{H_{1}}z \Biggr\} \Biggl[\sum _{i=1}^{n}{ \int _{t-r_{i}(t)}^{t} \biggl\{ { \frac{\partial Q_{i}(x(s),y(s))}{\partial x}y(s)}} \\ &{}+\frac{\partial Q_{i}(x(s),y(s))}{\partial y}\frac{z(s)}{H(s)}+ f'\bigl(x(s)\bigr)y(s)\,ds \biggr\} \Biggr]. \end{aligned} \end{aligned}$$

Now, we choose

$$\begin{aligned} \begin{aligned} \Phi _{4}(t) =& a\Phi _{3} \sum _{i=1}^{n}{ \int _{0}^{x}{f_{i}(\xi )\,d\xi}} - \frac{1}{H_{1}}\Phi _{1}(t) \sum_{i=1}^{n}{(ab_{i}-c_{i})}x y - \frac{ayz}{H_{1}}\Phi _{1}(t)-\frac{a a_{0}}{H_{1}}\Phi _{1}(t)y^{2}. \end{aligned} \end{aligned}$$

Since $|f_{i}'(x)|\leq L_{i}$, we obtain

$$\begin{aligned} \begin{aligned} \bigl\vert \Phi _{4}(t) \bigr\vert \leq{}& \frac{a}{2} \bigl\vert \Phi _{3}(t) \bigr\vert \sum _{i=1}^{n}{L_{i}}x^{2}+ \frac{1}{H_{1}} \bigl\vert \Phi _{1}(t) \bigr\vert \sum _{i=1}^{n}{(ab_{i}-c_{i})} \vert x \vert \vert y \vert \\ &{} +\frac{a \vert y \vert \vert z \vert }{H_{1}} \bigl\vert \Phi _{1}(t) \bigr\vert + \frac{a a_{0}}{H_{1}} \bigl\vert \Phi _{1}(t) \bigr\vert y^{2}. \end{aligned} \end{aligned}$$

Using the fact that $2pq\leq (p^{2}+q^{2})$, we get

$$\begin{aligned} \begin{aligned} \bigl\vert \Phi _{4}(t) \bigr\vert \leq & \sum_{i=1}^{n}{ \biggl\{ \frac{1}{2}L_{i}+ \frac{1}{H_{1}}(ab_{i}-c_{i}) +\frac{a}{H_{1}}+\frac{aa_{0}}{H_{1}} \biggr\} } \bigl( \bigl\vert \Phi _{1}(t) \bigr\vert + \bigl\vert \Phi _{3}(t) \bigr\vert \bigr) \bigl(x^{2}+y^{2}+z^{2} \bigr). \end{aligned} \end{aligned}$$

If we let

$$\begin{aligned} \eta _{2}(t)= \bigl\vert \Phi _{1}(t) \bigr\vert + \bigl\vert \Phi _{3}(t) \bigr\vert , \end{aligned}$$

then from (3.5), we conclude

$$\begin{aligned} \begin{aligned} \bigl\vert \Phi _{4}(t) \bigr\vert \leq \frac{ \varphi _{5}}{\varphi _{1}}U_{2}\eta _{2}(t), \end{aligned} \end{aligned}$$

(3.10)

where

$$\begin{aligned} \varphi _{5}=\max \biggl\{ \frac{1}{2}L_{i}, \frac{1}{H_{1}}(ab_{i}-c_{i}) +\frac{a}{H_{1}}+ \frac{aa_{0}}{H_{1}} \biggr\} . \end{aligned}$$

Considering the conditions $l(t)\leq \frac{1}{2}$, $y\frac{\partial \psi _{1}(x,y)}{\partial x}\leq 0$ and using equation (3.10), we find

$$\begin{aligned} \begin{aligned} L U_{2}\leq {}&\frac{\varphi _{5}}{\varphi _{1}}\eta _{2}(t) U_{2}- \frac{1}{H_{1}}\sum _{i=1}^{n}{(ab_{i}-c_{i})f_{i}(x)}x- \frac{a}{2} \sum_{i=1}^{n}{c_{i}}y^{2} \\ &{}+\frac{a}{H_{1}}\alpha ^{2}x^{2}(t)+\frac{m}{H_{1}} \sum_{i=1}^{n}{(ab_{i}-c_{i})} \vert x \vert + \frac{a a_{0} m}{H_{1}} \vert y \vert +\frac{a_{0} m}{H_{1}} \vert z \vert \\ &{}+ \Biggl\{ \frac{1}{H_{1}}\sum_{i=1}^{n}{(ab_{i}-c_{i})}x+ \frac{a a_{0} }{H_{1}}y+\frac{a_{0} }{H_{1}}z \Biggr\} \Biggl[\sum _{i=1}^{n}{ \int _{t-r_{i}(t)}^{t} \biggl\{ { \frac{\partial Q_{i}(x(s),y(s))}{\partial x}y(s)}} \\ &{}+\frac{\partial Q_{i}(x(s),y(s))}{\partial y}\frac{z(s)}{H(s)}+ f'\bigl(x(s)\bigr)y(s)\,ds \biggr\} \Biggr]. \end{aligned} \end{aligned}$$

Now, from the hypotheses $(h_{2})$ and $(h_{4})$, we obtain

$$\begin{aligned} \begin{aligned} L U_{2} \leq {}& \frac{\varphi _{5}}{\varphi _{1}}\eta _{2}(t)U_{2} + \Biggl\{ \frac{1}{H_{1}}\sum_{i=1}^{n}{(ab_{i}-c_{i})} \vert x \vert + \frac{a a_{0} }{H_{1}} \vert y \vert +\frac{a_{0} }{H_{1}} \vert z \vert \Biggr\} m \\ &{}+ \Biggl\{ \sum_{i=1}^{n}{(a b_{i}-c_{i})d_{i}- \frac{a \alpha ^{2}}{H_{1}}-\sum _{i=1}^{n}{ \biggl( \frac{(ab_{i}-c_{i}) (H_{1}(M_{i}+L_{i})+N_{i} ) }{2 H_{1}^{2}}\gamma _{i} \biggr)} \Biggr\} }x^{2} \\ &{}- \Biggl\{ \frac{a}{2}\sum_{i=1}^{n}{c_{i}} -\sum_{i=1}^{n}{ \biggl( \frac{aa_{0}(H_{1}(M_{i}+L_{i})+N_{i})}{2H_{1}^{2}} \gamma _{i} \biggr)} \Biggr\} y^{2} \\ &{}+\frac{a_{0}}{2H_{1}}\sum_{i=1}^{n}{ \bigl\{ \bigl(H_{1}(M_{i}+L_{i})+N_{i} \bigr) \gamma _{i} \bigr\} }z^{2} \\ &{} +\frac{1}{2H_{1}}\sum_{i=1}^{n}{{ \bigl\{ a_{0}(M_{i}+L_{i}) (a+1)+(ab_{i}-c_{i})L_{i} \bigr\} } \int _{t-r_{i}(t)}^{t}{y^{2}(\theta )\,d\theta}} \\ &{}+\frac{1}{2H^{2}_{1}}\sum_{i=1}^{n}{{ \bigl\{ a(a_{0}+1)+H_{1}(ab_{i}-c_{i}) \bigr\} } \int _{t-r_{i}(t)}^{t}{z^{2}(\theta )\,d\theta}}. \end{aligned} \end{aligned}$$

(3.11)

By compiling the above inequality with (2.11), from (3.1) and (3.2), we conclude

$$\begin{aligned} \begin{aligned} L U \leq {}& \Biggl\{ \frac{1}{H_{1}} \sum _{i=1}^{n}{(ab_{i}-c_{i}+1)} \vert x \vert + \biggl(\mu +\frac{a a_{0} }{H_{1}} \biggr) \vert y \vert + \frac{(a_{0}+1) }{H_{1}} \vert z \vert \Biggr\} m+\frac{E_{3}}{E_{1}} \eta _{1}(t) U_{1} \\ &{}+\frac{\varphi _{5}}{\varphi _{1}}\eta _{2}(t)U_{2}- \Biggl\{ \sum _{i=1}^{n}{(a b_{i}-c_{i}+1)d_{i}- \frac{1}{2}\sum_{i=1}^{n}{(a+b_{i}+2)}- \frac{(a+1) \alpha ^{2}}{H_{1}}} \\ &{}-\sum_{i=1}^{n}{ \biggl( \frac{(ab_{i}-c_{i}+H_{1})A_{i} }{2 H_{1}^{2}}\gamma _{i} \biggr)} \Biggr\} x^{2}- \Biggl\{ \sum_{i=1}^{n}{ \biggl(\frac{ab_{i}-c_{i}+2ac_{i}-2M_{i}-2b_{i}-6}{4} \biggr)} \\ &{}-\sum_{i=1}^{n}{ \biggl( \frac{(\mu H_{1}+aa_{0})A_{i}}{2H_{1}^{2}} \gamma _{i}-\lambda _{i} \gamma _{i} \biggr)} \Biggr\} y^{2} \\ &{} - \frac{1}{2 H_{1}} \Biggl\{ H_{1} (a+1)- 2 \mu - \frac{1}{H_{1}}(a_{0} +1) \sum_{i=1}^{n}{ A_{i}} \gamma _{i} -\delta _{i}\gamma _{i} \Biggr\} z^{2} \\ &{}+ \sum_{i=1}^{n}{} \biggl\{ \frac{1}{2H_{1}}F_{i}-(1-\beta _{i}) \lambda _{i} \biggr\} \int _{t-r_{i}(t)}^{t}{y^{2}(\theta )\,d\theta} \\ &{}+\sum_{i=1}^{n}{} \biggl\{ \frac{1}{2H_{1}^{2}}K_{i} -(1-\beta _{i}) \delta _{i} \biggr\} \int _{t-r_{i}(t)}^{t}{z^{2}(\theta )\,d\theta}. \end{aligned} \end{aligned}$$

We take

$$\begin{aligned} \lambda _{i} =&\frac{F_{i}}{2H_{1} (1-\beta _{i})} \quad \text{and}\quad \delta _{i}= \frac{K_{i}}{2H_{1}^{2} (1-\beta _{i})}. \end{aligned}$$

Therefore, from (2.3) and since $B_{i}=(1-\beta _{i})$, we obtain

$$\begin{aligned} L U \leq {}& \Biggl\{ \frac{1}{H_{1}} \sum _{i=1}^{n}{(ab_{i}-c_{i}+1)} \vert x \vert + \biggl(\mu +\frac{a a_{0} }{H_{1}} \biggr) \vert y \vert + \frac{(a_{0}+1) }{H_{1}} \vert z \vert \Biggr\} m+\frac{E_{3}}{E_{1}} \eta _{1}(t) U_{1} \\ &{}+\frac{\varphi _{5}}{\varphi _{1}}\eta _{2}(t)U_{2}- \Biggl\{ \sum _{i=1}^{n}{(a b_{i}-c_{i}+1)d_{i}- \sum_{i=1}^{n}{(a+b_{i}+1)}- \frac{(a+1) \alpha ^{2}}{H_{1}}} \\ &{}-\sum_{i=1}^{n}{ \biggl( \frac{(ab_{i}-c_{i}+H_{1})A_{i} }{2 H_{1}^{2}}\gamma _{i} \biggr)} \Biggr\} x^{2}-\sum _{i=1}^{n}{ \biggl\{ \frac{ab_{i}-c_{i}+2ac_{i}-2M_{i}-2b_{i}-6}{4}} \\ &{}-{ \frac{(\mu H_{1}+aa_{0})A_{i}}{2H_{1}^{2}} \gamma _{i}- \frac{F_{i}}{2H_{1} B_{i}} \gamma _{i}} \biggr\} y^{2} \\ &{} - \Biggl\{ \frac{1}{2 H_{1}}\bigl( H_{1} (a+1)- 2 \mu \bigr) - \frac{1}{2H_{1}^{2}} \sum_{i=1}^{n}{ \frac{(a_{0} +1)B_{i}A_{i}+K_{i}}{ B_{i}}} \gamma _{i} \Biggr\} z^{2}. \end{aligned}$$

Therefore, we can write the above inequality as follows

$$\begin{aligned} L U\leq{}& \frac{E_{3}}{E_{1}} \eta _{1}(t) U_{1}+ \frac{\varphi _{5}}{\varphi _{1}}\eta _{2}(t)U_{2} -\zeta \bigl(x^{2}+y^{2}+z^{2}\bigr)+ \kappa \zeta \bigl( \vert x \vert + \vert y \vert + \vert z \vert \bigr) \\ ={}&\frac{E_{3}}{E_{1}} \eta _{1}(t) U_{1}+ \frac{\varphi _{5}}{\varphi _{1}}\eta _{2}(t)U_{2} -\frac{\zeta}{2} \bigl(x^{2}+y^{2}+z^{2} \bigr) \\ &{} -\frac{\zeta}{2} \bigl\{ \bigl( \vert x \vert -\kappa \bigr)^{2}+\bigl( \vert y \vert -\kappa \bigr)^{2}+\bigl( \vert z \vert - \kappa \bigr)^{2} \bigr\} +\frac{3\zeta}{2}\kappa ^{2} \\ \leq{}& \frac{E_{3}}{E_{1}} \eta _{1}(t) U_{1}+ \frac{\varphi _{5}}{\varphi _{1}}\eta _{2}(t)U_{2}-\frac{\zeta}{2} \bigl(x^{2}+y^{2}+z^{2}\bigr)+ \frac{3\zeta}{2} \kappa ^{2},\quad \text{for some }\kappa ,\zeta >0, \end{aligned}$$

where

$$\begin{aligned} \kappa = m \max \biggl\{ ab_{i}-c_{i}+1, \mu + \frac{a a_{0} }{H_{1}}, \frac{a_{0}+1}{H_{1}} \biggr\} . \end{aligned}$$

From (2.8) and (3.8), we obtain the following estimate

$$\begin{aligned} \begin{aligned} \Phi _{5}(t)&=\frac{E_{3}}{E_{1}} \eta _{1}(t) U_{1}+ \frac{\varphi _{5}}{\varphi _{1}}\eta _{2}(t)U_{2} \\ & \leq \frac{E_{3}}{E_{1}} \eta _{1}(t) E_{2} \bigl(x^{2}+y^{2}+z^{2}\bigr)+ \frac{\varphi _{5}}{\varphi _{1}} \eta _{2}(t)\varphi _{3} \bigl(x^{2}+y^{2}+z^{2} \bigr) \\ & \leq \varphi _{6} \bigl( \bigl\vert \eta _{1}(t) \bigr\vert + \bigl\vert \eta _{2}(t) \bigr\vert \bigr) \bigl(x^{2}+y^{2}+z^{2}\bigr), \end{aligned} \end{aligned}$$

where

$$\begin{aligned} \begin{aligned} \varphi _{6}=\max \biggl\{ \frac{E_{2} E_{3}}{E_{1}}, \frac{\varphi _{3}\varphi _{5}}{\varphi _{1}} \biggr\} . \end{aligned} \end{aligned}$$

According to inequality (3.6), we conclude

$$\begin{aligned} \begin{aligned} \Phi _{5}(t) \leq \frac{ \varphi _{6}}{\varphi _{2}} \bigl( \bigl\vert \eta _{1}(t) \bigr\vert + \bigl\vert \eta _{2}(t) \bigr\vert \bigr) U. \end{aligned} \end{aligned}$$

It follows that

$$\begin{aligned} L U\leq \frac{\varphi _{6}}{\varphi _{2}} \bigl( \bigl\vert \eta _{1}(t) \bigr\vert + \bigl\vert \eta _{2}(t) \bigr\vert \bigr) U-\frac{\zeta}{2}\bigl(x^{2}+y^{2}+z^{2} \bigr)+\frac{3\zeta}{2}\kappa ^{2}. \end{aligned}$$

(3.12)

Define the Lyapunov functional $W_{2}(x_{t},y_{t},z_{t})$ as follows

$$\begin{aligned} W_{2}=\exp{ \biggl\{ -\frac{\varphi _{6}}{\varphi _{2}}\eta _{3}(t) \biggr\} U(x_{t},y_{t},z_{t})}, \end{aligned}$$

where

$$\begin{aligned} \eta _{3}(t)= \int _{0}^{t}{ \bigl\{ \eta _{1}(t)+\eta _{2}(t) \bigr\} \,d\eta}. \end{aligned}$$

Then, from the hypotheses $h_{1}$ and $h_{3}$ and (2.12), we conclude

$$\begin{aligned} \begin{aligned} \eta _{3}(t)={}& \int _{0}^{t}{ \bigl(\eta _{1}(s)+\eta _{2}(s) \bigr)\,ds } \leq D+\frac{C}{H_{1}^{2}}+ \int _{0}^{t}{ \bigl\{ \bigl\vert \Phi _{1}(s) \bigr\vert + \bigl\vert \Phi _{3}(s) \bigr\vert \bigr\} }\,ds \\ \leq{}& 2D+\frac{C}{H_{1}^{2}}+\frac{1}{H_{1}} \int _{\sigma _{1}(t)}^{ \sigma _{2}(t)}{ \biggl\vert \frac{\partial \psi _{1}(u,v)}{\partial u} \biggr\vert \,du}+\frac{1}{H_{1}} \int _{\sigma _{3}(t)}^{\sigma _{4}(t)}{ \biggl\vert \frac{\partial \psi _{1}(u,v)}{\partial v} \biggr\vert \,dv} \\ &{} +\frac{a_{0}}{H_{1}^{2}} \int _{\sigma _{1}(t)}^{\sigma _{2}(t)}{ \bigl\vert H'(u) \bigr\vert \,du} \\ \leq{}& 2D+\frac{C}{H_{1}^{2}}+\frac{1}{H_{1}} \int _{-\infty}^{\infty}{ \biggl\vert \frac{\partial \psi _{1}(u,v)}{\partial u} \biggr\vert \,du}+ \frac{1}{H_{1}} \int _{-\infty}^{\infty}{ \biggl\vert \frac{\partial \psi _{1}(u,v)}{\partial v} \biggr\vert \,dv} \\ &{} +\frac{a_{0}}{H_{1}^{2}} \int _{-\infty}^{\infty}{ \bigl\vert h'(u) \bigr\vert \,du}. \end{aligned} \end{aligned}$$

It follows form $(h_{8})$ that

$$\begin{aligned} \eta _{3}(t) \leq D \biggl( 2+ \frac{1}{H_{1}} \biggr)+ \frac{C}{H_{1}^{2}}(a_{0}+1)< \infty . \end{aligned}$$

Then, the stochastically derivative of $W_{2}$ becomes

$$\begin{aligned} L W_{2}=\exp{ \biggl\{ -\frac{\varphi _{6}}{\varphi _{2}}\eta _{3}(t) \biggr\} \biggl\{ L U- \frac{\varphi _{6}}{\varphi _{2}} \bigl( \bigl\vert \eta _{1}(t) \bigr\vert + \bigl\vert \eta _{2}(t) \bigr\vert \bigr)U \biggr\} }. \end{aligned}$$

Hence, from (3.12), we find

$$\begin{aligned} L W_{2} \leq M \biggl\{ -\frac{\zeta}{2} \bigl(x^{2}+y^{2}+z^{2}\bigr)+ \frac{3\zeta}{2} \kappa ^{2} \biggr\} ,\quad \text{for some } M>0. \end{aligned}$$

(3.13)

Thus, from inequalities (3.6) and (3.9) and by taking $\nu (t)=\zeta /2$, $\rho _{4}(t)=(3\zeta /2)\kappa ^{2}$ and $n=2$, we see that the conditions (i) and (ii) of Lemma 2.4 in [8, 14] are satisfied. As well as we can test that the condition (iii) is satisfied with $q_{1}=q_{2}=n=2$ with $\rho _{3} =0$. Then, all conditions of Lemma 2.4 in [8, 14] are achieved.

So, with $\nu (t)=\zeta /2$, $\beta (t)=(3\zeta /2)\kappa ^{2}$, $n=2$, and $\rho _{3} =0$, we note that

$$ \int ^{t}_{t_{0}}{\bigl\{ \rho _{3} \nu (u)+ \rho _{4}(u)\bigr\} e^{-\int ^{t}_{u}{ \nu (s)}\,ds}\,du}=(3\zeta /2)\kappa ^{2} \int ^{t}_{t_{0}}{e^{- \frac{\zeta}{2}\int ^{t}_{u}{ \,ds}}\,du} \leq 3\kappa ^{2}, $$

for all $t\geq t_{0}\geq 0$. Thus, condition (2.4) [8] holds. Now, since

$$\begin{aligned} &g^{T}= \bigl(0 0 -\alpha x\bigl(t-l(t)\bigr) \bigr), \\ & U_{x}=(U_{1})_{x}+(U_{2})_{x} \\ & \hphantom{U_{x}}=\mu \sum_{i=1}^{n}{f_{i}(x)}+ \sum_{i=1}^{n}{f_{i}(x)}+2x+z+ \frac{a\psi '_{i}(x,y)}{H(t)}\sum_{i=1}^{n}{ \int _{i=0}^{x}{f_{i}( \xi )\,d\xi }} \\ & \hphantom{U_{x}=}{}+\frac{a\psi _{i}(x,y)}{H(t)}\sum_{i=1}^{n}{f_{i}( \xi ) }+ \sum_{i=1}^{n}{\frac{b_{i}}{H_{1}}(ab_{i}-c_{i})}x+ \sum_{i=1}^{n}{(ab_{i}-c_{i}) \biggl( \frac{z}{H_{1}}+a_{0} y\biggr)}, \\ &U_{y}=(U_{1})_{y}+(U_{2})_{y} \\ &\hphantom{U_{y}} =\sum_{i=1}^{n}{f_{i}(x)} +\mu \psi _{i}(x,y) y+\sum_{i=1}^{n}{Q_{i}(x,y)}+ \mu z +aa_{0} \psi _{1}(x,y)y \\ &\hphantom{U_{y}=}{} + a\sum_{i=1}^{n}{f_{i}(x)}+a_{0} x y\sum_{i=1}^{n}{c_{i}}+ \sum _{i=1}^{n}{ab_{i}-c_{i}}+ \frac{a a_{0}}{H_{1}}z, \\ &U_{z}=(U_{1})_{z}+(U_{2})_{z}= \mu y+\frac{1}{H(t)}z+x + \frac{x}{H_{1}}\sum _{i=1}^{n}{(a b_{i}-c_{i})}x+ \frac{a}{H_{1}}z+ \frac{aa_{0}}{H_{1}}y, \end{aligned}$$

we have

$$\begin{aligned} \begin{aligned} \bigl\vert U_{x_{i}}(t,x)G_{ik}(t,x) \bigr\vert \leq{} & \alpha \biggl[ \biggl\{ \frac{H_{1}(\mu +1)+3+a(a_{0}+1)}{2H_{1}} \biggr\} x^{2}\bigl(t-l(t)\bigr) \\ &{} + \biggl(\frac{H_{1}+\sum_{i=1}^{n}{(ab_{i}-c_{i})}}{2H_{1}} \biggr)x^{2}+ \biggl( \frac{\mu +aa_{0}}{2} \biggr)y^{2} \\ & {}+ \biggl(\frac{a+1}{2H_{1}} \biggr) z^{2} \biggr]:=\chi (t). \end{aligned} \end{aligned}$$

Thus, condition (2.3) in [8, 14] is satisfied. Using Lemma 2.4 in [8, 14], we find that all solutions of (1.1) are USB, and we can also conclude

$$\begin{aligned} E^{x_{0}} \bigl\Vert x(t,t_{0},x_{0}) \bigr\Vert \leq \bigl\{ \mathcal{C} x_{0}^{2}+3\kappa ^{2} \bigr\} ^{\frac{1}{2}},\quad \text{for all } t\geq t_{0}\geq 0. \end{aligned}$$

Hence, the proof of Theorem 3.1 is now complete. □

4 Examples and discussion

Example 4.1

In a particular case $n=1$, consider the following third-order SDDE

$$\begin{aligned} \begin{aligned} & \biggl\{ \biggl(\frac{3}{4}+ \frac{1}{4}e^{-4x'} \biggr) x'' \biggr\} '+ \biggl\{ \biggl(19+\frac{\pi}{2}+\arctan \bigl(x x'\bigr) \biggr)x' \biggr\} '+9 x \bigl(t-r(t)\bigr) x'\bigl(t-r(t)\bigr) \\ &\quad {} +\sin{ \bigl(x\bigl(t-r(t)\bigr) x'\bigl(t-r(t)\bigr) \bigr)}+25x \bigl(t-r(t)\bigr) \\ &\quad {} +\frac{x(t-r(t))}{1+x(t-r(t))}+\frac{1}{4}\sin \biggl( x\biggl(t- \frac{1}{2}e^{-t}\biggr)\biggr)=0. \end{aligned} \end{aligned}$$

(4.1)

The equivalent system of (4.1) is

$$\begin{aligned}& x '=y, \\& y '=\frac{z}{\frac{3}{4}+\frac{1}{4}e^{-4y}}, \\& z'=- \biggl(19 +\frac{\pi}{2}+\arctan (x y) \biggr) \frac{z}{\frac{3}{4}+\frac{1}{4}e^{-4y}} \\& \hphantom{z'=}{} -y \biggl( \frac{-y^{2}}{1+x^{2} y^{2}} - \frac{x}{1+x^{2} y^{2}}\frac{z}{\frac{3}{4}+\frac{1}{4}e^{-4y}} \biggr)-\bigl(9 xy +\sin{(xy)}\bigr) \\& \hphantom{z'=}{} + \int _{t-r(t)}^{t}{ \bigl(9 y(s)+y(s)\cos \bigl(x(s)y(s) \bigr) \bigr)y(s)\,ds} \\& \hphantom{z'=}{} + \int _{t-r(t)}^{t}{ \bigl(9 y(s)+y(s)\cos \bigl(x(s)y(s) \bigr) \bigr) \frac{z(s)}{\frac{3}{4}+\frac{1}{4}e^{-4y(s)}}\,ds}- \biggl\{ 25 x+ \frac{x}{1+x^{4}} \biggr\} \\& \hphantom{z'=}{} + \int _{t-r(t)}^{t}{ \biggl\{ 25 x(s)+ \frac{1-2x^{4}(s)}{1+x^{4}(s)} \biggr\} \,ds}-\frac{1}{4}\sin \biggl(x\biggl(t- \frac{1}{4}e^{t} \biggr)\biggr). \end{aligned}$$

(4.2)

Comparing equation (1.2) with (4.2), we have

$$\begin{aligned} h(y)=\frac{3}{4}+\frac{1}{4}e^{-4y },\quad \text{then } \frac{3}{4} \leq h(y)\leq 1. \end{aligned}$$

Therefore, we get

$$\begin{aligned} H_{1}=\frac{3}{4}, \qquad H_{2}=1. \end{aligned}$$

The derivative of $h(y)$ is

$$\begin{aligned} h'(y)=-e^{-4y }. \end{aligned}$$

Then, we find

$$\begin{aligned} \int _{-\infty}^{\infty}{ \bigl\vert -e^{-4v} \bigr\vert \,dv} =&2 \int _{0}^{\infty}{ \bigl\vert e^{-4v} \bigr\vert \,dv}= \frac{1}{2}=C< \infty . \end{aligned}$$

(4.3)

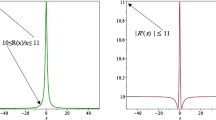
We can see that Fig. 1 illustrates the behavior of $h(y)$ in the interval $x\in [0,50]$.

Figure 1

Trajectory of $h(y)$

We also have the function

$$\begin{aligned} \psi _{1}(x,y)=19+\frac{\pi}{2}-\arctan (xy), \quad \text{so } 19 \leq \psi _{1}(x,y)\leq 19+\frac{\pi}{2}, \end{aligned}$$

then, we get $a=19$ and $a_{0}=19+\frac{\pi}{2}$.

We also obtain

$$\begin{aligned} \frac{\partial \psi _{1}(x,y)}{\partial x}=\frac{-y}{1+x^{2}y^{2}}, \quad \text{so } y \frac{\partial \psi _{1}(x,y)}{\partial x}= \frac{-y^{2}}{1+x^{2}y^{2}}\leq 0, \end{aligned}$$

and

$$\begin{aligned} \frac{\partial \psi _{1}(x,y)}{\partial y}=\frac{-x}{1+x^{2}y^{2}}. \end{aligned}$$

Therefore, we can conclude

$$\begin{aligned} \int _{-\infty}^{\infty}{ \biggl\vert \frac{-v}{1+u^{2}v^{2}} \biggr\vert \,du}+ \int _{-\infty}^{\infty}{ \biggl\vert \frac{-u}{1+u^{2}v^{2}} \biggr\vert \,dv}=2 \pi =D < \infty . \end{aligned}$$

(4.4)

Figure 2 shows the behavior of the function $\psi _{1}(x,y)$ through the interval $x\in [-4,4]$, $y\in [-4,4]$, and also it shows that $y \frac{\partial \psi _{1}}{\partial x}<0$, for all x, y.

Figure 2

Trajectory of $\psi _{1}(x,y)$, $y \frac{\partial \psi _{1}}{\partial x}$

The function

$$\begin{aligned} Q(x,y)=9xy+\sin (xy),\qquad Q(0,y)=Q(x,0)=0 \end{aligned}$$

fulfills

$$\begin{aligned} \frac{Q(x,y)}{y}=9x+\frac{\sin (xy)}{y}\geq 8=b. \end{aligned}$$

The derivatives of $Q(x,y)$ are defined as follows

$$\begin{aligned}& \frac{\partial Q(x,y)}{\partial x}=9y+y\cos (xy),\quad \text{so } \biggl\vert \frac{\partial Q(x,y)}{\partial x} \biggr\vert \leq 10=M,\\& \frac{\partial Q(x,y)}{\partial y}=9x+x\cos (xy),\quad \text{so } \biggl\vert \frac{\partial Q(x,y)}{\partial y} \biggr\vert \leq 10=N. \end{aligned}$$

For the behavior of the functions $\frac{\partial Q(x,y)}{\partial y}$, $\frac{\partial Q(x,y)}{\partial x}$, and $\frac{Q(x,y)}{y}$, see Fig. 3.

Figure 3

Trajectory of $\frac{\partial Q(x,y)}{\partial y}$, $\frac{\partial Q(x,y)}{\partial x}$ and $\frac{Q(x,y)}{y}$

Now, the function

$$\begin{aligned} f(x)=25 x +\frac{x}{1+x^{4}}, \quad \text{then } \frac{f(x)}{x}=25+ \frac{1}{1+x^{4}}\geq 25=d. \end{aligned}$$

It follows that

$$\begin{aligned} f'(x) =25+\frac{1-2x^{4}}{(1+x^{4})^{2}},\qquad \bigl\vert f'(x) \bigr\vert \leq 26=L. \end{aligned}$$

Therefore, we find

$$\begin{aligned} \sup{\bigl\{ f'(x)}\bigr\} =26 = \frac{c}{2}. \end{aligned}$$

Figure 4 gives the path of $\frac{f(x)}{x}$, $f'(x)$.

Figure 4

Path of $\frac{f(x)}{x}$, $f'(x)$

Finally, we obtain

$$\begin{aligned}& \alpha x\bigl(t-l(t)\bigr) =\frac{1}{4} \sin \biggl({x\biggl(t- \frac{1}{2}e^{-t}\biggr)}\biggr), \\& \text{so } \alpha = \frac{1}{4}\quad \text{and}\quad l(t)=\frac{1}{2} e^{-t },\quad \text{then } \bigl\vert l'(t) \bigr\vert =\frac{1}{2} e^{-t }\leq \frac{1}{2}. \end{aligned}$$

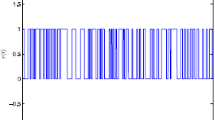
Figure 5 shows the behavior of the stochastic term $\frac{1}{4} \sin ({x(t-\frac{1}{2}e^{t})})$, and it also shows that $|l'(t)|<\frac{1}{2}$ on the interval $[0,30]$.

Figure 5

Trajectory of the stochastic term

Now, we have

$$\begin{aligned} \mu = \frac{ab+c}{4b}=6.38, \quad \text{then } ab-c =100>0, \end{aligned}$$

and

$$\begin{aligned} 2M+2b+6 =&42, \quad \text{so } ab-c> 2M+2b+6. \end{aligned}$$

Since $\alpha ^{2}= \frac{1}{16}$, we have

$$\begin{aligned} 2\alpha ^{2} =& \frac{1}{8}< 2H_{1}d-H_{1}(a+b+2)= 15.75, \end{aligned}$$

and

$$\begin{aligned} H_{1}(a-1) =&13.5>2\mu . \end{aligned}$$

Suppose that $\beta =\frac{1}{2}$, then we conclude

$$\begin{aligned} \begin{aligned} L U_{1}\leq & (10.42-24.67\gamma ) x^{2}-(14.5-585.89\gamma ) y^{2} -(0.49-125.06 \gamma )z^{2}+\frac{ E_{3}}{E_{1}} \eta _{1}(t) U_{1}. \end{aligned} \end{aligned}$$

Therefore, we get

$$\begin{aligned} \begin{aligned} \gamma \leq \min (0.42, 0.025, 0.004). \end{aligned} \end{aligned}$$

Hence all hypotheses of Theorem 2.1 are achieved, then the zero solution of (4.1) is USAS.

Example 4.2

Consider the following SDDE

$$\begin{aligned} \begin{aligned} & \biggl\{ \biggl(\frac{3}{4}+ \frac{1}{4}e^{-4x'} \biggr) x'' \biggr\} '+ \biggl\{ \biggl(19+\frac{\pi}{2}+\arctan \bigl(x x'\bigr) \biggr) \biggr\} ' \\ &\quad {}+9 x\bigl(t-r(t)\bigr) x'\bigl(t-r(t)\bigr) \\ &\quad {} +\sin{ \bigl(x\bigl(t-r(t)\bigr) x'\bigl(t-r(t)\bigr) \bigr)}+25x \bigl(t-r(t)\bigr) \\ &\quad {} +\frac{x(t-r(t))}{1+x(t-r(t))}+\frac{1}{4}\sin \biggl( x\biggl(t- \frac{1}{2}e^{t}\biggr)\biggr)= \varepsilon \bigl(x,x',x'' \bigr). \end{aligned} \end{aligned}$$

(4.5)

Using the estimates in Example 4.1, we get

$$\begin{aligned} \begin{aligned} &H_{1}=\frac{3}{4},\qquad H_{2}=1,\qquad a=19,\qquad a_{0}=19+ \frac{\pi}{2}, \\ & b=8,\qquad M=N= 10, \\ & c=52,\qquad d= 25,\qquad L=26,\qquad \mu =6.38\quad \text{and} \quad \alpha =\frac{1}{4}. \end{aligned} \end{aligned}$$

Since

$$\begin{aligned} \frac{H_{1}\{(ab-c+1)d-(a+b+2)\}}{a+1}=97.425, \end{aligned}$$

then we get

$$\begin{aligned} \alpha ^{2} < \frac{H_{1}\{(ab-c+1)d-(a+b+2)\}}{a+1}. \end{aligned}$$

Let $m=0.01$, so we obtain

$$\begin{aligned} \begin{aligned} L U \leq {}& -( 84.83-3313.56\gamma ) x^{2}-(508.5-27499.62 \gamma ) y^{2} \\ &{}-(1.49-1395.63\gamma )z^{2} +1.35 \vert x \vert +4.48 \vert y \vert +0.25 \vert z \vert , \end{aligned} \end{aligned}$$

provided that

$$\begin{aligned} \gamma < \min (0.025, 0.019, 0.0001). \end{aligned}$$

If we take $\zeta =0.2$ and $m=0.01$, then we find

$$\begin{aligned} \kappa =0.01 \max \{ 134.67, 447.92, 24.57\}\cong 4.48. \end{aligned}$$

Now, we can satisfy the condition (ii) of Theorem 2.2 in [28] by taking

$$\begin{aligned} \nu =&0.1 \quad \text{and}\quad \rho _{4}(t)=\biggl(\frac{3\zeta}{2}\biggr) \kappa ^{2}=6.04, \quad \text{with } n=2. \end{aligned}$$

Then, since $q_{1}=q_{2}=n=2$, we get all assumptions of Theorem 2.2 [28] are satisfied.

It follows from the above estimates, the following inequality holds

$$\begin{aligned} \int ^{t}_{t_{0}}\bigl\{ \rho _{3}\nu (u)+\rho _{4}(u)\bigr\} e^{\int ^{u}_{t_{0}} \nu (s)\,ds}\,du\leq 3\kappa ^{2}=60.2, \quad \text{for all } t\geq t_{0} \geq 0. \end{aligned}$$

And we also get

$$\begin{aligned} \bigl\vert U_{x_{i}}(t,x)G_{ik}(t,x) \bigr\vert \leq& \frac{1}{4} \bigl(239.13 x^{2} \bigl(t-l(t)\bigr)+67.17 x^{2}+ +168.77 y^{2}+13.33 z^{2} \bigr) \\ :=&\chi (t). \end{aligned}$$

Hence, Lemma 2.4 in [28] implies that the zero solution of (4.5) is USB.

Now, in view of Figs. 6 and 7, we find that the behavior for the solutions of (4.2) and (4.5) are asymptotically stable, such that the Figs. 6 and 7 illustrate the behavior of the solution, when $\alpha =0.25$ and $\alpha =1$, respectively. We note that, when α is increased, the stochasticity becomes more pronounced. On the other hand, if we take the function $\sin ( x(t-\frac{1}{2}e^{-t}))=1$, then we get Figs. 8 and 9, with $\alpha =0.25$ and $\alpha =1$, respectively.

Figure 6

The behavior of the solutions with $\alpha =0.25$

Figure 7

The behavior of the solutions with $\alpha =1$

Figure 8

The behavior of the solutions with $\sin ( x(t-\frac{1}{2}e^{-t}))=1$ and $\alpha =0.25$

Figure 9

The behavior of the solutions with $\sin ( x(t-\frac{1}{2}e^{-t}))=1$ and $\alpha =1$