Skip to main content
SpringerLink
Log in

Nonlinear damped transient response of sandwich auxetic plates with porous magneto-electro-elastic facesheets

  • Regular Article
  • Published:
The European Physical Journal Plus Aims and scope Submit manuscript

Abstract

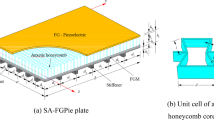
In this article, the effectiveness of the active controlled layer damping (ACLD) technique on attenuating the coupled nonlinear response of sandwich plates with auxetic core and porous functionally graded magneto-electro-elastic (PFGMEE) facings is numerically studied under the framework of finite element (FE) methods. The sandwich plate is subjected to multiphysics loading conditions, including mechanical, electrical and magnetic loads. A layerwise shear deformation theory using higher-order transverse deformation terms is used to arrive at the governing equations. This article aims to assess the integrated effects of porosity and auxetics on the nonlinear coupled response of sandwich structures. In addition, the detailed parametric study evaluates the influence of porosity distribution patterns, auxetic core's rib length ratio, inclination angle, ACLD patch positions, piezoelectric fibre (PE) fibre orientation angle, control gain and gradient index on the damped response of auxetic core/PFGMEE sandwich plates that have been presented. Emphasis has been made to understand the impact of applying open and closed circuits associated with the rest of the parameters on the controlled nonlinear behaviour of smart sandwich plates. The numerical results of this work make some major revelation about the implementation of auxetic cores in the smart structures, which make this work interesting.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13

Similar content being viewed by others

Data availability

The raw/processed data required to reproduce these findings cannot be shared at this time as the data also form part of an ongoing study.

References

  1. Y. Hou, Y.H. Tai, C. Lira, F. Scarpa, J.R. Yates, B. Gu, The bending and failure of sandwich structures with auxetic gradient cellular cores. Compos. A Appl. Sci. Manuf. 49, 119–131 (2013)

    Article  Google Scholar 

  2. X. Zhu, J. Zhang, W. Zhang, J. Chen, Vibration frequencies and energies of an auxetic honeycomb sandwich plate. Mech. Adv. Mater. Struct. 26(23), 1951–1957 (2019)

    Article  Google Scholar 

  3. T. Strek, H. Jopek, M. Nienartowicz, Dynamic response of sandwich panels with auxetic cores. Phys. Status Solidi (B). 252(7), 1540–50 (2015)

    Article  ADS  Google Scholar 

  4. N.D. Duc, C.H. Pham, Nonlinear dynamic response and vibration of sandwich composite plates with negative Poisson’s ratio in auxetic honeycombs. J. Sandwich Struct. Mater. 20(6), 692–717 (2018)

    Article  Google Scholar 

  5. N.D. Duc, K. Seung-Eock, P.H. Cong, N.T. Anh, N.D. Khoa, Dynamic response and vibration of composite double curved shallow shells with negative poisson’s ratio in auxetic honeycombs core layer on elastic foundations subjected to blast and damping loads. Int. J. Mech. Sci. 133, 504–512 (2017)

    Article  Google Scholar 

  6. P.H. Cong, N.D. Khanh, N.D. Khoa, N.D. Duc, New approach to investigate nonlinear dynamic response of sandwich auxetic double curves shallow shells using TSDT. Compos. Struct. 185, 455–465 (2018)

    Article  Google Scholar 

  7. N.D. Duc, T.Q. Quan, P.H. Cong, Nonlinear vibration of auxetic plates and shells (Vietnam National University Press, Hanoi, 2021)

    Google Scholar 

  8. P.H. Cong, P.T. Long, N.V. Nhat, N.D. Duc, Geometrically nonlinear dynamic response of eccentrically stiffened circular cylindrical shells with negative Poisson’s ratio in auxetic honeycombs core layer. Int. J. Mech. Sci. 152, 443–453 (2019)

    Article  Google Scholar 

  9. N.T. Duong, N.D. Duc, Evaluation of elastic properties and thermal expansion coefficient of composites reinforced by randomly distributed spherical particles with negative Poisson’s ratios. Compos. Struct. 153, 569–577 (2016)

    Article  Google Scholar 

  10. X.C. Zhang, L.Q. An, H.M. Ding et al., The influence of cell micro-structure on the inplane dynamic crushing of honeycombs with negative Poisson’s ratio. J. Sandwich Struct. Mater. 17(1), 26–55 (2015)

    Article  Google Scholar 

  11. T.T. Tran, Q.H. Pham, T. Nguyen-Thoi, Dynamic analysis of sandwich auxetic honeycomb plates subjected to moving oscillator load on elastic foundation. Adv. Mater. Sci. Eng. (2020). https://doi.org/10.1155/2020/6309130

    Article  Google Scholar 

  12. M. Vinyas, Computational analysis of smart magneto-electro-elastic materials and structures: review and classification. Arch. Comput. Methods Eng. 28(3), 1205–1248 (2021)

    Article  MathSciNet  Google Scholar 

  13. M. Vinyas, S.C. Kattimani, Static analysis of stepped functionally graded magneto-electro-elastic plates in thermal environment: a finite element study. Compos. Struct. 178, 63–86 (2017)

    Article  Google Scholar 

  14. M. Vinyas, S.C. Kattimani, Static behavior of thermally loaded multilayered magneto-electro-elastic beam. Struct. Eng. Mech 63(4), 481–495 (2017)

    Google Scholar 

  15. M. Vinyas, S.C. Kattimani, Static studies of stepped functionally graded magneto-electro-elastic beam subjected to different thermal loads. Compos. Struct. 163, 216–237 (2017)

    Article  Google Scholar 

  16. M. Vinyas, D. Harursampath, T.N. Thoi, A higher order coupled frequency characteristics study of smart magneto-electro-elastic composite plates with cutouts using finite element methods. Defence Technol 17(1), 100–118 (2021)

    Article  Google Scholar 

  17. M. Vinyas, D. Harursampath, Nonlinear vibrations of magneto-electro-elastic doubly curved shells reinforced with carbon nanotubes. Compos. Struct. 253, 112749 (2020)

    Article  Google Scholar 

  18. M. Vinyas, K.K. Sunny, D. Harursampath, T. Nguyen-Thoi, M.A.R. Loja, Influence of interphase on the multi-physics coupled frequency of three-phase smart magneto-electro-elastic composite plates. Compos. Struct. 226, 111254 (2019)

    Article  Google Scholar 

  19. M. Vinyas, S.C. Kattimani, Investigation of the effect of BaTiO3/CoFe2O4 particle arrangement on the static response of magneto-electro-thermo-elastic plates. Compos. Struct. 185, 51–64 (2018)

    Article  Google Scholar 

  20. Vinyas, M. and Kattimani, S.C., 2017. A finite element based assessment of static behavior of multiphase magneto-electro-elastic beams under different thermal loading. 62(5), pp. 519-535

  21. Vinyas M, Vishwas M, Harursampath D. Simulation-based assessment of coupled frequency response of magneto electro elastic auxetic multifunctional structures subjected to various electromagnetic circuits. Part L: Journal of Materials: Design and Applications doi: https://doi.org/10.1177/14644207211021933

  22. M. Vinyas, Nonlinear free vibration of multifunctional sandwich plates with auxetic core and magneto-electro-elastic facesheets of different micro-topological textures: FE approach". Mech. Adv. Mater. Struct. (2021). https://doi.org/10.1080/15376494.2021.1974619

    Article  Google Scholar 

  23. M. Vinyas, D.A. Harursampath, T. Nguyen-Thoi, Influence of active constrained layer damping on the coupled vibration response of functionally graded magneto-electro-elastic plates with skewed edges. Defence Technology 16(5), 1019–1038 (2020)

    Article  Google Scholar 

  24. S.C. Kattimani, M.C. Ray, Control of geometrically nonlinear vibrations of functionally graded magneto-electro-elastic plates. Int. J. Mech. Sci. 99, 154–167 (2015)

    Article  Google Scholar 

  25. D.J. Huang, H.J. Ding, W.Q. Chen, Analytical solution for functionally graded magneto-electro-elastic plane beams. Int. J. Eng. Sci. 45(2–8), 467–485 (2007)

    Article  Google Scholar 

  26. V. Mahesh, P.J. Sagar, S. Kattimani, Influence of coupled fields on free vibration and static behavior of functionally graded magneto-electro-thermo-elastic plate. J. Intell. Mater. Syst. Struct. 29(7), 1430–1455 (2018)

    Article  Google Scholar 

  27. J. Sladek, V. Sladek, S. Krahulec, C.S. Chen, D.L. Young, Analyses of circular magnetoelectroelastic plates with functionally graded material properties. Mech. Adv. Mater. Struct. 22, 479–489 (2015)

    Article  Google Scholar 

  28. W. Chang, X. Jin, Z. Huang, G. Cai, Random response of nonlinear system with inerter-based dynamic vibration absorber. J. Vibr. Eng. Technol. 9, 1–7 (2021). https://doi.org/10.1007/s42417-021-00334-6

    Article  Google Scholar 

  29. X. Wang, D. Wang, Three-dimensional vibration absorber platform for variable multiple frequency excitation and impulse response suppressing. J. Vibr. Eng. Technol. (2021). https://doi.org/10.1007/s42417-021-00320-y

    Article  Google Scholar 

  30. R.T. Faal, B. Crawford, R. Sourki et al., Experimental, numerical and analytical investigation of the torsional vibration suppression of a shaft with multiple optimal undamped absorbers. J. Vibr. Eng. Technol. (2021). https://doi.org/10.1007/s42417-021-00295-w

    Article  Google Scholar 

  31. A.R. Damanpack, M. Bodaghi, M.M. Aghdam, M. Shakeri, Active control of geometrically nonlinear transient response of sandwich beams with a flexible core using piezoelectric patches. Compos. Struct. 1(100), 517–531 (2013)

    Article  Google Scholar 

  32. J.X. Gao, Y.P. Shen, Active control of geometrically nonlinear transient vibration of composite plates with piezoelectric actuators. J. Sound Vib. 264(4), 911–928 (2003)

    Article  ADS  Google Scholar 

  33. A. Baz, S. Poh, Performance of an active control system with piezoelectric actuators. J. Sound Vib. 126, 327–343 (1988)

    Article  ADS  Google Scholar 

  34. S.K. Sarangi, M.C. Ray, Active damping of geometrically nonlinear vibrations of laminated composite plates using vertically reinforced 1–3 piezoelectric composites. Acta Mech. 222(3), 363–380 (2011)

    Article  Google Scholar 

  35. S.K. Sarangi, M.C. Ray, Smart damping of geometrically nonlinear vibrations of laminated composite beams using vertically reinforced 1–3 piezoelectric composites. Smart Mater. Struct. 19(7), 075020 (2010)

    Article  ADS  Google Scholar 

  36. J. Shivakumar, M.H. Ashok, M.C. Ray, Active control of geometrically nonlinear transient vibrations of laminated composite cylindrical panels using piezoelectric fiber reinforced composite. Acta Mech. 224(1), 1–5 (2013)

    Article  MathSciNet  Google Scholar 

  37. S. Panda, M.C. Ray, Active control of geometrically nonlinear vibrations of functionally graded laminated composite plates using piezoelectric fiber reinforced composites. J. Sound Vib. 325(1–2), 186–205 (2009)

    Article  ADS  Google Scholar 

  38. S.C. Kattimani, M.C. Ray, Smart damping of geometrically nonlinear vibrations of magneto-electro-elastic plates. Compos. Struct. 114, 51–63 (2014)

    Article  Google Scholar 

  39. M. Vinyas, D. Harursampath, T. Nguyen-Thoi, Influence of active constrained layer damping on the coupled vibration response of functionally graded magneto-electro-elastic plates with skewed edges. Defence Technol. 16(5), 1019–1038 (2020)

    Article  Google Scholar 

  40. M. Vinyas, Interphase effect on the controlled frequency response of three-phase smart magneto-electro-elastic plates embedded with active constrained layer damping: FE study. Mater. Res. Expr. 6(12), 125707 (2020)

    Article  Google Scholar 

  41. V. Mahesh, S. Kattimani, Finite element simulation of controlled frequency response of skew multiphase magneto-electro-elastic plates. J. Intell. Mater. Syst. Struct. 30(12), 1757–1771 (2019)

    Article  Google Scholar 

  42. M. Vinyas, Vibration control of skew magneto-electro-elastic plates using active constrained layer damping. Compos. Struct. 208, 600–617 (2019)

    Article  Google Scholar 

  43. M. Vinyas, Nonlinear damped transient vibrations of carbon nanotubes reinforced magneto-electro-elastic shells with different electromagnetic circuits. J. Vibr. Eng. Technol. (2021). https://doi.org/10.1007/s42417-021-00380-0

    Article  Google Scholar 

  44. M. Vinyas, Nonlinear pyrocoupled deflection of viscoelastic sandwich shell with CNT reinforced magneto-electro-elastic facing subjected to electromagnetic loads in thermal environment”. Eur. Phys. J. Plus 136, 796 (2021)

    Article  Google Scholar 

  45. Vinyas M. Effect of CNT reinforced magneto-electro-elastic facings on the pyrocoupled nonlinear deflection of viscoelastic sandwich skew plates in thermal environment”. Part L: Journal of Materials: Design and Applications

  46. A. Milazzo, Refined equivalent single layer formulations and finite elements for smart laminates free vibrations. Compos. Part B-Eng. 61, 238–253 (2014)

    Article  Google Scholar 

Download references

Acknowledgements

The financial support by the Royal Society, London, through the Newton International Fellowship (NIF\R1\212432) is sincerely acknowledged by the author Vinyas Mahesh

Author information

Authors and Affiliations

  1. Department of Mechanical Engineering and Aeronautics, City, University of London, London, EC1V 0HB, UK

    Vinyas Mahesh & Sathiskumar A. Ponnusami

Authors
  1. Vinyas Mahesh
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Sathiskumar A. Ponnusami
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Vinyas Mahesh.

Ethics declarations

Conflict of interest

None.

Appendix

Appendix

The strain displacement matrices can be expressed as follows:

$$ \left[ {B_{{{\text{tb}}}} } \right] = \left[ {\begin{array}{*{20}c} {{\partial \mathord{\left/ {\vphantom {\partial {\partial x}}} \right. \kern-\nulldelimiterspace} {\partial x}}} & 0 & 0 \\ 0 & {{\partial \mathord{\left/ {\vphantom {\partial {\partial y}}} \right. \kern-\nulldelimiterspace} {\partial y}}} & 0 \\ 0 & 0 & 0 \\ {{\partial \mathord{\left/ {\vphantom {\partial {\partial y}}} \right. \kern-\nulldelimiterspace} {\partial y}}} & {{\partial \mathord{\left/ {\vphantom {\partial {\partial x}}} \right. \kern-\nulldelimiterspace} {\partial x}}} & 0 \\ \end{array} } \right];\,\,\,\left[ {B_{{{\text{ts}}}} } \right] = \left[ {\begin{array}{*{20}c} 0 & 0 & {{\partial \mathord{\left/ {\vphantom {\partial {\partial x}}} \right. \kern-\nulldelimiterspace} {\partial x}}} \\ 0 & 0 & {{\partial \mathord{\left/ {\vphantom {\partial {\partial y}}} \right. \kern-\nulldelimiterspace} {\partial y}}} \\ \end{array} } \right] $$

\(\left[ {B_{{{\text{rb}}}} } \right] = \left[ {\begin{array}{*{20}c} {{\partial \mathord{\left/ {\vphantom {\partial {\partial x}}} \right. \kern-\nulldelimiterspace} {\partial x}}} & 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & {{\partial \mathord{\left/ {\vphantom {\partial {\partial y}}} \right. \kern-\nulldelimiterspace} {\partial y}}} & 0 & 0 & 0 & 0 & 0 & 0 \\ {{\partial \mathord{\left/ {\vphantom {\partial {\partial y}}} \right. \kern-\nulldelimiterspace} {\partial y}}} & {{\partial \mathord{\left/ {\vphantom {\partial {\partial x}}} \right. \kern-\nulldelimiterspace} {\partial x}}} & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 1 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & {{\partial \mathord{\left/ {\vphantom {\partial {\partial x}}} \right. \kern-\nulldelimiterspace} {\partial x}}} & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & {{\partial \mathord{\left/ {\vphantom {\partial {\partial y}}} \right. \kern-\nulldelimiterspace} {\partial y}}} & 0 & 0 \\ 0 & 0 & 0 & 0 & {{\partial \mathord{\left/ {\vphantom {\partial {\partial y}}} \right. \kern-\nulldelimiterspace} {\partial y}}} & {{\partial \mathord{\left/ {\vphantom {\partial {\partial x}}} \right. \kern-\nulldelimiterspace} {\partial x}}} & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & {{\partial \mathord{\left/ {\vphantom {\partial {\partial x}}} \right. \kern-\nulldelimiterspace} {\partial x}}} & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 0 & {{\partial \mathord{\left/ {\vphantom {\partial {\partial y}}} \right. \kern-\nulldelimiterspace} {\partial y}}} \\ 0 & 0 & 0 & 0 & 0 & 0 & {{\partial \mathord{\left/ {\vphantom {\partial {\partial y}}} \right. \kern-\nulldelimiterspace} {\partial y}}} & {{\partial \mathord{\left/ {\vphantom {\partial {\partial x}}} \right. \kern-\nulldelimiterspace} {\partial x}}} \\ \end{array} } \right],.....\left[ {B_{{{\text{rs}}}} } \right] = \left[ {\begin{array}{*{20}c} 1 & 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 1 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & 1 & 0 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 1 & 0 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 0 & 1 \\ 0 & 0 & {{\partial \mathord{\left/ {\vphantom {\partial {\partial x}}} \right. \kern-\nulldelimiterspace} {\partial x}}} & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & {{\partial \mathord{\left/ {\vphantom {\partial {\partial y}}} \right. \kern-\nulldelimiterspace} {\partial y}}} & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & {{\partial \mathord{\left/ {\vphantom {\partial {\partial x}}} \right. \kern-\nulldelimiterspace} {\partial x}}} & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & {{\partial \mathord{\left/ {\vphantom {\partial {\partial y}}} \right. \kern-\nulldelimiterspace} {\partial y}}} & 0 & 0 & 0 & 0 \\ \end{array} } \right]\),

The different transformation matrices [Z1] – [Z5] can be elaborated and expressed as follows:

$$ \begin{gathered} \left[ {Z_{1} } \right] = \left[ {\begin{array}{*{20}c} z & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & z & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & 0 & 1 & {2z} & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & z & 0 & 0 & 0 & 0 & 0 & 0 & 0 & 0 \\ \end{array} } \right] \hfill \\ \left[ {Z_{2} } \right] = \left[ {\begin{array}{*{20}c} {{h \mathord{\left/ {\vphantom {h 2}} \right. \kern-\nulldelimiterspace} 2}} & 0 & 0 & 0 & 0 & {h_{{\text{v}}} } & 0 & 0 & {\left( {z - h_{{\text{v}}} - {h \mathord{\left/ {\vphantom {h 2}} \right. \kern-\nulldelimiterspace} 2}} \right)} & 0 & 0 \\ 0 & {{h \mathord{\left/ {\vphantom {h 2}} \right. \kern-\nulldelimiterspace} 2}} & 0 & 0 & 0 & 0 & {h_{{\text{v}}} } & 0 & 0 & {\left( {z - h_{{\text{v}}} - {h \mathord{\left/ {\vphantom {h 2}} \right. \kern-\nulldelimiterspace} 2}} \right)} & 0 \\ 0 & 0 & 0 & 1 & {2z} & 0 & 0 & 0 & 0 & 0 & 0 \\ 0 & 0 & {{h \mathord{\left/ {\vphantom {h 2}} \right. \kern-\nulldelimiterspace} 2}} & 0 & 0 & 0 & 0 & {h_{{\text{v}}} } & 0 & 0 & {\left( {z - h_{{\text{v}}} - {h \mathord{\left/ {\vphantom {h 2}} \right. \kern-\nulldelimiterspace} 2}} \right)} \\ \end{array} } \right] \hfill \\ \left[ {Z_{3} } \right] = \left[ {\begin{array}{*{20}c} 0 & 0 & 0 & 0 & 0 & 0 & z & 0 & {z^{2} } & 0 \\ 0 & 0 & 0 & 0 & 0 & 0 & 0 & z & 0 & {z^{2} } \\ \end{array} } \right];\,\left[ {Z_{4} } \right] = \left[ {\begin{array}{*{20}c} 0 & 0 & 1 & 0 & 0 & 0 & z & 0 & {z^{2} } & 0 \\ 0 & 0 & 0 & 1 & 0 & 0 & 0 & z & 0 & {z^{2} } \\ \end{array} } \right] \hfill \\ \left[ {Z_{5} } \right] = \left[ {\begin{array}{*{20}c} 0 & 0 & 0 & 0 & 1 & 0 & z & 0 & {z^{2} } & 0 \\ 0 & 0 & 0 & 0 & 0 & 1 & 0 & z & 0 & z \\ \end{array} } \right] \hfill \\ \end{gathered} $$
(22)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Mahesh, V., Ponnusami, S.A. Nonlinear damped transient response of sandwich auxetic plates with porous magneto-electro-elastic facesheets. Eur. Phys. J. Plus 137, 563 (2022). https://doi.org/10.1140/epjp/s13360-022-02756-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1140/epjp/s13360-022-02756-x

Search

Navigation