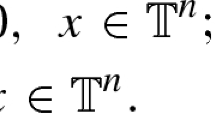Abstract
The hyperbolic Anosov C-systems have an exponential instability of their trajectories and as such represent the most natural chaotic dynamical systems. The C-systems defined on compact surfaces of the Lobachevsky plane of constant negative curvature are especially interesting. An example of such a system was introduced in a brilliant article published in 1924 by the mathematician Emil Artin. The dynamical system is defined on the fundamental region of the Lobachevsky plane, which is obtained by identifying points congruent with respect to the modular group, the discrete subgroup of the Lobachevsky plane isometries. The fundamental region in this case is a hyperbolic triangle. The geodesic trajectories of the non-Euclidean billiard are bounded to propagate on the fundamental hyperbolic triangle. Here, we present Artin’s results, calculate the correlation functions/observables defined on the phase space of the Artin billiard, and show that the correlation functions decay exponentially with time. We use the Artin symbolic dynamics, differential geometry, and the group theory methods of Gelfand and Fomin.
Similar content being viewed by others
References
E. Artin, “Ein mechanisches System mit quasiergodischen Bahnen,” Abh. Math. Sem. Univ. Hamburg, 3, 170–175 (1924).
J. Hadamard, “Les surfacesá courbures opposées et leur linges géodésiques,” J. Math. Pures Appl., 4, 27–73 (1898).
G. A. Hedlund, “The dynamics of geodesic flow,” Bull. Amer. Math. Soc., 45, 241–246 (1939).
D. V. Anosov, “Geodesic flows on closed Riemannian manifolds of negative curvature,” Proc. Steklov Inst. Math., 90, 1–235 (1967).
R. Bowen, Equilibrium States and the Ergodic Theory of Anosov Diffeomorphisms (Lect. Notes Math., Vol. 470), Springer, Berlin (1975).
A. N. Kolmogorov, “A new metric invariant of transitive dynamical systems and automorphisms of Lebesgue spaces,” Proc. Steklov Inst. Math., 169, 97–102 (1986).
A. N. Kolmogorov, “Entropy per unit time as a metric invariant of automorphisms,” Dokl. Akad. Nauk SSSR, 124, 754–755 (1959).
Ya. G. Sinai, “On the concept of entropy for a dynamic system [in Russian],” Dokl. Akad. Nauk SSSR, 124, 768–771 (1959).
D. Ruelle, Thermodynamic Formalism: The Mathematical Structures of Classical Equilibrium Statistical Mechanics, Addison-Wesley, Reading, Mass. (1978).
E. Hopf, “Ergodic theory and the geodesic flow on surfaces of constant negative curvature,” Bull. Amer. Math. Soc., 77, 863–877 (1971).
I. M. Gel’fand and S. V. Fomin, “Geodesic flows on manifolds of constant negative curvature,” Russ. Math. Surveys, 7, 118–137 (1952).
P. Collet, H. Epstein, and G. Gallavotti, “Perturbations of geodesic flows on surface of constant negative curvature and their mixing properties,” Commun. Math. Phys., 95, 61–112 (1984).
C. C. Moore, “Exponential decay of correlation coefficients for geodesic flows,” in: Group Representations, Ergodic Theory, Operator Algebras, and Mathematical Physics (Math. Sci. Res. Inst. Publ., Vol. 6, C. C. Moore, ed.), Springer, New York (1987), pp. 163–181.
N. S. Krylov, Works on the Foundation of Statistical Physics [in Russian], Acad. Sci. USSR Press, Moscow (1950); English transl., Princeton Univ. Press, Princeton, N. J. (1979).
E. Hopf, “Statistik der Lösungen geodätischer Probleme vom unstabilen Typus: II,” Math. Ann., 117, 590–608 (1940).
D. Dolgopyat, “On decay of correlations in Anosov flows,” Ann. of Math. (2), 147, 357–390 (1998).
N. I. Chernov, “Markov approximations and decay of correlations for Anosov flows,” Ann. Math. (2), 147, 269–324 (1998).
G. K. Savvidy, “The Yang–Mills classical mechanics as a Kolmogorov K-system,” Phys. Lett. B, 130, 303–307 (1983).
G. K. Savvidy, “Classical and quantum mechanics of non-abelian gauge fields,” Nucl. Phys. B, 246, 302–334 (1984).
G. K. Savvidy and N. G. Ter-Arutyunyan-Savvidy, “On the Monte Carlo simulation of physical systems,” J. Comput. Phys., 97, 566–572 (1991).
K. G. Savvidy, “The MIXMAX random number generator,” Comput. Phys. Commun., 196, 161–165 (2015).
G. K. Savvidy, “Anosov C-systems and random number generators,” Theor. Math. Phys., 188, 1155–1171 (2016).
K. G. Savvidy and G. K. Savvidy, “Spectrum and entropy of C-systems MIXMAX random number generator,” Chaos Solitons Fractals, 91, 33–38 (2016); arXiv:1510.06274v3 [math.DS] (2015).
J. Maldacena, S. H. Shenker, and D. Stanford, “A bound on chaos,” JHEP, 1608, 106 (2016); arXiv: 1503.01409v1 [hep-th] (2015).
H. Poincaré, “Théorie des groupes fuchsiens,” Acta Math., 1, 1–62 (1882).
L. Fuchs, “Über eine Klasse von Funktionen mehrerer Variablen, welche durch Umkehrung der Integrale von Lösungen der linearen Differentialgleichungen mit rationalen Coeffizienten entstehen,” J. Reine Angew. Math., 89, 151–169 (1880).
C. Burstin, “Über eine spezielle Klasse reeller periodischer Funktionen,” Monatsh. Math. Phys., 26, 229–262 (1915).
H. Minkowski, Geometrie der Zahlen, Teubner, Leipzig (1910).
H. Poincaré, “Mémoire sur les fonctions fuchsiennes,” Acta Math., 1, 193–294 (1882).
L. R. Ford, An Introduction to the Theory of Automorphic Functions, G. Bell and Sons, London (1915).
M. Pollicott, “On the rate of mixing of Axiom A flows,” Invent. Math., 81, 413–426 (1985).
G. K. Savvidy and K. G. Savvidy, “Exponential decay of correlations functions in MIXMAX generator of pseudorandom numbers,” Chaos Solitons Fractals, 107, 244–250 (2018).
Author information
Authors and Affiliations
Corresponding author
Additional information
This research was supported by the European Union’s Horizon 2020 research and innovation program (Marie Skíodowska-Curie grant agreement No. 644121).
Prepared from an English manuscript submitted by the authors; for the Russian version, see Teoreticheskaya i Matematicheskaya Fizika, Vol. 197, No. 2, pp. 230–251, November, 2018.
Rights and permissions
About this article
Cite this article
Poghosyan, H.R., Babujian, H.M. & Savvidy, G.K. Artin Billiard: Exponential Decay of Correlation Functions. Theor Math Phys 197, 1592–1610 (2018). https://doi.org/10.1134/S004057791811003X
Received:
Published:
Issue Date:
DOI: https://doi.org/10.1134/S004057791811003X