Skip to main content
SpringerLink
Log in

Adaptive Hierarchical Sliding Mode Control Design for 3D Ship-Mounted Container Crane with Saturating Actuators

  • Published:
Journal of Control, Automation and Electrical Systems Aims and scope Submit manuscript

Abstract

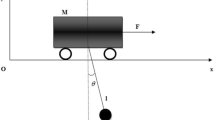
The study proposes a dynamic model and an adaptive controller for a 3D offshore container crane based on a hierarchical sliding mode control structure and artificial neural network. An overhead-type container crane mounted on a ship with actuator saturation is considered, and its dynamic model is derived from the Lagrange formulation. Hierarchical sliding mode control (HSMC) is utilized to construct the controller structure, while radial basis function (RBF) neural networks are integrated to estimate unknown dynamics and compensate for saturation nonlinearity. The control law is designed to ensure the stability of sliding surfaces, and an updated law for neural network’s weight matrices is constituted from a candidate of Lyapunov function. Simulations and experiments are provided to verify the effectiveness and robustness of the proposed control scheme in offshore conditions containing sea wave excitation and wind force.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25

Similar content being viewed by others

References

  • Almutairi, N. B., & Zribi, M. (2009). Sliding mode control of a three-dimensional overhead crane. Journal of vibration and control, 15(11), 1679–1730.

    Article  MathSciNet  MATH  Google Scholar 

  • Bartolini, G., Pisano, A., & Usai, E. (2002). Second-order sliding-mode control of container cranes. Automatica, 38, 1783–1790.

    Article  MathSciNet  MATH  Google Scholar 

  • Cho, S. K., & Lee, H. H. (2002). A fuzzy-logic antiswing controller for three-dimensional overhead cranes. ISA Transactions, 41, 235–243.

    Article  Google Scholar 

  • Craig, J. J. (2009). Introduction to robotics: Mechanics and control, 3/E. Pearson Education India.

  • Cuong, H. M., Dong, H. Q., Van Trieu, P., et al. (2021). Adaptive fractional-order terminal sliding mode control of rubber-tired gantry cranes with uncertainties and unknown disturbances. Mechanical Systems and Signal Processing, 154, 107601.

    Article  Google Scholar 

  • Cuong, H. M., Van Trieu, P., Nho, L. C., Thuan, V. D., et al. (2018). Adaptive neural network sliding mode control of shipboard container cranes considering actuator backlash. Mechanical Systems and Signal Processing, 112, 233–250.

    Article  Google Scholar 

  • Dasgupta, B., & Mruthyunjaya, T. S. (1998). Closed-form dynamic equations of the general Stewart platform through the Newton–Euler approach. Mechanism and Machine Theory, 33, 993–1012.

    Article  MathSciNet  MATH  Google Scholar 

  • Fang, Y., Zergeroglu, E., Dixon, W., & Dawson, D. (2001). Nonlinear coupling control laws for an overhead crane system. In Proceedings of the 2001 IEEE international conference on control applications (CCA’01) (Cat. No. 01CH37204) (pp. 639–644). IEEE.

  • Fujioka, D., & Singhose, W. (2015). Performance comparison of input-shaped model reference control on an uncertain flexible system. IFAC-PapersOnLine, 48(12), 129–134.

    Article  Google Scholar 

  • Gao, Wenzhi, & Selmic, R. R. (2006). Neural network control of a class of nonlinear systems with actuator saturation. IEEE Transactions on Neural Networks, 17(1), 147–156.

    Article  Google Scholar 

  • Hong, K. S., & Ngo, Q. H. (2012). Dynamics of the container crane on a mobile harbor. Ocean Engineering, 53, 16–24.

    Article  Google Scholar 

  • Hung, L. C., & Chung, H. Y. (2007). Decoupled control using neural network-based sliding-mode controller for nonlinear systems. Expert Systems with Applications, 32, 1168–1182.

    Article  Google Scholar 

  • Hung, L. C., & Chung, H. Y. (2007). Decoupled sliding-mode with fuzzy-neural network controller for nonlinear systems. International Journal of Approximate Reasoning, 46, 74–97.

    Article  MathSciNet  MATH  Google Scholar 

  • Jaafar, H. I., Mohamed, Z., Abidin, A. F. Z., & Ab Ghani, Z. (2012). Pso-tuned PID controller for a nonlinear gantry crane system. In 2012 IEEE international conference on control system, computing and engineering (pp. 515–519). IEEE.

  • Khatamianfar, A., & Savkin, A. V.: A new tracking control approach for 3d overhead crane systems using model predictive control. In 2014 European control conference (ECC) (pp. 796–801). IEEE.

  • Kim, D., & Park, Y. (2017). Tracking control in \(x-y\) plane of an offshore container crane, jvc/journal vib. control. In Journal of vibration and control (Vol. 23, pp. 469–483).

  • Kim, G. H., Pham, P. T., Ngo, Q. H., & Nguyen, Q. C. (2020). Neural network-based robust anti-sway control of an industrial crane subjected to hoisting dynamics and uncertain hydrodynamic forces. International Journal of Control, Automation and Systems, 19(5), 1–9.

    Google Scholar 

  • Lee, H. H. (1998). Modeling and control of a three-dimensional overhead crane. Journal of Dynamic Systems, Measurement, and Control, 120(4), 471–476.

  • Le, V. A., Le, H., Vu, D. T., Pham, V. T., Le, A. T., Hoang, M. C. (2018). Designing an adaptive controller for 3d overhead cranes using hierarchical sliding mode and neural network. In Proceeding of IEEE international conference on system science and engineering, New Taipei City, Taiwan.

  • Le, T. A., Kim, G. H., Kim, M. Y., & Lee, S. G. (2012). Partial feedback linearization control of overhead cranes with varying cable lengths. International Journal of Precision Engineering and Manufacturing, 13, 501–507.

    Article  Google Scholar 

  • Le, V. A., Le, H. X., Nguyen, L., & Phan, M. X. (2019). An efficient adaptive hierarchical sliding mode control strategy using neural networks for 3d overhead cranes. International Journal of Automation and Computing, 16(5), 614–627.

    Article  Google Scholar 

  • Lu, B., Fang, Y., Sun, N., & Wang, X. (2017). Antiswing control of offshore boom cranes with ship roll disturbances. IEEE Transactions on Control Systems Technology, 26(2), 740–747.

    Article  Google Scholar 

  • Majid, M. A., Ibrahim, W. S. W., Mohamad, S., & Bakar, Z. A. (2013). A comparison of PID and PD controller with input shaping technique for 3d gantry crane. In 2013 IEEE conference on systems, process & control (ICSPC) (pp. 144–148). IEEE.

  • Ngo, Q. H., & Hong, K. (2012). Sliding-mode antisway control of an offshore container crane. IEEE/ASME Transactions on Mechatronics, 17(2), 201–209.

    Article  Google Scholar 

  • Ngo, Q. H., Nguyen, N. P., Nguyen, C. N., Tran, T. H., & Bui, V. H. (2020). Payload pendulation and position control systems for an offshore container crane with adaptive-gain sliding mode control. Asian Journal of Control, 22(5), 2119–2128.

    Article  MathSciNet  Google Scholar 

  • Ngo, Q. H., Nguyen, N. P., Nguyen, C. N., Tran, T. H., & Ha, Q. P. (2017). Fuzzy sliding mode control of an offshore container crane. Ocean Engineering, 140, 125–134.

    Article  Google Scholar 

  • Park, M., Chwa, D., & Eom, M. (2014). Adaptive sliding-mode antisway control of uncertain overhead cranes with high-speed hoisting motion. IEEE Transactions on Fuzzy Systems, 22(5), 1262–1271.

    Article  Google Scholar 

  • Park, H., Chwa, D., & Hong, K. (2007). A feedback linearization control of container cranes: Varying rope length. International Journal of Control, Automation and Systems, 5, 379–387.

    Google Scholar 

  • Park, M., Chwa, D., & Hong, S. (2008). Antisway tracking control of overhead cranes with system uncertainty and actuator nonlinearity using an adaptive fuzzy sliding-mode control. IEEE Transactions on Industrial Electronics, 55(11), 3972–3984.

    Article  Google Scholar 

  • Park, J., & Sandberg, I. W. (1991). Universal approximation using radial-basis-function networks. Neural Computation, 3, 246–257.

    Article  Google Scholar 

  • Qian, D., Liu, X., & Yi, J. (2012). Adaptive control based on hierarchical sliding mode for under-actuated systems. In 2012 IEEE international conference on mechatronics and automation (pp. 1050–1055).

  • Qian, D., Yi, J., & Zhao, D. (2008). Hierarchical sliding mode control for a class of SIMO underactuated system. Control and Cybernetics, 37(1), 160–175.

    Google Scholar 

  • Qian, D., Yi, J., & Zhao, D. (2011). Control of overhead crane systems by combining sliding mode with fuzzy regulator. In IFAC Proceedings (Vol. 44, pp. 9320–9325).

  • Ramli, L., Mohamed, Z., Abdullahi, A. M., Jaafar, H., & Lazim, I. M. (2017). Control strategies for crane systems: A comprehensive review. Mechanical Systems and Signal Processing, 95, 1–23.

    Article  Google Scholar 

  • Shyu, K. K., Jen, C. L., & Shang, L. J. (2005). Design of sliding-mode controller for anti-swing control of overhead cranes. In 31st annual conference of IEEE industrial electronics society (pp. 147–152). IECON.

  • Solihin, M. I., & Wahyudi, & Legowo, A. (2010). Fuzzy-tuned PID anti-swing control of automatic gantry crane. Journal of Vibration and Control, 16(1), 127–145.

  • Sun, N., Fang, Y., Chen, H., & He, B. (2014). Adaptive nonlinear crane control with load hoisting/lowering and unknown parameters: Design and experiments. IEEE/ASME Transactions on Mechatronics, 20(5), 2107–2119.

    Article  Google Scholar 

  • Sun, N., Fang, Y., Chen, H., Wu, Y., & Lu, B. (2017). Nonlinear antiswing control of offshore cranes with unknown parameters and persistent ship-induced perturbations: Theoretical design and hardware experiments. IEEE Transactions on Industrial Electronics, 65(3), 2629–2641.

    Article  Google Scholar 

  • Thi, K. D. H., Le, V. A., Pham, T. D., Vo, H. T., & Pham, Q.T. (2019). Modeling and integral hierarchical sliding-mode control for 2d ship-mounted crane. In First international symposium on instrumentation, control, artificial intelligence, and robotics (ICA-SYMP) (pp. 82–85). IEEE.

  • Tsai, C., Wu, H.L., Chuang, K. (2012). Intelligent sliding-mode motion control using fuzzy wavelet networks for automatic 3d overhead cranes. In 2012 proceedings of SICE annual conference (SICE) (pp. 1256–1261).

  • Tuan, L. A., Ha, Q., Van Trieu, P. (2019). Observer-based nonlinear robust control of floating container cranes subject to output hysteresis. Journal of Dynamic Systems, Measurement, and Control, 141(11).

  • Tuan, L. A. (2019). Fractional-order fast terminal back-stepping sliding mode control of crawler cranes. Mechanism and Machine Theory, 137, 297–314.

    Article  Google Scholar 

  • Tuan, L. A., Cuong, H. M., Lee, S. G., Nho, L. C., & Moon, K. (2016). Nonlinear feedback control of container crane mounted on elastic foundation with the flexibility of suspended cable. Journal of Vibration and Control, 22, 3067–3078.

    Article  MathSciNet  Google Scholar 

  • Tuan, L. A., Kim, J. J., Lee, S. G., Lim, T. G., & Nho, L. C. (2014). Second-order sliding mode control of a 3d overhead crane with uncertain system parameters. International Journal of Precision Engineering and Manufacturing, 15, 811–819.

    Article  Google Scholar 

  • Tuan, L. A., Lee, S. G., Dang, V. H., Moon, S., & Kim, B. (2013). Partial feedback linearization control of a three-dimensional overhead crane. International Journal of Control, Automation and Systems, 11, 718–727.

    Article  Google Scholar 

  • Tuan, L. A., Lee, S. G., Nho, L. C., & Cuong, H. M. (2015). Robust controls for ship-mounted container cranes with viscoelastic foundation and flexible hoisting cable. Proceedings of the Institution of Mechanical Engineers, Part I: Journal of Systems and Control Engineering, 229, 662–674.

    Google Scholar 

  • Wang, L., Zhang, H., & Kong, Z.(2015). Anti-swing control of overhead crane based on double fuzzy controllers. In The 27th Chinese control and decision conference (2015 CCDC) (pp. 981–986).

  • Wang, W., Liu, X. D., & Yi, J. Q. (2007). Structure design of two types of sliding-mode controllers for a class of under-actuated mechanical systems. IET Control Theory & Applications, 1, 163–172.

    Article  MathSciNet  Google Scholar 

  • Wang, W., Yi, J., Zhao, D., & Liu, D. (2004). Design of a stable sliding-mode controller for a class of second-order underactuated systems. IEE Proceedings—Control Theory and Applications, 151, 683–690.

    Article  Google Scholar 

  • Wu, Z., Xia, X., & Zhu, B. (2015). Model predictive control for improving operational efficiency of overhead cranes. Nonlinear Dynamics, 79(4), 2639–2657.

    Article  Google Scholar 

  • Xu, W., Zheng, X., Liu, Y., Zhang, M., & Luo, Y. (2015). Adaptive dynamic sliding mode control for overhead cranes. In Proc. 34th Chinese control conf. (pp. 3287–3292).

  • Yang, B., & Xiong, B. (2010). Application of LQR techniques to the anti-sway controller of overhead crane. In Advanced materials research (Vol. 139, pp. 1933–1936). Trans Tech Publications Ltd.

  • Yang, J. H., & Yang, K. S. (2007). Adaptive coupling control for overhead crane systems. Mechatronics, 17(2–3), 143–152.

    Article  Google Scholar 

Download references

Acknowledgements

This research is funded by International School, Vietnam National University, Hanoi, Vietnam. We would like to thank Viet-Anh Le (Department of Mechanical Engineering, University of Delaware, USA) and Dr. Trieu Van Pham (Vietnam Maritime University) for assisting this study.

Author information

Authors and Affiliations

  1. International School, Vietnam National University, Hanoi, 100000, Vietnam

    Thai Dinh Kim, Linh Ngoc Nguyen & Hai Xuan Le

  2. Faculty of Electrical Engineering, Hanoi University of Industry, Hanoi, 100000, Vietnam

    Xuan Minh Dinh

Authors
  1. Thai Dinh Kim
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Linh Ngoc Nguyen
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Xuan Minh Dinh
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Hai Xuan Le
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Thai Dinh Kim.

Ethics declarations

Conflict of interest

The author declares that there are no conflicts of interest regarding the publication of this paper.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

6 Appendices

6 Appendices

1.1 6.1 Appendix A

The components of the \({\mathbf{M}}({\mathbf{q}})\) are given as follows:

$$\begin{aligned}&{{m}_{11}}={{m}_{1}}+{{m}_{2}}+{{m}_{c}};\\&{{m}_{12}}={{m}_{21}}=0;\\&{{m}_{13}}={{m}_{31}}={{m}_{c}}\left( c\beta s\alpha c\theta +s\beta s\varphi s\theta +c\alpha c\beta c\varphi s\theta \right) ;\\&{{m}_{14}}={{m}_{41}}={{m}_{c}}lc\beta \left( c\alpha c\theta -c\varphi s\alpha s\theta \right) ;\\&{{m}_{15}}={{m}_{51}}=-{{m}_{c}}l\left( s\alpha s\beta c\theta -c\beta s\varphi s\theta +c\alpha c\varphi s\beta s\theta \right) ;\\&{{m}_{22}}={{m}_{2}}+{{m}_{c}};\\&{{m}_{23}}={{m}_{32}}={{m}_{c}}\left( c\varphi s\beta -c\alpha c\beta s\varphi \right) ; \\&{{m}_{24}}={{m}_{42}}={{m}_{c}}lc\beta s\alpha s\varphi ;\\&{{m}_{25}}={{m}_{52}}={{m}_{c}}l\left( c\beta c\varphi +c\alpha s\beta s\varphi \right) ; \\&{{m}_{33}}={{m}_{c}};{{m}_{34}}={{m}_{43}}=0; {{m}_{35}}={{m}_{53}}=0; \\&{{m}_{44}}={{m}_{c}}{{l}^{2}}co{{s}^{2}}\beta ;\\&{{m}_{45}}={{m}_{54}}=0; \\&{{m}_{55}}={{m}_{c}}{{l}^{2}} \end{aligned}$$

The elements on the \(\mathbf {C}(\mathbf {q},\dot{\mathbf {q}})\) matrix are expressed as:

$$\begin{aligned}&{{c}_{11}}=0;\quad {{c}_{12}}=-\dot{\varphi }({{m}_{2}}+{{m}_{c}})s\theta ; \\&{c_{13}} = - {m_c}\left( \begin{array}{l} \dot{\beta }\left( {s\alpha s\beta c\theta - c\beta s\varphi s\theta + c\alpha c\varphi s\beta s\theta } \right) + \\ \dot{\alpha }\left( {c\beta c\varphi s\alpha s\theta - c\alpha c\beta c\theta } \right) \end{array} \right) ;\\&{c_{14}} = - {m_c}\left( \begin{array}{l} \dot{l}c\beta \left( {c\varphi s\alpha s\theta - c\alpha c\theta } \right) + \\ \dot{\alpha }lc\beta \left( {s\alpha c\theta + c\alpha c\varphi s\theta } \right) + \\ \dot{\beta }ls\beta \left( {c\alpha c\theta - c\varphi s\alpha s\theta } \right) \end{array} \right) ;\\&{c_{15}} = - {m_c}\left( \begin{array}{l} \dot{l}\left( {s\alpha s\beta c\theta - c\beta s\varphi s\theta + c\alpha c\varphi s\beta s\theta } \right) + \\ \dot{\alpha }ls\beta \left( {c\alpha c\theta - c\varphi s\alpha s\theta } \right) + \\ \dot{\beta }l\left( {c\beta s\alpha c\theta + s\beta s\varphi s\theta + c\alpha c\beta c\varphi s\theta } \right) \end{array} \right) ;\\&{{c}_{21}}=\dot{\varphi }({{m}_{2}}+{{m}_{c}})s\theta ; {{c}_{22}}=0;\\&{{c}_{23}}={{m}_{c}}(\dot{\beta }\left( c\beta c\varphi +c\alpha s\beta s\beta \right) +\dot{\alpha }c\beta s\alpha s\varphi ); \\&{{c}_{24}}={{m}_{c}}s\varphi \left( \dot{l}c\beta s\alpha +\dot{\alpha }lc\alpha c\beta -\dot{\beta }ls\alpha s\beta \right) ;\\&{c_{25}} = {m_c}\left( \begin{array}{l} \dot{l}\left( {c\beta c\varphi + c\alpha s\beta s\varphi } \right) + \dot{\beta }l\left( {c\alpha c\beta s\varphi - c\varphi s\beta } \right) + \\ \dot{\alpha }ls\alpha s\beta s\varphi \end{array} \right) ;\\&{c_{31}} = {m_c}\left( \begin{array}{l} \dot{\varphi }\left( {c\varphi s\beta s\theta - c\alpha c\beta s\varphi s\theta } \right) + \\ \dot{\theta }\left( {s\beta c\theta s\varphi + c\alpha c\beta c\varphi c\theta - c\beta s\alpha s\theta } \right) \end{array} \right) ;\\&{{c}_{32}}=-\dot{\varphi }{{m}_{c}}(s\beta s\varphi +c\alpha c\beta c\varphi ); {{c}_{33}}=0;\\&{{c}_{34}}=-\dot{\alpha }{{m}_{c}}l{{c}^{2}}\beta ; {{c}_{35}}=-\dot{\beta }{{m}_{c}}l;\\&{{c}_{41}}=-{{m}_{c}}lc\beta \left( \dot{\theta }\left( c\alpha s\theta +c\varphi s\alpha c\theta \right) -\dot{\varphi }s\alpha s\varphi s\theta \right) ;\\&{{c}_{42}}=\dot{\varphi }{{m}_{c}}lc\beta c\varphi s\alpha ; {{c}_{43}}=\dot{\alpha }{{m}_{c}}l{{c}^{2}}\beta ;\\&{{c}_{44}}={{m}_{c}}lc\beta \left( \dot{l}c\beta -\dot{\beta }ls\beta \right) ;{{c}_{45}}=-\dot{\alpha }{{m}_{c}}{{l}^{2}}s\beta c\beta ; \\&{c_{51}} = {m_c}l\left( \begin{array}{l} \dot{\varphi }\left( {c\beta c\varphi s\theta + c\alpha s\beta s\varphi s\theta } \right) + \\ \dot{\theta }\left( {c\beta c\theta s\varphi + s\alpha s\beta s\theta - c\alpha c\varphi s\beta c\theta } \right) \end{array} \right) ;\\&{{c}_{52}}=-\dot{\varphi }{{m}_{c}}l(c\beta s\varphi -c\alpha c\varphi s\beta ); \quad {{c}_{53}}=\dot{\beta }{{m}_{c}}l; \\&{{m}_{54}}=\dot{\alpha }{{m}_{c}}{{l}^{2}}s\beta c\beta ; \quad {{c}_{55}}=\dot{l}{{m}_{c}}l. \end{aligned}$$

The components of the gravitational force vector \(\mathbf {G}(\mathbf {q})\) are presented as:

$$\begin{aligned}&{{g}_{1}}=-gc\varphi s\theta ({{m}_{1}}+{{m}_{2}}+{{m}_{c}});\\&{{g}_{2}}=gs\varphi ({{m}_{2}}+{{m}_{c}}); \\&{{g}_{3}}=-g{{m}_{c}}c\alpha c\beta ; \\&{{g}_{4}}=g{{m}_{c}}lc\beta s\alpha ; {{g}_{5}}=g{{m}_{c}}lc\alpha s\beta . \end{aligned}$$

The elements of the vector of wave-wind disturbance \(\mathbf {W}\) is determined as follows:

$$\begin{aligned}&{{w}_{1}}=-{{m}_{16}}\ddot{\varphi }-{{m}_{17}}\ddot{\theta }-{{m}_{18}}\ddot{z}-{{c}_{16}}\dot{\varphi }-{{c}_{17}}\dot{\theta }-{{c}_{18}}\dot{z};\\&{{w}_{2}}=-{{m}_{26}}\ddot{\varphi }-{{m}_{27}}\ddot{\theta }-{{m}_{28}}\ddot{z}-{{c}_{26}}\dot{\varphi }-{{c}_{27}}\dot{\theta }-{{c}_{28}}\dot{z};\\&{{w}_{3}}=-{{m}_{36}}\ddot{\varphi }-{{m}_{37}}\ddot{\theta }-{{m}_{38}}\ddot{z}-{{c}_{36}}\dot{\varphi }-{{c}_{37}}\dot{\theta }-{{c}_{38}}\dot{z};\\&{{w}_{4}}={{f}_{wx}}-{{m}_{46}}\ddot{\varphi }-{{m}_{47}}\ddot{\theta }-{{m}_{48}}\ddot{z}-{{c}_{46}}\dot{\varphi }-{{c}_{47}}\dot{\theta }-{{c}_{48}}\dot{z};\\&{{w}_{5}}={{f}_{wy}}-{{m}_{56}}\ddot{\varphi }-{{m}_{57}}\ddot{\theta }-{{m}_{58}}\ddot{z}-{{c}_{56}}\dot{\varphi }-{{c}_{57}}\dot{\theta }-{{c}_{58}}\dot{z}. \end{aligned}$$

where

$$\begin{aligned}&{{m}_{16}}=-{{s}_{y}}s\theta ({{m}_{2}}+{{m}_{c}});\\&{{m}_{17}}=h({{m}_{1}}+{{m}_{2}}+{{m}_{c}});\\&{{m}_{18}}=-c\varphi s\theta ({{m}_{1}}+{{m}_{2}}+{{m}_{c}});\\&{{m}_{26}}=-\left( {{m}_{2}}+{{m}_{c}} \right) \left( hc\theta -{{s}_{x}}s\theta \right) ; \\&{{m}_{27}}=0; {{m}_{28}}=s\varphi ({{m}_{2}}+{{m}_{c}}); \\&{m_{36}} = - {m_c}\left( \begin{array}{l} {s_y}\left( {s\beta s\varphi + c\alpha c\beta c\varphi } \right) + \\ h\left( {c\varphi s\beta c\theta - c\alpha c\beta c\theta s\varphi } \right) + \\ {s_x}s\theta \left( {c\alpha c\beta s\varphi - c\varphi s\beta } \right) \end{array} \right) ;\\&{m_{37}} = {m_c}\left( \begin{array}{l} h\left( {c\beta s\alpha c\theta + s\beta s\varphi s\theta + c\alpha c\beta c\varphi s\theta } \right) + \\ {s_x}\left( {s\beta c\theta s\varphi + c\alpha c\beta c\varphi c\theta - c\beta s\alpha s\theta } \right) \end{array} \right) ;\\&{{m}_{38}}=-{{m}_{c}}\cos \alpha \cos \beta ;\\&{{m}_{46}}={{m}_{c}}lc\beta s\alpha ({{s}_{y}}c\varphi -hc\theta s\varphi +{{s}_{x}}s\varphi s\theta ); \\&{{m}_{47}}\!=\!-{{m}_{c}}lc\beta \left( {{s}_{x}}\left( c\alpha s\theta \!+\! c\varphi s\alpha c\theta \right) \!+\!h\left( c\varphi s\alpha s\theta \!-\! c\alpha c\theta \right) \right) ;\\&{{m}_{48}}={{m}_{c}}l\cos \beta \sin \alpha ; \\&{m_{56}} = - {m_c}l\left( \begin{array}{l} {s_y}\left( {c\beta s\varphi - c\alpha c\varphi s\beta } \right) +\\ h\left( {c\beta c\varphi c\theta + c\alpha s\beta c\theta s\varphi } \right) - \\ {s_x}\left( {c\beta c\varphi s\theta + c\alpha s\beta s\varphi s\theta } \right) \end{array} \right) ; \\&{m_{57}} = - {m_c}l\left( \begin{array}{l} h\left( {s\alpha s\beta c\theta - c\beta s\varphi s\theta + c\alpha c\varphi s\beta s\theta } \right) + \\ {s_x}\left( {c\alpha c\varphi s\beta c\theta - c\beta c\theta s\varphi - s\alpha s\beta s\theta } \right) \end{array} \right) ; \\&{{m}_{58}}={{m}_{c}}lc\alpha s\beta ;\\&{{c}_{16}}\!=\!\dot{\varphi }s\theta ({{m}_{1}}\!+\!{{m}_{2}}\!+\!{{m}_{c}})\left( hc\theta \!+\!{{s}_{x}}s\theta \right) \!-\!{{\dot{s}}_{y}}({{m}_{2}}\!+\!{{m}_{c}})s\theta ;\\&{{c}_{17}}=-\dot{\theta }{{s}_{x}}({{m}_{1}}+{{m}_{2}}+{{m}_{c}});\\&{{c}_{26}}=({{m}_{2}}+{{m}_{c}})\left( {{{\dot{s}}}_{x}}s\theta -\dot{\varphi }{{s}_{y}}+\dot{\theta }\left( hs\theta +{{s}_{x}}c\theta \right) \right) ;\\&{{c}_{27}}=\dot{\varphi }\left( {{m}_{2}}+{{m}_{c}} \right) \left( hs\theta +{{s}_{x}}c\theta \right) ; \\&{c_{36}} = - {m_c}\left( \begin{array}{l} {{\dot{s}}_y}\left( {s\beta s\varphi + c\alpha c\beta c\varphi } \right) + \\ {{\dot{s}}_x}s\theta \left( {c\alpha c\beta s\varphi - c\varphi s\beta } \right) + \\ \dot{\varphi }\left( \begin{array}{l} {s_y}\left( {c\varphi s\beta - c\alpha c\beta s\varphi } \right) + \\ {s_x}s\theta \left( {s\beta s\varphi + c\alpha c\beta c\varphi } \right) - \\ hc\theta \left( {c\alpha c\beta c\varphi + s\beta s\varphi } \right) \end{array} \right) + \\ \dot{\theta }\left( \begin{array}{l} hs\theta \left( {c\alpha c\beta s\varphi - c\varphi s\beta } \right) + \\ {s_x}c\theta \left( {c\alpha c\beta s\varphi - c\varphi s\beta } \right) \end{array} \right) \end{array} \right) ;\\&{c_{37}} = - {m_c}\left( \begin{array}{l} {{\dot{s}}_x}\left( {c\beta s\alpha s\theta - s\beta c\theta s\varphi - c\alpha c\beta c\varphi c\theta } \right) + \\ \dot{\varphi }\left( \begin{array}{l} hs\theta \left( {c\alpha c\beta s\varphi - c\varphi s\beta } \right) + \\ {s_x}c\theta \left( {c\alpha c\beta s\varphi - c\varphi s\beta } \right) \end{array} \right) \\ + \dot{\theta }\left( \begin{array}{l} h\left( \begin{array}{l} c\beta s\alpha s\theta - s\beta c\theta s\varphi - \\ c\alpha c\beta c\varphi c\theta \end{array} \right) + \\ {s_x}\left( \begin{array}{l} c\beta s\alpha c\theta + s\beta s\varphi s\theta + \\ c\alpha c\beta c\varphi s\theta \end{array} \right) \end{array} \right) \end{array} \right) ; \\&{c_{46}} = {m_c}lc\beta s\alpha \left( \begin{array}{l} {{\dot{s}}_y}c\varphi + {{\dot{s}}_x}s\varphi s\theta + \\ \dot{\varphi }\left( {{s_x}c\varphi s\theta - {s_y}s\varphi - hc\varphi c\theta } \right) + \\ \dot{\theta }s\varphi \left( {hs\theta + {s_x}c\theta } \right) \end{array} \right) ;\\&{c_{47}} = - {m_c}lc\beta \left( \begin{array}{l} {{\dot{s}}_x}\left( {c\alpha s\theta + c\varphi s\alpha c\theta } \right) - \\ \dot{\varphi }s\alpha s\varphi \left( {hs\theta - {s_x}c\theta } \right) \\ + \dot{\theta }\left( \begin{array}{l} h\left( {c\alpha s\theta + c\varphi s\alpha c\theta } \right) + \\ {s_x}\left( {c\alpha c\theta - c\varphi s\alpha s\theta } \right) \end{array} \right) \end{array} \right) ; \\&{c_{56}} = {m_c}l\left( \begin{array}{l} {{\dot{s}}_y}\left( {c\alpha c\varphi s\beta - c\beta s\varphi } \right) + \\ {{\dot{s}}_x}s\theta \left( {c\beta c\varphi + c\alpha s\beta s\varphi } \right) + \\ \dot{\varphi }\left( \begin{array}{l} - {s_y}\left( {c\beta c\varphi + c\alpha s\beta s\varphi } \right) + \\ hc\theta \left( {c\beta s\varphi - c\alpha c\varphi s\beta } \right) + \\ {s_x}s\theta \left( {c\alpha c\varphi s\beta - c\beta s\varphi } \right) \end{array} \right) \\ \dot{\theta }\left( \begin{array}{l} hs\theta \left( {c\beta c\varphi + c\alpha s\beta s\varphi } \right) + \\ {s_x}c\theta \left( {c\beta c\varphi + c\alpha s\beta s\varphi } \right) \end{array} \right) \end{array} \right) ;\\&{c_{57}} = {m_c}l\left( \begin{array}{l} {{\dot{s}}_x}\left( {c\beta c\theta s\varphi + s\alpha s\beta s\theta - c\alpha c\varphi s\beta c\theta } \right) \\ + \dot{\varphi }\left( \begin{array}{l} hs\theta \left( {c\beta c\varphi + c\alpha s\beta s\varphi } \right) + \\ {s_x}c\theta \left( {c\beta c\varphi + c\alpha s\beta s\varphi } \right) \end{array} \right) + \\ \dot{\theta }\left( \begin{array}{l} h\left( \begin{array}{l} c\beta c\theta s\varphi + s\alpha s\beta s\theta - \\ c\alpha c\varphi s\beta c\theta \end{array} \right) + \\ {s_x}\left( \begin{array}{l} s\alpha s\beta c\theta - c\beta s\varphi s\theta + \\ c\alpha c\varphi s\beta s\theta \end{array} \right) \end{array} \right) \end{array} \right) ;\\&{c_{18}} = {c_{28}} = {c_{38}} = {c_{48}} = {c_{58}} = 0; \end{aligned}$$

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Kim, T.D., Nguyen, L. ., Dinh, X. . et al. Adaptive Hierarchical Sliding Mode Control Design for 3D Ship-Mounted Container Crane with Saturating Actuators. J Control Autom Electr Syst 33, 1643–1658 (2022). https://doi.org/10.1007/s40313-022-00939-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40313-022-00939-6

Keywords

Search

Navigation