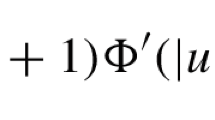Abstract
This paper is devoted to the study of static bifurcation theory for a class of degenerate boundary value problems for semilinear elliptic differential operators of second-order which includes as particular cases the Dirichlet and Robin problems. The purpose of this paper is to generalize some results of Szulkin (Nonlinear Anal TMA 6:95–116, 1982) to the degenerate case. We study the behavior of nontrivial solution branches for bounded nonlinear terms, and prove that the branches turn back towards the first eigenvalue of the linearized degenerate boundary value problem. Our proof is based on global static bifurcation theory for positive mappings in ordered Banach spaces, with the special emphasis on the Semenov approximation in Chemistry. The approach here is distinguished by the extensive use of the ideas and techniques characteristic of the recent developments in the \(L^{p}\) theory of pseudo-differential operators.





















Similar content being viewed by others
References
Adams, R.A., Fournier, J.J.F.: Sobolev Spaces. Pure and Applied Mathematics, vol. 140, 2nd edn. Elsevier/Academic Press, Amsterdam (2003)
Agmon, S.: Lectures on Elliptic Boundary Value Problems. Van Nostrand, Princeton (1965)
Agmon, S., Douglis, A., Nirenberg, L.: Estimates near the boundary for solutions of elliptic partial differential equations satisfying general boundary conditions I. Commun. Pure Appl. Math. 12, 623–727 (1959)
Amann, H.: On the number of solutions of nonlinear equations in ordered Banach spaces. J. Funct. Anal. 11, 346–384 (1972)
Amann, H.: Fixed point equations and nonlinear eigenvalue problems in ordered Banach spaces. SIAM Rev. 18, 620–709 (1976)
Amann, H.: Nonlinear eigenvalue problems having precisely two solutions. Math. Z. 150, 27–37 (1976)
Amann, H., Ambrosetti, A., Mancini, G.: Elliptic equations with noninvertible Fredholm linear part and bounded nonlinearities. Math. Z. 158, 179–194 (1978)
Ambrosetti, A., Malchiodi, A.: Nonlinear Analysis and Semilinear Elliptic Problems. Cambridge Studies in Advanced Mathematics, vol. 104. Cambridge University Press, Cambridge (2007)
Ambrosetti, A., Mancini, G.: Existence and multiplicity results for nonlinear elliptic problems with linear part at resonance. The case of the simple eigenvalue. J. Differ. Equ. 28, 220–245 (1978)
Ambrosetti, A., Mancini, G.: Theorems of existence and multiplicity for nonlinear elliptic problems with noninvertible linear part. Ann. Sc. Norm. Sup. Pisa Cl. Sci. (4) 5, 15–28 (1978)
Ambrosetti, A., Mancini, G.: Sharp nonuniqueness results for some nonlinear problems. Nonlinear Anal. TMA 3, 635–645 (1979)
Ambrosetti, A., Prodi, G.: On the inversion of some differentiable mappings with singularities between Banach spaces. Ann. Mat. Pura Appl. 93, 231–247 (1972)
Ambrosetti, A., Prodi, G.: A Primer of Nonlinear Analysis, Paperback Edition (with Corrections). Cambridge Studies in Advanced Mathematics, vol. 34. Cambridge University Press, Cambridge (1993)
Anh, C.T., My, B.K.: Existence and non-existence of solutions to a Hamiltonian strongly degenerate elliptic system. Adv. Nonlinear Anal. 8, 661–678 (2019)
Aronszajn, N., Krzywicki, A., Szarski, J.: A unique continuation theorem for exterior differential forms on Riemannian manifolds. Ark. Mat. 4, 417–453 (1962)
Berestycki, H.: Remarques sur le nombre de solutions de certains problèmes semi-linéaires elliptiques. C. R. Acad. Sci. Paris Sér. A-B 289, A633–A636 (1979)
Berger, M.S.: Nonlinearity and Functional Analysis. Academic Press, New York (1977)
Berger, M.S.: Nonlinear problems with exactly three solutions. Indiana Univ. Math. J. 28, 689–698 (1979)
Bergh, L., Löfström, J.: Interpolation Spaces, an Introduction. Springer, Berlin (1976)
Bony, J.-M.: Principe du maximum dans les espaces de Sobolev. C. R. Acad. Sc. Paris Sér. A-B 265, A333–A336 (1967)
Bourdaud, G.: \(L^{p}\)-estimates for certain non-regular pseudo-differential operators. Commun. Partial Differ. Equ. 7, 1023–1033 (1982)
Brown, R.F.: A Topological Introduction to Nonlinear Analysis, 3rd edn. Springer, Cham (2014)
Chang, K.-C.: Methods in Nonlinear Analysis. Springer Monographs in Mathematics. Springer, Berlin (2005)
Chazarain, J., Piriou, A.: Introduction à la théorie des équations aux dérivées partielles linéaires. Gauthier-Villars, Paris (1981)
Chow, S.N., Hale, J.K.: Methods of Bifurcation Theory. Springer, Berlin (1982)
Crandall, M.G., Rabinowitz, P.H.: Bifurcation from simple eigenvalues. J. Funct. Anal. 8, 321–340 (1971)
Dancer, E.N.: Global solution branches for positive mappings. Arch. Ration. Mech. Anal. 52, 181–192 (1973)
de Figueiredo, D.G.: Positive solutions of semilinear elliptic problems. In: Differential Equations. Lecture Notes in Mathematics, vol. 957, pp. 34–87. Springer, Berlin (1982)
Drábek, P., Milota, J.: Methods of Nonlinear Analysis, Applications to Differential Equations, 2nd ed. Birkhäuser Advanced Texts: Basler Lehrbücher. Birkhäuser/Springer, Basel (2013)
Gilbarg, D., Trudinger, N.S.: Elliptic Partial Differential Equations of Second Order, Reprint of the 1998 Edition. Classics in Mathematics. Springer, Berlin (2001)
Hörmander, L.: The Analysis of Linear Partial Differential Operators III, 1994th edn. Grundlehren Math. Wiss. Springer, Berlin (1994)
Iwasaki, C.: The asymptotic expansion of the fundamental solution for parabolic initial-boundary value problems and its application. Osaka J. Math. 31, 663–728 (1994)
Kazdan, J.L.: Unique continuation in geometry. Commun. Pure Appl. Math. 41, 667–681 (1988)
Kogoj, A., Lanconelli, E.: On semilinear \(\Delta _{\lambda }\)-Laplace equation. Nonlinear Anal. TMA 75, 4637–4649 (2012)
Kohn, J.J., Nirenberg, L.: Non-coercive boundary value problems. Commun. Pure Appl. Math. 18, 443–492 (1965)
Krasnosel’skii, M.A.: Positive Solutions of Operator Equations. P. Noordhoff, Groningen (1964)
Kreĭn, M.G., Rutman, M.A.: Linear operators leaving invariant a cone in a Banach space. Am. Math. Soc. Transl. 10, 199–325 (1962)
Landesman, E.A., Lazer, A.C.: Nonlinear perturbations of linear elliptic boundary value problems at resonance. J. Math. Mech. 19, 609–623 (1970)
Leray, J., Schauder, J.: Topologie et équations fonctionelles. J. Ann. Sci. École Norm. Sup. 51, 45–78 (1934)
Lyapunov, A.M.: The general problem of the stability of motion, Translated by A. T. Fuller from Édouard Davaux’s French translation (1907) of the 1892 Russian original. With an editorial (historical introduction) by Fuller, a biography of Lyapunov by V. I. Smirnov, and the bibliography of Lyapunov’s works collected by J. F. Barrett. Lyapunov centenary issue. Int. J. Control 55, 531–773 (1992)
Marano, S.A., Mosconi, S.J.N.: Some recent results on the Dirichlet problem for \((p, q)\)-Laplace equations. Discr. Contin. Dyn. Syst. Ser. S 11, 279–291 (2018)
Miranda, C.: Partial Differential Equations of Elliptic Type, second revised edn. Springer, Berlin (1970)
Nirenberg, L.: Topics in Nonlinear Functional Analysis, Revised Reprint of the 1974 Original. Courant Lecture Notes in Mathematics, No, 6. New York University, Courant Institute of Mathematical Sciences, New York. American Mathematical Society, Providence (2001)
Papageorgiou, N.S., Rădulescu, V.D., Repovš, D.D.: Nonlinear Analysis—Theory and Methods. Springer Monographs in Mathematics. Springer, Cham (2019)
Papageorgiou, N.S., Zhang, C.: Noncoercive resonant \((p,2)\)-equations with concave terms. Adv. Nonlinear Anal. 9, 228–249 (2020)
Plastock, R.A.: Nonlinear Fredholm maps of index zero and their singularities. Proc. Am. Math. Soc. 68, 317–322 (1978)
Rabinowitz, P.H.: Some global results for nonlinear eigenvalue problems. J. Funct. Anal. 7, 487–513 (1971)
Reed, M., Simon, B.: Methods of Modern Mathematical physics, IV: Analysis of Operators. Academic Press, San Diego (1978)
Runst, T., Sickel, W.: Sobolev Spaces of Fractional Order, Nemytskij Operators, and Nonlinear Partial Differential Equations. Walter de Gruyter, Berlin (1996)
Schmidt, E.: Zur Theorie der linearen und nichtlinearen Integralgleichungen, III. Teil. Über die Auflösung der nichtlinearen Integralgleichung und die Verzweigung ihrer Lösongen. Math. Ann. 65, 370–399 (1908)
Semenov, N.N.: Chemical Kinetics and Chain Reactions. Clarendon Press, Oxford (1935)
Szulkin, A.: On the number of solutions of some semilinear elliptic boundary value problems. Nonlinear Anal. TMA 6, 95–116 (1982)
Taira, K.: On some degenerate oblique derivative problems. J. Fac. Sci. Univ. Tokyo Sec. IA Math. 23, 259–287 (1976)
Taira, K.: Boundary value problems for elliptic integro-differential operators. Math. Z. 222, 305–327 (1996)
Taira, K.: Bifurcation for nonlinear elliptic boundary value problems I. Collect. Math. 47, 207–229 (1996)
Taira, K.: Diffusive logistic equations with degenerate boundary conditions. Mediterr. J. Math. 1, 315–365 (2004)
Taira, K.: Degenerate elliptic eigenvalue problems with indefinite weights. Mediterr. J. Math. 5, 133–162 (2008)
Taira, K.: Degenerate elliptic boundary value problems with asymmetric nonlinearity. J. Math. Soc. Jpn. 62, 431–465 (2010)
Taira, K.: Multiple solutions of semilinear degenerate elliptic boundary value problems. Math. Nachr. 284, 1–19 (2011)
Taira, K.: Multiple solutions of semilinear degenerate elliptic boundary value problems II. Math. Nachr. 284, 1554–1566 (2011)
Taira, K.: Degenerate elliptic boundary value problems with asymptotically linear nonlinearity. Rend. Circ. Mat. Palermo 2(60), 283–308 (2011)
Taira, K.: Multiple solutions of semilinear elliptic problems with degenerate boundary conditions. Mediterr. J. Math. 10, 731–752 (2013)
Taira, K.: A mixed problem of linear elastodynamics. J. Evol. Equ. 13, 481–507 (2013)
Taira, K.: Semigroups, Boundary Value Problems and Markov Processes. Springer Monographs in Mathematics, 2nd edn. Springer, Berlin (2014)
Taira, K.: Analytic Semigroups and Semilinear Initial-Boundary Value Problems. London Mathematical Society Lecture Note Series, vol. 434, 2nd edn. Cambridge University Press, Cambridge (2016)
Taira, K.: Spectral analysis of the hypoelliptic Robin problem. Ann. Univ. Ferrara Sez. VII Sci. Mat. 65, 171–199 (2019)
Taira, K.: Dirichlet problems with discontinuous coefficients and Feller semigroups. Rend. Circ. Mat. Palermo 2(69), 287–323 (2020)
Taira, K.: Ventcel’ boundary value problems for elliptic Waldenfels operators. Boll. Unione Mat. Ital. 13, 213–256 (2020)
Taira, K.: Boundary value problems and Markov processes. Lecture Notes in Mathematics, vol. 1499, 3rd edn. Springer, Berlin (2020)
Taira, K., Umezu, K.: Bifurcation for nonlinear elliptic boundary value problems II. Tokyo J. Math. 19, 387–396 (1996)
Triebel, H.: Theory of Function Spaces II. Monographs in Mathematics, vol. 84. Birkhäuser, Basel (1992)
Troianiello, G.M.: Elliptic Differential Equations and Obstacle Problems. The University Series in Mathematics. Plenum Press, New York (1987)
Wloka, J.: Partial Differential Equations. Cambridge University Press, Cambridge (1987)
Yosida, K.: Functional Analysis, Reprint of the Sixth (1980) Edition. Classics in Mathematics. Springer, Berlin (1980)
Zeidler, E.: Nonlinear Functional Analysis and Its Applications I. Springer, Berlin (1986)
Acknowledgements
I am grateful to the referees for their valuable suggestions and for informing me of additional references, which improved the presentation of this paper.
Author information
Authors and Affiliations
Corresponding author
Additional information
Dedicated to Professor Jerome Arthur Goldstein on the occasion of his 80th birthday.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix: The maximum principle in \(L^{p}\) Sobolev spaces
Appendix: The maximum principle in \(L^{p}\) Sobolev spaces
In this appendix we formulate various maximum principles for second-order, elliptic differential operators with discontinuous coefficients such as the weak and strong maximum principles (Theorems 10.1 and 10.2) and the Hopf boundary point lemma (Lemma 10.1) in the framework of \(L^{p}\) Sobolev spaces. The results here are adapted from Bony [20], Troianiello [72, Chapter 3] and also Taira [64, Chapter 8].
Let D be a bounded domain in Euclidean space \(\mathbf {R}^{N}\), \(N \ge 3\), with boundary \(\partial D\) of class \(C^{1,1}\). We consider a second-order, uniformly elliptic differential operator A with real discontinuous coefficients in non-divergence form
More precisely, we assume that the coefficients \(a^{ij}(x)\), \(b^{i}(x)\) and c(x) of the differential operator A satisfy the following three conditions:
-
(1)
\(a^{ij}(x) \in L^{\infty }(D)\), \(a^{ij}(x) = a^{ji}(x)\) for almost all \(x \in D\) and there exist a constant \(\lambda > 0\) such that
$$\begin{aligned} \dfrac{1}{\lambda } \vert \xi \vert ^{2} \le \sum _{i,j=1}^{N} a^{ij}(x)\xi _{i}\xi _{j} \le \lambda \left| \xi \right| ^{2} \quad \text{ for } \text{ almost } \text{ all } x \in D \text{ and } \text{ all } \xi \in \mathbf {R}^{N}. \end{aligned}$$ -
(2)
\(b^{i}(x) \in L^{\infty }(D)\) for all \(1 \le i \le N\).
-
(3)
\(c(x) \in L^{\infty }(D)\) and \(c(x) \ge 0\) for almost all \(x \in D\).
First, we state a variant of the weak maximum principle in the framework of \(L^{p}\) Sobolev spaces, due to Bony [20] [72, Chapter 3, Lemma 3.25]:
Theorem 10.1
(the weak maximum principle) If a function \(u \in W^{2,p}(D)\), \(N< p < \infty \), satisfies the condition
then we have the inequality
where
A detailed proof of Theorem 10.1 is given in Taira [67, Theorem 8.1].
Secondly, the Hopf boundary point lemma reads as follows [72, Chapter 3, Lemma 3.26, 68, Lemma 6.1]:
Lemma 10.1
(the boundary point lemma) Assume that a function \(u \in W^{2,p}(D)\), \(N< p < \infty \), satisfies the condition
If u(x) attains a non-negative, strict local maximum at a point \(x_{0}^{\prime }\) of \(\partial D\), then we have the inequality
(see Fig. 1).
Finally, we can obtain the following strong maximum principle for the operator A [20, Théorème 2, 68, Theorem 6.2, 72, Chapter 3, Theorem 3.27]:
Theorem 10.2
(the strong maximum principle) Assume that a function \(u \in W^{2,p}(D)\), \(N< p < \infty \),satisfies the condition
If u(x) attains a non-negative maximum at an interior point \(x_{0}\) of D, then it is a (non-negative) constant function.
Rights and permissions
About this article
Cite this article
Taira, K. Semilinear degenerate elliptic boundary value problems via the Semenov approximation. Rend. Circ. Mat. Palermo, II. Ser 70, 1305–1388 (2021). https://doi.org/10.1007/s12215-020-00560-z
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12215-020-00560-z
Keywords
- Semilinear elliptic problem
- Degenerate Robin condition
- Global static bifurcation
- Simple eigenvalue
- Leray–Schauder continuation method
- Semenov approximation