Linear and Weakly Nonlinear Stability Analyses of Two-Dimensional, Steady Brinkman–Bénard Convection Using Local Thermal Non-equilibrium Model

Abstract

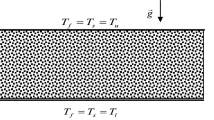
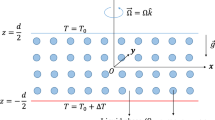
Effect of local thermal non-equilibrium (LTNE) on onset of Brinkman–Bénard convection and on heat transport is investigated. Rigid–rigid and free–free, isothermal boundaries are considered for investigation. The assumption of LTNE leads to an ‘advanced onset’ situation compared to that predicted by the local thermal equilibrium (LTE) assumption. This results in the ‘enhanced heat transport’ situation in the problem. Asymptotic analysis for small and large values of inter-phase heat transfer coefficient is also carried out on critical Rayleigh number, critical wave number and Nusselt number. In respect of boundary influences on onset and heat transport, it is found that classical results hold even under the LTNE assumption. The other parameters’ influences on onset and heat transport are qualitatively similar in LTNE and LTE cases.

Appendix

1.1 Derivation of Ginzburg–Landau Equation from the Lorenz Model by the Method of Multiscales (Siddheshwar and Kanchana 2017)

To use the method of multiscales, we expand the amplitudes in terms of the small quantity $\varepsilon$ as follows:

$$\begin{aligned} \left. \begin{aligned} A&=\varepsilon A_1+\varepsilon ^2 A_2+\varepsilon ^3 A_3+\cdots ,\\ B_1&=\varepsilon B_{11}+\varepsilon ^2 B_{12}+\varepsilon ^3 B_{13}+\cdots ,\\ D_1&=\varepsilon D_{11}+\varepsilon ^2 D_{12}+\varepsilon ^3 D_{13}+\cdots ,\\ C_1&=\varepsilon C_{11}+\varepsilon ^2 C_{12}+\varepsilon ^3 C_{13}+\cdots ,\\ E_1&=\varepsilon E_{11}+\varepsilon ^2 E_{12}+\varepsilon ^3 E_{13}+\cdots ,\\ r&= 1+\varepsilon ^2r_2, \end{aligned} \right\} , \end{aligned}$$

(66)

and a slow time scale may also be introduced as follows:

$$\begin{aligned} \tau ^*=\varepsilon ^2\tau . \end{aligned}$$

(67)

Substituting Eqs. (66)–(67) in Eq. (39), equating the coefficients of like powers of $\varepsilon$ on either side of the equation, we get

Coefficient of $\varepsilon$.

$$\begin{aligned} {L}{M^{(1)}}=0, \end{aligned}$$

(68)

where

$$\begin{aligned} L= & {} \left( \begin{array}{ccccc} -Fe_{1}\Lambda -\sigma ^2 &{}\quad 1 &{}\quad 0 &{}\quad 0 &{}\quad 0 \\ Fe_2 \text {} &{}\quad -\delta ^2-H &{}\quad 0 &{}\quad \dfrac{H^2 \gamma }{\delta ^2+H \gamma } &{}\quad 0 \\ 0 &{}\quad 0 &{}\quad -4 \pi ^2-H &{}\quad 0 &{}\quad \dfrac{H^2 \gamma }{4 \pi ^2+H \gamma } \\ 0 &{}\quad 1 &{}\quad 0 &{}\quad -1 &{}\quad 0 \\ 0 &{}\quad 0 &{}\quad 1 &{}\quad 0 &{}\quad -1 \end{array} \right) ,\\ M^{(i)}= & {} (A_i,B_{1i},D_{1i},C_{1i},E_{1i})^\mathrm{T}, \;\; (i=1, 2, 3). \end{aligned}$$

Solving Eq. (68), we get the solution of the first-order system as follows:

$$\begin{aligned} B_{11}= C_{11}=\frac{ \left( \gamma H+\delta ^2\right) Fe_{2} r_{0}}{\delta ^2 \left( \delta ^2+H+\gamma H\right) }A_{1},\;\;D_{11}= E_{11}=0. \end{aligned}$$

(69)

Coefficient of $\varepsilon ^2$

$$\begin{aligned} {L}{M^{(2)}}=[R_{21},\; R_{22},\; R_{23},\; R_{24},\; R_{25}]^\mathrm{T}, \end{aligned}$$

(70)

where

$$\begin{aligned} R_{21}= & {} 0,\;\; R_{22}= 0,\; R_{23}=-\frac{\left( \gamma H+\delta ^2\right) Fe_{2} r_{0} }{\delta ^2\left( \gamma H+H+\delta ^2\right) }A_{1}^2,\;\;R_{24}= 0,\;\; R_{25}= 0. \end{aligned}$$

Solving Eq. (70), we get the solution of the second-order system as follows:

$$\begin{aligned} A_{2}=B_{12}= C_{12}=0,\;\;D_{12}=E_{12}=\frac{\left( \gamma H+4 \pi ^2\right) \left( \gamma H+\delta ^2\right) Fe_{2} r_{0} }{4 \pi ^2 \delta ^2 \left( \gamma H+H+4 \pi ^2\right) \left( \gamma H+H+\delta ^2\right) }A_{1}^2. \end{aligned}$$

Coefficient of $\varepsilon ^3$

$$\begin{aligned} {L}{M^{(3)}}=[R_{31},\; R_{32},\; R_{33},\; R_{34},\; R_{35}]^\mathrm{T}, \end{aligned}$$

(71)

where

$$\begin{aligned} R_{31}= & {} \dfrac{1}{Pr}\dfrac{{A_1}}{\mathrm{d} \tau ^{*}},\;\; R_{32}= \dfrac{\mathrm{d} B_{11}}{\mathrm{d}\tau ^{*}}+A_1D_{12}-Fe_{2}r_2A_1, \\ R_{33}= & {} \dfrac{\mathrm{d} D_{11}}{\mathrm{d} \tau ^{*}}-A_{1} B_{12},\;\;R_{34}= \dfrac{\Gamma }{ \delta ^2+\gamma H}\dfrac{\mathrm{d} C_{11}}{\mathrm{d} \tau ^{*}},\;\; R_{35}= \dfrac{\Gamma }{4 \pi ^2+\gamma H}\dfrac{\mathrm{d} E_{11}}{\mathrm{d} \tau ^{*}}. \end{aligned}$$

The Fredholm solvability condition applied to the third-order system gives us the Ginzburg–Landau equation:

$$\begin{aligned} \dfrac{\mathrm{d}A_{1}(\tau ^*)}{\mathrm{d} \tau ^*}=\dfrac{Pr^*}{1+Pr^*}\left( P_{1}A_{1}(\tau ^*)-P_{2}A_{1}(\tau ^*)^{3}\right) , \end{aligned}$$

(72)

where

$$\begin{aligned} P_{1}= & {} \frac{\delta ^2 \left( \delta ^2+ \gamma H\right) \left( \delta ^2+H+\gamma H\right) r_{2}}{P_{3}},\;\; P_{2}=\left( \frac{\left( \gamma H+4 \pi ^2\right) (\delta ^2+ \gamma H)^2}{4 \pi ^2 P_{3}\left( \gamma H+H+4 \pi ^2\right) }\right) ,\\ Pr^{*}= & {} \frac{P_{3} Fe_{2} r_{0}}{\delta ^4 \left( \delta ^2+H+ \gamma H\right) ^2}Pr,\;\; P_{3}=\delta ^4+\gamma ^2 H^2+\gamma H \left( \Gamma H+2 \delta ^2 \right) . \end{aligned}$$