Skip to main content
SpringerLink
Log in

\({L}^{\varvec{p}}\) optimal feedback control of homoclinic bifurcation in a forced Duffing oscillator

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

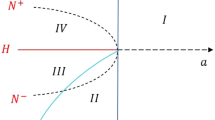
Abstract

This paper proposes an \(L^p\) optimal control method to shift the homoclinic bifurcation of single-degree-of-freedom nonlinear oscillators. The homoclinic intersection between stable and unstable manifolds is detected analytically using the Melnikov method. An optimization strategy is formulated to obtain the control gain that minimizes the absolute value of the largest magnitude characteristic multiplier of the periodic orbit that emerges due to the shift of the homoclinic bifurcation. The optimal control gain is obtained by considering the stability of this periodic orbit. The cross-entropy method is used to obtain a numerical optimal solution to the optimization problem and, consequently, to obtain an optimal controller for the feedback method presented here. As an example of the \(L^p\) optimal control strategy, we consider the periodically forced Duffing oscillator with a twin-well potential. The numerical results demonstrate the capability of the \(L^p\) optimal control procedure to shift the homoclinic bifurcation. Moreover, the \(L^p\) optimal control can help regularize the fractal basin boundaries of the two confined attractors in the two potential wells.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

Data availability statement

The manuscript has no associated data.

References

  1. Chicone, C.: Ordinary Differential Equations with Applications. Springer (2006)

  2. Nayfeh, A.H., Balachandran, B.: Applied nonlinear dynamics: analytical, computational, and experimental methods. John Wiley & Sons (2008)

  3. Lenci, S., Rega, G.: Optimal numerical control of single-well to cross-well chaos transition in mechanical systems. Chaos, Solitons Fractals 15(1), 173–186 (2003). https://doi.org/10.1016/S0960-0779(02)00116-9

    Article  MathSciNet  MATH  Google Scholar 

  4. Rega, G., Lenci. S, Thompson, J.M.: Controlling chaos: the OGY method, its use in mechanics, and an alternative unified framework for control of non-regular dynamics. In Nonlinear Dynamics and Chaos: Advances and Perspectives. Springer 211-269 (2010). https://doi.org/10.1007/978-3-642-04629-2_11

  5. Lenci, S., Rega, G.: Optimal control of homoclinic bifurcation: theoretical treatment and practical reduction of safe basin erosion in the Helmholtz oscillator. J. Vib. Control 9(3–4), 281–315 (2003). https://doi.org/10.1177/107754603030753

    Article  MathSciNet  MATH  Google Scholar 

  6. Lima, R., Pettini, M.: Suppression of chaos by resonant parametric perturbations. Phys. Rev. A 41(2), 726 (1990). https://doi.org/10.1103/PhysRevA.41.726

    Article  MathSciNet  Google Scholar 

  7. Dzhanoev, A.R., Loskutov, A., Cao, H., Sanjuán, M.A.: A new mechanism of the chaos suppression. Discrete Contin. Dyn. Syst. B 7(2), 275 (2007). https://doi.org/10.3934/dcdsb.2007.7.275

    Article  MathSciNet  MATH  Google Scholar 

  8. Lenci, S., Rega, G.: Optimal control of nonregular dynamics in a Duffing oscillator. Nonlinear Dyn. 33(1), 71–86 (2003). https://doi.org/10.1023/A:1025509014101

    Article  MathSciNet  MATH  Google Scholar 

  9. Lenci, S., Rega, G.: Control of the homoclinic bifurcation in buckled beams: infinite dimensional vs reduced order modeling. Int. J. Non-Linear Mech. 43(6), 474–89 (2008). https://doi.org/10.1016/j.ijnonlinmec.2007.10.007

    Article  MATH  Google Scholar 

  10. Lenci, S., Rega, G.: Global optimal control and system-dependent solutions in the hardening Helmholtz-Duffing oscillator. Chaos, Solitons Fractals 21(5), 1031–46 (2004). https://doi.org/10.1016/S0960-0779(03)00387-4

    Article  MathSciNet  MATH  Google Scholar 

  11. Cao, H.: Primary resonant optimal control for homoclinic bifurcations in single-degree-of-freedom nonlinear oscillators. Chaos, Solitons Fractals 24(5), 1387–98 (2005). https://doi.org/10.1016/j.chaos.2004.09.084

    Article  MathSciNet  MATH  Google Scholar 

  12. Chacón, R., Martínez, P.J., Martínez, J.A.: Dissipative dynamics of a particle in a vibrating periodic potential: chaos and control. Phys. Rev. E 92(6), 062921 (2015). https://doi.org/10.1103/PhysRevE.92.062921

    Article  MathSciNet  Google Scholar 

  13. Chacón, R.: Role of ultrasubharmonic resonances in taming chaos by weak harmonic perturbations. EPL (Europhys. Lett.) 54(2), 148 (2001). https://doi.org/10.1209/epl/i2001-00288-6

    Article  Google Scholar 

  14. Chacón, R., Martínez, J.A.: Inhibition of chaotic escape from a potential well by incommensurate escape-suppressing excitations. Phys. Rev. E 65(3), 036213 (2002). https://doi.org/10.1103/PhysRevE.65.036213

    Article  Google Scholar 

  15. Chacón, R., Miralles, J.J., Martínez, J.A., Balibrea, F.: Taming chaos in damped driven systems by incommensurate excitations. Commun. Nonlinear Sci. Numer. Simul. 73, 307–318 (2019). https://doi.org/10.1016/j.cnsns.2019.02.014

    Article  MathSciNet  MATH  Google Scholar 

  16. Martínez, P.J., Euzzor, S., Meucci, R., Chacón, R.: Suppression of chaos by incommensurate excitations: Theory and experimental confirmations. Commun. Nonlinear Sci. Numer. Simul. 83, 105–137 (2020). https://doi.org/10.1016/j.cnsns.2019.105137

    Article  MathSciNet  MATH  Google Scholar 

  17. Piccirillo, V.: Control of homoclinic bifurcation in two-dimensional dynamical systems by a feedback law based on \(L^p\) spaces. J. Franklin Inst. 359, 5097–5124 (2022). https://doi.org/10.1016/j.jfranklin.2022.04.032

    Article  MathSciNet  MATH  Google Scholar 

  18. Rubinstein, R.Y.: Optimization of computer simulation models with rare events. Eur. J. Oper. Res. 99(1), 89–112 (1997). https://doi.org/10.1016/S0377-2217(96)00385-2

    Article  MathSciNet  MATH  Google Scholar 

  19. Rega, G., Settimi, V.: Global dynamics perspective on macro-to nano-mechanics. Nonlinear Dyn. 103, 1259–1303 (2021). https://doi.org/10.1007/s11071-020-06198-x

    Article  Google Scholar 

  20. Hacker, E., Gottlieb, O.: Local and global bifurcations in magnetic resonance force microscopy. Nonlinear Dyn. 99(1), 201–225 (2020). https://doi.org/10.1007/s11071-019-05401-y

    Article  MATH  Google Scholar 

  21. Awrejcewicz, J., Holicke, M.: Analytical prediction of chaos in rotated Froude pendulum. Nonlinear Dyn. 47, 3–24 (2007). https://doi.org/10.1007/s11071-006-9054-8

    Article  MathSciNet  MATH  Google Scholar 

  22. Zhao, F., Ma, X., Cao, S.: Periodic bursting oscillations in a hybrid Rayleigh-Van der Pol-Duffing oscillator. Nonlinear Dyn. 111, 2263–2279 (2023). https://doi.org/10.1007/s11071-022-07940-3

    Article  Google Scholar 

  23. Han, N., Lu, P., Li, Z.: An approximate technique to test chaotic region in a rotating pendulum system with bistable characteristics. Nonlinear Dyn. 104, 191–214 (2021). https://doi.org/10.1007/s11071-021-06274-w

    Article  Google Scholar 

  24. Guckenheimer, J., Holmes, P.: Nonlinear oscillations, dynamical systems, and bifurcations of vector fields. Springer (1983)

  25. Perko, L.: Differential Equations and Dynamical Systems. Springer (2013)

  26. Slotine, J.J., Li, W.: Applied Nonlinear Control. Prentice hall (1991)

  27. Tereshko, V., Chacón, R., Preciado, V.: Controlling chaotic oscillators by altering their energy. Phys. Lett. A 320(5–6), 408–16 (2004). https://doi.org/10.1016/j.physleta.2003.11.057

    Article  MathSciNet  Google Scholar 

  28. Strogatz, S.H.: Nonlinear Dynamics and Chaos: with applications to physics, biology, chemistry, and engineering. CRC Press (2018)

  29. Deshmukh, V.S., Sinha, S.C.: Control of dynamic systems with time-periodic coefficients via the Lyapunov-Floquet transformation and backstepping technique. J. Vib. Control 10(10), 1517–1533 (2004). https://doi.org/10.1177/1077546304042064

  30. Pyragas, K.: Delayed feedback control of chaos. Philos. Transact. Royal Soc. A Math. Phys. Eng. Sci. 364(1846), 2309–2334 (2006). https://doi.org/10.1098/rsta.2006.1827

    Article  MathSciNet  MATH  Google Scholar 

  31. Zheng, Y.G., Yu, J.L.: Stabilization of multi-rotation unstable periodic orbits through dynamic extended delayed feedback control. Chaos, Solitons Fractals 161, 112362 (2022). https://doi.org/10.1016/j.chaos.2022.112362

    Article  MathSciNet  MATH  Google Scholar 

  32. Montagnier, P., Spiteri, R.J., Angeles, J.: The control of linear time-periodic systems using Floquet-Lyapunov theory. Int. J. Control 77(5), 472–90 (2004). https://doi.org/10.1080/00207170410001667477

    Article  MathSciNet  MATH  Google Scholar 

  33. Chagas, T.P., Toledo, B.A., Rempel, E.L., Chian, A.L., Valdivia, J.A.: Optimal feedback control of the forced van der Pol system. Chaos, Solitons Fractals 45(9–10), 1147–56 (2012). https://doi.org/10.1016/j.chaos.2012.06.004

    Article  MathSciNet  Google Scholar 

  34. Rubinstein, R.: The cross-entropy method for combinatorial and continuous optimization. Methodol. Comput. Appl. Probab. 1(2), 127–90 (1999). https://doi.org/10.1023/A:1010091220143

    Article  MathSciNet  MATH  Google Scholar 

  35. Cunha, A.: Enhancing the performance of a bistable energy harvesting device via the cross-entropy method. Nonlinear Dyn. 103(1), 137–55 (2021). https://doi.org/10.1007/s11071-020-06109-0

    Article  Google Scholar 

  36. Rubinstein, R.Y., Kroese, D.P.: The cross-entropy method: a unified approach to combinatorial optimization, Monte-Carlo simulation, and machine learning. Springer (2004)

  37. Kroese, D.P., Porotsky, S., Rubinstein, R.Y.: The cross-entropy method for continuous multi-extremal optimization. Methodol. Comput. Appl. Probab. 8(3), 383–407 (2006). https://doi.org/10.1007/s11009-006-9753-0

    Article  MathSciNet  MATH  Google Scholar 

  38. Nusse, H.E., Yorke, J.A.: Dynamics: Numerical Explorations. Springer (2012)

Download references

Funding

The authors declare that no funds, grants, or other support were received during the preparation of this manuscript.

Author information

Authors and Affiliations

  1. Department of Mathematics, Federal Technological University of Parana - UTFPR, Ponta Grossa, PR, Brazil

    Vinícius Piccirillo

Authors
  1. Vinícius Piccirillo
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Vinícius Piccirillo.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendix A: CE optimization Algorithm

Appendix A: CE optimization Algorithm

This appendix presents the computational recipe for the CE procedure. The algorithm for the computational implementation of the CE method is shown in Algorithm 1.

figure a

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Piccirillo, V. \({L}^{\varvec{p}}\) optimal feedback control of homoclinic bifurcation in a forced Duffing oscillator. Nonlinear Dyn 111, 13017–13037 (2023). https://doi.org/10.1007/s11071-023-08575-8

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-023-08575-8

Keywords

Search

Navigation