Skip to main content
SpringerLink
Log in

A generalized Padé–Lindstedt–Poincaré method for predicting homoclinic and heteroclinic bifurcations of strongly nonlinear autonomous oscillators

  • Original Paper
  • Published:
Nonlinear Dynamics Aims and scope Submit manuscript

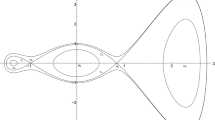
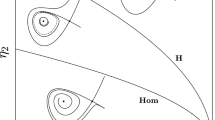
Abstract

By combining the generalized Padé approximation method and the well-known Lindstedt–Poincaré method, a novel technique, referred to as the generalized Padé–Lindstedt–Poincaré method, is proposed for determining homo-/heteroclinic orbits of nonlinear autonomous oscillators. First, the classical Padé approximation method is generalized. According to this generalization, the numerator and denominator of the Padé approximant are extended from polynomial functions to a series composed of any kind of continuous function, which means that the generalized Padé approximant is not limited to some certain forms, but can be constructed variously in solving different matters. Next, the generalized Padé approximation method is introduced into the Lindstedt–Poincaré method’s procedure for solving the perturbation equations. Via the proposed generalized Padé–Lindstedt–Poincaré method, the homo-/heteroclinic bifurcations of the generalized Helmholtz–Duffing–Van der Pol oscillator and \(\Phi ^{6}\)-Van der Pol oscillator are predicted. Meanwhile, the analytical solutions to these oscillators are also calculated. To illustrate the accuracy of the present method, the solutions obtained in this paper are compared with those of the Runge–Kutta method, which shows the method proposed in this paper is both effective and feasible. Furthermore, the proposed method can be also utilized to solve many other oscillators.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Guckenheimer, J., Holmes, P.: Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields. Springer, New York (1983)

    Book  MATH  Google Scholar 

  2. Wiggins, S.: Introduction to Applied Nonlinear Dynamical Systems and Chaos. Springer, New York (1990)

    Book  MATH  Google Scholar 

  3. Nayfeh, A.H.: Introduction to Perturbation Techniques. Wiley, New York (1981)

    MATH  Google Scholar 

  4. Mickens, R.E.: Nonlinear Oscillations. Cambridge University Press, New York (1981)

    MATH  Google Scholar 

  5. Chen, S.H., Chen, Y.Y., Sze, K.Y.: A hyperbolic perturbation method for determining homoclinic solution of certain strongly nonlinear autonomous oscillators. J. Sound Vib. 322, 381–392 (2009)

    Article  Google Scholar 

  6. Chen, Y.Y., Chen, S.H.: Homoclinic and heteroclinic solutions of cubic strongly nonlinear autonomous oscillators by the hyperbolic perturbation method. Nonlinear Dyn. 58, 417–429 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  7. Chen, S., Chen, Y., Sze, K.: Homoclinic and heteroclinic solutions of cubic strongly nonlinear autonomous oscillators by hyperbolic Lindstedt-Poincaré method. Sci. China Technol. Sci. 53, 692–702 (2010)

    Article  MATH  Google Scholar 

  8. Chen, Y.Y., Chen, S.H., Sze, K.Y.: A hyperbolic Lindstedt–Poincaré method for homoclinic motion of a kind of strongly nonlinear autonomous oscillators. Acta Mech. Sinica 25, 721–729 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  9. Xu, Z., Chan, H.S.Y., Chung, K.W.: Separatrices and limit cycles of strongly nonlinear oscillators by the perturbation-incremental method. Nonlinear Dyn. 11, 213–233 (1996)

    Article  MathSciNet  Google Scholar 

  10. Cao, Y.Y., Chung, K.W., Xu, J.: A novel construction of homoclinic and heteroclinic orbits in nonlinear oscillators by a perturbation-incremental method. Nonlinear Dyn. 64, 221–236 (2011)

    Article  MathSciNet  Google Scholar 

  11. Li, Z., Tang, J., Cai, P.: A generalized harmonic function perturbation method for determining limit cycles and homoclinic orbits of Helmholtz–Duffing oscillator. J. Sound Vib. 332, 5508–5522 (2013)

    Article  Google Scholar 

  12. Li Z., Tang J., Cai P.: Predicting Homoclinic and Heteroclinic Bifurcation of Generalized Duffing-Harmonic-van de Pol Oscillator. Qual. Theory Dyn. Syst. (2015). doi:10.1007/s12346-015-0138-z

  13. Mikhlin, Y.V.: Analytical construction of homoclinic orbits of two-and three-dimensional dynamic systems. J. Sound Vib. 230, 971–983 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  14. Manucharyan, G.V., Mikhlin, Y.V.: The construction of homo- and heteroclinic orbits in non-linear systems. J. Appl. Math. Mech. 69, 39–48 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  15. Zhang, Q.C., Feng, J.J., Wang, W.: The construction of homoclinic and heteroclinic orbit in two-dimensional nonlinear system based on the quasi-Padé approximation(in Chinese). Chin. J. Theor. Appl. Mech. 43, 914–921 (2011)

    MathSciNet  Google Scholar 

  16. Leung, A.Y.T., Guo, Z.: Homotopy perturbation for conservative Helmholtz–Duffing oscillators. J. Sound Vib. 325, 287–296 (2009)

    Article  Google Scholar 

  17. Lai, S.K., Harrington, J., Xiang, Y., Chow, K.W.: Accurate analytical perturbation approach for large amplitude vibration of functionally graded beams. Int. J. Nonlinear. Mech 47, 473–480 (2012)

    Article  Google Scholar 

  18. Lenzi, S.M., Lefteriu, S., Beriot, H., Desmet, W.: A fast frequency sweep approach using Padé approximations for solving Helmholtz finite element models. J. Sound Vib. 332, 1897–1917 (2013)

    Article  Google Scholar 

  19. Yang, C.-C.: Robust synchronization and anti-synchronization of identical \(\Phi ^{6}\) oscillators via adaptive sliding mode control. J. Sound Vib. 331, 501–509 (2012)

    Article  Google Scholar 

  20. Njah, A.N.: Synchronization via active control of identical and non-identical \(\Phi ^{6}\) chaotic oscillators with external excitation. J. Sound Vib. 327, 322–332 (2009)

    Article  Google Scholar 

  21. Yue, X., Xu, W., Jia, W., Wang, L.: Stochastic response of a \(\Phi ^{6}\) oscillator subjected to combined harmonic and Poisson white noise excitations. Phys. A: Stat. Mech. Appl. 392, 2988–2998 (2013)

    Article  MathSciNet  Google Scholar 

  22. Baker, G.A.: Essential of Padé Approximants. Academic, New York (1975)

  23. Ryaboy, V., Lefebvre, R., Moiseyev, N.: Cumulative reaction probabilities using Padé analytical continuation procedures. J. Chem. Phys. 99, 3509–3515 (1993)

    Article  Google Scholar 

  24. Chrysos, M., Lefebvre, R., Atabek, O.: On the self-generation of asymptotic boundary conditions in energy quantization. J. Phys. B: Atom. Mol. Opt. Phys. 27, 3005–3015 (1994)

  25. Emaci, E., Vakakis, A.F., Andrianov, I.V., Mikhlin, Y.: Study of two-dimensional axisymmetric breathers using Padé approximants. Nonlinear Dyn. 13, 327–338 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  26. Martin, P., Baker, J.G.A.: Two-point quasifractional approximant in physics. Truncation error. J. Math. Phys. 32, 1470–1477 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  27. Li, Z.B., Tang, J.S., Cai, P.: A generalized Padé approximation method of solving homoclinic and heteroclinic orbits of strongly nonlinear autonomous oscillators. Chin. Phys. B 23, 120501–120501 (2014)

    Article  Google Scholar 

  28. Belhaq, M., Fiedler, B., Lakrad, F.: Homoclinic connections in strongly self-excited nonlinear oscillators: the Melnikov function and the elliptic Lindstedt–Poincaré method. Nonlinear Dyn. 23, 67–86 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  29. Merkin, J.H., Needham, D.J.: On infinite period bifurcations with an application to roll waves. Acta Mech. 60, 1–16 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  30. Yarman, C.E., Flagg, G.M.: Generalization of padé approximation from rational functions to arbitrary analytic functions -Theory. Math. Comput. 84, 1835–1860 (2015)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgments

This work was supported by the National Natural Science Foundation of China (Grant Nos. 11172093 and 11372102).

Author information

Authors and Affiliations

  1. School of Mathematics and Physics, University of South China, Hengyang, 421001, China

    Zhenbo Li

  2. College of Mechanical and Vehicle Engineering, Hunan University, Changsha, 410082, China

    Jiashi Tang

Authors
  1. Zhenbo Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jiashi Tang
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Zhenbo Li.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Li, Z., Tang, J. A generalized Padé–Lindstedt–Poincaré method for predicting homoclinic and heteroclinic bifurcations of strongly nonlinear autonomous oscillators. Nonlinear Dyn 84, 1201–1223 (2016). https://doi.org/10.1007/s11071-015-2563-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11071-015-2563-6

Keywords

Search

Navigation