An approximate technique to test chaotic region in a rotating pendulum system with bistable characteristics

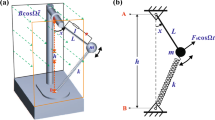
Abstract

This paper is concerned with the chaotic dynamics of a rotating pendulum system with bistable characteristics subjected to a viscous damping and a harmonic forcing. As a prototype of the single-degree-of-freedom system with bistable characteristics, this pendulum system exhibits a transition from smooth to discontinuous dynamics by changing a geometrical parameter. The dynamic behaviors of the unperturbed system with irrational nonlinearity bear significant similarities to the coupling of a simple pendulum and the smooth and discontinuous (SD) oscillator with the coexistence of the standard homoclinic orbits of Duffing type and pendulum type and the coexistence of the nonstandard homoclinic orbits of SD type and pendulum type in the smooth and discontinuous case, respectively. For the perturbed smooth system, we present an approximate technique to analytically obtain the lower bound line for horseshoes chaos arising from the homoclinic orbits of Duffing-type and Pendulum-type tangling, which overcomes the natural difficulties of solving the analytical expression of the homoclinic orbits and calculating the complicated Melnikov integrals. The chaotic thresholds of the perturbed discontinuous system are calculated by applying the numerical technique due to its non-smooth feature. Numerical simulations are carried out to certify the chaotic thresholds, which show the efficiency of the proposed techniques and demonstrate the predicated chaotic motions. Finally, different types of chaotic motions are illustrated via the cylindrical phase portraits. The contribution of this study is also helpful for exploring the dynamical behaviors of the complex nonlinear dynamical system containing the standard homoclinic or heteroclinic orbit in terms of the quantitative calculation.

Appendix

When $f_{0}=0$ and $\omega =0$, the damping pendulum system can be written as

$$\begin{aligned} \left\{ \begin{array}{l} {\dot{x}}=y,\\ {\dot{y}}=-\xi y-\sin {x}- q\lambda \sin {x}\left( 1-\displaystyle \frac{1}{\sqrt{1+\lambda ^{2}-2\lambda \cos {x}}}\right) . \end{array}\right. \end{aligned}$$

This study focuses on the rotating pendulum system with bistable characteristics which means that there exist five equilibria $({x_{i},y_{i}})_{i=1,2,3,4,5}$ in the damping pendulum system satisfying $|\rho |<1$ or

$$\begin{aligned} \left\{ (\lambda ,q)~\bigg |~q > \displaystyle \frac{\lambda -1}{\lambda \left( 2-\lambda \right) }, ~\lambda \in (1,2), ~q\in (0,+\infty ) \right\} . \end{aligned}$$

Then, the Jacobian matrix at equilibria $({x_{i},y_{i}})_{i=1,2,3,4,5}$ can be expressed as

$$\begin{aligned} J_{({x_{i},y_{i}})}=\left[ \begin{array}{ll} 0&{} \quad 1\\ K(x) &{} \quad -\xi \\ \end{array} \right] , \end{aligned}$$

and

$$\begin{aligned} K(x)= & {} -(1+q\lambda )\cos {x}+\displaystyle \frac{q\lambda \cos {x}}{\sqrt{1+\lambda ^{2}-2\lambda \cos {x}}}\\&- q\lambda ^{2}\sin ^{2}{x}\left( {{1+\lambda ^{2}-2\lambda \cos {x}}}\right) ^{-1.5}, \end{aligned}$$

which leads to the characteristic equation

$$\begin{aligned} \varLambda ^{2}+\xi \varLambda -K(x_{i})=0. \end{aligned}$$

Two eigenvalues $\varLambda _{1,2}$ can be derived by calculating the characteristic equation and written as

$$\begin{aligned} \varLambda _{1,2}=\displaystyle \frac{-\xi \pm \sqrt{\xi ^{2}+4K(x_{i})}}{2}, \end{aligned}$$

where

$$\begin{aligned} \Delta= & {} \xi ^{2}+4K(x_{i}),~~K(x_{1})\\= & {} q\displaystyle \frac{\lambda (2-\lambda )}{\lambda -1}-1>0,\\ K(x_{2,3})= & {} 1+\displaystyle \frac{q\lambda ^{2}}{1+\lambda }>0,~K(x_{4,5})\\= & {} -\displaystyle \frac{\left( 1-\rho ^2\right) \left( 1+q\lambda \right) ^3}{q^{2}\lambda }<0. \end{aligned}$$

Then, we will explore the effect of nonnegative damping $\xi$ on the equilibria $({x_{i},y_{i}})_{i=1,2,3,4,5}$ based upon two eigenvalues. The detailed analysis is listed in the following.

(a) When $\xi >0$ and $\xi =0$, for the equilibria $(x_{1},y_{1})$, $(x_{2},y_{2})$ and $(x_{3},y_{3})$, there exist two unequal real roots

$$\begin{aligned} \varLambda _{1,2}=\displaystyle \frac{-\xi \pm \sqrt{\xi ^{2}+4K(x_{1,2,3})}}{2},~~\varLambda _{1,2}=\pm \sqrt{K(x_{1,2,3})}. \end{aligned}$$

due to $\Delta >0$ and $K(x_{1,2,3})>0$. It is concluded that the equilibria $(x_{1},y_{1})$, $(x_{2},y_{2})$ and $(x_{3},y_{3})$ are saddle points.

(b) No damping: When $\xi =0$, for the equilibria $(x_{4},y_{4})$ and $(x_{5},y_{5})$, there exists a pair of conjugate pure virtual roots

$$\begin{aligned} \varLambda _{1,2}=\pm \sqrt{-K(x_{4,5})}~i. \end{aligned}$$

due to $\Delta <0$ and $K(x_{4,5})<0$. It is concluded that $(x_{4},y_{4})$ and $(x_{5},y_{5})$ are centers.

(c) Weak damping: When $\xi ^{2}+4K(x_{4,5})<0$, for the equilibria $(x_{4},y_{4})$ and $(x_{5},y_{5})$, there exists a pair of conjugate virtual roots

$$\begin{aligned} \varLambda _{1,2}=\displaystyle \frac{-\xi \pm \sqrt{-\xi ^{2}-4K(x_{1,2,3})}~i}{2} \end{aligned}$$

due to $\Delta <0$ and $K(x_{4,5})<0$. Since the real part of the eigenvalues are less than zero, we conclude that $(x_{4},y_{4})$ and $(x_{5},y_{5})$ are stable focus points.

(d) Strong damping: When $\xi ^{2}+4K(x_{4,5})>0$, for the equilibria $(x_{4},y_{4})$ and $(x_{5},y_{5})$, there exist two unequal real roots

$$\begin{aligned} \varLambda _{1,2}=\displaystyle \frac{-\xi \pm \sqrt{\xi ^{2}+4K(x_{1,2,3})}}{2} \end{aligned}$$

due to $\Delta >0$ and $K(x_{4,5})<0$. Since two eigenvalues are less than zero, we conclude that $(x_{4},y_{4})$ and $(x_{5},y_{5})$ are stable node points.

(e) Critical damping: When $\xi ^{2}+4K(x_{4,5})=0$, for the equilibria $(x_{4},y_{4})$ and $(x_{5},y_{5})$, there exist two equal real roots

$$\begin{aligned} \varLambda _{1,2}=-\displaystyle \frac{\xi }{2} \end{aligned}$$

due to $\Delta =0$ and $K(x_{4,5})<0$. It is concluded that $(x_{4},y_{4})$ and $(x_{5},y_{5})$ are stable degenerate nodes.