Several Theoretical Perspectives of Ferrite-Based Materials—Part 1: Transmission Line Theory and Microwave Absorption

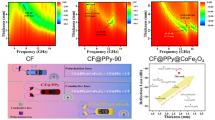
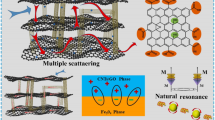
Abstract

A theoretical model for microwave absorption from both forced non-resonance oscillation and resonance is constructed using the transmission line theory familiar to microwave engineers. The model covers both the single-phase ferrite and its composites of interest to material scientists, and can be applied to a variety of different absorption mechanisms. The transmission line theory is also shown to be consistent with the band theory of solids, a relationship that has not been revealed previously. The work bridges the gap between the interests of microwave engineers and material scientists.

Appendices

Appendix: Applications of Bloch’s Theorem

A.1 One Band

A wave function in a crystal must obey Bloch’s theorem (136).

$$ \psi ({\mathrm {\mathbf r}})=e^{j{\mathrm{\mathbf{k}}}\cdot {\mathrm{\mathbf{r}}}}u({\mathrm{\mathbf r}}) $$

(136)

u(r) in (136) is a periodic function regarding symmetry operations in a crystal. Vector r specifies the position of the electron in the orbital. The direction of vector k defines a translation in the crystal and its value related to energy of the orbital is often written in the form 2 π/ λ where λ is the wavelength. This k also appears in (133) for a one-dimensional crystal. Bloch’s theorem (136) is related to phase shift represented by (121), which means that a translation symmetry operation on a well-behaved function in a crystal generates a phase shift for the function.

If every atom in a one-dimensional crystal separated by a distance of |a| contributes an atomic orbital φ to a well-behaved crystal wave function ψ, then (137) is a crystal wave function that satisfies Bloch’s theorem and this is proved from (138)–(139).

$$ \psi (\mathrm{\mathbf{r}})=\frac{1}{\sqrt N }\sum\limits_{n=0}^{N-1} {e^{j{\mathrm {\mathbf k}}\cdot n{\mathrm{\mathbf a}}}} \varphi ({\mathrm{\mathbf r}}-n{\mathrm{\mathbf a}}) $$

(137)

N is the number of atoms in a complex unit cell. Vector r originates from one of the atoms. It represents the position of an electron and the origin is translated from one atom to another in (137) [42]. (137) can be rewritten in the form of (136).

$$ \begin{array}{l} \psi ({\mathrm {\mathbf r}})=\frac{1}{\sqrt N }e^{j{\mathrm {\mathbf k}}\cdot {\mathrm {\mathbf r}}}\sum\limits_{n=0}^{N-1} {e^{-j{\mathrm {\mathbf k}}\cdot {\mathrm {\mathbf r}}}e^{j{\mathrm {\mathbf k}}\cdot n{\mathrm {\mathbf a}}}} \varphi ({\mathrm {\mathbf r}}-n{\mathrm {\mathbf a}}) \\ =e^{j{\mathrm {\mathbf k}}\cdot {\mathrm {\mathbf r}}}u({\mathrm {\mathbf r}}) \\ \end{array} $$

(138)

u(r) in (138) is a periodic function proved by (139) involving any translation operation m a.

$$ \begin{array}{l} u({\mathrm {\mathbf r}}-m{\mathrm {\mathbf a}})=\frac{1}{\sqrt N }\sum\limits_{n{=}0}^{N-1} {e^{-j{\mathrm {\mathbf k}}\cdot ({\mathrm {\mathbf r}}-m{\mathrm {\mathbf a}})}e^{j{\mathrm {\mathbf k}}\cdot n{\mathrm {\mathbf a}}}} \varphi [({\mathrm {\mathbf r}}-m{\mathrm {\mathbf a}})-n{\mathrm {\mathbf a}}] \\ =\frac{1}{\sqrt N }\sum\limits_{n=0}^{N-1} {e^{-j{\mathrm {\mathbf k}}\cdot [{\mathrm {\mathbf r}}-(m+n){\mathrm {\mathbf a}}]}} \varphi [{\mathrm {\mathbf r}}-(m+n){\mathrm {\mathbf a}}] \\ =\frac{1}{\sqrt N }\sum\limits_{n=0}^{N-1} {e^{-j{\mathrm {\mathbf k}}\cdot [{\mathrm {\mathbf r}}-n'(m,n){\mathrm {\mathbf a}}]}} \varphi [{\mathrm {\mathbf r}}-n^{\prime} (m,n){\mathrm {\mathbf a}}]=u({\mathrm {\mathbf r}}) \\ \end{array} $$

(139)

Equation (139) is valid because when n is summed over all the N atoms in the complex cell, $n^{\prime }$ can similarly be summed in an equivalent cell. If

$$ \int\limits_V {\varphi \ast } ({\mathrm {\mathbf r}}-n^{\prime} {\mathbf{a}})\hat{H}\varphi ({\mathbf{r}}-n{\mathbf{a}})d\tau =\left\{ {{\begin{array}{*{20}c} {\alpha,} & {n^{\prime} =n} \\ {\beta,}& {n^{\prime} =n\pm 1} \\ {0,} & {\text{otherwise}} \\ \end{array}}} \right. $$

(140)

Then, the energy of the crystal orbital will be

$$\begin{array}{@{}rcl@{}} &&E_{C} =\displaystyle\int\limits_{V} \psi ({\mathrm {\mathbf r}})\hat{H} \psi ({{\mathbf r}})d\tau =\frac{1}{N}\sum\limits_{{n}^{\prime} ,n} e^{j| \mathbf{k}|(n^{\prime}-n)| \mathbf{a} |}\\ &&\displaystyle\int\limits_{V} {\varphi\ast} ({\mathbf{r}}-n^{\prime} {\mathbf{a}})\hat{H} \varphi ({\mathbf{r}}-n {\mathbf{a}})d\tau =\frac{1}{N}\sum\limits_{n} \alpha\\ && \!\!+\frac{1}{N}\sum\limits_{n} {\beta (e^{j| {\mathbf{k}} || {\mathbf{a}} |}\!+e^{-j| {\mathbf{k}}|| {\mathbf{a}} |})} =\alpha \!+2\beta \cos (| {\mathbf{k}}| |\mathbf{a}|) \end{array} $$

(141)

When |k| takes the value from 0 to π/|a|, an energy band from (α + 2β) to (α − 2β) is created.

A.2 Two Bands

The theorem for two bands can be easily explained by reference to trans-polythene (Fig. 8). If each of the atoms from trans-polythene [44] contributes one atomic orbital (φ ₁ or φ ₂) to a crystal orbital, then the crystal-adapted orbitals (ϕ₁,ϕ₂) for the two sets of atoms shown by Fig. 1 can be written in a form similar to (137).

$$\begin{array}{@{}rcl@{}} \phi_{1} ({\mathrm {\mathbf r}})&=&\frac{1}{\sqrt N }\sum\limits_{n=0}^{N-1} {e^{jk(n\left| {{\mathrm {\mathbf a}}} \right|-d/2)}} \varphi_{1} [({\mathrm {\mathbf r}}+\frac{{\mathrm {\mathbf b}}}{2})-n{\mathrm {\mathbf a}}]\\ &=&\frac{1}{\sqrt N }\sum\limits_{n=0}^{N-1} {e^{jk(n\left| {{\mathrm {\mathbf a}}} \right|-d/2)}} \varphi_{1} ({\mathrm {\mathbf r}}_{1} -n{\mathrm {\mathbf a}}) \end{array} $$

(142)

$$\begin{array}{@{}rcl@{}} \phi_{2} ({\mathrm {\mathbf r}})&=&\frac{1}{\sqrt N }\sum\limits_{n=0}^{N-1} {e^{jk(n\left| {{\mathrm {\mathbf a}}} \right|+d/2)}} \varphi_{2} [({\mathrm {\mathbf r}}-\frac{{\mathrm {\mathbf b}}}{2})-n{\mathrm {\mathbf a}}]\\&=&\frac{1}{\sqrt N }\sum\limits_{n=0}^{N-1} {e^{jk(n\left| {{\mathrm {\mathbf a}}} \right|+d/2)}} \varphi_{2} ({\mathrm {\mathbf r}}_{2} -n{\mathrm {\mathbf a}}) \end{array} $$

(143)

Fig. 8

A segment of trans-polythene as a model for a one-dimensional crystal. b is the vector between the two atoms indicated. There are two sets of carbon atoms indicated by 1 and 2. In each set, the atoms are separated by |a|

The origin for r is at the middle of a double bond. A crystal-adapted orbital is an orbital conforming to Bloch’s theorem. Crystal orbitals ψ are obtained from crystal-adapted orbitals [45].

$$ \psi ({\mathrm {\mathbf r}})=\frac{1}{\sqrt 2 }[c_{1} \phi_{1} ({\mathrm {\mathbf r}})+c_{2} \phi_{1} ({\mathrm {\mathbf r}})] $$

(144)

$$ \hat{H}\psi ({\mathrm {\mathbf r}})=E_{C} \psi ({\mathrm {\mathbf r}}) $$

(145)

The energy of the crystal orbitals can be obtained by inserting (144) into (145).

$$ \left| {{\begin{array}{*{20}c} {H_{11} -E_{C} S_{11} } & \;\;{H_{12} -E_{C} S_{12} } \\ {H_{21} -E_{C} S_{21} } & \;\;{H_{22} -E_{C} S_{22} } \\ \end{array} }} \right|=0 $$

(146)

The evaluation can be simplified by the following assumptions with the same principle from (140).

$$\begin{array}{@{}rcl@{}} S_{11} &=&\displaystyle\int\limits_V {\phi_{1}^{\ast } } ({\mathrm {\mathbf r}})\phi_{1} ({\mathrm {\mathbf r}})d\tau =\frac{1}{N}\sum\limits_{n^{\prime} ,n} e^{jk(n-n^{\prime} )\left| {{\mathrm {\mathbf a}}} \right|}\\ &&{\kern-2.5pc} \displaystyle\int\limits_V {\varphi_{1}^{\ast } } ({\mathrm {\mathbf r}}+\frac{{\mathrm {\mathbf b}}}{2}-n^{\prime} {\mathrm {\mathbf a}})\varphi_{1} ({\mathrm {\mathbf r}}+\frac{{\mathrm {\mathbf b}}}{2}-n{\mathbf{a}})d\tau =1=S_{22} \end{array} $$

(147)

$$\begin{array}{@{}rcl@{}} S_{12} &=&\frac{1}{N}e^{jkd}\sum\limits_{n^{\prime} ,n} e^{jk(n-n^{\prime} )\left| {{\mathrm {\mathbf a}}} \right|}\\ &&{\kern-2.5pc} \int\limits_V {\varphi_{1}^{\ast } } ({\mathrm {\mathbf r}}+\frac{{\mathrm {\mathbf b}}}{2}-n^{\prime} {\mathrm {\mathbf a}})\varphi_{2} ({\mathrm {\mathbf r}}-\frac{{\mathrm {\mathbf b}}}{2}-n{\mathrm {\mathbf a}})d\tau =0=S_{21}^{\ast} \end{array} $$

(148)

$$\begin{array}{@{}rcl@{}} H_{11} &=&\displaystyle\int\limits_{V} {\phi_{1}^{\ast } } ({\mathrm {\mathbf r}})\hat{H} \phi_{1} ({\mathbf{r}})d\tau =\frac{1}{N}\sum\limits_{n^{\prime} ,n} e^{jk(n-n^{\prime} )\left| \mathbf{a} \right|}\\ &&{\kern-2.7pc} \displaystyle\int\limits_V {\varphi_{1}^{\ast } } ({\mathrm {\mathbf r}}+\frac{{\mathrm {\mathbf b}}}{2}-n^{\prime} {\mathrm {\mathbf a}})\hat{H} \varphi_{1} ({\mathrm {\mathbf r}}+\frac{{\mathbf{b}}}{2}-n{\mathrm {\mathbf a}})d\tau =\alpha =H_{22}\\ \end{array} $$

(149)

$$\begin{array}{@{}rcl@{}} H_{12} &=&\frac{1}{N}e^{jkd}\sum\limits_{n^{\prime} ,n}e^{jk(n-n^{\prime} )\left| {{\mathrm {\mathbf a}}} \right|}\\ &&{\kern-2.7pc} \displaystyle\int\limits_V {\varphi_{1}^{\ast } } ({\mathrm {\mathbf r}}+\frac{{\mathrm {\mathbf b}}}{2}-n^{\prime} {\mathrm {\mathbf a}})\hat{H} \varphi _{2} ({\mathrm {\mathbf r}}-\frac{{\mathrm {\mathbf b}}}{2}-n{\mathrm {\mathbf a}})d\tau\\ &&{\kern-2.8pc} =e^{jkd}(\beta +\beta^{\prime} e^{-jk\left| {{\mathrm {\mathbf a}}} \right|}) \end{array} $$

(150)

$$\begin{array}{@{}rcl@{}} H_{21}&=&\frac{1}{N}e^{-jkd}\sum\limits_{n^{\prime} ,n} e^{jk(n-n^{\prime} )\left| {{\mathrm {\mathbf a}}} \right|}\\ &&{\kern-2.7pc} \displaystyle\int\limits_V {\varphi_{2}^{\ast } } ({\mathrm {\mathbf r}}-\frac{{\mathrm {\mathbf b}}}{2}-n^{\prime} {\mathrm {\mathbf a}})\hat{H} \varphi_{1} ({\mathrm {\mathbf r}}+\frac{{\mathrm {\mathbf b}}}{2}-n{\mathrm {\mathbf a}})d\tau \\ &&{\kern-2.8pc} =e^{-jkd}(\beta +\beta^{\prime} e^{jk\left| {{\mathrm {\mathbf a}}} \right|})=H_{12}^{\ast } \end{array} $$

(151)

The parameters β and $\beta ^{\prime }$ are specific for the double and single bonds in the polythene, respectively. By inserting (147)–(151) into (146), the energy for the crystal orbital is obtained as shown by (153).

$$ \left|\begin{array}{ll} {\kern2pc} {\alpha -E_{C}} &{e^{jkd}(\beta +\beta^{\prime} e^{-jk\left| {{\mathrm {\mathbf a}}} \right|})} \\ {e^{-jkd}(\beta +\beta^{\prime} e^{jk\left| {{\mathrm {\mathbf a}}} \right|})} & {{\kern2pc} \alpha -E_{C} } \\ \end{array} \right|=0 $$

(152)

$$ E_{C} =\alpha \pm [{\beta}^{2}+{\beta^{\prime}}^{2}+2{\beta\beta}^{\prime}\cos (k\left| {\mathbf{a}}\right|)]^{1/2} $$

(153)

Two energy bands are obtained by varying k from 0 to π/|a|. If all the C–C bond lengths in the polythene are the same, then $\beta = \beta ^{\prime }$ and there will be no energy gap between the bands, thus, the polymer is a conductor. However, if the double and single bonds are distinct with different bond lengths then the upper energy boundary of the lower band will be lowered from α to ($\alpha - | {\beta } -{\beta }^{\prime }|$) while the lower energy boundary for the upper band will be raised from the α to ($\alpha + ~| {\beta } - {\beta }^{\prime } |$). Thus, there is an energy gap of $2|\beta - \beta ^{\prime }|$ between the two bands at k = π/|a| and the polymer is a semiconductor. Note the upper band is vacant while the lower band is filled with electrons for this neutral polymer.