Joint production and repair efficiency planning of a multiple deteriorating system

Abstract

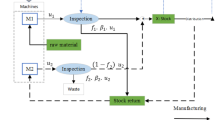
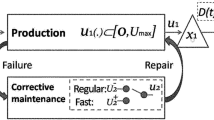
This paper presents an integrated model for the simultaneous production and repair activity planning of a manufacturing system whose performance output is subject to progressive deterioration. In this context, an appropriate joint control strategy is critical to reduce costs and remain competitive. The obtained control policy balances the amount of maintenance activities needed to increase the availability and reduce defects against the increase in the total cost from downtime and deterioration. The production system consists of an unreliable machine that produces one product type and where unmet demand is backlogged. The rate of defects of the machine depends on its level of deterioration, which is defined through a set of multiple operational states and the age of the machine. Additionally, an intensity control model is adapted to define the repair efficiency applied to the system, aiming to mitigate the effect of deterioration that is mainly observed on the failure intensity of the system. The solution is obtained numerically through the formulation of a Hamilton–Jacobi–Bellman equation. A numerical example is provided and an extensive sensitivity analysis is conducted to validate the obtained results.

Appendix: Optimality conditions

The value function $V(\alpha ,x,a)$ defined in Eq. (17) provides the viscosity solution that satisfies the HJB Equations (18). The relevance of Eq. (18) is that it defines the optimality conditions of the model that are necessary and sufficient for an optimum. The HJB equations can be derived by the principle of optimality: if $V(\cdot,t)$ denotes a cost-to-go function at time t, then the value function $V(\alpha ,x,a)$ between t and $t + \delta t$ can be replaced by:

$$V\left( {\alpha \left( 0 \right),x\left( 0 \right),a\left( 0 \right),0} \right) = \mathop {inf}\limits_{{\begin{array}{*{20}c} {u\left( t \right), v\left( t \right)} \\ {0 \le t \le \infty } \\ \end{array} }} E\left\{ {\left. {\begin{array}{*{20}c} {\mathop \smallint \limits_{0}^{t} e^{ - \rho t} g\left( {\left( {\alpha (t} \right),x\left( t \right),a\left( t \right),u\left( t \right), v\left( t \right)} \right)dt} \\ + \\ { \mathop \smallint \limits_{t}^{\infty } e^{ - \rho t} g\left( {\left( {\alpha (t} \right),x\left( t \right),a\left( t \right),u\left( t \right), v\left( t \right)} \right)dt} \\ \end{array} } \right|\alpha \left( 0 \right), x\left( 0 \right), a\left( 0 \right)} \right\}$$

(31)

Since the integral in the interval $\left[ {t,\infty } \right]$ is the value function, we obtain the one-step counterpart of $V\left( {\alpha \left( t \right),x\left( t \right),a\left( t \right),t} \right)$ in the interval $\left[ {t,t + \delta t} \right]$ for transforming the discount rate $\rho$.

$$V\left( {\alpha \left( t \right),x\left( t \right),a\left( t \right),t} \right) = \mathop {inf}\limits_{{\begin{array}{*{20}c} {u\left( s \right), v\left( s \right)} \\ {t \le s \le t + \delta t} \\ \end{array} }} E\left\{ {\left. {\begin{array}{*{20}c} {\mathop \smallint \limits_{t}^{t + \delta t} e^{ - \rho t} g\left( {\left( {\alpha (t} \right),x\left( t \right),a\left( t \right),u\left( t \right), v\left( t \right)} \right)ds} \\ + \\ { \frac{1}{1 + \rho \delta t}V\left( {\alpha \left( {t + \delta t} \right),x\left( {t + \delta t} \right),a\left( {t + \delta t} \right),t + \delta t} \right)} \\ \end{array} } \right|\alpha \left( t \right), x\left( t \right), a\left( t \right)} \right\}$$

(32)

The variables $u\left( s \right)$ and $v\left( s \right)$ are treated as constants in the interval $t \le s \le t + \delta t$. We can further reduce Eq. (32) if we use the conditional expectation operator $\tilde{E}$. For instance, for any function $H\left( \alpha \right)$, the operator $\tilde{E}$ is defined as follows:

$$\tilde{E}\left\{ {H\left( {\alpha \left( {t + \delta t} \right)} \right)} \right\} = E\left\{ {H\left( {\alpha \left( {t + \delta t} \right)} \right) |\alpha \left( t \right)} \right\}$$

(33)

Therefore, upon using the conditional expectation operation $\tilde{E}$ we obtain Eq. (34):

$$\rho V\left( {\alpha \left( t \right),x\left( t \right),a\left( t \right),t} \right) = \mathop {inf}\limits_{u\left( t \right), v\left( t \right)} \tilde{E} \left\{ {\begin{array}{*{20}c} {g\left( {\left( {\alpha (t} \right),x\left( t \right),a\left( t \right),u\left( t \right), v\left( t \right)} \right)\delta t + } \\ + \\ {\frac{{V\left( {\alpha \left( {t + \delta t} \right),x\left( {t + \delta t} \right),a\left( {t + \delta t} \right),t + \delta t} \right) - V\left( {\alpha \left( t \right),x\left( t \right),a\left( t \right),t} \right)}}{\delta t}} \\ \end{array} } \right\} + o\left( {\delta t} \right)$$

(34)

We can expand the conditional expectation in Eq. (34), with the following expression:

$$\tilde{E}\,H\left( {\alpha \left( {t + \delta t} \right)} \right) = H\left( {\alpha \left( t \right)} \right) + \mathop \sum \limits_{j} H\left( j \right)\lambda_{{j\alpha \left( {\text{t}} \right)}} \delta t + o\left( {\delta t} \right)$$

(35)

The second term in Eq. (34) denotes the derivative of $V\left( {\alpha ,x,a} \right)$, so its full derivative can be applied. Thus, after some transformations we get:

$$\rho V\left( {\alpha \left( t \right),x\left( t \right),a\left( t \right),t } \right) = \mathop {inf}\limits_{u\left( t \right), v\left( t \right)} \left\{ {\begin{array}{*{20}c} {g\left( {\left( {\alpha (t} \right),x\left( t \right),a\left( t \right),u\left( t \right), v\left( t \right)} \right)\delta t + } \\ {\frac{\partial V}{\partial x}\left( {\alpha \left( t \right),x\left( t \right),a\left( t \right),t} \right)\delta x\left( t \right) + \frac{\partial V}{\partial a}\left( {\alpha \left( t \right),x\left( t \right),a\left( t \right),t} \right)\delta a\left( t \right)} \\ {\frac{\partial V}{\partial t}\left( {\alpha \left( t \right),x\left( t \right),a\left( t \right),t} \right)\delta t + \mathop \sum \limits_{\alpha '} \lambda_{{\alpha \alpha^{ '} }} V\left( {\alpha ',x\left( t \right),a\left( t \right),t} \right)\delta t} \\ \end{array} } \right\} + o\left( {\delta t} \right)$$

(36)

In Eq. (36) the expectation symbol is replaced by the summation term. Moreover, we can replace $\delta x\left( t \right)$ by $\delta x\left( t \right) = \dot{x}\left( t \right)\delta t$ and $\delta a\left( t \right)$ by $\delta a\left( t \right) = \dot{a}\left( t \right)\delta t$, so that after some standard transformations we have:

$$\rho V\left( {\alpha ,x,a,t} \right) - \frac{\partial }{\partial t}V\left( {\alpha ,x,a,t} \right) = \mathop {min}\limits_{u\left( t \right), v\left( t \right)} \left\{ {g\left( {\alpha ,x,a,u, v} \right) + \dot{x}\frac{\partial }{\partial x}V\left( {\alpha ,x,a,t} \right) + \dot{a}\frac{\partial }{\partial a}V\left( {\alpha ,x,a,t} \right) + \mathop \sum \limits_{{\alpha^{\prime}}} \lambda_{{\alpha \alpha^{\prime}}} \left( \cdot \right)V\left( {\alpha^{{\prime }} ,x,a,t} \right)} \right\}$$

(37)

By considering that a steady-state distribution exists for α and that $V\left( {\alpha ,x,a,t} \right) \to V\left( {\alpha ,x,a} \right)$ and $\partial /\partial t \to 0$ as $t \to \infty$, we finally obtain the so-called Hamilton–Jacobi–Bellman (HJB) equations:

$$\rho V\left( {\alpha ,x,a} \right) = \mathop {\hbox{min} }\limits_{{\left( {u, v} \right) \in \varGamma \left( \alpha \right)}} \left\{ {g\left( {\alpha ,x,a,u, v} \right) + \dot{x}\frac{\partial }{\partial x}V\left( {\alpha ,x,a} \right) + \dot{a}\frac{\partial }{\partial a}V\left( {\alpha ,x,a} \right) + \mathop \sum \limits_{{\alpha^{{\prime }} }} \lambda_{{\alpha \alpha^{{\prime }} }} \left( \cdot \right)V\left( {\alpha ,x,\varphi \left( {\xi ,a} \right)} \right)\left( \alpha \right)} \right\}$$

(38)

Equation (38) can be further simplified:

$$\rho V\left( {\alpha ,x,a} \right) = \left\{ {\begin{array}{*{20}l} {\mathop {min}\limits_{v \in \varGamma \left( \alpha \right)} \left[ {g\left( \cdot \right) + \dot{x}\frac{\partial }{\partial x}V\left( \cdot \right) + \mathop \sum \limits_{{\alpha^{{\prime }} }} \lambda_{{\alpha \alpha^{{\prime }} }} \left( \cdot \right)V\left( {\alpha ,x,\varphi \left( {\xi ,a} \right)} \right)\left( \alpha \right)} \right],} \hfill & {for\;\left( {\alpha = r} \right)} \hfill \\ {\mathop {min}\limits_{u \in \varGamma \left( \alpha \right)} \left[ {g\left( \cdot \right) + \dot{x}\frac{\partial }{\partial x}V\left( \cdot \right) + \dot{a}\frac{\partial }{\partial a}V\left( \cdot \right) + \mathop \sum \limits_{{\alpha^{{\prime }} }} \lambda_{{\alpha \alpha^{{\prime }} }} \left( \cdot \right)V\left( {\alpha ,x,\varphi \left( {\xi ,a} \right)} \right)\left( \alpha \right)} \right], } \hfill & {for\;\left( {\alpha = 1, \ldots ,n} \right)} \hfill \\ \end{array} } \right.$$

(39)

The minimization of $u\left( t \right)$ is performed in the $n$ operational states, the repair efficiency is determined in the failure mode and $\varphi \left( {\xi ,a} \right)$ denotes the reset function that describes the benefit of the repair.

$$\varphi \left( {\xi ,a} \right) = \left\{ {\begin{array}{*{20}c} {\begin{array}{*{20}l} {v \cdot a\left( {\sigma^{ - } } \right)} \\ {a\left( {\sigma^{ - } } \right)} \\ \end{array} } & {\begin{array}{*{20}c} {if\; \xi \left( {\sigma^{ + } } \right) = 1\quad and\quad \xi \left( {\sigma^{ - } } \right) = r} \\ {otherwise} \\ \end{array} } \\ \end{array} } \right.$$

(40)

The control polices obtained from Eq. (39) are optimal, since the HJB Equations are a sufficient condition for optimality, as indicated by Gershwin (2002) and Sethi and Thompson (2000).