Skip to main content
SpringerLink
Log in

Stability behavior of rotating axially moving conical shell made of shape memory alloy

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

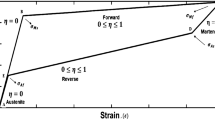
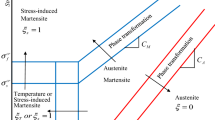
The current study investigates the nonlinear vibration characteristic of rotating axially moving conical shell made of shape memory alloy (SMA). For this purpose, the material behavior of SMA is simulated via Boyd-Lagoudas and Brinson models, and three nonlinear governing equations are derived by employing Hamilton principle, Donnell’s nonlinear theory assumptions, and SMA constitutive relations. By applying a suitable parametric airy stress function, three nonlinear equations of motion are reduced to one in radial direction, which must be solved with the help of the compatibility equation. By the aid of Jordan conical form and applying the Galerkin method on the equilibrium equation in the radial direction, seven nonlinear nonhomogeneous ODEs are resulted. Then, the set of nonlinear equations is solved using the fourth-order Runge–Kutta method and pseudo-arc length continuation. Furthermore, the bifurcation analysis based on the different parameters especially frequency responses along with the curves of the time histories and phase portraits mention the influence of different phases of the material, axial motion and spinning on the conical shells made of SMA. The results of the present work are validated with available approved data, which shows good agreements.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14

Similar content being viewed by others

References

  1. Nekouei, M., Raghebi, M., Mohammadi, M.: Free vibration analysis of laminated composite conical shells reinforced with shape memory alloy fibers. Acta Mech. 230(12), 4235–4255 (2019)

    MathSciNet  MATH  Google Scholar 

  2. Lagoudas, D.C.: Shape Memory Alloys: Modeling and Engineering Applications. Springer, Cham (2008)

    MATH  Google Scholar 

  3. Wang, X.-M., Wang, Z.-L., Xiao, H.: SMA pseudo-elastic hysteresis with tension–compression asymmetry: explicit simulation based on elastoplasticity models. Contin. Mech. Thermodyn. 27, 959–970 (2015)

    MathSciNet  MATH  Google Scholar 

  4. Brinson, L.C.: One-dimensional constitutive behavior of shape memory alloys: thermomechanical derivation with non-constant material functions and redefined martensite internal variable. J. Intell. Mater. Syst. Structur. 4(2), 229–242 (1993)

    Google Scholar 

  5. Tanaka, K.: A thermomechanical sketch of shape memory effect. One-dimens. Tensile Behav. 18, 251 (1986)

    Google Scholar 

  6. Tanaka, K., Kobayashi, S., Sato, Y.: Thermomechanics of transformation pseudoelasticity and shape memory effect in alloys. Int. J. Plast. 2(1), 59–72 (1986)

    Google Scholar 

  7. Liang, C., Rogers, C.A.: One-dimensional thermomechanical constitutive relations for shape memory materials. J. Intell. Mater. Syst. Struct. 8(4), 285–302 (1997)

    Google Scholar 

  8. Achenbach, M.: A model for an alloy with shape memory. Int. J. Plast. 5(4), 371–395 (1989)

    Google Scholar 

  9. Ivshin, Y., Pence, T.J.: A thermomechanical model for a one variant shape memory material. J. Intell. Mater. Syst. Struct. 5(4), 455–473 (1994)

    Google Scholar 

  10. Bekker, A., Brinson, L.: Phase diagram based description of the hysteresis behavior of shape memory alloys. Acta Mater. 46(10), 3649–3665 (1998)

    Google Scholar 

  11. Bekker, A., Brinson, L.C.: Temperature-induced phase transformation in a shape memory alloy: phase diagram based kinetics approach. J. Mech. Phys. Solids 45(6), 949–988 (1997)

    MathSciNet  MATH  Google Scholar 

  12. Leclercq, S., Lexcellent, C.: A general macroscopic description of the thermomechanical behavior of shape memory alloys. J. Mech. Phys. Solids 44(6), 953–980 (1996)

    Google Scholar 

  13. Helm, D., Haupt, P.: Shape memory behaviour: modelling within continuum thermomechanics. Int. J. Solids Struct. 40(4), 827–849 (2003)

    MATH  Google Scholar 

  14. Huo, Y., Müller, I.: Nonequilibrium thermodynamics of pseudoelasticity. Contin. Mech. Thermodyn. 5, 163–204 (1993)

    MathSciNet  MATH  Google Scholar 

  15. Civalek, Ö.: An efficient method for free vibration analysis of rotating truncated conical shells. Int. J. Press. Vessels Pip. 83(1), 1–12 (2006)

    Google Scholar 

  16. Chen, C., Dai, L.: Nonlinear vibration and stability of a rotary truncated conical shell with intercoupling of high and low order modals. Commun. Nonlinear Sci. Numer. Simul. 14(1), 254–269 (2009)

    Google Scholar 

  17. Sofiyev, A.: The non-linear vibration of FGM truncated conical shells. Compos. Struct. 94(7), 2237–2245 (2012)

    MathSciNet  Google Scholar 

  18. Sofiyev, A.: On the vibration and stability behaviors of heterogeneous-CNTRC-truncated conical shells under axial load in the context of FSDT. Thin-Walled Struct. 151, 106747 (2020)

    Google Scholar 

  19. Hua, L.: Influence of boundary conditions on the free vibrations of rotating truncated circular multi-layered conical shells. Compos. Part B Eng. 31(4), 265–275 (2000)

    Google Scholar 

  20. Hua, L.: Frequency characteristics of a rotating truncated circular layered conical shell. Compos. Struct. 50(1), 59–68 (2000)

    Google Scholar 

  21. Lam, K., Hua, L.: Influence of boundary conditions on the frequency characteristics of a rotating truncated circular conical shell. J. Sound Vib. 223(2), 171–195 (1999)

    MATH  Google Scholar 

  22. Abolhassanpour, H., et al.: Stability and vibration analysis of an axially moving thin walled conical shell. J. Vib. Control 28, 7600 (2021). https://doi.org/10.1177/1077546321997600

    Article  MathSciNet  Google Scholar 

  23. Vahidi, H., et al.: Nonlinear vibration, stability, and bifurcation of rotating axially moving conical shells. Acta Mech. 233, 1–22 (2022)

    MathSciNet  Google Scholar 

  24. Sarkheil, S., Foumani, M.S.: An improvement to motion equations of rotating truncated conical shells. Eur. J. Mech. A Solids 62, 110–120 (2017)

    MathSciNet  MATH  Google Scholar 

  25. Sofiyev, A., Pancar, E.: The effect of heterogeneity on the parametric instability of axially excited orthotropic conical shells. Thin-Walled Struct. 115, 240–246 (2017)

    Google Scholar 

  26. Najafov, A., Sofiyev, A., Kuruoglu, N.: Vibration analysis of nonhomogeneous orthotropic cylindrical shells including combined effect of shear deformation and rotary inertia. Meccanica 49(10), 2491–2502 (2014)

    MathSciNet  MATH  Google Scholar 

  27. Anh, V.T.T., Duc, N.D.: Vibration and nonlinear dynamic response of eccentrically stiffened functionally graded composite truncated conical shells surrounded by an elastic medium in thermal environments. Acta Mech. 230(1), 157–178 (2019)

    MathSciNet  MATH  Google Scholar 

  28. Kerboua, Y., Lakis, A., Hmila, M.: Vibration analysis of truncated conical shells subjected to flowing fluid. Appl. Math. Model. 34(3), 791–809 (2010)

    MathSciNet  MATH  Google Scholar 

  29. Shahgholi, M., et al.: Stability analysis of an axially moving thin wall conical shells made of shape memory alloy. Iran. J. Mech. Eng. Trans. ISME 23, 105–128 (2022)

    Google Scholar 

  30. Vahidi, H., et al.: Stability analysis of an axially moving thin wall conical shells made of shape memory alloy. Iran. J. Mech. Eng. Trans. ISME 23(2), 105–128 (2022)

    MathSciNet  Google Scholar 

  31. Chen, Y., et al.: Vibrations of high speed rotating shells with calculations for cylindrical shells. J. Sound Vib. 160(1), 137–160 (1993)

    MATH  Google Scholar 

  32. Mohamadi, A., Shahgholi, M., Ghasemi, F.A.: Free vibration and stability of an axially moving thin circular cylindrical shell using multiple scales method. Meccanica 54(14), 2227–2246 (2019)

    MathSciNet  Google Scholar 

  33. Wang, Y., Ding, H., Chen, L.-Q.: Nonlinear vibration of axially accelerating hyperelastic beams. Int. J. Nonlinear Mech. 99, 302–310 (2018)

    Google Scholar 

  34. Vahidi, H., Hanzaki, A.R., Shahgholi, M.: Free vibration and stability study of an axially rotating circular cylindrical shell made of shape memory alloy. Iran. J. Sci. Technol. Trans. Mech. Eng. 47, 1–20 (2022)

    Google Scholar 

  35. He, C.-H., et al.: Controlling the kinematics of a spring-pendulum system using an energy harvesting device. J. Low Freq. Noise Vib. Act. Control 41(3), 1234–1257 (2022)

    MathSciNet  Google Scholar 

  36. Safaei, B., et al.: Free vibration investigation on RVE of proposed honeycomb sandwich beam and material selection optimization. Facta Univ. Ser. Mech. Eng. 21(1), 031–050 (2023)

    Google Scholar 

  37. Chen, Y., et al.: Multi-stability of the hexagonal origami hypar based on group theory and symmetry breaking. Int. J. Mech. Sci. 247, 108196 (2023)

    Google Scholar 

  38. Chen, Y., et al.: Data-driven design and morphological analysis of conical six-fold origami structures. Thin-Walled Struct. 185, 110626 (2023)

    Google Scholar 

  39. He, J.-H., et al.: Stability of three degrees-of-freedom auto-parametric system. Alex. Eng. J. 61(11), 8393–8415 (2022)

    Google Scholar 

  40. Xu, L., et al.: A three-dimensional constitutive modeling for shape memory alloys considering two-way shape memory effect and transformation-induced plasticity. In: AIAA Scitech 2019 Forum. Scitech, San Diego (2019)

    Google Scholar 

  41. Brinson, L., Lammering, R.: Finite element analysis of the behavior of shape memory alloys and their applications. Int. J. Solids Struct. 30(23), 3261–3280 (1993)

    MATH  Google Scholar 

  42. Sadd, M.H.: Elasticity: Theory, Applications, and Numerics. Academic Press, Cambridge (2009)

    Google Scholar 

  43. Amabili, M.: Nonlinear Vibrations and Stability of Shells and Plates. Cambridge University Press, Cambridge (2008)

    MATH  Google Scholar 

  44. Sofiyev, A., Kuruoglu, N.: Non-linear buckling of an FGM truncated conical shell surrounded by an elastic medium. Int. J. Press. Vessels Pip. 107, 38–49 (2013)

    Google Scholar 

  45. He, J.-H.: Generalized variational principles for buckling analysis of circular cylinders. Acta Mech. 231(3), 899–906 (2020)

    MathSciNet  MATH  Google Scholar 

  46. He, J.-H.: Generalized equilibrium equations for shell derived from a generalized variational principle. Appl. Math. Lett. 64, 94–100 (2017)

    MathSciNet  MATH  Google Scholar 

  47. Reddy, J.N.: Mechanics of Laminated Composite Plates and Shells: Theory and Analysis. CRC Press, Boca Raton (2003)

    Google Scholar 

  48. Mohamadi, A., Shahgholi, M., Ghasemi, F.A.: Nonlinear vibration of axially moving simply-supported circular cylindrical shell. Thin-Walled Struct. 156, 107026 (2020)

    Google Scholar 

  49. Sofiyev, A.: Non-linear buckling behavior of FGM truncated conical shells subjected to axial load. Int. J. Nonlinear Mech. 46(5), 711–719 (2011)

    MathSciNet  Google Scholar 

  50. Soedel, W.: Vibrations of Shells and Plates. CRC Press, Boca Raton (2004)

    MATH  Google Scholar 

  51. Abolhassanpour, H., et al.: Nonlinear vibration analysis of an axially moving thin-walled conical shell. Int. J. Nonlinear Mech. 134, 103747 (2021)

    Google Scholar 

  52. Qu, Y., et al.: A variational method for free vibration analysis of joined cylindrical-conical shells. J. Vib. Control 19(16), 2319–2334 (2013)

    MathSciNet  MATH  Google Scholar 

  53. He, J.-H., et al.: Pull-down instability of the quadratic nonlinear oscillators. Facta Univ. Ser. Mech. Eng. 45, 3456 (2023). https://doi.org/10.22190/FUME230114007H

    Article  Google Scholar 

  54. He, J.-H., Moatimid, G.M., Zekry, M.H.: Forced nonlinear oscillator in a fractal space. Facta Univ. Ser. Mech. Eng. 20(1), 001–020 (2022)

    Google Scholar 

  55. Mohamadi, A., Ashenai Ghasemi, F., Shahgholi, M.: Nonlinear vibration, stability, and bifurcation analysis of axially moving and spinning cylindrical shells. Mech. Based Des. Struct. Mach. 51, 1–31 (2021)

    Google Scholar 

Download references

Funding

This research received no specific grant from any funding agency in the public, commercial, or not-for-profit sectors.

Author information

Authors and Affiliations

  1. Faculty of Mechanical Engineering, Shahid Rajaee Teacher Training University, Tehran, Iran

    Hadi Vahidi, Ali Rahmani Hanzaki, Majid Shahgholi & Arash Mohamadi

Authors
  1. Hadi Vahidi
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ali Rahmani Hanzaki
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Majid Shahgholi
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Arash Mohamadi
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Ali Rahmani Hanzaki.

Ethics declarations

Conflit of interest

The authors declare that they have no known competing financial interests or personal relationship that could have appeared to influence the work reported in this paper.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Appendix A

In this portion, the relation for equation of motion which is mentioned in Sect. 3 is presented.

$$L_{11} = S\frac{{e^{x} }}{\cot \left( \alpha \right)}\left( {\frac{{\partial^{2} }}{{\partial x^{2} }} + 3\frac{\partial }{\partial x} + 2} \right) - \cot \left( \alpha \right) \times \int gd\varphi$$
(A1)
$$\begin{gathered} L_{12} = \frac{1}{{e^{2x} }}\left( { - A3\left( {\frac{{\partial^{4} }}{{\partial \varphi^{4} }} + 2\frac{{\partial^{2} }}{{\partial \varphi^{2} }} + \frac{{\partial^{4} }}{{\partial x^{4} }} - 4\frac{{\partial^{3} }}{{\partial x^{3} }} + 4\frac{{\partial^{2} }}{{\partial x^{2} }}} \right) - 2\left( {A4 + A6} \right)\left( {\frac{{\partial^{4} }}{{\partial x^{2} \partial \varphi^{2} }} - 2\frac{{\partial^{3} }}{{\partial x\partial \varphi^{2} }} + \frac{{\partial^{2} }}{{\partial \varphi^{2} }}} \right)} \right. \hfill \\ \left. {\quad \quad - S^{4} e^{4x} \left( {\rho h\left( {\frac{{\partial^{2} }}{{\partial t^{2} }} + \frac{{2V_{c} \left( t \right)}}{{Se^{x} }}\frac{\partial }{\partial t} + \frac{{V_{c} \left( t \right)^{2} }}{{S^{2} e^{2x} }}\frac{{\partial^{2} }}{{\partial x^{2} }}} \right)} \right) + C\left( {\frac{\partial }{\partial t} + \frac{{V_{c} \left( t \right)}}{{Se^{x} }}\frac{\partial }{\partial t}} \right)} \right) \hfill \\ \quad \quad + \int gd\varphi \times (\frac{1}{{se^{x} }}\left( {\frac{{\partial^{2} }}{{\partial \varphi^{2} }} + \frac{\partial }{\partial x}} \right) + \rho h\omega_{r}^{2} \left( {se^{x} \frac{\partial }{\partial x}\cos \left( \alpha \right)\cot \left( \alpha \right) + \frac{{\partial^{2} }}{{\partial \varphi^{2} }} + \cos \left( \alpha \right)^{2} } \right) \hfill \\ \end{gathered}$$
(A2)
$$L_{13} = e^{2x} (\left( {\frac{{\partial^{2} }}{{\partial \varphi^{2} }} + \frac{\partial }{\partial x} + 2} \right)\left( {\frac{{\partial^{2} }}{{\partial x^{2} }} - \frac{\partial }{\partial x}} \right) + \left( {\frac{{\partial^{2} }}{{\partial x^{2} }} + 3\frac{\partial }{\partial x} + 2} \right)\left( {\frac{{\partial^{2} }}{{\partial \varphi^{2} }} + \frac{\partial }{\partial x}} \right) - 2\left( {\frac{{\partial^{2} }}{\partial x\partial \varphi } + \frac{\partial }{\partial \varphi }} \right)\left( {\frac{{\partial^{2} }}{\partial x\partial \varphi } - \frac{\partial }{\partial \varphi }} \right)$$
(A3)
$$\begin{gathered} L_{21} = B1.e^{2x} \frac{{\partial^{4} }}{{\partial \varphi^{4} }} + 2\left( {\frac{1 + \mu }{{Eh}} - \frac{\mu }{Eh}} \right)e^{2x} \frac{{\partial^{4} }}{{\partial x^{2} \partial \varphi^{2} }} + 4\left( {\frac{1 + \mu }{{Eh}} - \frac{\mu }{Eh}} \right)e^{2x} \frac{{\partial^{3} }}{{\partial x\partial \varphi^{2} }} \hfill \\ \quad \quad + 2\left( {\frac{1 + \mu }{{Eh}} - \frac{\mu }{Eh} + \frac{1}{Eh}} \right)e^{2x} \frac{{\partial^{2} }}{{\partial \varphi^{2} }} + B1.e^{2x} \frac{{\partial^{4} }}{{\partial x^{4} }} + 4B1.e^{2x} \frac{{\partial^{3} }}{{\partial x^{3} }} + 4B1.e^{2x} \frac{{\partial^{2} }}{{\partial x^{2} }} \hfill \\ \end{gathered}$$
(A4)
$$L_{22} = - B4\frac{{\partial^{4} }}{{\partial \varphi^{4} }} - \left( {\frac{{Se^{x} }}{\cot \left( \alpha \right)}} \right)\frac{{\partial^{2} }}{{\partial x^{2} }}$$
(A5)
$$L_{23} = - \left( {\frac{\partial }{\partial \varphi }} \right)^{2} + 2\left( {\frac{\partial }{\partial \varphi }} \right)\left( {\frac{{\partial^{2} }}{\partial x\partial \varphi }} \right) - \left( {\frac{\partial }{\partial x} - \frac{{\partial^{2} }}{{\partial x^{2} }}} \right)\left( {\frac{{\partial^{2} }}{{\partial \varphi^{2} }}} \right) - \left( {\frac{{\partial^{2} }}{\partial x\partial \varphi }} \right)^{2} - \left( {\frac{\partial }{\partial x} - \frac{{\partial^{2} }}{{\partial x^{2} }}} \right)\left( {\frac{\partial }{\partial x}} \right)$$
(A6)

Appendix B

$$\begin{aligned} & A_{29} \left( {\left( {A_{1,n} } \right)} \right)^{3} + A_{37} \left( {\left( {A_{1,n} } \right)} \right)^{2} A_{2,n} + \left( {A_{48} \left( {\left( {A_{2,n} } \right)} \right)^{2} + A_{60} \left( {\left( {A_{1,n} } \right)} \right)^{2} + \left( {A_{5,0} A_{90} + A_{3,0} A_{94} + A_{93} } \right)} \right.A_{10} \hfill \\ &\quad + A_{66} \left( {\left( {A_{3,0} } \right)} \right)^{2} + \left( {A_{5,0} A_{97} + A_{3,0} A_{94} + A_{96} } \right)A_{30} + A_{42} \left( {\left( {B_{1,n} } \right)} \right)^{2} + A_{54} \left( {\left( {B_{2,n} } \right)} \right)^{2} + A_{72} \left( {\left( {A_{5,0} } \right)} \right)^{2} \hfill \\ &\quad \left. { + A_{85} B_{1,n} B_{2,n} + \left( {A_{5,0} A_{98} } \right) + A_{1,5} } \right)A_{1,n} + A_{31} \left( {\left( {A_{2,n} } \right)} \right)^{3} + \left( {A_{62} \left( {\left( {A_{1,0} } \right)} \right)^{2} } \right. \hfill \\ &\quad + \left( {A_{109} \left( {A_{3,0} } \right) + A_{110} \left( {A_{5,0} } \right) + A_{108} } \right)A_{1,0} + A_{68} \left( {\left( {A_{3,0} } \right)} \right)^{2} + A_{3,0} \left( {A_{112} A_{5,0} + A_{111} } \right) + A_{43} \left( {\left( {B_{1,n} } \right)} \right)^{2} \hfill \\ &\quad \left. { + A_{56} \left( {\left( {B_{2,n} } \right)} \right)^{2} + A_{74} \left( {\left( {A_{5,0} } \right)} \right)^{2} + \left( {A_{100} B_{1,n} B_{2,n} } \right) + A_{113} \left( {A_{5,0} } \right) + A_{17} } \right)A_{2,n} - F\cos \left( {\omega t} \right) \hfill \\ \end{aligned}$$
(B1)
$$\begin{aligned} & B_{2} \frac{{d^{2} }}{{dt^{2} }}\left( {B_{1,n} } \right) + B_{4} \frac{{d^{2} }}{{dt^{2} }}\left( {B_{2,n} } \right) + B_{9} \frac{d}{dt}\left( {B_{1,n} } \right) + B_{11} \frac{d}{dt}\left( {B_{2,n} } \right) + B_{6} B_{1,n} \hfill \\ &\quad + B_{18} B_{2,n} + B_{30} \left( {\left( {B_{1,n} } \right)} \right)^{3} + B_{32} \left( {\left( {B_{2,n} } \right)} \right)^{3} + \left( {\left( {A_{1,n} } \right)} \right)^{2} \left( {B_{36} \left( {B_{1,n} } \right) + B_{38} \left( {B_{2,n} } \right)} \right) \hfill \\ &\quad + B_{44} \left( {\left( {B_{1,n} } \right)} \right)^{2} B_{2,n} + B_{49} \left( {\left( {A_{2,n} } \right)} \right)^{2} B_{1,n} + B_{50} \left( {\left( {A_{2,n} } \right)} \right)^{2} B_{2,n} + B_{55} \left( {\left( {B_{2,n} } \right)} \right)^{2} B_{1,n} \hfill \\ &\quad + A_{1,0}^{2} \left( {B_{61} B_{1,n} + B_{63} B_{2,n} } \right) + A_{3,0}^{2} \left( {B_{67} B_{1,n} + B_{69} B_{2,n} } \right) + A_{5,0}^{2} \left( {B_{73} B_{1,n} + B_{75} B_{2,n} } \right) \hfill \\ &\quad + A_{1,n} A_{2,n} \left( {B_{79} B_{1,n} + B_{80} B_{2,n} } \right) + B_{1,n} A_{1,0} \left( {B_{119} A_{3,0} + B_{118} } \right) \hfill \\ &\quad + \left( {A_{5,0} B_{120} A_{1,0} + A_{3,0} B_{122} + B_{123} } \right)A_{5,0} + A_{3,0} B_{121} )B_{1,n} \hfill \\ &\quad + B_{2,n} A_{1,0} \left( {A_{3,0} B_{125} + A_{5,0} B_{126} + B_{124} } \right) + B_{2,n} \left( {A_{5,0} B_{128} + B_{127} } \right)A_{3,0} + A_{5,0} B_{129} \hfill \\ \end{aligned}$$
(B2)
$$\begin{aligned} & E_{29} \left( {\left( {A_{1,n} } \right)} \right)^{3} + E_{37} \left( {\left( {A_{1,n} } \right)} \right)^{2} A_{2,n} \hfill \\ &\quad + \left( {E_{48} \left( {\left( {A_{2,n} } \right)} \right)^{2} + E_{60} \left( {\left( {A_{1,n} } \right)} \right)^{2} + (A_{5,0} E_{95} + A_{3,0} E_{94} + E_{93} } \right)A_{10} \hfill \\ &\quad + E_{66} \left( {\left( {A_{3,0} } \right)\left( t \right)} \right)^{2} + \left( {A_{5,0} E_{97} + E_{96} } \right)A_{30} + E_{42} \left( {\left( {B_{1,n} } \right)} \right)^{2} + E_{56} \left( {\left( {B_{2,n} } \right)} \right)^{2} \hfill \\ &\quad + E_{72} \left( {\left( {A_{5,0} } \right)} \right)^{2} + E_{85} B_{1,n} \left( t \right)B_{2,n} + \left( {A_{5,0} E_{98} ) + E_{1,5} } \right)A_{1,n} \hfill \\ &\quad + \left( {A_{2,n} } \right)^{2} (E_{31} \left( {\left( {A_{2,n} } \right)} \right)^{2} + (E_{62} \left( {\left( {A_{1,0} } \right)} \right)^{2} + \left( {E_{109} \left( {A_{3,0} } \right) + E_{110} \left( {A_{5,0} } \right)} \right. \hfill \\ &\quad \left. { + E_{108} } \right)A_{1,0} + E_{68} \left( {\left( {A_{3,0} } \right)} \right)^{2} + A_{3,0} \left( {E_{112} A_{5,0} + EA_{111} } \right) + E_{43} \left( {\left( {B_{1,n} } \right)} \right)^{2} \hfill \\ &\quad + E_{56} \left( {\left( {B_{2,n} } \right)} \right)^{2} + E_{74} \left( {\left( {A_{5,0} } \right)} \right)^{2} + \left( {E_{100} B_{1,n} B_{2,n} } \right) + E_{113} \left( {A_{5,0} } \right) + E_{17} )A_{2,n} \hfill \\ \end{aligned}$$
(B3)
$$\begin{aligned} & G_{30} \left( {\left( {B_{1,n} } \right)} \right)^{3} + G_{44} \left( {\left( {B_{1,n} } \right)} \right)^{2} B_{2,n} + B_{49} \left( {\left( {A_{2,n} } \right)} \right)^{2} B_{1,n} + (G_{55} \left( {\left( {B_{2,n} } \right)} \right)^{2} + G_{67} \left( {\left( {A_{3,0} } \right)} \right)^{2} \hfill \\ &\quad + (E_{122} A_{5,0} + G_{119} A_{1,0} + E_{112} )A_{3,0} + G_{73} \left( {\left( {A_{5,0} } \right)} \right)^{2} + (E_{120} A_{1,0} + E_{123} )A_{5,0} + \left( {\left( {A_{1,n} } \right)} \right)^{2} G_{36} + \left( {\left( {A_{2,n} } \right)} \right)^{2} G_{49} \hfill \\ &\quad + A_{1,0}^{2} \left( {G_{61} } \right) + G_{79} \left( {A_{2,n} A_{1,n} } \right) + A_{1,0} \left( t \right)\left( {G_{118} } \right) + G_{16} )B_{1,n} + \left( {\left( {A_{3,0} } \right)} \right)^{2} G_{69} \hfill \\ &\quad + \left( {E_{125} A_{1,0} + E_{128} A_{5,0} + E_{127} } \right)A_{3,0} ) + \left( {\left( {A_{5,0} } \right)} \right)^{2} G_{75} + (E_{126} A_{1,0} + E_{129} )A_{5,0} + G_{38} \left( {\left( {A_{1,n} } \right)} \right)^{2} \hfill \\ &\quad + G_{50} \left( {\left( {A_{2,n} } \right)} \right)^{2} + G_{63} \left( {\left( {A_{1,0} } \right)} \right)^{2} + G_{90} \left( {A_{2,n} A_{1,n} } \right) + E_{124} A_{1,0} + G_{18} )B_{2,n} + E_{126} \left( {A_{3,0} } \right)A_{5,0} \hfill \\ \end{aligned}$$
(B4)
$$\begin{aligned} & K_{33} \left( {\left( {A_{1,0} } \right)} \right)^{3} + (K_{64} \left( {\left( {A_{3,0} } \right)} \right)^{2} + (A_{5,0} K_{65} + A_{3,0} K_{64} + K_{26} ))\left( {\left( {A_{1,0} } \right)} \right)^{2} \hfill \\ &\quad + \left( {K_{70} \left( {\left( {A_{3,0} } \right)} \right)^{2} + \left( {K_{131} A_{5,0} + K_{130} } \right)A_{5,0} + \left( {\left( {A_{1,n} } \right)} \right)^{2} K_{39} + K_{45} \left( {\left( {B_{1,n} } \right)} \right)^{2} } \right. \hfill \\ &\quad \left. { + K_{51} \left( {\left( {A_{2,n} } \right)} \right)^{2} + K_{57} \left( {\left( {B_{1,n} } \right)} \right)^{2} + K_{76} \left( {\left( {A_{5,0} } \right)} \right)^{2} + K_{81} \left( {A_{2,n} A_{1,n} } \right) + K_{115} B_{1,n} \left( t \right)B_{2,n} + \left( {A_{5,0} } \right)K_{132} + K_{19} } \right) \hfill \\ &\quad + A_{1,0} + K_{34} \left( {\left( {A_{3,0} } \right)} \right)^{3} + \left( {(A_{5,0} K_{71} + K_{27} } \right)\left( {\left( {A_{3,0} } \right)} \right)^{2} + (K_{40} \left( {\left( {A_{1,n} } \right)} \right)^{2} + \left( {\left( {B_{1,n} } \right)} \right)^{2} K_{46} + K_{52} \left( {\left( {A_{2,n} } \right)} \right)^{2} \hfill \\ &\quad + K_{58} \left( {\left( {B_{2,n} } \right)} \right)^{2} + \left( {\left( {A_{5,0} } \right)} \right)^{2} K_{77} + K_{82} \left( {A_{2,n} A_{1,n} } \right) + K_{116} \left( {B_{2,n} B_{1,n} } \right) + K_{133} A_{5,0} + K_{20} )A_{3,0} + K_{35} \left( {\left( {A_{3,0} } \right)} \right)^{3} \hfill \\ &\quad + K_{28} \left( {\left( {A_{5,0} } \right)} \right)^{2} + \left( {\left( {\left( {A_{1,n} } \right)} \right)^{2} K_{41} + K_{47} \left( {\left( {B_{1,n} } \right)} \right)^{2} + K_{53} \left( {\left( {A_{2,n} } \right)} \right)^{2} + K_{59} \left( {\left( {B_{2,n} } \right)} \right)^{2} + K_{83} \left( {A_{2,n} A_{1,n} } \right) + K_{21} } \right)\left( {A_{5,0} } \right) \hfill \\ &\quad + \left( {\left( {A_{1,n} } \right)} \right)^{2} K_{22} + \left( {\left( {B_{1,n} } \right)} \right)^{2} K_{23} + K_{24} \left( {\left( {A_{2,n} } \right)} \right)^{2} + \left( {\left( {B_{1,n} } \right)} \right)^{2} K_{25} + K_{79} \left( {A_{2,n} A_{1,n} } \right) + K_{116} \left( {B_{2,n} B_{1,n} } \right) \hfill \\ \end{aligned}$$
(B5)
$$\begin{aligned} & J_{33} \left( {\left( {A_{1,0} } \right)} \right)^{3} + (J_{64} \left( {\left( {A_{3,0} } \right)} \right)^{2} + (A_{5,0} J_{65} + A_{3,0} K_{64} + J_{26} ))\left( {\left( {A_{1,0} } \right)} \right)^{2} \hfill \\ &\quad + \left( {J_{70} \left( {\left( {A_{3,0} } \right)} \right)^{2} + \left( {J_{131} A_{5,0} + J_{130} } \right)A_{5,0} + \left( {\left( {A_{1,n} } \right)} \right)^{2} J_{39} + J_{45} \left( {\left( {B_{1,n} } \right)} \right)^{2} } \right. \hfill \\ &\quad \left. { + J_{51} \left( {\left( {A_{2,n} } \right)} \right)^{2} + J_{57} \left( {\left( {B_{1,n} } \right)} \right)^{2} + J_{76} \left( {\left( {A_{5,0} } \right)} \right)^{2} + J_{81} \left( {A_{2,n} A_{1,n} } \right) + J_{115} B_{1,n} \left( t \right)B_{2,n} + \left( {A_{5,0} } \right)J_{132} + J_{19} } \right)A_{1,0} \hfill \\ &\quad + J_{34} \left( {\left( {A_{3,0} } \right)} \right)^{3} + \left( {(A_{5,0} J_{71} + J_{27} } \right)\left( {\left( {A_{3,0} } \right)} \right)^{2} + (J_{40} \left( {\left( {A_{1,n} } \right)} \right)^{2} + \left( {\left( {B_{1,n} } \right)} \right)^{2} J_{46} + J_{52} \left( {\left( {A_{2,n} } \right)} \right)^{2} \hfill \\ &\quad + J_{58} \left( {\left( {B_{2,n} } \right)} \right)^{2} + \left( {\left( {A_{5,0} } \right)} \right)^{2} J_{77} + J_{82} \left( {A_{2,n} A_{1,n} } \right) + J_{116} \left( {B_{2,n} B_{1,n} } \right) + J_{133} A_{5,0} + J_{20} )A_{3,0} \hfill \\ &\quad + J_{35} \left( {\left( {A_{3,0} } \right)} \right)^{3} + J_{28} \left( {\left( {A_{5,0} } \right)} \right)^{2} + \left( {\left( {\left( {A_{1,n} } \right)} \right)^{2} J_{41} + J_{47} \left( {\left( {B_{1,n} } \right)} \right)^{2} + J_{53} \left( {\left( {A_{2,n} } \right)} \right)^{2} + J_{59} \left( {\left( {B_{2,n} } \right)} \right)^{2} + J_{83} \left( {A_{2,n} A_{1,n} } \right) + J_{21} } \right) \hfill \\ &\quad + \left( {A_{5,0} } \right) + \left( {\left( {A_{1,n} } \right)} \right)^{2} J_{22} + \left( {\left( {B_{1,n} } \right)} \right)^{2} J_{23} + J_{24} \left( {\left( {A_{2,n} } \right)} \right)^{2} + \left( {\left( {B_{1,n} } \right)} \right)^{2} J_{25} + J_{79} \left( {A_{2,n} A_{1,n} } \right) + J_{114} \left( {B_{2,n} B_{1,n} } \right) \hfill \\ \hfill \\ \end{aligned}$$
(B6)
$$\begin{aligned} & K_{33} \left( {\left( {A_{1,0} } \right)} \right)^{3} + (K_{64} \left( {\left( {A_{3,0} } \right)} \right)^{2} + (A_{5,0} K_{65} + A_{3,0} K_{64} + K_{26} ))\left( {\left( {A_{1,0} } \right)} \right)^{2} \hfill \\ &\quad + \left( {K_{70} \left( {\left( {A_{3,0} } \right)} \right)^{2} + \left( {K_{131} A_{5,0} + K_{130} } \right)A_{5,0} + \left( {\left( {A_{1,n} } \right)} \right)^{2} K_{39} + K_{45} \left( {\left( {B_{1,n} } \right)} \right)^{2} + K_{51} \left( {\left( {A_{2,n} } \right)} \right)^{2} } \right. \hfill \\ &\quad \left. { + K_{57} \left( {\left( {B_{1,n} } \right)} \right)^{2} + K_{76} \left( {\left( {A_{5,0} } \right)} \right)^{2} + K_{81} \left( {A_{2,n} A_{1,n} } \right) + K_{115} B_{1,n} \left( t \right)B_{2,n} + \left( {A_{5,0} } \right)K_{132} + K_{19} } \right)A_{1,0} \hfill \\ &\quad + K_{34} \left( {\left( {A_{3,0} } \right)} \right)^{3} + \left( {(A_{5,0} K_{71} + K_{27} } \right)\left( {\left( {A_{3,0} } \right)} \right)^{2} + (K_{40} \left( {\left( {A_{1,n} } \right)} \right)^{2} + \left( {\left( {B_{1,n} } \right)} \right)^{2} K_{46} \hfill \\ &\quad + K_{52} \left( {\left( {A_{2,n} } \right)} \right)^{2} + K_{58} \left( {\left( {B_{2,n} } \right)} \right)^{2} + \left( {\left( {A_{5,0} } \right)} \right)^{2} K_{77} + K_{82} \left( {A_{2,n} A_{1,n} } \right) + K_{116} \left( {B_{2,n} B_{1,n} } \right) \hfill \\ &\quad + K_{133} A_{5,0} + K_{20} )A_{3,0} + K_{35} \left( {\left( {A_{3,0} } \right)} \right)^{3} + K_{28} \left( {\left( {A_{5,0} } \right)} \right)^{2} \hfill \\ &\quad + \left( {\left( {\left( {A_{1,n} } \right)} \right)^{2} K_{41} + K_{47} \left( {\left( {B_{1,n} } \right)} \right)^{2} + K_{53} \left( {\left( {A_{2,n} } \right)} \right)^{2} + K_{59} \left( {\left( {B_{2,n} } \right)} \right)^{2} + K_{83} \left( {A_{2,n} A_{1,n} } \right) + K_{21} } \right)\left( {A_{5,0} } \right) \hfill \\ &\quad + \left( {\left( {A_{1,n} } \right)} \right)^{2} K_{22} + \left( {\left( {B_{1,n} } \right)} \right)^{2} K_{23} + K_{24} \left( {\left( {A_{2,n} } \right)} \right)^{2} + \left( {\left( {B_{1,n} } \right)} \right)^{2} K_{25} + K_{79} \left( {A_{2,n} A_{1,n} } \right) + K_{116} \left( {B_{2,n} B_{1,n} } \right) \hfill \\ \end{aligned}$$
(B7)

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Vahidi, H., Rahmani Hanzaki, A., Shahgholi, M. et al. Stability behavior of rotating axially moving conical shell made of shape memory alloy. Acta Mech 234, 5725–5748 (2023). https://doi.org/10.1007/s00707-023-03674-4

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-023-03674-4

Search

Navigation