Skip to main content
SpringerLink
Log in

Rheological analysis of the general fractional-order viscoelastic model involving the Miller–Ross kernel

  • Original Paper
  • Published:
Acta Mechanica Aims and scope Submit manuscript

Abstract

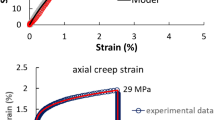
The present work is mainly stimulated by the definition of the general fractional-order derivative operator (GFODO) involving the Miller–Ross kernel in the sense of the Liouville–Sonine type. The novel emphasis is the introduction of the GFODO within the Miller–Ross kernel into the Maxwell model and Kelvin–Voigt model, thereby constructing viscoelastic constitutive models with the property of inheritance and memorability. It is noteworthy that the fractional viscoelasticity can capture the strain response within a wide range of strain rates. The procedure used in our paper to calculate the creep compliance of the proposed model is the Laplace transform, and then comparisons between the general fractional-order models and the classical integer-order models are presented. In summing up, it may be stated that the general fractional-order Kelvin–Voigt model exhibits a very different behavior compared to the classical Kelvin–Voigt model and it can describe the whole creep process including the accelerated creep stage.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

References

  1. Wang, P., Liu, E., Zhi, B., et al.: A macro-micro viscoelastic-plastic constitutive model for saturated frozen soil. Mech. Mater. 147, 103411 (2020)

    Article  Google Scholar 

  2. Chen, Y., Yang, P., Zhou, Y., et al.: A micromechanics-based constitutive model for linear viscoelastic particle-reinforced composites. Mech. Mater. 140, 103228 (2020)

    Article  Google Scholar 

  3. Decraemer, W.F., Maes, M.A., Vanhuyse, V.J., et al.: A non-linear viscoelastic constitutive equation for soft biological tissues, based upon a structural model. J. Biomech. 13(7), 559–564 (1980)

    Article  Google Scholar 

  4. Sun, L., Zhu, Y.: A serial two-stage viscoelastic viscoplastic constitutive model with thermodynamical consistency for characterizing time-dependent deformation behavior of asphalt concrete mixtures. Constr. Build. Mater. 40, 584–595 (2013)

    Article  Google Scholar 

  5. Yang, W., et al.: Time-dependent behavior of diabase and a nonlinear creep model. Rock Mech. Rock Eng. 47, 1211–1224 (2014)

    Article  Google Scholar 

  6. Fung, Y.C.: Foundation of Solid Mechanics. Prentice-Hall Inc, Englewood Cliffs, N. J. (1965)

    Google Scholar 

  7. Cunha-Filho, A.G., Briend, Y., et al.: A new and efficient constitutive model based on fractional time derivatives for transient analyses of viscoelastic systems. Mech. Syst. Signal Process. 146, 107042 (2021)

    Article  Google Scholar 

  8. Yajima, T., Nagahama, H.: Differential geometry of viscoelastic models with fractional-order derivatives. J. Phys. A Math. Theor. 43, 385207 (2010)

    Article  MathSciNet  Google Scholar 

  9. Somarathna, H.M.C.C., et al.: Hyper-viscoelastic constitutive models for predicting the material behavior of polyurethane under varying strain rates and uniaxial tensile loading. Constr. Build. Mater. 236, 117417 (2020)

    Article  Google Scholar 

  10. Bahraini, S.M.S., et al.: Large deflection of viscoelastic beams using fractional derivative model. J. Mech. Sci. Technol. 27(4), 1063–1070 (2013)

    Article  Google Scholar 

  11. Martin, O.-S., Zhang, J.: Does a fractal microstructure require a fractional viscoelastic model? Fractal Fract. 2, 12 (2018)

    Article  Google Scholar 

  12. Cattani, C.: Fractal and fractional. Fractal Fract. 1, 1 (2017)

    Article  Google Scholar 

  13. Liouville, J.: Memoire sur le calcul des different idles a indices quelconques. Journal de EcolePolytechnique 13(21), 71–162 (1832)

    Google Scholar 

  14. Riemann, B.: Versucheinerallgemeinen auffassung der integration und differentiation. Bernhard Riemanns Gesammelte Mathematische Werke, Janvier, pp. 353–362 (1847)

  15. Caputo, M.: Linear model of dissipation whose q is almost frequency independent-II. Geophys. J. Int. 13, 529–539 (1967)

    Article  Google Scholar 

  16. Caputo, M.: Elasticitàe Dissipazione. Zanichelli, Bologna (1969)

    Google Scholar 

  17. Weyl, H.: Bemerkungen zum Begriff des Differentialquotienten gebrochener Ordnung. Zür. Naturf. Ges. 62, 296–302 (1917)

    MathSciNet  MATH  Google Scholar 

  18. Hilfer, R.: Applications of Fractional Calculus in Physics. World Scientific Publishing, New York (2000)

    Book  Google Scholar 

  19. Fernandez, A., Ozarslan, M.A., Baleanu, D.: On fractional calculus with general analytic kernels. Appl. Math. Comput. 354, 248–265 (2019)

    MathSciNet  MATH  Google Scholar 

  20. Baleanu, D., Fernandez, A., Akgul, A.: On a fractional operator combining proportional and classical differintegrals. Mathematics 8, 360 (2020)

    Article  Google Scholar 

  21. Cattani, C.: A review on harmonic wavelets and their fractional extension. J. Adv. Eng. Comput. 2(4), 224–238 (2018)

    Article  Google Scholar 

  22. Fernandez, A., Baleanu, D., Srivastava, H.M.: Series representations for fractional-calculus operators involving generalised Mittag–Leffler functions. Commun. Nonlinear Sci. Numer. Simul. 67, 517–527 (2019)

    Article  MathSciNet  Google Scholar 

  23. Dou, L., Yang, X.J., Liu, J.: A new general fractional-order wave model involving Miller–Ross kernel. Thermal ence 23(S3), S245–S245 (2019)

    Google Scholar 

  24. Feng, Y.Y., Liu, J.G.: Anomalous diffusion equation using a new general fractional derivative within the Miller–Ross kernel. Mod. Phys. Lett. B 34, 27 (2020)

    MathSciNet  Google Scholar 

  25. Yang, X.J., Gao, F., Ju, Y.: General Fractional Derivatives With Applications in Viscoelasticity. Academic Press, London (2020)

    MATH  Google Scholar 

  26. Miller, K.S., Ross, B.: An Introduction to the Fractional Calculus and Fractional Differential Equations. Wiley, New York (1993)

    MATH  Google Scholar 

  27. Yang, X.J.: General Fractional Derivatives: Theory, Methods and Applications. CRC Press, New York (2019)

    Book  Google Scholar 

  28. Doetch, G.: Theorie und Anwendung der Laplace-Transformation. Springer, Berlin (1937)

    Book  Google Scholar 

  29. Morro, A.: Modelling of viscoelastic materials and creep behaviour. Meccanica 52, 3015–3021 (2017)

    Article  MathSciNet  Google Scholar 

  30. Blair, G.S.: The role of psychophysics in rheology. J. Colloid Sci. 2(1), 21–32 (1947)

    Article  Google Scholar 

  31. Heaviside, O.: Electrical Papers, Elibron Classics, London, 1892, v. 1

  32. Prabhakar, T.R.: A singular integral equation with a generalized Mittag–Leffler function in the kernel. Yokohama Math. J. 19(1), 7–15 (1971)

    MathSciNet  MATH  Google Scholar 

Download references

Acknowledgements

This work was supported by the Yue-Qi Scholar of the China University of Mining and Technology (Grant No. 102504180004).

Author information

Authors and Affiliations

  1. School of Mechanics and Civil Engineering, China University of Mining and Technology, Xuzhou, 221116, Jiangsu, China

    Yi-Ying Feng, Xiao-Jun Yang, Jian-Gen Liu & Zhan-Qing Chen

  2. State Key Laboratory for Geomechanics and Deep Underground Engineering, China University of Mining and Technology, Xuzhou, 221116, Jiangsu, China

    Yi-Ying Feng, Xiao-Jun Yang, Jian-Gen Liu & Zhan-Qing Chen

  3. School of Mathematics, China University of Mining and Technology, Xuzhou, 221116, Jiangsu, China

    Xiao-Jun Yang & Jian-Gen Liu

Authors
  1. Yi-Ying Feng
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Xiao-Jun Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jian-Gen Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Zhan-Qing Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Xiao-Jun Yang.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Feng, YY., Yang, XJ., Liu, JG. et al. Rheological analysis of the general fractional-order viscoelastic model involving the Miller–Ross kernel. Acta Mech 232, 3141–3148 (2021). https://doi.org/10.1007/s00707-021-02994-7

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00707-021-02994-7

Search

Navigation