A new numerical approach to the solution of the 2-D Helmholtz equation with optimal accuracy on irregular domains and Cartesian meshes

Abstract

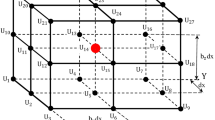
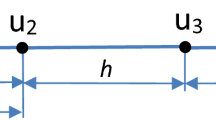
A new numerical approach for the time independent Helmholtz equation on irregular domains has been developed. Trivial Cartesian meshes and simple 9-point stencil equations with unknown coefficients are used for 2-D irregular domains. The calculation of the coefficients of the stencil equations is based on the minimization of the local truncation error of the stencil equations and yields the optimal order of accuracy. At similar 9-point stencils, the accuracy of the new approach is two orders higher for the Dirichlet boundary conditions and one order higher for the Neumann boundary conditions than that for the linear finite elements. The numerical results for irregular domains also show that at the same number of degrees of freedom, the new approach is even much more accurate than the quadratic and cubic finite elements with much wider stencils. The new approach can be equally applied to the Helmholtz and screened Poisson equations.

Appendices

Appendix A: The coefficients $b_p$ used in Eq. (10) in Sect. 2.1.

The first five coefficients $b_p$ ($p=1,2,\ldots ,5$) used in Eq. (10) are presented below. All coefficients $b_p$ used these formulas are given in the attached file ’b-coeff-1.pdf’

Eq. (10):

$$\begin{aligned} b_{1}= & {} {k_1}+{k_2}+{k_3}+{k_4}+{k_5}+{k_6}+{k_7}+{k_8}+{k_9} \\ b_{2}= & {} -{d_1} {k_1}+{d_3} {k_3}-{d_4} {k_4}+{d_5} {k_6}-{d_6} {k_7}+{d_8} {k_9} \\ b_{3}= & {} {b_y} (-{d_1} {k_1}-{d_2} {k_2}-{d_3} {k_3}+{d_6} {k_7}+{d_7} {k_8}+{d_8} {k_9}) \\ b_{4}= & {} -\frac{{d_1}^2 {k_1}}{2}-\frac{{d_3}^2 {k_3}}{2}-\frac{{d_4}^2 {k_4}}{2}-\frac{{d_5}^2 {k_6}}{2}-\frac{{d_6}^2 {k_7}}{2}\\&-\frac{{d_8}^2 {k_9}}{2}+{m_1}+{m_2}+{m_3}\\&+\,{m_4}+{m_5}+{m_6}+{m_7}+{m_8}+{m_9} \\ b_{5}= & {} {b_y} ({d_1}^2 {k_1}-{d_3}^2 {k_3}-{d_6}^2 {k_7}+{d_8}^2 {k_9}) \\ \end{aligned}$$

Appendix B: The coefficients $b_p$ used in Eq. (10) for the Neumann boundary conditions in Sect. 2.2.

The first five coefficients $b_p$ ($p=1,2,\ldots ,5$) used in Eq. (10) are presented below. All coefficients $b_p$ used these formulas are given in the attached file ’b-coeff-2.pdf’

Eq. (10):

$$\begin{aligned} b_{1}= & {} k_{1}+k_{2}+k_{3}+k_{4}+k_{5}+k_{6}+k_{7}+k_{8}+k_{9} \\ b_{2}= & {} -\,d_{1} k_{1}+d_{3} k_{3}-d_{4} k_{4}+d_{6} k_{6}-d_{7} k_{7}+d_{9} k_{9}\\&+\,\bar{k}_{1} n_{11}+\bar{k}_{2} n_{12}+\bar{k}_{3} n_{13}+\bar{k}_{4} n_{14}\\&+\,\bar{k}_{5} n_{15}+\bar{k}_{6} n_{16}+\bar{k}_{7} n_{17}+\bar{k}_{8} n_{18}\\&+\,\bar{k}_{9} n_{19} \\ b_{3}= & {} {b_y} (-d_{1} k_{1}-d_{2} k_{2}-d_{3} k_{3}+d_{7} k_{7}+d_{8} k_{8}\\&+\,d_{9} k_{9})+\bar{k}_{1} n_{21}+\bar{k}_{2} n_{22}+\bar{k}_{3} n_{23}\\&+\,\bar{k}_{4} n_{24}+\bar{k}_{5} n_{25}+\bar{k}_{6} n_{26}+\bar{k}_{7} n_{27}\\&+\,\bar{k}_{8} n_{28}+\bar{k}_{9} n_{29} \\ b_{4}= & {} -\frac{d_{1}^2 k_{1}}{2}+d_{1} \bar{k}_{1} n_{11}-\frac{d_{3}^2 k_{3}}{2}\\&-\,d_{3} \bar{k}_{3} n_{13}-\frac{d_{4}^2 k_{4}}{2}\\&+\,d_{4} \bar{k}_{4} n_{14}-\frac{d_{6}^2 k_{6}}{2}-d_{6} \bar{k}_{6} n_{16}\\&-\,\frac{d_{7}^2 k_{7}}{2}+d_{7} \bar{k}_{7} n_{17}-\frac{d_{9}^2 k_{9}}{2}\\&-\,d_{9} \bar{k}_{9} n_{19}+m_{1}+m_{2}\\&+\,m_{3}+m_{4}+m_{5}+m_{6}+m_{7}+m_{8}+m_{9} \\ b_{5}= & {} {b_y} (d_{1}^2 k_{1}-d_{1} \bar{k}_{1} n_{11}-d_{2} \bar{k}_{2} n_{12}-d_{3}^2 k_{3}\\&-\,d_{3} \bar{k}_{3} n_{13}-d_{7}^2 k_{7}+d_{7} \bar{k}_{7} n_{17}+d_{8} \bar{k}_{8} n_{18}\\&+\,d_{9}^2 k_{9}+d_{9} \bar{k}_{9} n_{19})-d_{1} \bar{k}_{1} n_{21}+d_{3} \bar{k}_{3} n_{23}\\&-\,d_{4} \bar{k}_{4} n_{24}+d_{6} \bar{k}_{6} n_{26}-d_{7} \bar{k}_{7} n_{27}\\&+\,d_{9} \bar{k}_{9} n_{29} \\ \end{aligned}$$