Unified Discrete Multisymplectic Lagrangian Formulation for Hyperelastic Solids and Barotropic Fluids

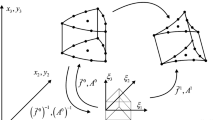
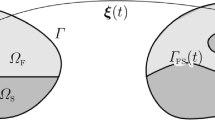
Abstract

We present a geometric variational discretization of nonlinear elasticity in 2D and 3D in the Lagrangian description. A main step in our construction is the definition of discrete deformation gradients and discrete Cauchy–Green deformation tensors, which allows for the development of a general discrete geometric setting for frame indifferent isotropic hyperelastic models. The resulting discrete framework is in perfect adequacy with the multisymplectic discretization of fluids proposed earlier by the authors. Thanks to the unified discrete setting, a geometric variational discretization can be developed for the coupled dynamics of a fluid impacting and flowing on the surface of an hyperelastic body. The variational treatment allows for a natural inclusion of incompressibility and impenetrability constraints via appropriate penalty terms. We test the resulting integrators in 2D and 3D with the case of a barotropic fluid flowing on incompressible rubber-like nonlinear models.

A Appendix

1.1 2D Discrete Hyperelastic Models

To compute the partial derivatives $D_k {\mathcal {L}} ^j_{a,b}$ of the discrete Lagrangian (34) we note

for all $\ell =1,...,4$ and all $k=1,3,5,7$. We observe that the 16 expressions are independent of the value of the field $\varphi _d$ and on the location of the spacetime node (if the spacing $\Delta s_a$ and $\Delta s_b$ are constant), i.e., we can write . For instance, for $\ell =1$, we have

$$\begin{aligned} D_1 {\mathbf {F}} _1 \cdot \delta \varphi&= \left[ - \frac{1}{ \Delta s_a} \delta \varphi , - \frac{1}{ \Delta s_b} \delta \varphi \right]&D_3 {\mathbf {F}} _1 \cdot \delta \varphi&= \left[ \frac{1}{ \Delta s_a} \delta \varphi , 0 \right] \\ D_5 {\mathbf {F}} _1 \cdot \delta \varphi&= \left[ 0 , \frac{1}{ \Delta s_b} \delta \varphi \right]&D_7 {\mathbf {F}} _1 \cdot \delta \varphi&= \left[ 0, 0 \right] \end{aligned}$$

and for $\ell =2$, we have

$$\begin{aligned} D_1 {\mathbf {F}} _2 \cdot \delta \varphi&= \left[ 0 , \frac{1}{ \Delta s_a} \delta \varphi \right]&D_3 {\mathbf {F}} _2 \cdot \delta \varphi&= \left[ - \frac{1}{ \Delta s_b} \delta \varphi , - \frac{1}{ \Delta s_a} \delta \varphi \right] \\ D_7 {\mathbf {F}} _2 \cdot \delta \varphi&= \left[ \frac{1}{ \Delta s_b} \delta \varphi , 0 \right]&D_5 {\mathbf {F}} _2 \cdot \delta \varphi&= \left[ 0 , 0 \right] , \end{aligned}$$

similarly for $\ell =3,4$. We can thus write

where in the last equality we defined the operator ${\mathfrak {D}}_k^\ell$, $k=1,3,5,7$, $\ell =1,2,3,4$, by duality.

From this, the partial derivatives $D_k {\mathcal {L}} _{a,b}^j$ of the discrete Lagrangian can be computed. For instance for $k=1$ one has

Using this result, one then derives (42) from (75).

1.2 3D Discrete Jacobian

1.3 3D Discrete Cauchy–Green Deformation Tensor

1.4 3D Derivatives of Impenetrability Constraints (77)

$$\begin{aligned} \begin{aligned} D_1\Psi _{\mathrm{im_1}}&= -(\varphi _{a-1,b,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{a,b-1,c}^j -\varphi _{a,b,c}^j) \\&\quad - (\varphi _{a,b-1,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{d,e,f}^j - \varphi _{a,b,c}^j ) \\&\quad - (\varphi _{d,e,f}^j - \varphi _{a,b,c}^j) \times (\varphi _{a-1,b,c}^j -\varphi _{a,b,c}^j), \\D_2\Psi _{\mathrm{im_1}}&= (\varphi _{a,b-1,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{d,e,f}^j - \varphi _{a,b,c}^j ), \\ D_3\Psi _{\mathrm{im_1}}&= (\varphi _{d,e,f}^j - \varphi _{a,b,c}^j ) \times (\varphi _{a-1,b,c}^j -\varphi _{a,b,c}^j), \\ D_4 \Psi _{\mathrm{im_1}}&= (\varphi _{a-1,b,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{a,b-1,c}^j -\varphi _{a,b,c}^j), \\ D_1\Psi _{\mathrm{im_2}}&= - (\varphi _{a,b-1,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{a+1,b,c}^j -\varphi _{a,b,c}^j) \\&\quad - (\varphi _{d+1,e,f}^j - \varphi _{a,b,c}^j ) \times (\varphi _{a,b-1,c}^j -\varphi _{a,b,c}^j) \\&\quad - (\varphi _{a+1,b,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{d+1,e,f}^j - \varphi _{a,b,c}^j ), \\ D_2\Psi _{\mathrm{im_2}}&= (\varphi _{d+1,e,f}^j - \varphi _{a,b,c}^j) \times (\varphi _{a,b-1,c}^j -\varphi _{a,b,c}^j), \\ D_3\Psi _{\mathrm{im_2}}&= (\varphi _{a+1,b,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{d+1,e,f}^j - \varphi _{a,b,c}^j), \\ D_4\Psi _{\mathrm{im_2}}&= (\varphi _{a,b-1,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{a+1,b,c}^j -\varphi _{a,b,c}^j), \\ D_1\Psi _{\mathrm{im_3}}&= - (\varphi _{a,b+1,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{a-1,b,c}^j -\varphi _{a,b,c}^j) \\&\quad -(\varphi _{d,e+1,f}^j - \varphi _{a,b,c}^j ) \times (\varphi _{a,b+1,c}^j -\varphi _{a,b,c}^j), \\&\quad - (\varphi _{a-1,b,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{d,e+1,f}^j - \varphi _{a,b,c}^j ), \\ D_2\Psi _{\mathrm{im_3}}&= (\varphi _{d,e+1,f}^j - \varphi _{a,b,c}^j ) \times (\varphi _{a,b+1,c}^j -\varphi _{a,b,c}^j), \\ D_3\Psi _{\mathrm{im_3}}&=(\varphi _{a-1,b,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{d,e+1,f}^j - \varphi _{a,b,c}^j ), \\ D_4\Psi _{\mathrm{im_3}}&= (\varphi _{a,b+1,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{a-1,b,c}^j -\varphi _{a,b,c}^j), \\ D_1\Psi _{\mathrm{im_4}}&= - (\varphi _{a+1,b,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{a,b+1,c}^j -\varphi _{a,b,c}^j) \\&\quad -(\varphi _{d+1,e+1,f}^j - \varphi _{a,b,c}^j ) \times (\varphi _{a+1,b,c}^j -\varphi _{a,b,c}^j) \\&\quad - (\varphi _{a,b+1,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{d+1,e+1,f}^j - \varphi _{a,b,c}^j), \\ D_2\Psi _{\mathrm{im_4}}&= (\varphi _{a,b+1,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{d+1,e+1,f}^j - \varphi _{a,b,c}^j), \\ D_3\Psi _{\mathrm{im_4}}&= (\varphi _{d+1,e+1,f}^j - \varphi _{a,b,c}^j) \times (\varphi _{a+1,b,c}^j -\varphi _{a,b,c}^j), \\ D_4\Psi _{\mathrm{im_4}}&= (\varphi _{a+1,b,c}^j -\varphi _{a,b,c}^j) \times (\varphi _{a,b+1,c}^j -\varphi _{a,b,c}^j). \end{aligned} \end{aligned}$$