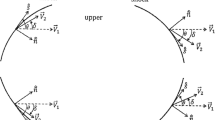
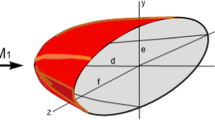
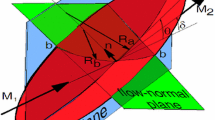
Abstract
A detached bow shock wave is strongest where it is normal to the upstream velocity. While the jump conditions across the shock are straightforward, many properties, such as the shock’s curvatures and derivatives of the pressure, along and normal to a normal shock, are indeterminate. A novel procedure is introduced for resolving the indeterminacy when the unsteady flow is three-dimensional and the upstream velocity may be nonuniform. Utilizing this procedure, normal shock relations are provided for the nonunique orientation of the flow plane and the corresponding shock’s curvatures and, e.g., the downstream normal derivatives of the pressure and the velocity components. These algebraic relations explicitly show the dependence of these parameters on the shock’s shape and the upstream velocity gradient. A simple relation, valid only for a normal shock, is obtained for the average curvatures. Results are also obtained when the shock is an elliptic paraboloid shock. These derivatives are both simple and proportional to the average curvature.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
Abbreviations
- \(A_a ,A_c ,A_e \) :
-
Defined by (40)
- \(\tilde{b}\) :
-
Coordinate normal to the flow plane
- \(\hat{\tilde{b}}\) :
-
Unit vector normal to the flow plane
- \(c_i ,c_{{ ij}} \) :
-
Coefficient defining the shock’s configuration
- \(e_{{ ij}} \) :
-
Coefficient defining the upstream velocity gradient
- F :
-
\(F=0\) provides the shock’s configuration
- \(G_i, H_i \) :
- \(h_3\) :
-
\(\tilde{b}\)-coordinate scale factor
- \({\hat{|}} _i \) :
-
Cartesian coordinate basis
- \(K_i \) :
-
Defined by (20)
- \(k_2 ,k_3 \) :
-
Defined by (25)
- \(L_i \) :
-
Defined by (21)
- M :
-
Mach number
- m :
-
\(M_1^2 \)
- \(\tilde{n}\) :
-
Coordinate normal to the shock, positive in the downstream direction
- \(\hat{\tilde{n}}\) :
-
Unit vector normal to the shock, oriented in the downstream direction
- p :
-
Pressure
- r :
-
Nose radius of the shock when the shock is two-dimensional or axisymmetric
- R :
-
Gas constant
- \(\tilde{s}\) :
-
Arc length along the shock in the flow plane
- \(\hat{\tilde{s}}\) :
-
Unit vector tangent to the shock in the flow plane
- S :
-
Entropy
- \(S_a \) :
-
Shock curvature in the flow plane
- \(S_b \) :
-
Shock curvature in a plane normal to the shock and the flow plane
- \(S_1 ,S_2 \) :
-
Orthogonal shock curvatures
- t :
-
Time
- u :
-
Velocity component tangent to the shock in the flow plane, just downstream of the shock
- v :
-
Velocity component normal to the shock, just downstream of the shock
- \(\vec {V}\) :
-
Velocity
- w :
-
\(M_1^2 \hbox {sin}^{2}\beta \)
- \(x_i \) :
-
Cartesian coordinates centered at a point on the shock, where \(x_i \) is parallel to \(\vec {V}_1\)
- X :
-
\(1+\frac{\gamma -1}{2}w\)
- Y :
-
\(\gamma w-\frac{\gamma -1}{2}\)
- Z :
-
\(w-1\)
- \(\alpha \) :
-
Angle between planes containing \(S_1 \) and \(S_a \)
- \(\gamma \) :
-
Ratio of specific heats
- \(\theta \) :
-
Shock wave angle measured relative to \(\vec {V}_1\) in the flow plane
- \(\varepsilon \) :
-
Small parameter
- \(\rho \) :
-
Density
- \(\sigma \) :
-
\(=0/1\) for a two-dimensional/axisymmetric flow
- \(\chi \) :
-
Defined by (22)
- \({\psi }\) :
-
Defined by (67)
- 1:
-
Flow state just upstream of the shock
- 2:
-
Flow state just downstream of the shock
- o :
-
Location where the shock is a normal shock
- ( )\(^*\) :
-
Location where the shock is not a normal shock
- (\(\,\hat{\,}\,\)):
-
Denotes a unit vector
References
Emanuel, G.: Analytical Fluid Dynamics, 3rd edn. CRC Press, Boca Raton (2016). doi:10.1201/b19392
Mölder, S.: Curved aerodynamic shock waves. PhD Dissertation. McGill University (2012)
Mölder, S.: Curved shock theory. Shock Waves 26, 337–353 (2016). doi:10.1007/s00193-015-0589-9
Struik, D.J.: Differential Geometry, p. 81. Addison-Wesley Press, Cambridge (1950)
Acknowledgements
The author acknowledges the helpful comments of S. Mölder.
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by D. Zeitoun and A. Higgins.
Rights and permissions
About this article
Cite this article
Emanuel, G. Flow derivatives and curvatures for a normal shock. Shock Waves 28, 427–435 (2018). https://doi.org/10.1007/s00193-017-0742-8
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00193-017-0742-8