Skip to main content
SpringerLink
Log in

Analytical solution for the micropolar cylindrical shell: Carrera unified formulation (CUF) approach

  • Original Article
  • Published:
Continuum Mechanics and Thermodynamics Aims and scope Submit manuscript

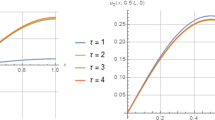
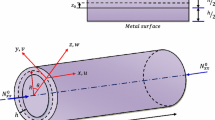
Abstract

Here, an analytical form Navier solution for higher-order micropolar theory of cylindrical shell was developed based on the CUF approach. The cases of complete linear expansion and model based on Timoshenko-Mindlin shear deformation hypothesis are considered in detail. The two-dimensional system of differential equations obtained here using the principle of virtual displacements for the theory of micropolar elastic cylindrical shell of a higher order is solved here for the case of freely supported cylindrical shell using the Navier variable separation method. Some numerical examples were performed and the influence of the rotational field, as well as the micropolar couple stress on the stress–strain state, was analyzed. The equations presented here can be used for the stress–strain calculation and for thin-walled structures modeling in macro-, micro-, and nanoscale, with considering effects of micropolar couple stress and rotation.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Altenbach, H., Eremeyev, V.: On the linear theory of micropolar plates. J. Appl. Math. Mech. ZAMM 89(4), 242–256 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  2. Altenbach, J., Altenbach, H., Eremeyev, V.A.: On generalized Cosserat-type theories of plates and shells. A short review and bibliography. Arch. Appl. Mech. 80, 73–92 (2010)

    Article  ADS  MATH  Google Scholar 

  3. Ambartsumian, S.A.: The theory of transverse bending of plates with asymmetric elasticity. Mech. Compos. Mater. 32(1), 30–38 (1996)

    Article  ADS  Google Scholar 

  4. Ambartsumian, S.A.: The Micropolar Theory of Shells and Plates, p. 187. Springer Nature, Switzerland (2021)

    Book  MATH  Google Scholar 

  5. Anderson, W.B., Lakes, R.: Size effects due to Cosserat elasticity and surface damage in closed-cell polymethacrylimide foam. J. Mater. Sci. 29(24), 6413–6419 (1994)

    Article  ADS  Google Scholar 

  6. Askar, A.: Molecular crystals and the polar theories of the continua. Experimental values of material coefficients for KNO3. Int. J. Eng. Sci. 10(3), 293–300 (1972)

    Article  MATH  Google Scholar 

  7. Bigoni, D., Drugan, W.J.: Analytical derivation of Cosserat moduli via homogenization of heterogeneous elastic materials. J. Appl. Mech. 74(4), 741–753 (2006)

    Article  MathSciNet  Google Scholar 

  8. Carrera, E.: A class of two-dimensional theories for anisotropic multilayered plates analysis. Atti della accademia delle scienze di Torino. classe di scienze fisiche matematiche e naturali 19—-20, 1–39 (1995)

    MathSciNet  Google Scholar 

  9. Carrera, E.: Developments, ideas and evaluations based upon the Reissner’s mixed variational theorem in the modeling of multilayered plates and shells. Appl. Mech. Rev. 54, 301–329 (2001)

    Article  ADS  Google Scholar 

  10. Carrera, E.: Theories and finite elements for multilayered plates and shells: a unified compact formulation with numerical assessment and benchmarking. Arch. Comput. Methods Eng. 10(3), 215–296 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  11. Carrera, E., Cinefra, M., Petrolo, M., Zappino, E.: Finite Element Analysis of Structures Through Unified Formulation. John Wiley & Sons Ltd, Chichester (2014)

    Book  MATH  Google Scholar 

  12. Carrera, E., Elishakoff, I., Petrolo, M.: Who needs refined structural theories? Compos. Struct. 264, 113671 (2021)

    Article  Google Scholar 

  13. Carrera, E., Zozulya, V.V.: Carrera unified formulation (CUF) for the micropolar beams: analytical solutions. Mech. Adv. Mater. Struct. (2019). https://doi.org/10.1080/15376494.2019.1578013

  14. Carrera, E., Zozulya, V.V.: Carrera unified formulation (CUF) for the micropolar plates and shells. I. Higher order theory. Mech. Adv. Mater. Struct. 29(6), 773–795 (2022). https://doi.org/10.1080/15376494.2020.1793241

    Article  Google Scholar 

  15. Carrera, E., Zozulya, V.V.: Carrera unified formulation (CUF) for the micropolar plates and shells. II. Complete linear expansion case. Mech. Adv. Mater. Struct. 29(6), 796–815 (2022). https://doi.org/10.1080/15376494.2020.1793242

    Article  Google Scholar 

  16. Carrera, E., Zozulya, V.V.: Carrera unified formulation for the micropolar plates. Mech. Adv. Mater. Struct. (2021). https://doi.org/10.1080/15376494.2021.1889726

  17. Carrera, E., Zozulya, V.V.: Closed-form solution for the micropolar plates: carrera unified formulation (CUF) approach. Arch. Appl. Mech. 91, 91–116 (2021). https://doi.org/10.1007/s00419-020-01756-6

    Article  ADS  Google Scholar 

  18. Chiroiu, V., Munteanu, L.: Estimation of micropolar elastic moduli by inversion of vibrational data. Complex. Int. 9, 1–10 (2002)

    MathSciNet  MATH  Google Scholar 

  19. Chung, J., Waas, A.M.: The micropolar elasticity constants of circular cell honeycombs. Proc. R. Soc. London A Math. Phys. Eng. Sci. 465, 25–39 (2009). https://doi.org/10.1098/rspa.2008.0225

    Article  ADS  MathSciNet  MATH  Google Scholar 

  20. Cosserat, E., Cosserat, F.: Théorie des corps déformables. Paris, France, A. Hermann et Fils, 1909, 242 p. (English translation by D.H. Delphenich available at http://www.uni-due.de/~hm0014/Cosserat?les/Cosserat09 eng.pdf)

  21. Eremeyev, V.A., Lebedev, L.P., Altenbach, H.: Foundations of Micropolar Mechanics, p. 144. Springer, New York (2013)

    Book  MATH  Google Scholar 

  22. Eremeyev, V.A., Skrzat, A., Stachowicz, F.: Linear micropolar elasticity analysis of stresses in bones under static loads. Strength Mater. 49(4), 575–585 (2017)

    Article  Google Scholar 

  23. Eremeyev, V.A., Skrzat, A., Vinakurava, A.: Application of the micropolar theory to the strength analysis of bioceramic materials for bone reconstruction. Strength Mater. 48(4), 573–582 (2016)

    Article  Google Scholar 

  24. Ericksen, J.L., Truesdell, C.: Exact theory of stress and strain in rods and shells. Arch. Ration. Mech. Anal. 1(1), 295–323 (1958)

    Article  MathSciNet  MATH  Google Scholar 

  25. Eringen, A.C.: Theory of micropolar plates. J. Appl. Math. Phys. ZAMP 18(1), 12–30 (1967)

    Google Scholar 

  26. Eringen, A.C.: Microcontinuum Field Theories I. Foundations and Solids, p. 336. Springer, New York (1999)

    Book  MATH  Google Scholar 

  27. Eugster, S.R., dell’Isola, F.: Exegesis of the Introduction and Sect. I from “Fundamentals of the Mechanics of Continua’’ by E. Hellinger. J. Appl. Math. Mech. ZAMM 97(4), 477–506 (2017)

    Article  MathSciNet  Google Scholar 

  28. Eugster, S.R., dell’Isola, F.: Exegesis of Sect. II and III. A from “Fundamentals of the Mechanics of Continua by E. Hellinger. J. Appl. Math. Mech. ZAMM 98(1), 31–68 (2018)

    Article  MathSciNet  Google Scholar 

  29. Eugster, S.R., dell’Isola, F.: Exegesis of Sect. III. B from “Fundamentals of the Mechanics of Continua’’ by E. Hellinger. J. Appl. Math. Mech. ZAMM 98(1), 69–105 (2018)

    Article  MathSciNet  Google Scholar 

  30. Gauthier, R.D.: Experimental Investigation on Micropolar Media, In: Brulin O., Hsieh R.K.T. (eds.) Mechanics of Micropolar Media, CISM Lecture Notes, World Scientific Publishing Co Pte Ltd, P.O. Box 128, Farrer Road, Singepore 9128, pp. 395–463, (1982)

  31. Gauthier, R.D., Jahsman, W.E.: A quest for micropolar elastic constants. J. Appl. Mech. 42(2), 369–374 (1975)

    Article  ADS  MATH  Google Scholar 

  32. Gauthier, R.D., Jahsman, W.E.: A quest for micropolar elastic constants. Part II. Arch. Mech. 33(5), 717–737 (1981)

    MATH  Google Scholar 

  33. Hassanpour, S., Heppler, G.R.: Micropolar elasticity theory: a survey of linear isotropic equations, representative notations, and experimental investigations. Math. Mech. Solids 22(2), 224–242 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  34. Jemielita, G.: Biharmonic representaion of the solution to a plate made of a Cosserat material. Mechanika Theoretyczna i Stosowana 2(30), 359–367 (1992)

    Google Scholar 

  35. Karttunen, A.T., Reddy, J.N., Romanoff, J.: Two-scale micropolar plate model for web-core sandwich panels. Int. J. Solids Struct. 170, 82–94 (2019)

    Article  Google Scholar 

  36. Kovvali, R.K., Hodges, D.H.: Variational asymptotic modeling of Cosserat elastic plates. AIAA J. 55(6), 2060–2073 (2017)

    Article  ADS  Google Scholar 

  37. Kvasov, R., Steinberg, L.: Numerical modeling of bending of micropolar plates. Thin-Walled Struct. 69, 67–78 (2013)

    Article  Google Scholar 

  38. Lakes, R.: Size effects and micromechanics of a porous solid. J. Mater. Sci. 18(9), 2572–2580 (1983). https://doi.org/10.1007/BF00547573

    Article  ADS  Google Scholar 

  39. Lakes, R.S.: Experimental microelasticity of two porous solids. Int. J. Solids Struct. 22, 55–63 (1986)

    Article  Google Scholar 

  40. Lakes, R.S.: Experimental micro mechanics methods for conventional and negative Poisson’s ratio cellular solids as Cosserat continua. J. Eng. Mater. Technol. 113(1), 148–155 (1991)

    Article  Google Scholar 

  41. Lakes, R.S.: Experimental methods for study of Cosserat elastic solids and other generalized continua. In: Muhlhaus, H., Wiley, J. (eds.) Continuum Models for Materials with Micro-structure, pp. 1–22. Wiley, New York (1995)

    MATH  Google Scholar 

  42. Liebenstein, S., Zaiser, M.: Determining Cosserat constants of 2D cellular solids from beam models. Mater. Theory 2(2), 1–20 (2018). https://doi.org/10.1186/s41313-017-0009-x

    Article  Google Scholar 

  43. Mindlin, R.D.: Influence of rotatory inertia and shear in flexural motions of isotropic elastic plates. J. Appl. Mech. 18(1), 31–38 (1951)

    Article  ADS  MATH  Google Scholar 

  44. Nowacki, W.: Theory of Axymmetric Elasticity, p. 390. Pergamon Press, New York (1986)

    Google Scholar 

  45. Park, H.C., Lakes, R.S.: Cosserat micromechanics of human bone: strain redistribution by a hydration-sensitive constituent. J. Biomech. 19(5), 385–397 (1986)

    Article  Google Scholar 

  46. Pelekh, B.L., Sukhorol’skii, M.A.: Contact Problems of the Theory of Elastic Anisotropic Shells. Naukova dumka, Kiev (1980). (in Russian)

  47. Pelekh, B.L.: Theory of Shells with Finite Shear Stifness. Naukova dumka, Kiev (1973). (in Russian)

  48. Petrolo, M., Carrera, E.: Methods and guidelines for the choice of shell theories. Acta Mech. 231, 395–434 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  49. Reddy, J.N.: Mechanics of Laminated Composite Plates and Shells: Theory and Analysis, 2nd edn., p. 855. CRC Press LLC, New York (2004)

    Google Scholar 

  50. Reddy, J.N.: Theory and Analysis of Elastic Plates and Shells, 2nd edn., p. 561. CRC Press LLC, New York (2006)

    Book  Google Scholar 

  51. Rueger, Z., Lakes, R.S.: Cosserat elasticity of negative Poisson’s ratio foam: experiment. Smart Mater. Struct. 25(5), 054004 (2016)

    Article  ADS  Google Scholar 

  52. Rueger, Z., Lakes, R.S.: Experimental Cosserat elasticity in open cell polymer foam. J. Philos. Mag. Part A: Mater. Sci. 96(2), 93–111 (2016)

    Article  Google Scholar 

  53. Sargsyan, S.H.: Mathematical model of micropolar elastic thin plates and their strength and stiffness characteristics. J. Appl. Mech. Tech. Phys. 53(2), 275–282 (2012)

    Article  ADS  Google Scholar 

  54. Shaw, S.: Bending of a thin rectangular isotropic plate: a Cosserat elasticity analysis. Compos. Mech. Comput. Appl. 8(4), 299–314 (2017)

    Article  Google Scholar 

  55. Steinberg, L.: Deformation of micropolar plates of moderate thickness. Int. J. Appl. Math. Mech. 6(17), 1–24 (2010)

    Google Scholar 

  56. Steinberg, L., Kvasov, R.: Enhanced mathematical model for Cosserat plate bending. Thin-Walled Struct. 63, 51–62 (2013)

    Article  Google Scholar 

  57. Timoshenko, S.P.: (a). On the transverse vibrations of bars of uniform cross section. Phil. Mag. 43, 125–131 (1922)

    Article  Google Scholar 

  58. Timoshenko, S., Wojnowsky-Krieger, S.: Theory of Plates and Shells, 2nd edn. McGraw-Hill Book Company, Paris (1959)

    Google Scholar 

  59. Truesdell, C., Toupin, R.: The classical field theories. In: Flügge, S. (ed.) Handbuch der Physik. Springer, Berlin (1960)

    Google Scholar 

  60. Vekua, I.N.: Shell theory, general methods of construction, p. 287. Pitman Advanced Pub. Program, Boston (1986)

  61. Washizu, K.: Variational Methods in Elasticity and Plasticity, 3rd edn. Pergamon Press, New York (1982)

    MATH  Google Scholar 

  62. Yang, J.F.C., Lakes, R.S.: Experimental study of micropolar and couple stress elasticity in compact bone in bending. J. Biomech. 15(2), 91–98 (1982)

    Article  Google Scholar 

  63. Zozulya, V.V.: A higher order theory for shells, plates and rods. Int. J. Mech. Sci. 103, 40–54 (2015)

    Article  Google Scholar 

  64. Zozulya, V.V.: Micropolar curved rods. 2-D, high order, Timoshenko’s and Euler-Bernoulli models. Curved Layer. Struct. 4, 104–118 (2017)

    Article  Google Scholar 

  65. Zozulya, V.V.: Couple stress theory of curved rods. 2-D, high order, Timoshenko’s and Euler-Bernoulli models. Curved Layer. Struct. 4, 119–132 (2017)

    Article  Google Scholar 

  66. Zozulya, V.V.: Higher order theory of micropolar plates and shells. J. Appl. Math. Mech. ZAMM 98(6), 886–918 (2018)

    Article  MathSciNet  Google Scholar 

  67. Zozulya, V.V.: Higher order couple stress theory of plates and shells. J. Appl. Math. Mech. ZAMM 98(10), 1834–1863 (2018)

    Article  MathSciNet  Google Scholar 

  68. Zozulya, V.V.: Higher order theory of micropolar curved rods. In: Altenbach, H., Öchsner, A. (eds.) Encyclopedia of Continuum Mechanics, pp. 1–11. Springer, Berlin, Heidelberg (2018)

    Google Scholar 

  69. Zozulya, V.V., Carrera, E.: Carrera unified formulation (CUF) for the micropolar plates and shells III. Classical models. Mech. Adv. Mater. Struct. (2021). https://doi.org/10.1080/15376494.2021.1975855

    Article  Google Scholar 

Download references

Acknowledgements

This work was supported by the visiting professor grants provided by Politecnico di Torino Research Excellence 2021 and the Committee of Science and Technology of Mexico (Ciencia Basica, Ref. No 256458), which are gratefully acknowledged.

Author information

Authors and Affiliations

  1. Department of Aeronautics and Aerospace Engineering, Politecnico di Torino, Corso Duca degli Abruzzi, 24, 10129, Torino, Italy

    E. Carrera & V. V. Zozulya

  2. S. P. Timoshenko Institute of Mechanics, National Academy of Sciences of Ukraine, Nesterov Str. 3, Kyiv, 252680, Ukraine

    V. V. Zozulya

  3. Department of Mechanical Engineering, College of Engineering, Prince Mohammad Bin Fahd University, Al Khobar, Kingdom of Saudi Arabia

    E. Carrera

Authors
  1. E. Carrera
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. V. V. Zozulya
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to V. V. Zozulya.

Additional information

Communicated by Andreas Öchsner.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Appendices

Appendix 1. Coefficients of the fundamental nucleus matrixes \({\mathbf{K}}_{\tau ,s}^{n,m,loc} \)

$$\begin{aligned} K^{n,m,\tau ,s}_{u_{x} ,u_{x} }= & {} C_{55} J_{\tau _{r} ,s_{r} }^{u_{x} ,u_{x} } +C_{11} J_{\tau ,s}^{u_{x} ,u_{x} } \frac{\pi ^{2}n^{2}}{L^{2} }+C_{44} J_{\tau ,s}^{u_{x} ,u_{x} } \frac{\pi ^{2}m^{2}}{R^{2}\varphi _{0}^{2} },\\ K^{n,m,\tau ,s}_{u_{x} ,u_{\varphi } }= & {} \left( {C_{12} +C_{44}^{T} } \right) \frac{\pi ^{2}nm}{LR\varphi _{0} }J_{\tau ,s}^{u_{x} ,u_{\varphi } }, \\ K^{n,m,\tau ,s}_{u_{x} ,u_{r} }= & {} \left( {C_{55}^{T} J_{\tau _{r} ,s}^{u_{x} ,u_{r} } -J_{\tau ,s}^{u_{x} ,u_{r} } C_{12} /R-C_{13} J_{\tau ,s_{r} }^{u_{x} ,u_{r} } } \right) \frac{\pi n}{L}, K^{n,m,\tau ,s}_{u_{x} ,\omega _{x} } =0,\\ K^{n,m,\tau ,s}_{u_{x} ,\omega _{y} }= & {} \left( {C_{55}^{T} -C_{55} } \right) J_{\tau _{z} ,s}^{u_{x} ,\omega _{y} } , K^{n,m,\tau ,s}_{u_{x} ,\omega _{z} } =\left( {C_{44} -C_{44}^{T} } \right) J_{\tau ,s}^{u_{x} ,\omega _{z} } \frac{\pi m}{R\varphi _{0} }, \\ K^{n,m,\tau ,s}_{u_{\varphi } ,u_{x} }= & {} \left( {C_{12} +C_{44}^{T} } \right) J_{\tau ,s}^{u_{\varphi } ,u_{x} } \frac{\pi ^{2}nm}{LR\varphi _{0} },\\ K^{n,m,\tau ,s}_{u_{\varphi } ,u_{\varphi } }= & {} \frac{J_{\tau _{r} ,s_{r} }^{u_{\varphi } ,u_{\varphi } } C_{66} -\left( {J_{\tau ,s_{r} }^{u_{\varphi } ,u_{\varphi } } +J_{\tau _{r} ,s}^{u_{\varphi } ,u_{\varphi } } } \right) C_{66}^{T} }{R^{2}}+C_{44} J_{\tau ,s}^{u_{\varphi } ,u_{\varphi } } \frac{\pi ^{2}n^{2}}{L^{2} }+C_{22} J_{\tau ,s}^{u_{\varphi } ,u_{\varphi } } \frac{\pi ^{2}m^{2}}{R^{2}\varphi _{0}^{2} },\\ K^{n,m,\tau ,s}_{u_{\varphi } ,u_{r} }= & {} -\frac{\pi m\left( {J_{\tau ,s}^{u_{\varphi } ,u_{r} } C_{22} +J_{\tau ,s_{r} }^{u_{\varphi } ,u_{r} } RC_{23} +J_{\tau _{r} ,s_{r} }^{u_{\varphi } ,u_{r} } C_{66} -J_{\tau _{r} ,s}^{u_{\varphi } ,u_{r} } C_{66}^{T} } \right) }{R^{2}\varphi _{0} }, K^{n,m,\tau ,s}_{u_{\varphi } ,\omega _{\varphi } } =0,\\ K^{n,m,\tau ,s}_{u_{\varphi } ,\omega _{x} }= & {} \left( {C_{66} -C_{66}^{T} } \right) \left( {J_{\tau _{r} ,s}^{u_{\varphi } ,\omega _{x} } +J_{\tau ,s}^{u_{\varphi } ,\omega _{x} } /R} \right) , K^{n,m,\tau ,s}_{u_{\varphi } ,\omega _{r} } =\left( {C_{44}^{T} -C_{44} } \right) J_{\tau ,s}^{u_{\varphi } ,\omega _{r} } \frac{\pi n}{L}, \\ K^{n,m,\tau ,s}_{u_{r} ,u_{x} }= & {} \left( {C_{55}^{T} J_{\tau ,s_{r} }^{u_{r} ,u_{x} } -C_{13} J_{\tau _{r} ,s}^{u_{r} ,u_{x} } +C_{12} J_{\tau ,s}^{u_{r} ,u_{x} } /R} \right) \frac{\pi n}{L},\\ K^{n,m,\tau ,s}_{u_{r} ,u_{\varphi } }= & {} \left( {C_{66}^{T} J_{\tau ,s_{r} }^{u_{r} ,u_{\varphi } } -C_{23} J_{\tau _{r} ,s}^{u_{r} ,u_{\varphi } } -C_{22} J_{\tau ,s}^{u_{r} ,u_{\varphi } } /R} \right) \frac{\pi m}{R\varphi _{0} },\\ K^{n,m,\tau ,s}_{u_{r} ,u_{r} }= & {} \frac{C_{22} J_{\tau ,s}^{u_{r} ,u_{r} } }{R^{2}}+\frac{\left( {J_{\tau _{r} ,s}^{u_{r} ,u_{r} } +J_{\tau ,s_{r} }^{u_{r} ,u_{r} } } \right) C_{23} }{R}+C_{33} J_{\tau _{r} ,s_{r} }^{u_{r} ,u_{r} } +C_{55} J_{\tau ,s}^{u_{r} ,u_{r} } \frac{\pi ^{2}n^{2}}{L^{2} }+C_{66} J_{\tau ,s}^{u_{r} ,u_{r} }\\ \frac{\pi ^{2}m^{2}}{R^{2}\varphi _{0}^{2} }, K^{n,m,\tau ,s}_{u_{r} ,\omega _{r} } =0, \\ K^{n,m,\tau ,s}_{u_{r} ,\omega _{x} }= & {} \left( {C_{66} -C_{66}^{T} } \right) J_{\tau ,s}^{u_{r} ,\omega _{x} } \frac{\pi m}{R\varphi _{0} }, K^{n,m,\tau ,s}_{u_{r} ,\omega _{\varphi } } =\left( {C_{55} -C_{55}^{T} } \right) J_{\tau ,s}^{u_{r} ,\omega _{\varphi } } \frac{\pi n}{L}, \\ K^{n,m,\tau ,s}_{\omega _{x} ,u_{x} } = 0, K^{n,m,\tau ,s}_{\omega _{x} ,u_{\varphi } }= & {} \left( {C_{66} -C_{66}^{T} } \right) \left( {J_{\tau ,s_{r} }^{\omega _{x} ,u_{\varphi } } +J_{\tau ,s}^{\omega _{x} ,u_{\varphi } } /R} \right) \quad L^{n,m,\tau ,s}_{\omega _{x} ,u_{r} } =\left( {C_{66}^{T} -C_{66} } \right) J_{\tau ,s}^{\omega _{x} ,u_{z} } \frac{\pi m}{R\varphi _{0} }, \\ K^{n,\tau ,s}_{\omega _{x} ,\omega _{x} }= & {} A_{55} J_{\tau _{r} ,s_{r} }^{\omega _{x} ,\omega _{x} } +J_{\tau ,s}^{\omega _{x} ,\omega _{x} } \left( {2\left( {C_{66} -C_{66}^{T} } \right) +\pi ^{2}\left( {\frac{n^{2}A_{11} }{L^{2} }+\frac{m^{2}A_{44} }{R^{2}\varphi _{0}^{2} }} \right) } \right) ,\\ K^{n,m,\tau ,s}_{\omega _{x} ,\omega _{\varphi } }= & {} \left( {A_{11} +A_{44}^{T} } \right) J_{\tau ,s}^{\omega _{x} ,\omega _{\varphi } } \frac{\pi ^{2}nm}{LR\varphi _{0} },\\ K^{n,m,\tau ,s}_{\omega _{x} ,\omega _{r} }= & {} \left( {A_{12} J_{\tau _{x} ,s}^{\omega _{x} ,\omega _{r} } /R+A_{13} J_{\tau _{x} ,s_{r} }^{\omega _{x} ,\omega _{r} } -C_{55}^{T} J_{\tau _{r} ,s_{x} }^{\omega _{x} ,\omega _{r} } } \right) \frac{\pi n}{L}, \\ K^{n,m,\tau ,s}_{\omega _{\varphi } ,u_{x} }= & {} \left( {C_{55}^{T} -C_{55} } \right) J_{\tau ,s_{r} }^{\omega _{\varphi } ,u_{x} } , K^{n,m,\tau ,s}_{\omega _{\varphi } ,u_{\varphi } } =0, \quad K^{n,m,\tau ,s}_{\omega _{\varphi } ,u_{r} } =\left( {C_{55}^{T} -C_{55} } \right) J_{\tau ,s}^{\omega _{\varphi } ,u_{r} } \frac{\pi n}{L},\\ K^{n,m,\tau ,s}_{\omega _{\varphi } ,\omega _{x} }= & {} \left( {A_{12} +A_{44}^{T} } \right) J_{\tau ,s}^{\omega _{\varphi } ,\omega _{x} } \frac{\pi ^{2}nm}{LR\varphi _{0} },\\ K^{n,m,\tau ,s}_{\omega _{\varphi } ,\omega _{r} }= & {} \left( {A_{23} J_{\tau ,s_{r} }^{\omega _{\varphi } ,\omega _{r} } +A_{66} J_{\tau ,s}^{\omega _{\varphi } ,\omega _{r} } /R-A_{66}^{T} J_{\tau _{r} ,s}^{\omega _{\kappa } ,\omega _{r} } } \right) \frac{\pi m}{R\varphi _{0} }, \\ K^{n,m,\tau ,s}_{\omega _{\varphi } ,\omega _{\varphi } }= & {} \left( {\frac{J_{\tau ,s}^{\omega _{\varphi } ,\omega _{\varphi } } }{R^{2}}+J_{\tau _{r} ,s_{r} }^{\omega _{\varphi } ,\omega _{\varphi } } } \right) A_{66} -\frac{\left( {J_{\tau ,s_{r} }^{\omega _{\varphi } ,\omega _{\varphi } } +J_{\tau _{r} ,s}^{\omega _{\varphi } ,\omega _{\varphi } } } \right) A_{66}^{T} }{R}\\&\quad +\, J_{\tau ,s}^{\omega _{\varphi } ,\omega _{\varphi } } \left( {2C_{55} -2C_{55}^{T} +\pi ^{2}\left( {\frac{n^{2}A_{44} }{L^{2} }+\frac{m^{2}A_{22} }{R^{2}\varphi _{0}^{2} }} \right) } \right) , \\ K^{n,m,\tau ,s}_{\omega _{r} ,u_{x} }= & {} \left( {C_{44}^{T} -C_{44} } \right) J_{\tau ,s}^{\omega _{r} ,u_{x} } \frac{\pi m}{R\varphi _{0} }, K^{n,m,\tau ,s}_{\omega _{r} ,u_{\varphi } } =\left( {C_{44} -C_{44}^{T} } \right) J_{\tau ,s}^{\omega _{r} ,u_{\varphi } } \frac{\pi n}{L}, K^{n,m,\tau ,s}_{\omega _{r} ,u_{r} } =0,\\ K^{n,m,\tau ,s}_{\omega _{r} ,\omega _{x} }= & {} \left( {A_{12} J_{\tau ,s}^{\omega _{r} ,\omega _{x} } /R+A_{13} J_{\tau _{r} ,s}^{\omega _{r} ,\omega _{x} } +A_{55}^{T} J_{\tau ,s_{r} }^{\omega _{r} ,\omega _{x} } } \right) \frac{\pi n}{L},\\ K^{n,m,\tau ,s}_{\omega _{r} ,\omega _{\varphi } }= & {} \left( {A_{22} J_{\tau ,s}^{\omega _{r} ,\omega _{\varphi } } /R+A_{23} J_{\tau _{r} ,s}^{\omega _{r} ,\omega _{\varphi } } -A_{66}^{T} J_{\tau ,s_{r} }^{\omega _{r} ,\omega _{\varphi } } } \right) \frac{\pi m}{\varphi _{0} }, \\ K^{n,m,\tau ,s}_{\omega _{r} ,\omega _{r} }= & {} \frac{A_{22} J_{\tau ,s}^{\omega _{r} ,\omega _{r} } }{R^{2}}+\frac{\left( {J_{\tau _{r} ,s}^{\omega _{r} ,\omega _{r} } +J_{\tau ,s_{r} }^{\omega _{r} ,\omega _{r} } } \right) A_{23} }{R}+A_{33} J_{\tau _{r} ,s_{r} }^{\omega _{r} ,\omega _{r} } +2\left( {C_{44} -C_{44}^{T} } \right) J_{\tau ,s}^{\omega _{r} ,\omega _{r} } \\&\quad +A_{55} J_{\tau ,s}^{\omega _{r} ,\omega _{r} } \frac{\pi ^{2}n^{2}}{L^{2} }+A_{66} J_{\tau ,s}^{\omega _{r} ,\omega _{r} } \frac{\pi ^{2}m^{2}}{R^{2}\varphi _{0}^{2} }. \end{aligned}$$

Appendix 2. Coefficients of the fundamental nucleus matrixes \({\mathbf{K}}_{\tau ,s}^{n,m,loc} \)for CLEC.

For \(\tau =1\) and \(s=1\)

$$\begin{aligned} K^{n,m,1,1}_{u_{x} ,u_{x} }= & {} 2h\pi ^{2}\left( {\frac{n^{2}C_{11} }{L^{2} }+\frac{m^{2}C_{44} }{R^{2}\varphi _{0}^{2} }} \right) , K^{n,m,1,1}_{u_{x} ,u_{\varphi } } = \frac{2hmn\pi ^{2}\left( {C_{12} +C_{44}^{T} } \right) }{LR\varphi _{0} }, K^{n,m,1,1}_{u_{x} ,u_{r} } =-\frac{2hn\pi C_{12} }{RL},\\ K^{n,m,1,1}_{u_{x} ,\omega _{x} }= & {} 0, K^{n,m,1,1}_{u_{x} ,\omega _{y} } =2h\left( {C_{55}^{T} -C_{55} } \right) , K^{n,m,1,1}_{u_{x} ,\omega _{z} } =\frac{2hm\pi \left( {C_{44} -C_{44}^{T} } \right) }{R\varphi _{0} },\\ K^{n,m,1,1}_{u_{\varphi } ,u_{x} }= & {} \frac{2hmn\pi ^{2}\left( {C_{12} +C_{44}^{T} } \right) }{LR\varphi _{0} }\left( {C_{12} +C_{44}^{T} } \right) , K^{n,m,1,1}_{u_{\varphi } ,u_{\varphi } } =\frac{2hC_{66} }{R^{2}}+2h\pi ^{2}\left( {\frac{n^{2}C_{44} }{L^{2} }+\frac{m^{2}C_{22} }{R^{2}\varphi _{0}^{2} }} \right) , \\ K^{n,m,1,1}_{u_{\varphi } ,\omega _{\varphi } }= & {} 0, K^{n,m,1,1}_{u_{\varphi } ,u_{r} } =-\frac{m\pi \left( {2hC_{22} +2hC_{66} } \right) }{R^{2}\varphi _{0} }, K^{n,m,1,1}_{u_{\varphi } ,\omega _{x} } =-\frac{2h\left( {-C_{66} +C_{66}^{T} } \right) }{R},\\ K^{n,m,1,1}_{u_{\varphi } ,\omega _{r} }= & {} \frac{2hn\pi \left( {C_{44}^{T} -C_{44} } \right) }{L}, K^{n,m,1,1}_{u_{r} ,u_{x} } =-\frac{2hn\pi C_{12} }{RL}, K^{n,m,1,1}_{u_{r} ,u_{\varphi } } =-\frac{2hm\pi \left( {C_{22} +C_{66} } \right) }{R^{2}\varphi _{0} },\\ K^{n,m,1,1}_{u_{r} ,u_{r} }= & {} \frac{2hC_{22} }{R^{2}}+\frac{2hn^{2}\pi ^{2}C_{55} }{L^{2} }+\frac{2hm^{2}\pi ^{2}C_{66} }{R^{2}\varphi _{0}^{2} },\\ K^{n,m,1,1}_{u_{r} ,\omega _{x} }= & {} \frac{2hm\pi \left( {C_{66} -C_{66}^{T} } \right) }{R\varphi _{0} }J_{\tau ,s}^{u_{r} ,\omega _{x} } , K^{n,m,1,1}_{u_{r} ,\omega _{\varphi } } {=}\frac{2hn\pi \left( {C_{55} -C_{55}^{T} } \right) }{L}, K^{n,m,1,1}_{u_{r} ,\omega _{r} } =0, \\ K^{n,m,1,1}_{\omega _{x} ,u_{x} }= & {} 0, K^{n,m,1,1}_{\omega _{x} ,u_{\varphi } } =\frac{2h\left( {C_{66} -C_{66}^{T} } \right) }{R}, \quad L^{n,m,1,1}_{\omega _{x} ,u_{r} } {=}\frac{2hm\pi \left( {C_{66}^{T} -C_{66} } \right) }{R\varphi _{0} }, \\ K^{n,m,1,1}_{\omega _{x} ,\omega _{x} }= & {} 2h\left( {2\left( {C_{66} -C_{66}^{T} } \right) +\pi ^{2}\left( {\frac{n^{2}A_{11} }{L^{2} }+\frac{m^{2}A_{44} }{R^{2}\varphi _{0}^{2} }} \right) } \right) , K^{n,m,1,1}_{\omega _{x} ,\omega _{\varphi } } =\frac{2hmn\pi ^{2}\left( {A_{12} +A_{44}^{T} } \right) }{LR\varphi _{0} },\\ K^{n,m,1,1}_{\omega _{x} ,\omega _{r} }= & {} \frac{2hn\pi A_{12} }{RL}, K^{n,m,1,1}_{\omega _{\varphi } ,u_{x} } =0,\\ K^{n,m,1,1}_{\omega _{\varphi } ,u_{\varphi } }= & {} 0, \quad K^{n,m,1,1}_{\omega _{\varphi } ,u_{r} } =\frac{2hn\pi \left( {C_{55}^{T} -C_{55} } \right) }{L}, K^{n,m,1,1}_{\omega _{\varphi } ,\omega _{x} } =\frac{2hmn\pi ^{2}\left( {A_{12} +A_{44}^{T} } \right) }{LR\varphi _{0} },\\ K^{n,m,1,1}_{\omega _{\varphi } ,\omega _{\varphi } }= & {} \frac{2hA_{66} }{R^{2}}+2h\left( {2C_{55} -2C_{55}^{T} +\pi ^{2}\left( {\frac{n^{2}A_{44} }{L^{2} }+\frac{m^{2}A_{22} }{R^{2}\varphi _{0}^{2} }} \right) } \right) , K^{n,m,1,1}_{\omega _{\varphi } ,\omega _{r} } =\frac{2hm\pi A_{66} }{R^{2}\varphi _{0} }, \\ K^{n,m,1,1}_{\omega _{r} ,u_{x} }= & {} \frac{2hm\pi \left( {C_{44}^{T} -C_{44} } \right) }{R\varphi _{0} }, K^{n,m,1,1}_{\omega _{r} ,u_{\varphi } } =\frac{2hn\pi \left( {C_{44} -C_{44}^{T} } \right) }{L}, K^{n,m,1,1}_{\omega _{r} ,u_{r} } =0, K^{n,m,1,1}_{\omega _{r} ,\omega _{x} } =\frac{2hn\pi A_{12} }{RL},\\ K^{n,m,1,1}_{\omega _{r} ,\omega _{\varphi } }= & {} \frac{2hn\pi A_{12} }{RL}, K^{n,m,1,1}_{\omega _{r} ,\omega _{r} } =\frac{2hA_{22} }{R^{2}}+4h\left( {C_{44} -C_{44}^{T} } \right) +\frac{2hn^{2}\pi ^{2}A_{55} }{L^{2} }+\frac{2hm^{2}\pi ^{2}A_{66} }{R^{2}\varphi _{0}^{2} }. \end{aligned}$$

For \(\tau =1\) and \(s=2\)

$$\begin{aligned} K^{n,m,1,2}_{u_{x} ,u_{x} }= & {} 0, K^{n,m,1,2}_{u_{x} ,u_{\varphi } } =0, K^{n,m,1,2}_{u_{x} ,u_{r} } =-\frac{2hn\pi C_{13} }{L}, K^{n,m,1,2}_{u_{x} ,\omega _{x} } =0, K^{n,m,1,2}_{u_{x} ,\omega _{y} } =0, K^{n,m,1,2}_{u_{x} ,\omega _{z} } =0, \\ K^{n,m,1,2}_{u_{\varphi } ,u_{x} }= & {} 0, K^{n,m,1,2}_{u_{\varphi } ,u_{\varphi } } =-\frac{2hC_{66}^{T} }{R}, K^{n,m,1,2}_{u_{\varphi } ,u_{r} } =-\frac{2hm\pi C_{23} }{R\varphi _{0} }, K^{n,m,1,2}_{u_{\varphi } ,\omega _{x} } =0, K^{n,m,1,2}_{u_{\varphi } ,\omega _{\varphi } } =0, K^{n,m,1,2}_{u_{\varphi } ,\omega _{r} } =0, \\ K^{n,m,1,2}_{u_{r} ,u_{x} }= & {} \frac{2hn\pi C_{55}^{T} }{L}, K^{n,m,1,2}_{u_{r} ,u_{\varphi } } =\frac{2hm\pi C_{66}^{T} }{R\varphi _{0} }, K^{n,m,1,2}_{u_{r} ,u_{r} } =\frac{2hC_{23} }{R}, K^{n,m,1,2}_{u_{r} ,\omega _{r} } =0, K^{n,m,1,2}_{u_{r} ,\omega _{x} } =0, K^{n,m,1,2}_{u_{r} ,\omega _{\varphi } } =0, \\ K^{n,m,1,2}_{\omega _{x} ,u_{x} }= & {} 0, K^{n,m,1,2}_{\omega _{x} ,u_{\varphi } } =2h\left( {C_{66} -C_{66}^{T} } \right) , \quad L^{n,m,1,2}_{\omega _{x} ,u_{r} } =0, K^{n,1,2}_{\omega _{x} ,\omega _{x} } =0, K^{n,m,0,1}_{\omega _{x} ,\omega _{\varphi } } =0, K^{n,m,1,2}_{\omega _{x} ,\omega _{r} } =\frac{2hn\pi A_{13} }{L}, \\ K^{n,m,1,2}_{\omega _{\varphi } ,u_{x} }= & {} 2h\left( {C_{55}^{T} -C_{55} } \right) , K^{n,m,1,2}_{\omega _{\varphi } ,u_{\varphi } } =0, \quad K^{n,m,1,2}_{\omega _{\varphi } ,u_{r} } =0, K^{n,m,1,2}_{\omega _{\varphi } ,\omega _{x} } =0, K^{n,m,1,2}_{\omega _{\varphi } ,\omega _{\varphi } } =\frac{2hm\pi A_{23} }{R\varphi _{0} }, K^{n,m,1,2}_{\omega _{\varphi } ,\omega _{r} } =-\frac{2hA_{66}^{T} }{R}, \\ K^{n,m,1,2}_{\omega _{r} ,u_{x} }= & {} 0, K^{n,m,0,1}_{\omega _{r} ,u_{\varphi } } =0, K^{n,m,1,2}_{\omega _{r} ,u_{r} } =0, K^{n,m,1,2}_{\omega _{r} ,\omega _{x} } =-\frac{2hn\pi A_{55}^{T} }{L}, K^{n,m,1,2}_{\omega _{r} ,\omega _{\varphi } } =-\frac{2hm\pi A_{66}^{T} }{R\varphi _{0} }, K^{n,m,1,2}_{\omega _{r} ,\omega _{r} } =\frac{2hA_{23} }{R}. \end{aligned}$$

]For \(\tau =2\) and \(s=1\)

$$\begin{aligned} K^{n,m,2,1}_{u_{x} ,u_{x} }= & {} 0, K^{n,m,2,1}_{u_{x} ,u_{\varphi } } =0, K^{n,m,2,1}_{u_{x} ,u_{r} } =\frac{2hn\pi C_{55}^{T} }{L}, K^{n,m,2,1}_{u_{x} ,\omega _{x} } =0, K^{n,m,2,1}_{u_{x} ,\omega _{y} } =0, K^{n,m,2,1}_{u_{x} ,\omega _{z} } =0, \\ K^{n,m,2,1}_{u_{\varphi } ,u_{x} }= & {} 0, K^{n,m,2,1}_{u_{\varphi } ,u_{\varphi } } =-\frac{2hC_{66}^{T} }{R}, K^{n,m,2,1}_{u_{\varphi } ,u_{r} } =\frac{2hm\pi C_{66}^{T} }{R\varphi _{0} } K^{n,m,2,1}_{u_{\varphi } ,\omega _{x} } =2h\left( {C_{66} -C_{66}^{T} } \right) , K^{n,m,2,1}_{u_{\varphi } ,\omega _{\varphi } } =0, K^{n,m,2,1}_{u_{\varphi } ,\omega _{r} } =0, \\ K^{n,m,2,1}_{u_{r} ,u_{x} }= & {} -\frac{2hn\pi C_{13} }{L}, K^{n,m,2,1}_{u_{r} ,u_{\varphi } } =-\frac{2hm\pi C_{23} }{R\varphi _{0} }, K^{n,m,2,1}_{u_{r} ,u_{r} } =\frac{2hC_{23} }{R}, K^{n,m,2,1}_{u_{r} ,\omega _{r} } =0, K^{n,m,2,1}_{u_{r} ,\omega _{x} } =0, K^{n,m,2,1}_{u_{r} ,\omega _{\varphi } } =0, \\ K^{n,m,2,1}_{\omega _{x} ,u_{x} }= & {} 0, K^{n,m,2,1}_{\omega _{x} ,u_{\varphi } } =0, \quad L^{n,m,2,1}_{\omega _{x} ,u_{r} } =0, K^{n,m,2,1}_{\omega _{x} ,\omega _{x} } =0, K^{n,m,2,1}_{\omega _{x} ,\omega _{\varphi } } =0, K^{n,m,2,1}_{\omega _{x} ,\omega _{r} } =-\frac{2hn\pi A_{55}^{T} }{L}, \\ K^{n,m,2,1}_{\omega _{\varphi } ,u_{x} }= & {} 0, K^{n,m,2,1}_{\omega _{\varphi } ,u_{\varphi } } =0, \quad K^{n,m,2,1}_{\omega _{\varphi } ,u_{r} } =0, K^{n,m,2,1}_{\omega _{\varphi } ,\omega _{x} } =0, K^{n,m,2,1}_{\omega _{\varphi } ,\omega _{\varphi } } =-\frac{2hA_{66}^{T} }{R}, K^{n,m,2,1}_{\omega _{\varphi } ,\omega _{r} } =-\frac{2hm\pi A_{66}^{T} }{R\varphi _{0} }, \\ K^{n,m,2,1}_{\omega _{r} ,u_{x} }= & {} 0, K^{n,m,2,1}_{\omega _{r} ,u_{\varphi } } =0, K^{n,m,2,1}_{\omega _{r} ,u_{r} } =0, K^{n,m,2,1}_{\omega _{r} ,\omega _{x} } =\frac{2hn\pi A_{13} }{L}, K^{n,m,2,1}_{\omega _{r} ,\omega _{\varphi } } =\frac{2hm\pi A_{23} }{R\varphi _{0} }, K^{n,m,2,1}_{\omega _{r} ,\omega _{r} } =\frac{2hA_{23} }{R}. \end{aligned}$$

For \(\tau =2\) and \(s=2\)

$$\begin{aligned} K^{n,m,2,2}_{u_{x} ,u_{x} }= & {} 2hC_{55} +\frac{2}{3}h^{3}\pi ^{2}\left( {\frac{n^{2}C_{11} }{L^{2}}+\frac{m^{2}C_{44} }{R^{2}\varphi _{0}^{2} }} \right) , K^{n,m,2,2}_{u_{x} ,u_{\varphi } } =\frac{2h^{3}mn\pi ^{2}\left( {C_{12} +C_{44}^{T} } \right) }{3LR\varphi _{0} }, K^{n,m,2,2}_{u_{x} ,u_{r} } =-\frac{2h^{3}n\pi C_{12} }{3LR}, K^{n,m,2,2}_{u_{x} ,\omega _{x} } =0,\\ K^{n,m,2,2}_{u_{x} ,\omega _{y} }= & {} \frac{2}{3}h^{3}\left( {C_{55}^{T} -C_{55} } \right) , K^{n,m,2,2}_{u_{x} ,\omega _{z} } =\frac{2h^{3}m\pi \left( {C_{44} -C_{44}^{T} } \right) }{3R\varphi _{0} }, \\ K^{n,m,2,2}_{u_{\varphi } ,u_{x} }= & {} \frac{2h^{3}mn\pi ^{2}\left( {C_{12} +C_{44}^{T} } \right) }{3LR\varphi _{0} }, K^{n,m,2,2}_{u_{\varphi } ,u_{\varphi } } =\left( {2h+\frac{2h^{3}}{3R^{2}}} \right) C_{66} +\frac{2}{3}h^{3}\pi ^{2}\left( {\frac{n^{2}C_{44} }{L^{2}}+\frac{m^{2}C_{22} }{R^{2}\varphi _{0}^{2} }} \right) , K^{n,m,2,2}_{u_{\varphi } ,\omega _{\varphi } } =0,\\ K^{n,m,2,2}_{u_{\varphi } ,u_{r} }= & {} -\frac{2h^{3}m\pi \left( {C_{22} +C_{66} } \right) }{3R^{2}\varphi _{0} }, K^{n,m,2,2}_{u_{\varphi } ,\omega _{x} } =\frac{2h^{3}}{3R}\left( {C_{66} -C_{66}^{T} } \right) , K^{n,m,2,2}_{u_{\varphi } ,\omega _{r} } =\frac{2h^{3}n\pi }{3L}\left( {C_{44}^{T} -C_{44} } \right) , \\ K^{n,m,2,2}_{u_{r} ,u_{x} }= & {} -\frac{2h^{3}n\pi C_{12} }{3LR}, K^{n,m,2,2}_{u_{r} ,u_{\varphi } } =-\frac{2h^{3}m\pi \left( {C_{22} +C_{66} } \right) }{3R^{2}\varphi _{0} }, K^{n,m,2,2}_{u_{r} ,\omega _{x} } =\frac{2h^{3}\pi m}{3R\varphi _{0} }\left( {C_{66} -C_{66}^{T} } \right) ,\\ K^{n,m,2,2}_{u_{r} ,u_{r} }= & {} \frac{2h^{3}C_{22} }{3R^{2}}+2hC_{33} +\frac{2h^{3}n^{2}\pi ^{2}C_{55} }{3L^{2}}+\frac{2h^{3}m^{2}\pi ^{2}C_{66} }{3R^{2}\varphi _{0}^{2} }, K^{n,m,2,2}_{u_{r} ,\omega _{\varphi } } =\frac{2h^{3}\pi n}{3L}\left( {C_{55} -C_{55}^{T} } \right) , \quad K^{n,m,2,2}_{u_{r} ,\omega _{r} } =0, \\ K^{n,m,2,2}_{\omega _{x} ,u_{x} }= & {} 0, K^{n,m,2,2}_{\omega _{x} ,u_{\varphi } } =\frac{2h^{3}\left( {C_{66} -C_{66}^{T} } \right) }{3R}, \quad L^{n,m,2,2}_{\omega _{x} ,u_{r} } =\frac{2h^{3}\pi m\left( {C_{66}^{T} -C_{66} } \right) }{3R\varphi _{0} }, K^{n,m,2,2}_{\omega _{x} ,\omega _{\varphi } } =\frac{2h^{3}\pi ^{2}nm\left( {A_{12} +A_{44}^{T} } \right) }{3LR\varphi _{0} }, \\ K^{n,2,2}_{\omega _{x} ,\omega _{x} }= & {} 2hA_{55} +\frac{2}{3}h^{3}\left( {2C_{66} -2C_{66}^{T} +\pi ^{2}\left( {\frac{n^{2}A_{11} }{L^{2}}+\frac{m^{2}A_{44} }{R^{2}\varphi _{0}^{2} }} \right) } \right) , K^{n,m,2,2}_{\omega _{x} ,\omega _{r} }= \frac{2h^{3}\pi nA_{12} }{3LR}, \\ K^{n,m,2,2}_{\omega _{\varphi } ,u_{x} }= & {} 0, K^{n,m,2,2}_{\omega _{\varphi } ,u_{\varphi } } =0, \quad K^{n,m,2,2}_{\omega _{\varphi } ,u_{r} } =\frac{2h^{3}n\pi \left( {C_{55}^{T} -C_{55} } \right) }{3L}, K^{n,m,2,2}_{\omega _{\varphi } ,\omega _{x} } =\frac{2h^{3}\pi ^{2}nm\left( {A_{12} +A_{44}^{T} } \right) }{3LR\varphi _{0} },\\ K^{n,m,2,2}_{\omega _{\varphi } ,\omega _{\varphi } }= & {} \left( {2h+\frac{2h^{3}}{3R^{2}}} \right) A_{66} +\frac{2}{3}h^{3}\left( {2C_{55} -2C_{55}^{T} +\pi ^{2}\left( {\frac{n^{2}A_{44} }{L^{2}}+\frac{m^{2}A_{22} }{R^{2}\varphi _{0}^{2} }} \right) } \right) , K^{n,m,2,2}_{\omega _{\varphi } ,\omega _{r} } =\frac{2h^{3}\pi mA_{66} }{3R^{2}\varphi _{0} }, \\ K^{n,m,2,2}_{\omega _{r} ,u_{x} }= & {} \frac{2h^{3}\pi m\left( {C_{44}^{T} -C_{44} } \right) }{3R\varphi _{0} }, K^{n,m,2,2}_{\omega _{r} ,u_{\varphi } } =\frac{2h^{3}\pi n\left( {C_{44}^{T} -C_{44} } \right) }{3L}, K^{n,m,2,2}_{\omega _{r} ,u_{r} } =0, K^{n,m,2,2}_{\omega _{r} ,\omega _{x} } =\frac{2h^{3}\pi nA_{12} }{3LR},\\ K^{n,m,2,2}_{\omega _{r} ,\omega _{\varphi } }= & {} \frac{2h^{3}m\pi \left( {A_{22} +A_{66} } \right) }{3R^{2}\varphi _{0} }, K^{n,m,2,2}_{\omega _{r} ,\omega _{r} } =\frac{2h^{3}A_{22} }{3R^{2}}+2hA_{33} +\frac{2h^{3}\pi ^{2}n^{2}A_{55} }{3L^{2}}+\frac{4h^{3}}{3}\left( {C_{44} -C_{44}^{T} } \right) +\frac{2h^{3}\pi ^{2}m^{2}A_{66} }{3R^{2}\varphi _{0}^{2} }. \end{aligned}$$

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Carrera, E., Zozulya, V.V. Analytical solution for the micropolar cylindrical shell: Carrera unified formulation (CUF) approach. Continuum Mech. Thermodyn. (2022). https://doi.org/10.1007/s00161-022-01156-x

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s00161-022-01156-x

Keywords

Search

Navigation