Skip to main content
SpringerLink
Log in

Continuum model of fractured media in direct and inverse seismic problems

  • Original Article
  • Published:
Continuum Mechanics and Thermodynamics Aims and scope Submit manuscript

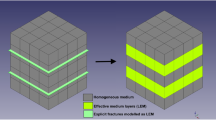
Abstract

A numerical modeling of a seismic survey is an important part of modern geological exploration process. Novel algorithms for seismic inverse problems are capable of handling heterogeneous geological media but require high quality results of direct problem modeling. The most difficult object in the forward modeling of the geological media is the fractured inclusion. Different approaches exist to describe its dynamic behavior, mostly limited to the linear contact conditions on crack boundaries. This work presents the nonlinear continuum model of the layered medium with visco-plastic interlayers and adopts it to the dynamic problem of wave propagation. The model relies on the linear isotropic theory and the theory of periodic media. The slip velocity vector and the delamination velocity vector are treated as continuous functions of time and coordinates. The formulated system in partial derivatives is semi-linear and contains small parameter in the denominator of the free term. To prevent the oscillation occurrence on the explicit finite-difference schemes, a novel explicit-implicit method is proposed in this paper. The method splits the problem into the elastic part and the correction procedure at each time step. The method is used to generate synthetic day surface data for the Marmousi II model containing the fractured inclusion. Deep convolutional neural networks are used to provide a fast solution for inverse problem of restoring the spatial position of the fractured inclusion based on the surface measurements.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

References

  1. Li, Y., Alkhalifah, T.: Multi-parameter reflection waveform inversion for acoustic transversely isotropic media with a vertical symmetry axis. Geophys. Prospect. 68, 1878–1892 (2020). https://doi.org/10.1111/1365-2478.12966

    Article  ADS  Google Scholar 

  2. Takougang, E., Ali, M., Bouzidi, Y., Bouchaala, F., Sultan, A., Mohamed, A.: Characterization of a carbonate reservoir using elastic full-waveform inversion of vertical seismic profile data. Geophys. Prospect. 68, 1944–1957 (2020). https://doi.org/10.1111/1365-2478.12962

    Article  ADS  Google Scholar 

  3. Malovichko, M., Khokhlov, N., Yavich, N., Zhdanov, M.: Incorporating known petrophysical model in the seismic full-waveform inversion using the gramian constraint. Geophys. Prospect. 68, 1361–1378 (2020). https://doi.org/10.1111/1365-2478.12932

    Article  ADS  Google Scholar 

  4. Alali, A., Sun, B., Alkhalifah, T.: The effectiveness of a pseudo-inverse extended born operator to handle lateral heterogeneity for imaging and velocity analysis applications. Geophys. Prospect. 68, 1154–1166 (2019). https://doi.org/10.1111/1365-2478.12916

    Article  ADS  Google Scholar 

  5. Golubev, V., Khokhlov, N., Nikitin, I., Churyakov, M.: Application of compact grid-characteristic schemes for acoustic problems. J. Phys. Conf. Ser. 1479 (2020). https://doi.org/10.1088/1742-6596/1479/1/012058

  6. Golubev, V.I., Shevchenko, A.V., Khokhlov, N.I., Nikitin, I.S.: Numerical investigation of compact grid-characteristic schemes for acoustic problems. J. Phys. Conf. Ser. 1902(1), 012110 (2021). https://doi.org/10.1088/1742-6596/1902/1/012110

    Article  Google Scholar 

  7. Favorskaya, A.V., Zhdanov, M.S., Khokhlov, N.I., Petrov, I.B.: Modelling the wave phenomena in acoustic and elastic media with sharp variations of physical properties using the grid-characteristic method. Geophys. Prospect. 66, 1485–1502 (2018). https://doi.org/10.1111/1365-2478.12639

    Article  ADS  Google Scholar 

  8. Vershinin, A., Konovalov, D., Kukushkin, A., Levin, V.: Geomechanical modeling using variable order spectral element method at non-conformal meshes. (2021). https://doi.org/10.23967/wccm-eccomas.2020.154

  9. Vershinin, A.V., Levin, V.A., Zingerman, K.M., Sboychakov, A.M., Yakovlev, A.M.: Software for estimation of second order effective material properties of porous samples with geometrical and physical nonlinearity accounted for. Adv. Eng. Softw. 86, 80–84 (2015). https://doi.org/10.1016/j.advengsoft.2015.04.007

    Article  Google Scholar 

  10. Chentsov, E., Sadovskii, V., Sadovskaya, O.: Modeling of wave processes in a blocky medium with fluid-saturated porous interlayers. AIP Conf. Proc. 1895 (2017). https://doi.org/10.1063/1.5007396

  11. Golubev, V.I., Shevchenko, A.V., Petrov, I.B.: Application of the Dorovsky model for taking into account the fluid saturation of geological media. J. Phys. Conf. Ser. 1715(1), 012056 (2021). https://doi.org/10.1088/1742-6596/1715/1/012056

    Article  Google Scholar 

  12. Berryman, J.G.: Effective medium approximation for elastic constants of porous solids with microscopic heterogeneity. J. Appl. Phys. 59(4), 1136–1140 (1986). https://doi.org/10.1063/1.336550

    Article  ADS  Google Scholar 

  13. Levin, V.A., Lokhin, V.V., Zingerman, K.M.: Method of estimation of effective properties of porous bodies undergoing finite deformation. Int. J. Fract. 80(1), 9–12 (1996). https://doi.org/10.1007/BF00012435

    Article  Google Scholar 

  14. Levin, V.A., Vdovichenko, I.I., Vershinin, A.V., Yakovlev, M.Y., Zingerman, K.M.: An approach to the computation of effective strength characteristics of porous materials. Lett. Mater. 7, 452–454 (2017). https://doi.org/10.22226/2410-3535-2017-4-452-454

    Article  Google Scholar 

  15. De la Cruz, V., Spanos, T.J.T.: Seismic wave propagation in a porous medium. Geophysics 50(10), 1556–1565 (1985). https://doi.org/10.1190/1.1441846

    Article  ADS  Google Scholar 

  16. Levin, V.A., Lokhin, V.V., Zingerman, K.M.: Effective elastic properties of porous materials with randomly dispersed pores: finite deformation. J. Appl. Mech. 67, 667–670 (2000). https://doi.org/10.1115/1.1286287

    Article  ADS  MATH  Google Scholar 

  17. Levin, V.A., Zingermann, K.M.: Effective constitutive equations for porous elastic materials at finite strains and superimposed finite strains. J. Appl. Mech. 70, 809–816 (2003). https://doi.org/10.1115/1.1630811

    Article  ADS  MATH  Google Scholar 

  18. Placidi, L., Dell’Isola, F., Ianiro, N., Sciarra, G.: Variational formulation of pre-stressed solid-fluid mixture theory, with an application to wave phenomena. Eur. J. Mech. A Solids 27(4), 582–606 (2008). https://doi.org/10.1016/j.euromechsol.2007.10.003

    Article  ADS  MathSciNet  MATH  Google Scholar 

  19. Schoenberg, M.: Elastic wave behavior across linear slip interfaces. J. Acoust. Soc. Am. 68, 1516 (1980). https://doi.org/10.1121/1.385077

    Article  ADS  MATH  Google Scholar 

  20. Hsu, C., Schoenberg, M.: Elastic waves through a simulated fractured medium. Geophysics 58, 964–977 (1993). https://doi.org/10.1190/1.1443487

    Article  ADS  Google Scholar 

  21. Izvekov, O., Kondaurov, V.: Scattered fracture of porous materials with brittle skeleton. Mech. Solids 45, 445–464 (2010). https://doi.org/10.3103/S0025654410030155

    Article  ADS  Google Scholar 

  22. Barchiesi, E., Yang, H., Tran, C., Placidi, L., Müller, W.: Computation of brittle fracture propagation in strain gradient materials by the fenics library. Math. Mech. Solids 26(3), 325–340 (2021). https://doi.org/10.1177/1081286520954513

    Article  MathSciNet  MATH  Google Scholar 

  23. Placidi, L., Barchiesi, E.: Energy approach to brittle fracture in strain-gradient modelling. Proc Math. Phys. Eng. Sci. 474(2210), (2018). https://doi.org/10.1098/rspa.2017.0878

  24. Scala, I., Rosi, G., Placidi, L., Nguyen, V.-H., Naili, S.: Effects of the microstructure and density profiles on wave propagation across an interface with material properties. Continuum Mech. Thermodyn. 31, 1165–1180 (2019). https://doi.org/10.1007/s00161-018-0740-9

    Article  ADS  MathSciNet  Google Scholar 

  25. Rosi, G., Placidi, L., Nguyen, V.-H., Naili, S.: Wave propagation across a finite heterogeneous interphase modeled as an interface with material properties. Mech. Res. Commun. 84, 43–48 (2017). https://doi.org/10.1016/J.MECHRESCOM.2017.06.004

    Article  Google Scholar 

  26. Placidi, L., Rosi, G., Giorgio, I., Madeo, A.: Reflection and transmission of plane waves at surfaces carrying material properties and embedded in second-gradient materials. Math. Mech. Solids 19, 555–578 (2014). https://doi.org/10.1177/1081286512474016

    Article  MathSciNet  MATH  Google Scholar 

  27. Dell’isola, F., Madeo, A., Placidi, L.: Linear plane wave propagation and normal transmission and reflection at discontinuity surfaces in second gradient 3d continua. ZAMM J. Appl. Math. Mech./ Zeitschrift für Angewandte Mathematik und Mechanik 92 (2012). https://doi.org/10.1002/zamm.201100022

  28. Pan, X., Zhang, G.: Estimation of fluid indicator and dry fracture compliances using azimuthal seismic reflection data in a gas-saturated fractured reservoir. J. Petrol. Sci. Eng. 167, 737–751 (2018). https://doi.org/10.1016/j.petrol.2018.04.054

    Article  Google Scholar 

  29. Fang, X., Zheng, Y., Fehler, M.: Fracture clustering effect on amplitude variation with offset and azimuth analyses. Geophysics 82(1), 13–25 (2017). https://doi.org/10.1190/geo2016-0045.1

    Article  Google Scholar 

  30. Chen, H., Chen, T., Innanen, K.A.: Estimating tilted fracture weaknesses from azimuthal differences in seismic amplitude data. Geophysics 85(3), 135–146 (2020). https://doi.org/10.1190/geo2019-0344.1

    Article  ADS  Google Scholar 

  31. Sadovskii, V.M., Sadovskaya, O.V., Lukyanov, A.A.: Modeling of wave processes in blocky media with porous and fluid-saturated interlayers. J. Comput. Phys. 345, 834–855 (2017). https://doi.org/10.1016/j.jcp.2017.06.001

    Article  ADS  MathSciNet  MATH  Google Scholar 

  32. Budiansky, B., O’Connell, R.J.: Seismic velocities in dry and saturated cracked solids. J. Geophys. Res. 79, 5412–5426 (1974). https://doi.org/10.1029/JB079i035p05412

    Article  ADS  Google Scholar 

  33. Hudson, J.A., Pointer, T., Liu, E.: Effective-medium theories for fluid-saturated materials with aligned cracks. Geophys. Prospect. 49, 509–522 (2001). https://doi.org/10.1046/j.1365-2478.2001.00272.x

    Article  ADS  Google Scholar 

  34. Grechka, V., Kachanov, M.: Seismic characterisation of multiple fracture sets: Does orthotropy suffice? Geophysics 71(3), 93–105 (2006). https://doi.org/10.1190/1.2196872

    Article  Google Scholar 

  35. Batdorf, S.B., Budiansky, B.: Polyaxial stress–strain relations of strain-hardening metal. J. Appl. Mech. 21(4), 323–326 (1954). https://doi.org/10.1115/1.4010929

    Article  ADS  Google Scholar 

  36. Timofeev, D., Barchiesi, E., Misra, A.K., Placidi, L.: Hemivariational continuum approach for granular solids with damage-induced anisotropy evolution. Math. Mech. Solids 26, 738–770 (2020). https://doi.org/10.1177/1081286520968149

    Article  MathSciNet  MATH  Google Scholar 

  37. Placidi, L., Barchiesi, E., Misra, A.K., Timofeev, D.: Micromechanics-based elasto-plastic-damage energy formulation for strain gradient solids with granular microstructure. Contin. Mech. Thermodyn. 1–29 (2021). https://doi.org/10.1007/s00161-021-01023-1

  38. Maksimov, V., Barchiesi, E., Misra, A.K., Placidi, L., Timofeev, D.: Two-dimensional analysis of size effects in strain-gradient granular solids with damage-induced anisotropy evolution. J. Eng. Mech. (2021). https://doi.org/10.1061/(ASCE)EM.1943-7889.0002010

  39. Bakulin, A.: Intrinsic and layer-induced vertical transverse isotropy. Geophysics 68(5), 1708–1713 (2003). https://doi.org/10.1190/1.1620644

    Article  ADS  Google Scholar 

  40. Schoenberg, M., Sayers, C.: Seismic anisotropy of fractured rock. Geophysics 60, 204–211 (1995). https://doi.org/10.1190/1.1443748

    Article  ADS  Google Scholar 

  41. Davy, P., Darcel, C., Romain, L.G., Diego, M.I.: Elastic properties of fractured rock masses with frictional properties and power-law fracture size distributions. J. Geophys. Res. Solid Earth 123(8), 6521–6539 (2018). https://doi.org/10.1029/2017JB015329

    Article  ADS  Google Scholar 

  42. Kachanov, M.: A microcrack model of rock inelasticity. Mech. Mater. 1, 19–41 (1982). https://doi.org/10.1016/0167-6636(82)90021-7

    Article  Google Scholar 

  43. Molotkov, L.A., Bakulin, A.V.: An effective model of a fractured medium with fractures modeled by the surfaces of discontinuity of displacements. J. Math. Sci. 86(3), 2735–2746 (1997). https://doi.org/10.1007/BF02355164

    Article  MathSciNet  Google Scholar 

  44. Morland, L.W.: Continuum model of regularly jointed mediums. J. Geophys. Res. 79(3), 357–362 (1974). https://doi.org/10.1029/JB079i002p00357

    Article  ADS  Google Scholar 

  45. Nikitin, I.S.: Dynamic models of layered and block media with slip, friction and separation. Mech. Solids 43(4), 652–661 (2008). https://doi.org/10.3103/S0025654408040134

    Article  ADS  Google Scholar 

  46. Favorskaya, A., Golubev, V.: Study of anisotropy of seismic response from fractured media. Smart Innov. Syst. Technol. 238, 231–240 (2021). https://doi.org/10.1007/978-981-16-2765-1_19

    Article  Google Scholar 

  47. Golubev, V., Nikitin, I., Golubeva, Y., Petrov, I.: Numerical simulation of the dynamic loading process of initially damaged media. AIP Conf. Proc. 2309, 020006 (2020). https://doi.org/10.1063/5.0033949

    Article  Google Scholar 

  48. Golubev, V., Nikitin, I., Ekimenko, A.: Simulation of seismic responses from fractured marmousi2 model. AIP Conf. Proc. 2312, 050006 (2020). https://doi.org/10.1063/5.0035495

    Article  Google Scholar 

  49. Misra, A.K.: Mechanistic model for contact between rough surfaces. J. Eng. Mech-ASCE 123, 475–484 (1997). https://doi.org/10.1061/(ASCE)0733-9399(1997)123:5(475)

    Article  Google Scholar 

  50. Misra, A.K.: Effect of asperity damage on shear behavior of single fracture. Eng. Fract. Mech. 69, 1997–2014 (2002). https://doi.org/10.1016/S0013-7944(02)00073-5

    Article  Google Scholar 

  51. Misra, A.K., Marangos, O.: Rock-joint micromechanics: relationship of roughness to closure and wave propagation. Int. J. Geomech. 11, 431–439 (2010). https://doi.org/10.1061/(ASCE)GM.1943-5622.0000021

    Article  Google Scholar 

  52. Misra, A.K., Poorsolhjouy, P.: Granular micromechanics based micromorphic model predicts frequency band gaps. Continuum Mech. Thermodyn. 28, 215–234 (2016). https://doi.org/10.1007/s00161-015-0420-y

    Article  ADS  MathSciNet  MATH  Google Scholar 

  53. Misra, A.K., Nejadsadeghi, N.: Longitudinal and transverse elastic waves in 1D granular materials modeled as micromorphic continua. Wave Motion (2019). https://doi.org/10.1016/j.wavemoti.2019.05.005

  54. Bakhvalov, N.S., Panasenko, G.: Homogenisation: Averaging Processes in Periodic Media: Mathematical Problems in the Mechanics of Composite Materials. Springer, Dordrecht (1989)

    MATH  Google Scholar 

  55. Burago, N.G., Nikitin, I.S.: Improved model of a layered medium with slip on the contact boundaries. J. Appl. Math. Mech. 80(2), 164–172 (2016). https://doi.org/10.1016/j.jappmathmech.2016.06.010

    Article  MathSciNet  MATH  Google Scholar 

  56. Nikitin, I.S., Golubev, V.I.: Explicit–implicit schemes for calculating thedynamics of layered media with nonlinear conditions at contact boundaries. J. Siber. Federal Univ. Math. Phys. 14(6), 768–778 (2021). https://doi.org/10.17516/1997-1397-2021-14-6-768-778

    Article  MATH  Google Scholar 

  57. Martin, G.S., Wiley, R., Marfurt, K.J.: Marmousi2: an elastic upgrade for marmousi. Lead. Edge 25(2), 156–166 (2006). https://doi.org/10.1190/1.2172306

    Article  Google Scholar 

  58. Waldeland, A.U., Solberg, A.H.S.S.: Salt classification using deep learning. In: 79th EAGE Conference and Exhibition 2017, vol. 2017, pp. 1–5 (2017). https://doi.org/10.3997/2214-4609.201700918

  59. Waldeland, A.U., Jensen, A.C., Gelius, L.-J., Solberg, A.H.S.: Convolutional neural networks for automated seismic interpretation. Geophysics 37(7), 529–537 (2018). https://doi.org/10.1190/tle37070529.1

    Article  Google Scholar 

  60. Shi, Y., Wu, X., Fomel, S.: Automatic salt-body classification using deep-convolutional neural network, pp. 1971–1975 (2018). https://doi.org/10.1190/segam2018-2997304.1

  61. Zhao, T.: Seismic facies classification using different deep convolutional neural networks, pp. 2046–2050 (2018). https://doi.org/10.1190/segam2018-2997085.1

  62. Yang, F., Ma, J.: Deep-learning inversion: a next-generation seismic velocity model building method. Geophysics 84(4), 583–599 (2019). https://doi.org/10.1190/geo2018-0249.1

    Article  ADS  Google Scholar 

  63. Das, V., Pollack, A., Wollner, U., Mukerji, T.: Convolutional neural network for seismic impedance inversion. Geophysics 84(6), 869–880 (2019). https://doi.org/10.1190/geo2018-0838.1

    Article  ADS  Google Scholar 

  64. Dujardin, J.R., Sauvin, G., Vanneste, M.: Acoustic impedance inversion of high resolution marine seismic data with deep neural network. In: NSG2020 4th Applied Shallow Marine Geophysics Conference Proceedings, vol. 2020, pp. 1–5 (2020). https://doi.org/10.3997/2214-4609.202020169

  65. Araya-Polo, M., Farris, S., Florez, M.: Deep learning-driven velocity model building workflow. Geophysics 38(11) (2019). https://doi.org/10.1190/tle38110872a1.1

  66. Park, M.J., Sacchi, M.D.: Automatic velocity analysis using convolutional neural network and transfer learning. Geophysics 85(1) (2020). https://doi.org/10.1190/geo2018-0870.1

  67. Ronneberger, O., Fischer, P., Brox, T.: U-Net: Convolutional Networks for Biomedical Image Segmentation. Preprint at arxiv:1505.04597 (2015)

  68. Kingma, D.P., Ba, J.: Adam: A method for stochastic optimization. Preprint at arxiv:1412.6980 (2014)

Download references

Acknowledgements

This work was carried out with the financial support of the Russian Science Foundation, Project No. 19-71-10060, https://rscf.ru/en/project/19-71-10060/.

Author information

Author notes

  1. Alexey Vasykov, Ilia Nikitin, Andrey Stankevich, Igor Petrov have contributed equally to this work.

Authors and Affiliations

  1. Laboratory of Applied Numerical Geophysics, Moscow Institute of Physics and Technology, Institutsky lane 9, Dolgoprudny, Moscow Region, Russia, 141700

    Vasily Golubev, Alexey Vasykov, Andrey Stankevich & Igor Petrov

  2. Department of Mathematical Simulation, Institute of Computer Aided Design of RAS, 2-nd Brestskaya Str. 19/18, Moscow, Russia, 123056

    Vasily Golubev & Ilia Nikitin

Authors
  1. Vasily Golubev
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Alexey Vasykov
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Ilia Nikitin
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Andrey Stankevich
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Igor Petrov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Vasily Golubev.

Additional information

Communicated by Andreas Öchsner.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Golubev, V., Vasykov, A., Nikitin, I. et al. Continuum model of fractured media in direct and inverse seismic problems. Continuum Mech. Thermodyn. 35, 1459–1472 (2023). https://doi.org/10.1007/s00161-022-01149-w

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00161-022-01149-w

Keywords

Search

Navigation