Considerations on alternative solutions for stress analysis of anisotropic materials: a beam case study

Abstract

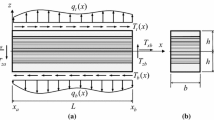
Anisotropic materials are those that the value of a property depends on the direction of analysis. This article addresses both analytical and numerical stress computation for orthotropic beams under normal and shear loads. The analytical solution is based on potential polynomial functions and the present work establishes general strategies which allow systematic evaluation of the polynomial coefficients. The numerical approximation uses an alternative formulation of finite volumes based on an area weighted average associated with parabolic interpolation functions. The numerical scheme is verified against results obtained for orthotropic beams using both the analytical method and a classical finite volume approximation. Assessment of the global error based on the $L_2$ norm indicates a high convergence rate and substantially smaller absolute differences when compared to the conventional finite volume approximation.

Appendix

This Appendix provides the coefficients for $\phi _p(y)$ of Eq. (14) for a function potential of maximum order $n=4$, corresponding to a prescribed normal load distribution equation at the beam boundaries with a maximum of second order. The coefficients for equations $\phi _{n-1}(y)$, $\phi _{n-2}(y)$, $\ldots$, $\phi _{n-4}(y)$ are obtained by back substitution from $\phi _{n}(y)$, which are jointly determined by using the boundary conditions. The coefficients are as follows:

Coefficients for $p=n$, $\phi _{n}(y)= \sum _{k=0}^{3} a_{n}^{k}y^k$ are

$$\begin{aligned} a_n^0, ~ a_n^1,~ a_n^2 \quad \text {and} \quad a_n^3 \end{aligned}$$

(23)

Coefficients for $p=n-1$, $\phi _{n-1}(y)= \sum _{k=0}^{4} a_{n-1}^{k}y^k$ are

$$\begin{aligned} a_{n-1}^{4}=\frac{1}{2} n \frac{s_{16}}{s_{11}}_n a^{3}_{n} , \end{aligned}$$

(24)

Coefficients for $p=n-2$, $\phi _{n-2}(y)= \sum _{k=0}^{5} a_{n-2}^{k}y^k$ are

$$\begin{aligned} \begin{array}{l} \displaystyle a_{n-2}^{4}=-\frac{1}{12} n (n-1) \left( 2\frac{s_{12}}{s_{11}} + \frac{s_{66}}{s_{11}} \right) a^{2}_{n} + \frac{1}{2}(n-1) \frac{s_{16}}{s_{11}} a^{3}_{n-1} \\ \displaystyle a_{n-2}^{5}=-\frac{1}{20} n (n-1) \left( 2\frac{s_{12}}{s_{11}} + \frac{s_{66}}{s_{11}} \right) a^{3}_{n} + \frac{2}{5}(n-1) \frac{s_{16}}{s_{11}} a^{4}_{n-1} \end{array} . \end{aligned}$$

(25)

Coefficients for $p=n-3$, $\phi _{n-3}(y)= \sum _{k=0}^{6} a_{n-3}^{k}y^k$ are

$$\begin{aligned} \begin{array}{l} \displaystyle a_{n-3}^{4}=\frac{1}{12} n (n-1) (n-2)\frac{s_{26}}{s_{11}}a^{1}_{n} -\frac{1}{12} (n-1)(n-2) \left( 2\frac{s_{12}}{s_{11}} + \frac{s_{66}}{s_{11}} \right) a^{2}_{n-1} \\ \qquad \qquad \displaystyle +\frac{1}{2}(n-2) \frac{s_{16}}{s_{11}} a^{3}_{n-2} \\ \displaystyle a_{n-3}^{5}=\frac{1}{30} n (n-1) (n-2)\frac{s_{26}}{s_{11}}a^{2}_{n} -\frac{1}{20} (n-1)(n-2) \left( 2\frac{s_{12}}{s_{11}} + \frac{s_{66}}{s_{11}} \right) a^{3}_{n-1} \\ \qquad \qquad \displaystyle +\frac{2}{5}(n-2) \frac{s_{16}}{s_{11}} a^{4}_{n-2} \\ \displaystyle a_{n-3}^{6}=\frac{1}{60} n (n-1) (n-2)\frac{s_{26}}{s_{11}}a^{3}_{n} -\frac{1}{30} (n-1)(n-2) \left( 2\frac{s_{12}}{s_{11}} + \frac{s_{66}}{s_{11}} \right) a^{4}_{n-1} \\ \qquad \qquad \displaystyle +\frac{1}{3}(n-2) \frac{s_{16}}{s_{11}} a^{5}_{n-2} \end{array} . \end{aligned}$$

(26)

Coefficients for $p=n-4$, $\phi _{n-4}(y)= \sum _{k=0}^{7} a_{n-4}^{k}y^k$ are

$$\begin{aligned} \begin{array}{l} \displaystyle a_{n-4}^{4}= -\frac{1}{24} n (n-1) (n-2) (n-3)\frac{s_{22}}{s_{11}}a^{0}_{n} +\frac{1}{12} (n-1) (n-2) (n-3)\frac{s_{26}}{s_{11}}a^{1}_{n-1} \\ \qquad \qquad \displaystyle -\frac{1}{12} (n-2)(n-3) \left( 2\frac{s_{12}}{s_{11}} + \frac{s_{66}}{s_{11}} \right) a^{2}_{n-2} + \frac{1}{2}(n-3) \frac{s_{16}}{s_{11}} a^{3}_{n-3} \\ \displaystyle a_{n-4}^{5}= -\frac{1}{120} n (n-1) (n-2) (n-3)\frac{s_{22}}{s_{11}}a^{1}_{n} +\frac{1}{30} (n-1) (n-2) (n-3)\frac{s_{26}}{s_{11}}a^{2}_{n-1} \\ \qquad \qquad \displaystyle -\frac{1}{20} (n-2)(n-3) \left( 2\frac{s_{12}}{s_{11}} + \frac{s_{66}}{s_{11}} \right) a^{3}_{n-2} + \frac{2}{5}(n-3) \frac{s_{16}}{s_{11}} a^{4}_{n-3} \\ \displaystyle a_{n-4}^{6}= -\frac{1}{360} n (n-1) (n-2) (n-3)\frac{s_{22}}{s_{11}}a^{2}_{n} +\frac{1}{60} (n-1) (n-2) (n-3)\frac{s_{26}}{s_{11}}a^{3}_{n-1} \\ \qquad \qquad \displaystyle -\frac{1}{30} (n-2)(n-3) \left( 2\frac{s_{12}}{s_{11}} + \frac{s_{66}}{s_{11}} \right) a^{4}_{n-2} + \frac{1}{3}(n-3) \frac{s_{16}}{s_{11}} a^{5}_{n-3} \\ \displaystyle a_{n-4}^{7}= -\frac{1}{840} n (n-1) (n-2) (n-3)\frac{s_{22}}{s_{11}}a^{3}_{n} +\frac{1}{105} (n-1) (n-2) (n-3)\frac{s_{26}}{s_{11}}a^{4}_{n-1} \\ \qquad \qquad \displaystyle -\frac{1}{42} (n-2)(n-3) \left( 2\frac{s_{12}}{s_{11}} + \frac{s_{66}}{s_{11}} \right) a^{5}_{n-2} + \frac{2}{7}(n-3) \frac{s_{16}}{s_{11}} a^{6}_{n-3} \end{array} . \end{aligned}$$

(27)