Skip to main content
SpringerLink
Log in

Symmetry Breaking in the Periodic Thomas–Fermi–Dirac–von Weizsäcker Model

  • Published:
Annales Henri Poincaré Aims and scope Submit manuscript

Abstract

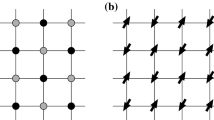
We consider the Thomas–Fermi–Dirac–von Weizsäcker model for a system composed of infinitely many nuclei placed on a periodic lattice and electrons with a periodic density. We prove that if the Dirac constant is small enough, the electrons have the same periodicity as the nuclei. On the other hand, if the Dirac constant is large enough, the 2-periodic electronic minimizer is not 1-periodic, and hence, symmetry breaking occurs. We analyze in detail the behavior of the electrons when the Dirac constant tends to infinity and show that the electrons all concentrate around exactly one of the 8 nuclei of the unit cell of size 2, which is the explanation of the breaking of symmetry. Zooming at this point, the electronic density solves an effective nonlinear Schrödinger equation in the whole space with nonlinearity \(u^{7/3}-u^{4/3}\). Our results rely on the analysis of this nonlinear equation, in particular on the uniqueness and non-degeneracy of positive solutions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Bahouri, H., Gérard, P.: High frequency approximation of solutions to critical nonlinear wave equations. Am. J. Math. 121, 31–175 (1999)

    MathSciNet  MATH  Google Scholar 

  2. Bellazzini, J., Ghimenti, M.: Symmetry breaking for Schrödinger–Poisson–Slater energy? arXiv:1601.05626 (2016)

  3. Berestycki, H., Lions, P.-L.: Nonlinear scalar field equations. I. Existence of a ground state. Arch. Ration. Mech. Anal. 82, 313–345 (1983)

    Article  MathSciNet  MATH  Google Scholar 

  4. Bokanowski, O., Grebert, B., Mauser, N.J.: Local density approximations for the energy of a periodic Coulomb model. Math. Models Methods Appl. Sci. 13, 1185–1217 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  5. Bokanowski, O., Mauser, N.J.: Local approximation for the Hartree–Fock exchange potential: a deformation approach. Math. Models Methods Appl. Sci. 9, 941–961 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  6. Brothers, J.E., Ziemer, W.P.: Minimal rearrangements of Sobolev functions. J. Reine Angew. Math. 384, 153–179 (1988)

    MathSciNet  MATH  Google Scholar 

  7. Catto, I., Le Bris, C., Lions, P.-L.: The Mathematical Theory of Thermodynamic Limits: Thomas–Fermi Type Models. Oxford Mathematical Monographs. The Clarendon Press, Oxford University Press, New York (1998)

    MATH  Google Scholar 

  8. Chen, M., Xia, J., Huang, C., Dieterich, J.M., Hung, L., Shin, I., Carter, E.A.: Introducing PROFESS 3.0: an advanced program for orbital-free density functional theory molecular dynamics simulations. Comput. Phys. Commun. 190, 228–230 (2015)

    Article  ADS  MATH  Google Scholar 

  9. Dirac, P.A.: Note on exchange phenomena in the Thomas atom. Proc. Camb. Philos. Soc. 26, 376–385 (1930)

    Article  ADS  MATH  Google Scholar 

  10. Frank, R.L., Lenzmann, E.: Uniqueness of non-linear ground states for fractional Laplacians in \(\mathbb{R}\). Acta Math. 210, 261–318 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  11. Frank, R.L., Lenzmann, E., Silvestre, L.: Uniqueness of radial solutions for the fractional Laplacian. Commun. Pure Appl. Math. 69, 1671–1726 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  12. Frank, R.L., Lieb, E.H.: Possible lattice distortions in the Hubbard model for graphene. Phys. Rev. Lett. 107, 066801 (2011)

    Article  ADS  Google Scholar 

  13. Friesecke, G.: Pair correlations and exchange phenomena in the free electron gas. Commun. Math. Phys. 184, 143–171 (1997)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  14. Fröhlich, H.: On the theory of superconductivity: the one-dimensional case. Proc. R. Soc. Lond. A 223, 296–305 (1954)

    Article  ADS  MATH  Google Scholar 

  15. Garcia Arroyo, M., Séré, E.: Existence of kink solutions in a discrete model of the polyacetylene molecule. Working paper or preprint (2012)

  16. Gérard, P.: Description du défaut de compacité de l’injection de Sobolev. ESAIM Control Optim. Calc. Var. 3, 213–233 (1998)

    Article  MathSciNet  Google Scholar 

  17. Gidas, B., Ni, W.M., Nirenberg, L.: Symmetry of positive solutions of nonlinear elliptic equations in \({\mathbb{R}}^N\). Adv. Math. Suppl. Stud. A 7, 369–402 (1981)

    MATH  Google Scholar 

  18. Graf, G.M., Solovej, J.P.: A correlation estimate with applications to quantum systems with Coulomb interactions. Rev. Math. Phys. 6, 977–997 (1994). Special issue dedicated to Elliott H. Lieb

    Article  MathSciNet  MATH  Google Scholar 

  19. Grillakis, M., Shatah, J., Strauss, W.: Stability theory of solitary waves in the presence ofsymmetry. I. J. Funct. Anal. 74, 160–197 (1987)

    Article  MathSciNet  MATH  Google Scholar 

  20. Hmidi, T., Keraani, S.: Blowup theory for the critical nonlinear Schrödinger equations revisited. Int. Math. Res. Not. 2005, 2815–2828 (2005)

    Article  MATH  Google Scholar 

  21. Johnson, R.A.: Empirical potentials and their use in the calculation of energies of point defects in metals. J. Phys. F Met. Phys. 3, 295 (1973)

    Article  ADS  Google Scholar 

  22. Kennedy, T., Lieb, E.H.: An itinerant electron model with crystalline or magnetic long range order. Phys. A 138, 320–358 (1986)

    Article  MathSciNet  MATH  Google Scholar 

  23. Kennedy, T., Lieb, E.H.: Proof of the Peierls instability in one dimension. Phys. Rev. Lett. 59, 1309–1312 (1987)

    Article  ADS  MathSciNet  Google Scholar 

  24. Killip, R., Oh, T., Pocovnicu, O., Vişan, M.: Solitons and scattering for the cubic-quintic nonlinear Schrödinger equation on \(\mathbb{R}^3\). Arch. Ration. Mech. Anal. 225, 469–548 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  25. Killip, R., Vişan, M.: Nonlinear Schrödinger Equations at Critical Regularity. Lecture Notes for the Summer School of Clay Mathematics Institute (2008)

  26. Kin-Lic Chan, G., Handy, N.C.: Optimized Lieb–Oxford bound for the exchange-correlation energy. Phys. Rev. A 59, 3075–3077 (1999)

    Article  ADS  Google Scholar 

  27. Le Bris, C.: Quelques problèmes mathématiques en chimie quantique moléculaire. Ph.D. thesis, École Polytechnique (1993)

  28. Lenzmann, E.: Uniqueness of ground states for pseudorelativistic Hartree equations. Anal. PDE 2, 1–27 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  29. Levy, M., Perdew, J.P.: Tight bound and convexity constraint on the exchange-correlation-energy functional in the low-density limit, and other formal tests of generalized-gradient approximations. Phys. Rev. B 48, 11638–11645 (1993)

    Article  ADS  Google Scholar 

  30. Lewin, M.: Variational methods in quantum mechanics. Unpublished lecture notes (University of Cergy-Pontoise) (2010)

  31. Lewin, M., Lieb, E.H.: Improved Lieb–Oxford exchange-correlation inequality with a gradient correction. Phys. Rev. A 91, 022507 (2015)

    Article  ADS  MathSciNet  Google Scholar 

  32. Lewin, M., Rota Nodari, S.: Uniqueness and non-degeneracy for a nuclear nonlinear Schrödinger equation. Nonlinear Differ. Equ. Appl. 22, 673–698 (2015)

    Article  MATH  Google Scholar 

  33. Li, C.: Monotonicity and symmetry of solutions of fully nonlinear elliptic equations on unbounded domains. Commun. Partial Differ. Equ. 16, 585–615 (1991)

    Article  MathSciNet  MATH  Google Scholar 

  34. Lieb, E.H.: Existence and uniqueness of the minimizing solution of Choquard’s nonlinear equation. Stud. Appl. Math. 57, 93–105 (1976/1977)

  35. Lieb, E.H.: A lower bound for Coulomb energies. Phys. Lett. A 70, 444–446 (1979)

    Article  ADS  MathSciNet  Google Scholar 

  36. Lieb, E.H.: Thomas–Fermi and related theories of atoms and molecules. Rev. Mod. Phys. 53, 603–641 (1981)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  37. Lieb, E.H.: A model for crystallization: a variation on the Hubbard model. Phys. A 140, 240–250 (1986). Statphys 16 (Boston, MA, 1986)

    Article  MathSciNet  MATH  Google Scholar 

  38. Lieb, E.H., Loss, M.: Analysis. Vol. 14 of Graduate Studies in Mathematics, 2nd edn. American Mathematical Society, Providence, RI (2001)

    MATH  Google Scholar 

  39. Lieb, E.H., Nachtergaele, B.: Dimerization in ring-shaped molecules: the stability of the Peierls instability. In: XIth International Congress of Mathematical Physics (Paris, 1994), pp. 423–431. International Press, Cambridge, MA (1995)

  40. Lieb, E.H., Nachtergaele, B.: Stability of the Peierls instability for ring-shaped molecules. Phys. Rev. B 51, 4777 (1995)

    Article  ADS  MATH  Google Scholar 

  41. Lieb, E.H., Nachtergaele, B.: Bond alternation in ring-shaped molecules: the stability of the Peierls instability. Int. J. Quantum Chem. 58, 699–706 (1996)

    Article  Google Scholar 

  42. Lieb, E.H., Oxford, S.: Improved lower bound on the indirect Coulomb energy. Int. J. Quantum Chem. 19, 427–439 (1980)

    Article  Google Scholar 

  43. Lieb, E.H., Seiringer, R.: The Stability of Matter in Quantum Mechanics. Cambridge University Press, Cambridge (2010)

    MATH  Google Scholar 

  44. Lieb, E.H., Simon, B.: The Thomas–Fermi theory of atoms, molecules and solids. Adv. Math. 23, 22–116 (1977)

    Article  MathSciNet  MATH  Google Scholar 

  45. Lions, P.-L.: The concentration-compactness principle in the calculus of variations. The locally compact case. II. Ann. Inst. Henri Poincaré Anal. Non Linéaire 1, 223–283 (1984)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  46. Lions, P.-L.: Solutions of Hartree–Fock equations for Coulomb systems. Commun. Math. Phys. 109, 33–97 (1987)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  47. Morrey Jr., C.B.: On the analyticity of the solutions of analytic non-linear elliptic systems of partial differential equations. I. Analyticity in the interior. Am. J. Math. 80, 198–218 (1958)

    Article  MathSciNet  MATH  Google Scholar 

  48. Nam, P.T., Van Den Bosch, H.: Nonexistence in Thomas–Fermi–Dirac–von Weizsäcker theory with small nuclear charges. Math. Phys. Anal. Geom. 20, 6 (2017)

    Article  MATH  Google Scholar 

  49. Peierls, R.E.: Quantum Theory of Solids. Clarendon Press, Oxford (1955)

    MATH  Google Scholar 

  50. Perdew, J.P.: Unified theory of exchange and correlation beyond the local density approximation. In: Ziesche, P., Eschrig, H. (eds.) Electronic Structure of Solids ’91, pp. 11–20. Akademie Verlag, Berlin (1991)

    Google Scholar 

  51. Perdew, J.P., Burke, K., Ernzerhof, M.: Generalized gradient approximation made simple. Phys. Rev. Lett. 77, 3865–3868 (1996)

    Article  ADS  Google Scholar 

  52. Perdew, J.P., Wang, Y.: Accurate and simple analytic representation of the electron-gas correlation energy. Phys. Rev. B 45, 13244–13249 (1992)

    Article  ADS  Google Scholar 

  53. Prodan, E.: Symmetry breaking in the self-consistent Kohn–Sham equations. J. Phys. A 38, 5647–5657 (2005)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  54. Prodan, E., Nordlander, P.: Hartree approximation. III. Symmetry breaking. J. Math. Phys. 42, 3424–3438 (2001)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  55. Ricaud, J.: On uniqueness and non-degeneracy of anisotropic polarons. Nonlinearity 29, 1507–1536 (2016)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  56. Ricaud, J.: Symétrie et brisure de symétrie pour certains problèmes non linéaires. Ph.D. thesis, Université de Cergy-Pontoise (2017)

  57. Seiringer, R.: A correlation estimate for quantum many-body systems at positive temperature. Rev. Math. Phys. 18, 233–253 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  58. Serrin, J., Tang, M.: Uniqueness of ground states for quasilinear elliptic equations. Indiana Univ. Math. J. 49, 897–923 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  59. Sherrill, C.D., Lee, M.S., Head-Gordon, M.: On the performance of density functional theory for symmetry-breaking problems. Chem. Phys. Lett. 302, 425–430 (1999)

    Article  ADS  Google Scholar 

  60. Tod, P., Moroz, I.M.: An analytical approach to the Schrödinger–Newton equations. Nonlinearity 12, 201–216 (1999)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  61. Weinstein, M.I.: Modulational stability of ground states of nonlinear Schrödinger equations. SIAM J. Math. Anal. 16, 472–491 (1985)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Département de Mathématiques, CNRS, Université de Cergy-Pontoise, 95000, Cergy-Pontoise, France

    Julien Ricaud

  2. CNRS, Ceremade, Université Paris-Dauphine, PSL Research University, 75016, Paris, France

    Julien Ricaud

Authors
  1. Julien Ricaud
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Julien Ricaud.

Additional information

Communicated by Nader Masmoudi.

The author is grateful to Mathieu Lewin for helpful discussions and advices, and to Enno Lenzmann for bringing our attention to the facts mentioned in the first remark after Conjecture 7. The author acknowledges financial support from the European Research Council under the European Community’s Seventh Framework Program (FP7/2007-2013 Grant Agreement MNIQS 258023).

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ricaud, J. Symmetry Breaking in the Periodic Thomas–Fermi–Dirac–von Weizsäcker Model. Ann. Henri Poincaré 19, 3129–3177 (2018). https://doi.org/10.1007/s00023-018-0711-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00023-018-0711-5

Search

Navigation